| Klimeschia lutumella | |
|---|---|
Scientific classification  | |
| Domain: | Eukaryota |
| Kingdom: | Animalia |
| Phylum: | Arthropoda |
| Class: | Insecta |
| Order: | Lepidoptera |
| Family: | Douglasiidae |
| Genus: | Klimeschia |
| Species: | K. lutumella |
| Binomial name | |
| Klimeschia lutumella Amsel, 1938 | |
Klimeschia lutumella is a moth in the family Douglasiidae. It was described by Hans Georg Amsel in 1938. It is found in Israel. [1]
In calculus, the chain rule is a formula that expresses the derivative of the composition of two differentiable functions f and g in terms of the derivatives of f and g. More precisely, if is the function such that for every x, then the chain rule is, in Lagrange's notation, or, equivalently,
In mathematics, convolution is a mathematical operation on two functions that produces a third function. The term convolution refers to both the result function and to the process of computing it. It is defined as the integral of the product of the two functions after one is reflected about the y-axis and shifted. The integral is evaluated for all values of shift, producing the convolution function. The choice of which function is reflected and shifted before the integral does not change the integral result. Graphically, it expresses how the 'shape' of one function is modified by the other.
In physics, the kinetic energy of an object is the form of energy that it possesses due to its motion.
The tiger is a large cat and a member of the genus Panthera native to Asia. It has a powerful, muscular body with a large head and paws, a long tail and orange fur with black, mostly vertical stripes. It is traditionally classified into nine recent subspecies, though some recognise only two subspecies, mainland Asian tigers and the island tigers of the Sunda Islands.
In mathematical analysis, the Dirac delta function, also known as the unit impulse, is a generalized function on the real numbers, whose value is zero everywhere except at zero, and whose integral over the entire real line is equal to one. Thus it can be represented heuristically as
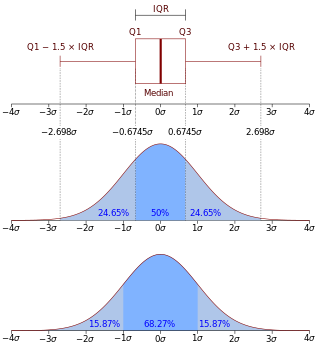
In probability theory, a probability density function (PDF), density function, or density of an absolutely continuous random variable, is a function whose value at any given sample in the sample space can be interpreted as providing a relative likelihood that the value of the random variable would be equal to that sample. Probability density is the probability per unit length, in other words, while the absolute likelihood for a continuous random variable to take on any particular value is 0, the value of the PDF at two different samples can be used to infer, in any particular draw of the random variable, how much more likely it is that the random variable would be close to one sample compared to the other sample.
Big O notation is a mathematical notation that describes the limiting behavior of a function when the argument tends towards a particular value or infinity. Big O is a member of a family of notations invented by German mathematicians Paul Bachmann, Edmund Landau, and others, collectively called Bachmann–Landau notation or asymptotic notation. The letter O was chosen by Bachmann to stand for Ordnung, meaning the order of approximation.
In physics, engineering and mathematics, the Fourier transform (FT) is an integral transform that takes a function as input and outputs another function that describes the extent to which various frequencies are present in the original function. The output of the transform is a complex-valued function of frequency. The term Fourier transform refers to both this complex-valued function and the mathematical operation. When a distinction needs to be made, the output of the operation is sometimes called the frequency domain representation of the original function. The Fourier transform is analogous to decomposing the sound of a musical chord into the intensities of its constituent pitches.
The orbital period is the amount of time a given astronomical object takes to complete one orbit around another object. In astronomy, it usually applies to planets or asteroids orbiting the Sun, moons orbiting planets, exoplanets orbiting other stars, or binary stars. It may also refer to the time it takes a satellite orbiting a planet or moon to complete one orbit.
In chemistry, the molar mass of a chemical compound is defined as the ratio between the mass and the amount of substance of any sample of the compound. The molar mass is a bulk, not molecular, property of a substance. The molar mass is an average of many instances of the compound, which often vary in mass due to the presence of isotopes. Most commonly, the molar mass is computed from the standard atomic weights and is thus a terrestrial average and a function of the relative abundance of the isotopes of the constituent atoms on Earth. The molar mass is appropriate for converting between the mass of a substance and the amount of a substance for bulk quantities.
In mathematics, a function from a set X to a set Y assigns to each element of X exactly one element of Y. The set X is called the domain of the function and the set Y is called the codomain of the function.
In thermodynamics, the Gibbs free energy is a thermodynamic potential that can be used to calculate the maximum amount of work, other than pressure-volume work, that may be performed by a thermodynamically closed system at constant temperature and pressure. It also provides a necessary condition for processes such as chemical reactions that may occur under these conditions. The Gibbs free energy is expressed asWhere:
In signal processing, cross-correlation is a measure of similarity of two series as a function of the displacement of one relative to the other. This is also known as a sliding dot product or sliding inner-product. It is commonly used for searching a long signal for a shorter, known feature. It has applications in pattern recognition, single particle analysis, electron tomography, averaging, cryptanalysis, and neurophysiology. The cross-correlation is similar in nature to the convolution of two functions. In an autocorrelation, which is the cross-correlation of a signal with itself, there will always be a peak at a lag of zero, and its size will be the signal energy.
Douglasiidae is a small Lepidopteran family including around 28 species of micromoth whose adults are collectively called Douglas moths. The largest genus in the family is Tinagma. They are primarily found in the Palearctic and Nearctic realms. The adults have a 6 to 15 mm wingspan, with a reduced hindwing venation and long fringes. The larvae are leaf miners or borers, primarily in stems and petioles, belonging to Boraginaceae, Labiatae, and Rosaceae.
Klimeschia is a genus of moths in the family Douglasiidae. It is found in the Palearctic realm.
Klimeschia afghanica is a moth in the family Douglasiidae. It was described by Reinhard Gaedike in 1974. It is found in Afghanistan, Iran and Tuva, Russia.
Klimeschia paghmanella is a moth in the family Douglasiidae. It was described by Reinhard Gaedike in 1974. It is found in Afghanistan.
Klimeschia thymetella is a moth in the family Douglasiidae. It was described by Otto Staudinger in 1859. It is found in Portugal and Spain.
Klimeschia transversella is a moth in the family Douglasiidae. It was described by Zeller in 1839. It is found in Spain, Portugal, Italy, France, Belgium, Germany, Poland, Denmark, Austria, the Czech Republic, Slovakia, Croatia, Bosnia and Herzegovina, Hungary, Romania, North Macedonia, Greece, Finland, Sweden, Belarus, the Baltic region and Russia.
Klimeschia vibratoriella is a moth in the family Douglasiidae. It was described by Josef Johann Mann in 1862. It is found in Turkey.
 | This article relating to the superfamily Gracillarioidea is a stub. You can help Wikipedia by expanding it. |