Op amp integrator

The operational amplifier integrator is an electronic integration circuit. Based on the operational amplifier (op-amp), it performs the mathematical operation of integration with respect to time; that is, its output voltage is proportional to the input voltage integrated over time.

Applications

The integrator circuit is mostly used in analog computers, analog-to-digital converters and wave-shaping circuits. A common wave-shaping use is as a charge amplifier and they are usually constructed using an operational amplifier though they can use high gain discrete transistor configurations.

Design

The input current is offset by a negative feedback current flowing in the capacitor, which is generated by an increase in output voltage of the amplifier. The output voltage is therefore dependent on the value of input current it has to offset and the inverse of the value of the feedback capacitor. The greater the capacitor value, the less output voltage has to be generated to produce a particular feedback current flow.

The input capacitance of the circuit is almost zero because of the Miller effect. This ensures that the stray capacitances (the cable capacitance, the amplifier input capacitance, etc.) are virtually grounded and have no influence on the output signal. ^[1]

Ideal circuit

This circuit operates by passing a current that charges or discharges the capacitor ${\displaystyle C_{\text{F}}}$ during the time under consideration, which strives to retain the virtual ground condition at the input by off-setting the effect of the input current:

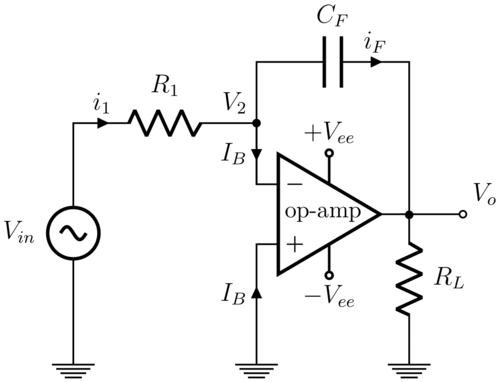
Referring to the above diagram, if the op-amp is assumed to be ideal, then the voltage at the inverting (-) input is held equal to the voltage at the non-inverting (+) input as a virtual ground. The input voltage passes a current ${\displaystyle V_{\text{in}}/{R_{1}}}$ through the resistor producing a compensating current flow through the series capacitor to maintain the virtual ground. This charges or discharges the capacitor over time. Because the resistor and capacitor are connected to a virtual ground, the input current does not vary with capacitor charge, so a linear integration that works across all frequencies is achieved (unlike RC circuit § Integrator).

The circuit can be analyzed by applying Kirchhoff's current law at the inverting input:

${\displaystyle i_{\text{1}}=I_{\text{B}}+i_{\text{F}}}$

For an ideal op-amp, ${\displaystyle I_{\text{B}}=0}$ amps, so:

${\displaystyle i_{\text{1}}=i_{\text{F}}}$

Furthermore, the capacitor has a voltage-current relationship governed by the equation:

${\displaystyle i_{\text{F}}=C_{\text{F}}{\frac {d(V_{\text{2}}-V_{\text{o}})}{dt}}}$

Substituting the appropriate variables:

${\displaystyle {\frac {V_{\text{in}}-V_{\text{2}}}{R_{\text{1}}}}=C_{\text{F}}{\frac {d(V_{\text{2}}-V_{\text{o}})}{dt}}}$

For an ideal op-amp, ${\displaystyle V_{2}=0}$ volts, so:

${\displaystyle {\frac {V_{\text{in}}}{R_{\text{1}}}}=-C_{\text{F}}{\frac {dV_{\text{o}}}{dt}}}$

Integrating both sides with respect to time:

${\displaystyle \int _{0}^{t}{\frac {V_{\text{in}}}{R_{\text{1}}}}\ dt\ =-\int _{0}^{t}C_{\text{F}}{\frac {dV_{\text{o}}}{dt}}\,dt}$

If the initial value of ${\displaystyle V_{\text{o}}}$ is assumed to be 0 volts, the output voltage will simply be proportional to the integral of the input voltage: ^[2]

${\displaystyle V_{\text{o}}=-{\frac {1}{R_{\text{1}}C_{\text{F}}}}\int _{0}^{t}V_{\text{in}}\,dt}$

Practical circuit

This practical integrator attempts to address a number of flaws of the ideal integrator circuit:

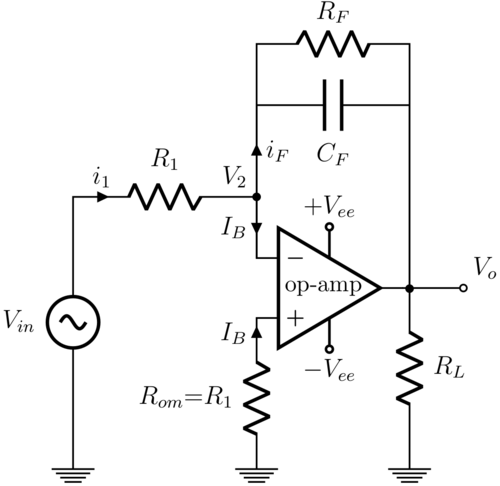
Real op-amps have a finite open-loop gain, an input offset voltage ${\displaystyle (V_{\text{OS}})}$ and input bias currents ${\displaystyle (I_{\text{B}})}$, which may not be well-matched and may be distinguished as ${\displaystyle I_{\text{B-}}}$ going into the inverting input and ${\displaystyle I_{\text{B+}}}$ going into the non-inverting input. This can cause several issues for the ideal design; most importantly, if ${\displaystyle V_{\text{in}}=0}$, both the output offset voltage and the input bias current ${\displaystyle I_{\text{B-}}}$ can cause current to pass through the capacitor, causing the output voltage to drift over time until the op-amp saturates. Similarly, if ${\displaystyle V_{\text{in}}}$ were a signal centered about zero volts (i.e. without a DC component), no drift would be expected in an ideal circuit, but may occur in a real circuit.

To negate the effect of the input bias current, it is necessary for the non-inverting terminal to include a resistor ${\displaystyle R_{\text{om}}=R_{1}||R_{\text{F}}||R_{\text{L}},}$ which simplifies to ${\displaystyle R_{1}}$ provided that ${\displaystyle R_{1}}$ is much smaller than the load resistance ${\displaystyle R_{L}}$ and the feedback resistance ${\displaystyle R_{F}}$. Well-matched input bias currents then cause the same voltage drop of ${\displaystyle R_{1}I_{\text{B}}}$ at both the inverting and non-inverting terminals, to effectively cancel out the effect of bias current at those inputs.

Also, in a DC steady state, an ideal capacitor acts as an open circuit. The DC gain of the ideal circuit is therefore infinite (or in practice, the open-loop gain of a non-ideal op-amp). Any DC (or very low frequency) component may then cause the op amp output to drift into saturation. ^[3] To prevent this, the DC gain can be limited to a finite value by inserting a large resistor ${\displaystyle R_{\text{F}}}$ in parallel with the feedback capacitor. Note that some op amps have a large internal feedback resistor, and many real capacitors have leakage that is effectively a large feedback resistor. ^[4]

The addition of these resistors turns the output drift into a finite, preferably small, DC error voltage:

${\displaystyle V_{\text{error}}=\left({\frac {R_{\text{F}}}{R_{1}}}+1\right)\left(V_{\text{OS}}+I_{\text{B-}}\left(R_{\text{F}}\parallel R_{1}\right)\right).}$

Notes on offset: a variation of this circuit simply uses an adjustable voltage source instead of ${\displaystyle R_{\text{om}}}$ and some op amps with very low offset voltage may not even require offset correction. ^[5] Offset correction is a bigger concern for older op amps, particularly BJT types. Another variation circuit to avoid offset correction that works for AC signals only is to capacitively-couple the input with large input capacitor before ${\displaystyle R_{1}}$which will naturally charge up to the offset voltage. Additionally, because offset may drift over time and temperature, some op amps provide null offset pins, which can be connected to a potentiometer whose wiper connects to the negative supply to allow readjusting when conditions change. These methods may be combined. ^[4]

Frequency response

Both the ideal and practical integrator have a gain of 1 at a single frequency called the unity gain frequency ${\displaystyle f_{\text{0dB}}}$:

${\displaystyle f_{\text{0dB}}={\frac {1}{{2\pi }{R_{\text{1}}}{C_{\text{F}}}}}\,.}$

But the overall frequency response of the two circuits differ due to their different pole locations.

Ideal integrator

The ideal integrator's transfer function ${\displaystyle {\tfrac {1}{s}}}$ corresponds to the time-domain integration property of the Laplace transform. Since its denominator is just ${\displaystyle s}$, the transfer function has a pole frequency at ${\displaystyle f{=}0}$. Thus its frequency response has a steady -20 dB per decade slope across all frequencies and appears as a downward-sloping line in a Bode plot.

Practical integrator

The practical integrator's feedback resistor ${\displaystyle R_{F}}$ in parallel with the feedback capacitor ${\displaystyle C_{\text{F}}}$ turns the circuit into an active low-pass filter with a pole at the -3 dB cutoff frequency:

${\displaystyle f_{\text{cutoff}}={\frac {1}{{2\pi }{R_{\text{F}}}{C_{\text{F}}}}}\,.}$

The frequency response has a relatively constant gain up to ${\displaystyle f_{\text{cutoff}}}$, and then decreases by 20 dB per decade. While this circuit is no longer an integrator for low frequencies around and below ${\displaystyle f_{\text{cutoff}}}$, the error is decreases to only 0.5% at one decade above ${\displaystyle f_{\text{cutoff}}}$ and the response approaches that of an ideal integrator as the frequency increases. ^[3] Real op amps also have a limited gain-bandwidth product (GBWP), which adds an additional high frequency pole. Integration only occurs along the -20 dB per decade slope, which is steady only from frequencies about a decade above ${\displaystyle f_{\text{cutoff}}}$ to about a decade below the op amp's GBWP. ^[5]

References

1. Transducers with Charge Output
2. "AN1177 Op Amp Precision Design: DC Errors" (PDF). Microchip. 2 January 2008. Archived (PDF) from the original on 2019-07-09. Retrieved 26 December 2012.
3. Stata, Ray (1967). "Operational Integrators" (PDF). Analog Dialogue . pp. 10–11. Archived (PDF) from the original on 2020-11-12. Retrieved 2024-02-16.
4. Pavlic, Theodore (2009) [2007]. "Practical Integrators and Operational Amplifier Offset - Practical Integrators and Operational Amplifier Offset - ECE 327: Electronic Devices and Circuits Laboratory I" (PDF). Archived (PDF) from the original on 2022-10-11. Retrieved 2023-08-20.
5. "Analog Engineer's Circuit: Amplifiers - SBOA275A" (PDF). Texas Instruments . 2019 [2018]. Archived (PDF) from the original on 2022-09-01. Retrieved 2023-08-20.