| Szilassi polyhedron | |
|---|---|
 | |
| Type | Toroidal polyhedron |
| Faces | 7 hexagons |
| Edges | 21 |
| Vertices | 14 |
| Euler char. | 0 (Genus 1) |
| Vertex configuration | 6.6.6 |
| Symmetry group | C1, [ ]+, (11) |
| Dual polyhedron | Császár polyhedron |
| Properties | Non-convex |
In geometry, the Szilassi polyhedron is a nonconvex polyhedron, topologically a torus, with seven hexagonal faces.
The 14 vertices and 21 edges of the Szilassi polyhedron form an embedding of the Heawood graph onto the surface of a torus. [1] Each face of this polyhedron shares an edge with each other face. As a result, it requires seven colours to colour all adjacent faces. This example shows that, on surfaces topologically equivalent to a torus, some subdivisions require seven colors, providing the lower bound for the seven colour theorem. The other half of the theorem states that all toroidal subdivisions can be colored with seven or fewer colors.
The Szilassi polyhedron has an axis of 180-degree symmetry. This symmetry swaps three pairs of congruent faces, leaving one unpaired hexagon that has the same rotational symmetry as the polyhedron.
The tetrahedron and the Szilassi polyhedron are the only two known polyhedra in which each face shares an edge with each other face.
If a polyhedron with f faces is embedded onto a surface with h holes, in such a way that each face shares an edge with each other face, it follows by some manipulation of the Euler characteristic that
This equation is satisfied for the tetrahedron with h = 0 and f = 4, and for the Szilassi polyhedron with h = 1 and f = 7.
The next possible solution, h = 6 and f = 12, would correspond to a polyhedron with 44 vertices and 66 edges. However, it is not known whether such a polyhedron can be realized geometrically without self-crossings (rather than as an abstract polytope). More generally this equation can be satisfied precisely when f is congruent to 0, 3, 4, or 7 modulo 12. [2] [3]
The Szilassi polyhedron is named after Hungarian mathematician Lajos Szilassi, who discovered it in 1977. [4] [1] The dual to the Szilassi polyhedron, the Császár polyhedron, was discovered earlier by ÁkosCsászár ( 1949 ); it has seven vertices, 21 edges connecting every pair of vertices, and 14 triangular faces. Like the Szilassi polyhedron, the Császár polyhedron has the topology of a torus. [5]
In geometry, a dodecahedron or duodecahedron is any polyhedron with twelve flat faces. The most familiar dodecahedron is the regular dodecahedron with regular pentagons as faces, which is a Platonic solid. There are also three regular star dodecahedra, which are constructed as stellations of the convex form. All of these have icosahedral symmetry, order 120.
In geometry, every polyhedron is associated with a second dual structure, where the vertices of one correspond to the faces of the other, and the edges between pairs of vertices of one correspond to the edges between pairs of faces of the other. Such dual figures remain combinatorial or abstract polyhedra, but not all can also be constructed as geometric polyhedra. Starting with any given polyhedron, the dual of its dual is the original polyhedron.
In geometry, an octahedron is a polyhedron with eight faces. One special case is the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex. Regular octahedra occur in nature as crystal structures. Many types of irregular octahedra also exist, including both convex and non-convex shapes.
In geometry, a polyhedron is a three-dimensional figure with flat polygonal faces, straight edges and sharp corners or vertices.
In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent regular polygons, and the same number of faces meet at each vertex. There are only five such polyhedra:
In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 equilateral triangle faces, 12 vertices and 18 edges. It can be constructed by truncating all 4 vertices of a regular tetrahedron.
In geometry, the truncated cube, or truncated hexahedron, is an Archimedean solid. It has 14 regular faces, 36 edges, and 24 vertices.
The triaugmented triangular prism, in geometry, is a convex polyhedron with 14 equilateral triangles as its faces. It can be constructed from a triangular prism by attaching equilateral square pyramids to each of its three square faces. The same shape is also called the tetrakis triangular prism, tricapped trigonal prism, tetracaidecadeltahedron, or tetrakaidecadeltahedron; these last names mean a polyhedron with 14 triangular faces. It is an example of a deltahedron, composite polyhedron, and Johnson solid.
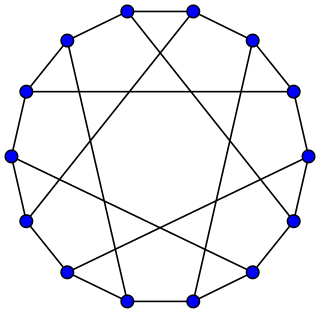
In the mathematical field of graph theory, the Heawood graph is an undirected graph with 14 vertices and 21 edges, named after Percy John Heawood.
In geometry, a truncated 5-cell is a uniform 4-polytope formed as the truncation of the regular 5-cell.
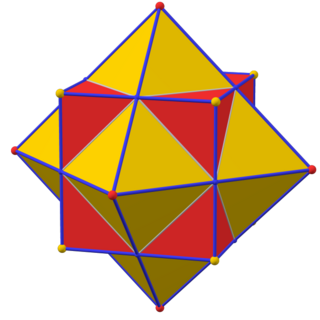
In geometry, the octahemioctahedron or allelotetratetrahedron is a nonconvex uniform polyhedron, indexed as U3. It has 12 faces (8 triangles and 4 hexagons), 24 edges and 12 vertices. Its vertex figure is a crossed quadrilateral.
In geometry and topology, Conway polyhedron notation, invented by John Horton Conway and promoted by George W. Hart, is used to describe polyhedra based on a seed polyhedron modified by various prefix operations.
A heptahedron is a polyhedron having seven sides, or faces.
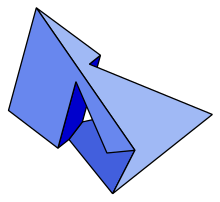
In geometry, the Császár polyhedron is a nonconvex toroidal polyhedron with 14 triangular faces.
14 (fourteen) is the natural number following 13 and preceding 15.
In mathematics, a regular map is a symmetric tessellation of a closed surface. More precisely, a regular map is a decomposition of a two-dimensional manifold into topological disks such that every flag can be transformed into any other flag by a symmetry of the decomposition. Regular maps are, in a sense, topological generalizations of Platonic solids. The theory of maps and their classification is related to the theory of Riemann surfaces, hyperbolic geometry, and Galois theory. Regular maps are classified according to either: the genus and orientability of the supporting surface, the underlying graph, or the automorphism group.
In geometry, a toroidal polyhedron is a polyhedron which is also a toroid, having a topological genus of 1 or greater. Notable examples include the Császár and Szilassi polyhedra.
In geometry, chamfering or edge-truncation is a topological operator that modifies one polyhedron into another. It is similar to expansion: it moves the faces apart (outward), and adds a new face between each two adjacent faces; but contrary to expansion, it maintains the original vertices. For a polyhedron, this operation adds a new hexagonal face in place of each original edge.
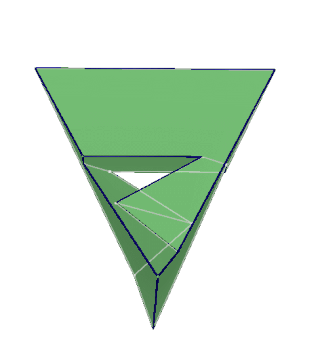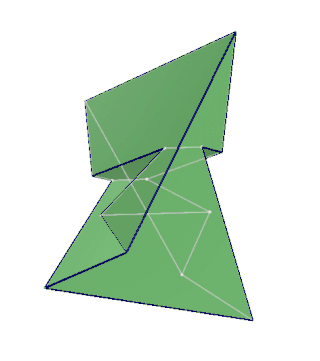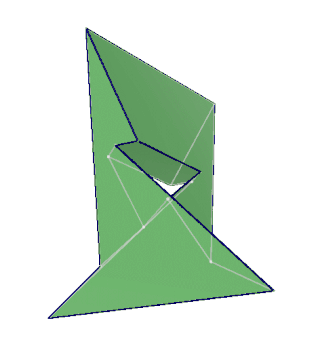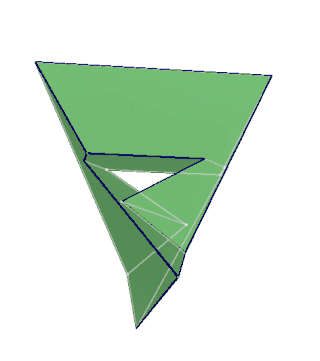
Lajos Szilassi was a professor of mathematics at the University of Szeged who worked in projective and non-Euclidean geometry, applying his research to computer generated solutions of geometric problems.
Adventures Among the Toroids: A study of orientable polyhedra with regular faces is a book on toroidal polyhedra that have regular polygons as their faces. It was written, hand-lettered, and illustrated by mathematician Bonnie Stewart, and self-published under the imprint "Number One Tall Search Book" in 1970. Stewart put out a second edition, again hand-lettered and self-published, in 1980. Although out of print, the Basic Library List Committee of the Mathematical Association of America has recommended its inclusion in undergraduate mathematics libraries.
