Evolutionary landscape

Last updated

An evolutionary landscape is a metaphor [1] or a construct used to think about and visualize the processes of evolution (e.g. natural selection and genetic drift) acting on a biological entity [2] (e.g. a gene, protein, population, or species). [3] This entity can be viewed as searching or moving through a search space. For example, the search space of a gene would be all possible nucleotide sequences. The search space is only part of an evolutionary landscape. The final component is the "y-axis", which is usually fitness. Each value along the search space can result in a high or low fitness for the entity. [1] If small movements through search space cause changes in fitness that are relatively small, then the landscape is considered smooth. Smooth landscapes happen when most fixed mutations have little to no effect on fitness, which is what one would expect with the neutral theory of molecular evolution. In contrast, if small movements result in large changes in fitness, then the landscape is said to be rugged. [1] In either case, movement tends to be toward areas of higher fitness, though usually not the global optima.

Contents

What exactly constitutes an "evolutionary landscape" is frequently confused in the literature; the term is often used interchangeably with "adaptive landscape" and "fitness landscape", although some authors have different definitions of adaptive and fitness landscapes. Additionally, there is a large disagreement whether the concept of an evolutionary landscape should be used as a visual metaphor disconnected from the underlying math, a tool for evaluating models of evolution, or a model in and of itself used to generate hypotheses and predictions.

History

Pre-Wright

According to McCoy (1979), the first evolutionary landscape was presented by Armand Janet of Toulon, France, in 1895. [4] In Janet's evolutionary landscape, a species is represented as a point or an area on a polydimensional surface of phenotypes, which is reduced to two dimensions for simplicity. The size of the population is proportional to the amount of variation within the population. [5] Natural selection (the influence of the exterior features) is represented by a vector. Unlike the evolutionary landscapes of those who would follow, in Janet's concept, natural selection pulls species toward the minima instead of the maxima. This is because the y-axis does not represent fitness but stability. [4] One important aspect of Janet's evolutionary landscape (versus Wright's) is that the landscape changes as the environment changes. [5]

Wrightian landscapes

Credit for the first evolutionary landscape typically goes to Sewall Wright, and his idea has arguably had a much larger audience and greater influence on the field of evolutionary biology than any other comparable understanding of "evolutionary landscape". [5] In his 1932 paper, Wright presents the concept of an evolutionary landscape composed of a polydimensional array of gene (allele) or genotype frequencies and an axis of fitness, which served as a visual metaphor to explain his shifting balance theory. Similarly to Janet, Wright felt the landscape could be reduced to two dimensions for simplicity. (This is one of the greatest criticisms, which are discussed below.) Populations are represented by areas, with the size of the area corresponding to the amount of genetic diversity within the population. Natural selection drives populations toward maxima, while drift represents wandering and could potentially cause a peak shift. Movement across the landscape represented changes in gene frequencies. [1] This landscape was represented as a series of contour lines, much like a topographical map; while selection kept or moved a biological entity to a peak, genetic drift allowed different peaks to be explored. [1]

In 1944, Simpson expanded Wright's landscape to include phenotypes. [6] In Simpson's model, the landscape is a means of visualizing the "relationship between selection, structure, and adaptation." [5] [6] Unlike Wright, Simpson used the landscape to represent both natural selection and genetic drift. Uphill movements are due to positive selection, and downhill movements are due to negative selection. The size and shape of a peak indicated the relative specificity of selection; i.e. a sharp and high peak indicates highly specific selection. Another difference between Simpson's and Wright's landscapes is the level at which evolution is acting. For Wright, a population geneticist, only populations of a species were shown. In Simpson's figures, the circles drawn represent all of Equidae. [6] The most important difference is that in Simpson's model, the landscape could vary through time, while in Wright's model, the landscape was static. Wright reviewed Simpson's work (Tempo and Mode in Evolution) and did not object to Simpson's use of evolutionary landscapes. [5] In later writings, Simpson referred to peaks as adaptive zones. [7]

In a series of papers, Russell Lande developed a mathematical model for Simpson's phenotypic landscape. [7] [8] Lande reconciled Wright's population-level view with Simpson's use of higher taxonomic levels. [7] Lande considers fitness peaks to be determined by the environment and thus represent ecological niches or adaptive zones for a population. Clusters of peaks inhabited by phenotypically similar populations can be viewed as higher taxonomic levels. [7]

Molecular era

The concept of evolutionary landscapes changed once more as the modern understanding of molecular evolution emerged. It is claimed that Maynard Smith (1970) was the first to visualize protein evolution as a network of proteins one mutational step away from others. [5] However, for this to be true, there must be pathways between functional proteins. Acknowledging the work of Kimura, King, and Jukes (the neutral theory of molecular evolution), Maynard Smith realized the proteins along such pathways could have equal functionality or be neutral. In other words, not all moves in evolution are "uphill". [5] In 1984, Gillespie adapted the concept of evolutionary landscapes to nucleotide sequences and so visualized the "mutational landscape" whereby all nucleotide sequences are one mutational step away from another, [5] which is remarkably similar and yet fundamentally different from Wright's original concept. This conceptual shift, along with the development of vast computational power, has allowed evolutionary landscapes to move from being a simple visual metaphor to a working model of evolution. As one might expect, this has drawn heavy criticism and generated much research. [9]

A hypothetical evolutionary landscape. The horizontal axes represent the biological parameters being measured (i.e. component of phenotype, genotype, nucleotide sequence combinations) and the vertical axis represents fitness. This particular example is a typical evolutionary landscape with peaks (lighter areas) and valleys (darker areas) generated from a shuffled dataset concerning the lichen Physcia millegrana. EvoLandscape1.png
A hypothetical evolutionary landscape. The horizontal axes represent the biological parameters being measured (i.e. component of phenotype, genotype, nucleotide sequence combinations) and the vertical axis represents fitness. This particular example is a typical evolutionary landscape with peaks (lighter areas) and valleys (darker areas) generated from a shuffled dataset concerning the lichen Physcia millegrana .

Criticisms

One of the first criticisms (or at least difficulty) with evolutionary landscapes is their dimensionality. Wright recognized that true landscapes can have thousands of dimensions, but he also felt reducing those dimensions to two was acceptable since his point in doing so was simply to convey a complex idea. [1] As a visual metaphor, this might be a valid reduction; however, the work of Gavrilets has shown that taking the high dimensionality of evolutionary landscapes into consideration may matter. [10] In a high-dimensional framework, the peaks and valleys disappear and are replaced with hypervolume areas of high fitness and low fitness, which can be visualized as curved surfaces and holes in a three-dimensional landscape. [10] While this does not affect visualization of the landscape per se (i.e. holes are equivalent to valleys), it does affect the underlying mathematical model and the predicted outcomes.

A hypothetical evolutionary landscape. The horizontal axes represent the biological parameters being measured (i.e. component of phenotype, genotype, nucleotide sequence combinations) and the vertical axis represents fitness. This particular landscape is an exaggerated example of Gavrilets' holey landscape. EvoLandscape2.png
A hypothetical evolutionary landscape. The horizontal axes represent the biological parameters being measured (i.e. component of phenotype, genotype, nucleotide sequence combinations) and the vertical axis represents fitness. This particular landscape is an exaggerated example of Gavrilets' holey landscape.

The work of Gavrilets, along with other issues, has prompted Kaplan (2008) to propose abandoning the metaphor of evolutionary landscapes (which he calls adaptive or fitness landscapes). [10] Kaplan (2008) has six main criticisms of the metaphor: (1) it has no explanatory power; (2) it lacks a relevant mathematical model; (3) it has no heuristic role; (4) it is imprecise; (5) it confuses more than it explains; and (6) there is no longer a reason to keep thinking in 2D or 3D when we have the computational power to consider higher dimensionality. Others feel Kaplan's criticisms are not warranted because he (and others) want evolutionary landscapes to meet the standards of a mathematical model; however, the landscape metaphor is just that, a metaphor. It has heuristic value as a metaphorical tool allowing one to visualize and evaluate the common core of assumptions in an evolutionary model. [11]

While Kaplan (2008) wishes to discard the idea of landscapes all together, Massimo Pigliucci is less drastic. He acknowledges four categories of landscapes: fitness landscapes, adaptive landscapes, fitness surfaces, and morphospaces. Fitness landscapes are those similar to what Wright (1932) proposed (called adaptive and fitness landscapes below). Adaptive landscapes are the phenotypic landscapes proposed by Simpson (1944), and fitness surfaces are the phenotypic landscapes with Lande's mathematical models applied to them. Morphospaces, pioneered by Raup (1966), are phenotypic landscapes developed a priori using mathematical models onto which observed measurements are mapped. They lack a fitness axis, and are used to show the occupied areas within the potential phenotypic space. Pigliucci suggests we abandon Wrightian fitness landscapes. Adaptive landscapes and fitness surfaces can be used with caution, i.e. with the understanding that they are not phenotypic versions of Wright's original concept and that they are fraught with potentially misleading assumptions. Finally, Pigliucci calls for further research into morphospaces due to their heuristic value but also their ability to generate understandable and testable hypotheses. [12]

Types of evolutionary landscapes

Adaptive landscapes

Adaptive landscapes represent populations (of biological entities) as a single point, and the axes correspond to frequencies of alleles or genotypes and the mean population fitness. [1] [10]

Visualization of two dimensions of an NK fitness landscape. The arrows represent various mutational paths that the population could follow while evolving on the fitness landscape. Visualization of two dimensions of a NK fitness landscape.png
Visualization of two dimensions of an NK fitness landscape. The arrows represent various mutational paths that the population could follow while evolving on the fitness landscape.

Fitness landscapes

Fitness landscapes represent populations (of biological entities) as clusters of points with each point representing a unique genotype. The axes correspond to the loci of those genotypes and the resulting mean population fitness. [10]

Phenotypic landscapes

Phenotypic landscapes represent populations or species as clusters of points with each point representing a phenotype. The axes correspond to frequencies of phenotypes and the mean population fitness. [10] See the visualizations below for examples of phenotypic landscapes. Visualization of a population evolving in a static fitness landscape.gif Visualization of a population evolving in a dynamic fitness landscape.gif

Selection-weighted attraction graphing

Selection-weighted attraction graphing (SWAG) uses force-directed network graphing to visualize fitness landscapes. [13] In this visualization, genotypes are represented by nodes which are attracted to each other in proportion to the relative change in fitness between them (nodes will tend to be closer if there is stronger selection strength between them on average). Additionally, fitness values may be assigned to the z-axis to create an empirical three-dimensional model of the landscape and depict fitness peaks and valleys. Clusters in this depiction may represent local fitness peaks.

Phenotypic plasticity landscapes

Phenotypic plasticity landscapes depart from the other landscapes in that they do not use the mean population fitness. Instead, that axis represents characters (phenotypic traits) and other axes represent the underlying factors affecting the character. [10]

Epigenetic landscapes

Epigenetic landscapes are "used to describe modal developmental tendency and major deviations" with a "space of abstract variables." [10]

Morphospaces

Morphospaces also lack a dimension of fitness. Instead, their axes are mathematical models of phenotypic traits developed a priori to observational measurements. Observational measurements are then mapped onto the resulting surface to indicate areas of possible phenotypic space occupied by the species under consideration. [12]

Applications to genomics

Recent increases in computational power and ease of sequencing have allowed the concept of evolutionary landscapes to be taken from a purely conceptual metaphor to something that can be modeled and explored. This is especially true of the field of genomics. One good example is the research article "The Evolutionary Landscape of Cytosolic Microbial Sensors in Humans." [14] In their study, Vasseur et al. were interested in the evolution of the innate immune system; specifically, they wished to map the genetic diversity—the occupied evolutionary landscape—and patterns of selection and diversification—the movements made and being made along that landscape—of the NOD-like receptor (NLR) family of the pattern-recognition receptors (PRRs) that drive the innate immune response. These genes are responsible for detecting patterns/chemicals (e.g. chitin, oxidative stress) associated with invading microbes, tissue damage, and stress. To achieve this, they sequenced 21 genes from 185 humans and used several statistical methods to examine patterns of selection and diversification. The NLR family can be divided into two subfamilies—the NALP subfamily and the NOD/IPAF subfamily. The authors found that the NALP family was under strong purifying selection and exhibited low genetic and functional diversity. They hypothesize this is because these genes have vital, non-redundant roles. Evidence in favor of this hypothesis comes from the independent discovery of rare alleles with mutations in two of the genes leading to a severe inflammatory disease and pregnancy complications. The NOD/IPAF subfamily seems to have evolved under relaxed selection and exhibits a fair amount of genetic and functional diversity. The authors also found evidence of positive selection. The gene with the strongest positive selection was NLRP1, which has two haplotypes undergoing selective sweeps. The first haplotype is seven amino acids in strong linkage disequilibrium. This haplotype is global and seems to be moving toward fixation, which started in Asia and is still occurring in Europe and Africa. The second haplotype is restricted to Europe and is not in linkage disequilibrium with the global haplotype. This European haplotype carries with it a mutation associated with autoimmune diseases. The authors hypothesize another mutation in the haplotype is what selection is acting on and this deleterious mutation is simply hitchhiking along with it. [14]

The second example comes from a paper titled "Synonymous Genes Explore Different Evolutionary Landscapes." [15] The authors of this paper are broadly interested in the ability of a protein to evolve. They specifically wanted to know how synonymous substitutions affected the evolutionary landscape of a protein. To do this, they used a program called the Evolutionary Landscape Printer to design a synonymous version of the antibiotic resistance gene aac(6')-IB. A synonymous protein has the same amino acid sequence but different nucleotide sequences. Thus, a synonymous protein has the same function and fitness value but a different surrounding evolutionary landscape. Basically, this is a way to jump peaks without actually moving. [15] The landscape of the original protein and the synonymous copy were explored computationally with Monte Carlo simulations and error-prone PCR. The PCR products were then inserted into competent E. coli cells and screened for novel antibiotic resistance. They found that each of the two proteins gives rise to a very different novel phenotype that, theoretically, is unreachable from the other. From their results, the authors concluded that synonymous codons allow for a wider exploration of the local evolutionary landscape, and that the method they used increases the odds of finding an advantageous mutation, which is useful for predicting how a population might change and for designing better organisms for industry. [15]

Related Research Articles

<span class="mw-page-title-main">Natural selection</span> Mechanism of evolution by differential survival and reproduction of individuals

Natural selection is the differential survival and reproduction of individuals due to differences in phenotype. It is a key mechanism of evolution, the change in the heritable traits characteristic of a population over generations. Charles Darwin popularised the term "natural selection", contrasting it with artificial selection, which is intentional, whereas natural selection is not.

Quantum evolution is a component of George Gaylord Simpson's multi-tempoed theory of evolution proposed to explain the rapid emergence of higher taxonomic groups in the fossil record. According to Simpson, evolutionary rates differ from group to group and even among closely related lineages. These different rates of evolutionary change were designated by Simpson as bradytelic, horotelic, and tachytelic.

Molecular evolution is the process of change in the sequence composition of cellular molecules such as DNA, RNA, and proteins across generations. The field of molecular evolution uses principles of evolutionary biology and population genetics to explain patterns in these changes. Major topics in molecular evolution concern the rates and impacts of single nucleotide changes, neutral evolution vs. natural selection, origins of new genes, the genetic nature of complex traits, the genetic basis of speciation, the evolution of development, and ways that evolutionary forces influence genomic and phenotypic changes.

Population genetics is a subfield of genetics that deals with genetic differences within and among populations, and is a part of evolutionary biology. Studies in this branch of biology examine such phenomena as adaptation, speciation, and population structure.

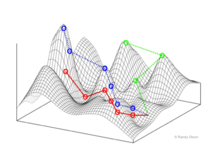
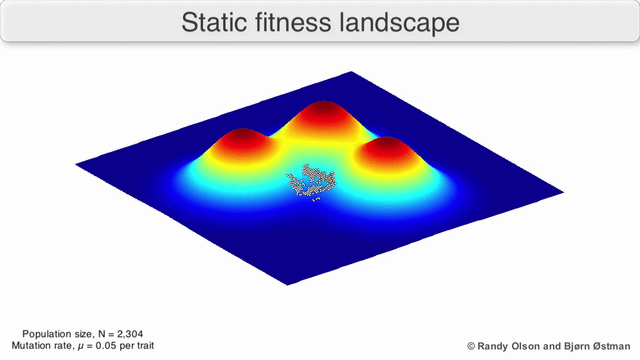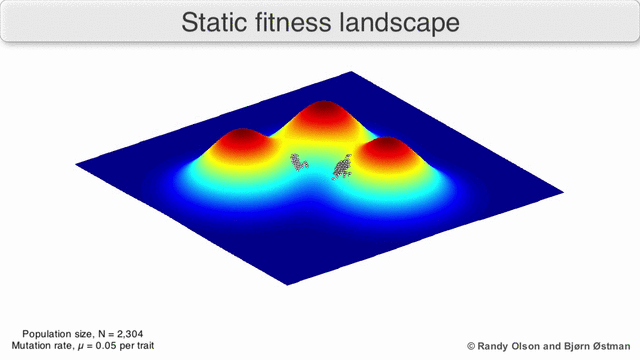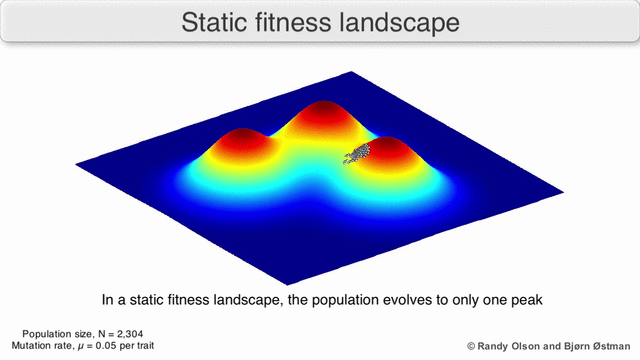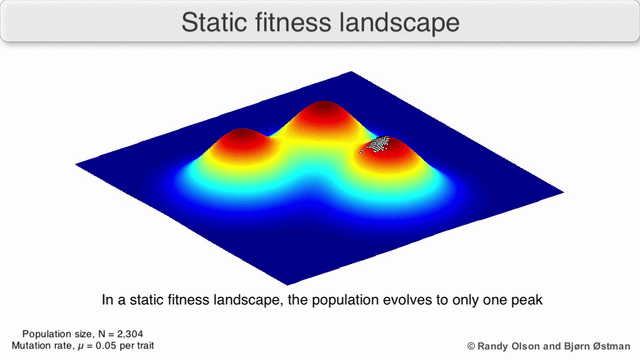
In evolutionary biology, fitness landscapes or adaptive landscapes are used to visualize the relationship between genotypes and reproductive success. It is assumed that every genotype has a well-defined replication rate. This fitness is the "height" of the landscape. Genotypes which are similar are said to be "close" to each other, while those that are very different are "far" from each other. The set of all possible genotypes, their degree of similarity, and their related fitness values is then called a fitness landscape. The idea of a fitness landscape is a metaphor to help explain flawed forms in evolution by natural selection, including exploits and glitches in animals like their reactions to supernormal stimuli.

<span class="mw-page-title-main">Index of evolutionary biology articles</span>

This is a list of topics in evolutionary biology.

Evolvability is defined as the capacity of a system for adaptive evolution. Evolvability is the ability of a population of organisms to not merely generate genetic diversity, but to generate adaptive genetic diversity, and thereby evolve through natural selection.

<span class="mw-page-title-main">Baldwin effect</span> Effect of learned behavior on evolution

In evolutionary biology, the Baldwin effect describes an effect of learned behaviour on evolution. James Mark Baldwin and others suggested that an organism's ability to learn new behaviours will affect its reproductive success and will therefore have an effect on the genetic makeup of its species through natural selection. It posits that subsequent selection might reinforce the originally learned behaviors, if adaptive, into more in-born, instinctive ones. Though this process appears similar to Lamarckism, that view proposes that living things inherited their parents' acquired characteristics. The Baldwin effect only posits that learning ability, which is genetically based, is another variable in / contributor to environmental adaptation. First proposed during the Eclipse of Darwinism in the late 19th century, this effect has been independently proposed several times, and today it is generally recognized as part of the modern synthesis.

<span class="mw-page-title-main">Pleiotropy</span> Influence of a single gene on multiple phenotypic traits

Pleiotropy occurs when one gene influences two or more seemingly unrelated phenotypic traits. Such a gene that exhibits multiple phenotypic expression is called a pleiotropic gene. Mutation in a pleiotropic gene may have an effect on several traits simultaneously, due to the gene coding for a product used by a myriad of cells or different targets that have the same signaling function.

Evolutionary capacitance is the storage and release of variation, just as electric capacitors store and release charge. Living systems are robust to mutations. This means that living systems accumulate genetic variation without the variation having a phenotypic effect. But when the system is disturbed, robustness breaks down, and the variation has phenotypic effects and is subject to the full force of natural selection. An evolutionary capacitor is a molecular switch mechanism that can "toggle" genetic variation between hidden and revealed states. If some subset of newly revealed variation is adaptive, it becomes fixed by genetic assimilation. After that, the rest of variation, most of which is presumably deleterious, can be switched off, leaving the population with a newly evolved advantageous trait, but no long-term handicap. For evolutionary capacitance to increase evolvability in this way, the switching rate should not be faster than the timescale of genetic assimilation.

<span class="mw-page-title-main">Canalisation (genetics)</span> Measure of the ability of a population to produce the same phenotype

Canalisation is a measure of the ability of a population to produce the same phenotype regardless of variability of its environment or genotype. It is a form of evolutionary robustness. The term was coined in 1942 by C. H. Waddington to capture the fact that "developmental reactions, as they occur in organisms submitted to natural selection...are adjusted so as to bring about one definite end-result regardless of minor variations in conditions during the course of the reaction". He used this word rather than robustness to consider that biological systems are not robust in quite the same way as, for example, engineered systems.

<i>The Neutral Theory of Molecular Evolution</i>

The Neutral Theory of Molecular Evolution is an influential monograph written in 1983 by Japanese evolutionary biologist Motoo Kimura. While the neutral theory of molecular evolution existed since his article in 1968, Kimura felt the need to write a monograph with up-to-date information and evidences showing the importance of his theory in evolution.

Neutral mutations are changes in DNA sequence that are neither beneficial nor detrimental to the ability of an organism to survive and reproduce. In population genetics, mutations in which natural selection does not affect the spread of the mutation in a species are termed neutral mutations. Neutral mutations that are inheritable and not linked to any genes under selection will be lost or will replace all other alleles of the gene. That loss or fixation of the gene proceeds based on random sampling known as genetic drift. A neutral mutation that is in linkage disequilibrium with other alleles that are under selection may proceed to loss or fixation via genetic hitchhiking and/or background selection.

Genetic assimilation is a process described by Conrad H. Waddington by which a phenotype originally produced in response to an environmental condition, such as exposure to a teratogen, later becomes genetically encoded via artificial selection or natural selection. Despite superficial appearances, this does not require the (Lamarckian) inheritance of acquired characters, although epigenetic inheritance could potentially influence the result. Waddington stated that genetic assimilation overcomes the barrier to selection imposed by what he called canalization of developmental pathways; he supposed that the organism's genetics evolved to ensure that development proceeded in a certain way regardless of normal environmental variations.

<span class="mw-page-title-main">Shifting balance theory</span> One version of the theory of evolution

The shifting balance theory is a theory of evolution proposed in 1932 by Sewall Wright, suggesting that adaptive evolution may proceed most quickly when a population divides into subpopulations with restricted gene flow. The name of the theory is borrowed from Wright's metaphor of fitness landscapes, attempting to explain how a population may move across an adaptive valley to a higher adaptive peak. According to the theory, this movement occurs in three steps:

  1. Genetic drift allows a locally adapted subpopulation to move across an adaptive valley to the base of a higher adaptive peak.
  2. Natural selection will move the subpopulation up the higher peak.
  3. This new superiorly adapted subpopulation may then expand its range and outcompete or interbreed with other subpopulations, causing the spread of new adaptations and movement of the global population toward the new fitness peak.
<span class="mw-page-title-main">Phenotypic integration</span>

Phenotypic Integration is a metric for measuring the correlation of multiple functionally-related traits to each other. Complex phenotypes often require multiple traits working together in order to function properly. Phenotypic integration is significant because it provides an explanation as to how phenotypes are sustained by relationships between traits. Every organism's phenotype is integrated, organized, and a functional whole. Integration is also associated with functional modules. Modules are complex character units that are tightly associated, such as a flower. It is hypothesized that organisms with high correlations between traits in a module have the most efficient functions. The fitness of a particular value for one phenotypic trait frequently depends on the value of the other phenotypic traits, making it important for those traits evolve together. One trait can have a direct effect on fitness, and it has been shown that the correlations among traits can also change fitness, causing these correlations to be adaptive, rather than solely genetic. Integration can be involved in multiple aspects of life, not just at the genetic level, but during development, or simply at a functional level.

<span class="mw-page-title-main">Epistasis</span> Dependence of a gene mutations phenotype on mutations in other genes

Epistasis is a phenomenon in genetics in which the effect of a gene mutation is dependent on the presence or absence of mutations in one or more other genes, respectively termed modifier genes. In other words, the effect of the mutation is dependent on the genetic background in which it appears. Epistatic mutations therefore have different effects on their own than when they occur together. Originally, the term epistasis specifically meant that the effect of a gene variant is masked by that of different gene.

This glossary of genetics and evolutionary biology is a list of definitions of terms and concepts used in the study of genetics and evolutionary biology, as well as sub-disciplines and related fields, with an emphasis on classical genetics, quantitative genetics, population biology, phylogenetics, speciation, and systematics. Overlapping and related terms can be found in Glossary of cellular and molecular biology, Glossary of ecology, and Glossary of biology.

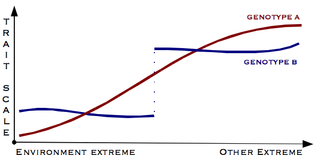
In evolutionary biology, developmental bias refers to the production against or towards certain ontogenetic trajectories which ultimately influence the direction and outcome of evolutionary change by affecting the rates, magnitudes, directions and limits of trait evolution. Historically, the term was synonymous with developmental constraint, however, the latter has been more recently interpreted as referring solely to the negative role of development in evolution.

Bias in the introduction of variation is a theory in the domain of evolutionary biology that asserts biases in the introduction of heritable variation are reflected in the outcome of evolution. It is relevant to topics in molecular evolution, evo-devo, and self-organization. In the context of this theory, "introduction" ("origination") is a technical term for events that shift an allele frequency upward from zero. Formal models demonstrate that when an evolutionary process depends on introduction events, mutational and developmental biases in the generation of variation may influence the course of evolution by a first come, first served effect, so that evolution reflects the arrival of the likelier, not just the survival of the fitter. Whereas mutational explanations for evolutionary patterns are typically assumed to imply or require neutral evolution, the theory of arrival biases distinctively predicts the possibility of mutation-biased adaptation. Direct evidence for the theory comes from laboratory studies showing that adaptive changes are systematically enriched for mutationally likely types of changes. Retrospective analyses of natural cases of adaptation also provide support for the theory. This theory is notable as an example of contemporary structuralist thinking, contrasting with a classical functionalist view in which the course of evolution is determined by natural selection.

References

  1. 1 2 3 4 5 6 7 Wright, Sewall (1932) The Roles of Mutation, Inbreeding, Crossbreeding, and Selection in Evolution. Proceedings of the Sixth International Congress of Genetics 1: 356–366
  2. ↑ Wright, Sewall (1988) Surfaces of Selective Value Revisited. The American Naturalist 131(1):115-123
  3. ↑ Lee, Carol E. & Gelebiuk, Gregory W. (2008) Evolutionary origins of invasive populations. "Evolutionary Applications" 1: 427–448.
  4. 1 2 McCoy J. Wynne. 1979. The Origin of the "Adaptive Landscape" Concept. The American Naturalist 113(4):610-613.
  5. 1 2 3 4 5 6 7 8 Dietrich MR & Skipper RA. 2012 "A Shifting Terrain: A Brief History of the Adaptive Landscape." In: The Adaptive Landscape in Evolutionary Biology. eds. Erik Svensson & Ryan Calsbeek Oxford Press.
  6. 1 2 3 Simpson, GG. 1944. Tempo and Mode in Evolution Columbia University Press, New York.
  7. 1 2 3 4 Lande, R. (1976) Natural selection and random genetic drift in phenotypic evolution. Evolution 30: 314-334.
  8. ↑ Lande, R. (1979) Effective Deme Sizes During Long-Term Evolution Estimated from Rates of Chromosomal Rearrangement. Evolution 33:234–251
  9. ↑ The Adaptive Landscape in Evolutionary Biology. Eds. Erik Svensson & Ryan Calsbeek. 2012
  10. 1 2 3 4 5 6 7 8 Kaplan J. (2008) The end of the adaptive landscape metaphor? Bio Philos 23:625-638
  11. ↑ Skipper RA & Dietrich MR. 2012 "Sewall Wright's adaptive landscape: Philosophical reflections on heuristic value." In: The Adaptive Landscape in Evolutionary Biology. eds. Erik Svensson & Ryan Calsbeek Oxford Press.
  12. 1 2 Pigliucci M 2012. "Landscapes, surfaces, and morphospaces: What are they good for?" In: The Adaptive Landscape in Evolutionary Biology. eds. Erik Svensson & Ryan Calsbeek Oxford Press.
  13. ↑ Steinberg, Barrett; Ostermeier, Marc (2016-01-01). "Environmental changes bridge evolutionary valleys". Science Advances. 2 (1): e1500921. Bibcode:2016SciA....2E0921S. doi:10.1126/sciadv.1500921. ISSN   2375-2548. PMC   4737206 . PMID   26844293.
  14. 1 2 Vasseur E, M Boniotto, E Patin, G Laval, H Quach, J Manry, B Crouau-Roy, & L Quintana-Murci. 2012. The Evolutionary Landscape of Cytosolic Microbial Sensors in Humans. The American Journal of Human Genetics 91:27-37.
  15. 1 2 3 Cambray G & D Mazel. 2008. Synonymous Genes Explore Different Evolutionary Landscapes. PLoS Genetics 4(11).

Further reading

Examples of visualized evolutionary landscapes:

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.