Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates functions of complex numbers. It is helpful in many branches of mathematics, including algebraic geometry, number theory, analytic combinatorics, and applied mathematics, as well as in physics, including the branches of hydrodynamics, thermodynamics, quantum mechanics, and twistor theory. By extension, use of complex analysis also has applications in engineering fields such as nuclear, aerospace, mechanical and electrical engineering.
In complex analysis, an entire function, also called an integral function, is a complex-valued function that is holomorphic on the whole complex plane. Typical examples of entire functions are polynomials and the exponential function, and any finite sums, products and compositions of these, such as the trigonometric functions sine and cosine and their hyperbolic counterparts sinh and cosh, as well as derivatives and integrals of entire functions such as the error function. If an entire function has a root at , then , taking the limit value at , is an entire function. On the other hand, the natural logarithm, the reciprocal function, and the square root are all not entire functions, nor can they be continued analytically to an entire function.

In geometry, the tangent line (or simply tangent) to a plane curve at a given point is, intuitively, the straight line that "just touches" the curve at that point. Leibniz defined it as the line through a pair of infinitely close points on the curve. More precisely, a straight line is tangent to the curve y = f(x) at a point x = c if the line passes through the point (c, f(c)) on the curve and has slope f'(c), where f' is the derivative of f. A similar definition applies to space curves and curves in n-dimensional Euclidean space.
In complex analysis, a branch of mathematics, the Casorati–Weierstrass theorem describes the behaviour of holomorphic functions near their essential singularities. It is named for Karl Theodor Wilhelm Weierstrass and Felice Casorati. In Russian literature it is called Sokhotski's theorem.
In mathematics, the Laurent series of a complex function is a representation of that function as a power series which includes terms of negative degree. It may be used to express complex functions in cases where a Taylor series expansion cannot be applied. The Laurent series was named after and first published by Pierre Alphonse Laurent in 1843. Karl Weierstrass had previously described it in a paper written in 1841 but not published until 1894.

In complex analysis, an essential singularity of a function is a "severe" singularity near which the function exhibits striking behavior.

In mathematics, the complex plane is the plane formed by the complex numbers, with a Cartesian coordinate system such that the horizontal x-axis, called the real axis, is formed by the real numbers, and the vertical y-axis, called the imaginary axis, is formed by the imaginary numbers.
In mathematics, an affine algebraic plane curve is the zero set of a polynomial in two variables. A projective algebraic plane curve is the zero set in a projective plane of a homogeneous polynomial in three variables. An affine algebraic plane curve can be completed in a projective algebraic plane curve by homogenizing its defining polynomial. Conversely, a projective algebraic plane curve of homogeneous equation h(x, y, t) = 0 can be restricted to the affine algebraic plane curve of equation h(x, y, 1) = 0. These two operations are each inverse to the other; therefore, the phrase algebraic plane curve is often used without specifying explicitly whether it is the affine or the projective case that is considered.
In mathematics, a differentiable function of one real variable is a function whose derivative exists at each point in its domain. In other words, the graph of a differentiable function has a non-vertical tangent line at each interior point in its domain. A differentiable function is smooth and does not contain any break, angle, or cusp.
In mathematics, the support of a real-valued function is the subset of the function domain containing the elements which are not mapped to zero. If the domain of is a topological space, then the support of is instead defined as the smallest closed set containing all points not mapped to zero. This concept is used widely in mathematical analysis.
In number theory, the local zeta functionZ(V, s) (sometimes called the congruent zeta function or the Hasse–Weil zeta function) is defined as
In mathematics, the étale cohomology groups of an algebraic variety or scheme are algebraic analogues of the usual cohomology groups with finite coefficients of a topological space, introduced by Grothendieck in order to prove the Weil conjectures. Étale cohomology theory can be used to construct ℓ-adic cohomology, which is an example of a Weil cohomology theory in algebraic geometry. This has many applications, such as the proof of the Weil conjectures and the construction of representations of finite groups of Lie type.

In mathematics, the oscillation of a function or a sequence is a number that quantifies how much that sequence or function varies between its extreme values as it approaches infinity or a point. As is the case with limits, there are several definitions that put the intuitive concept into a form suitable for a mathematical treatment: oscillation of a sequence of real numbers, oscillation of a real-valued function at a point, and oscillation of a function on an interval.
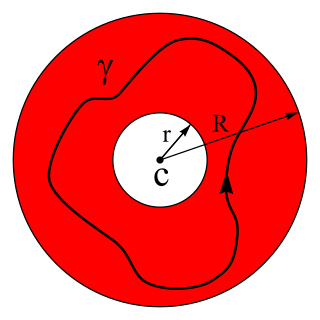
In the mathematical field of complex analysis, a branch point of a multivalued function is a point such that if the function is -valued at that point, all of its neighborhoods contain a point that has more than values. Multi-valued functions are rigorously studied using Riemann surfaces, and the formal definition of branch points employs this concept.
In functional analysis, a branch of mathematics, a compact operator is a linear operator , where are normed vector spaces, with the property that maps bounded subsets of to relatively compact subsets of . Such an operator is necessarily a bounded operator, and so continuous. Some authors require that are Banach, but the definition can be extended to more general spaces.
In mathematics, infinite-dimensional holomorphy is a branch of functional analysis. It is concerned with generalizations of the concept of holomorphic function to functions defined and taking values in complex Banach spaces, typically of infinite dimension. It is one aspect of nonlinear functional analysis.
In mathematics, the derivative is a fundamental construction of differential calculus and admits many possible generalizations within the fields of mathematical analysis, combinatorics, algebra, geometry, etc.
Continuous functions are of utmost importance in mathematics, functions and applications. However, not all functions are continuous. If a function is not continuous at a limit point of its domain, one says that it has a discontinuity there. The set of all points of discontinuity of a function may be a discrete set, a dense set, or even the entire domain of the function.
In mathematics, a surface is a mathematical model of the common concept of a surface. It is a generalization of a plane, but, unlike a plane, it may be curved; this is analogous to a curve generalizing a straight line.
Most of the terms listed in Wikipedia glossaries are already defined and explained within Wikipedia itself. However, glossaries like this one are useful for looking up, comparing and reviewing large numbers of terms together. You can help enhance this page by adding new terms or writing definitions for existing ones.