International System of Quantities

Last updated October 30, 2024

The International System of Quantities (ISQ) is a standard system of quantities used in physics and in modern science in general. It includes basic quantities such as length and mass and the relationships between those quantities.^{[lower-alpha 1]} This system underlies the International System of Units (SI)^{[lower-alpha 2]} but does not itself determine the units of measurement used for the quantities.

Base quantities

The base quantities of a given system of physical quantities is a subset of those quantities, where no base quantity can be expressed in terms of the others, but where every quantity in the system can be expressed in terms of the base quantities. Within this constraint, the set of base quantities is chosen by convention. There are seven ISQ base quantities. The symbols for them, as for other quantities, are written in italics.^[1]

The dimension of a physical quantity does not include magnitude or units. The conventional symbolic representation of the dimension of a base quantity is a single upper-case letter in roman (upright) sans-serif ^{[lower-alpha 3]} type.

Base quantity	Symbol for dimension	Symbol for quantity^{[lower-alpha 4]}	SI base unit ^{[lower-alpha 4]}	SI unit symbol^{[lower-alpha 4]}
length	${\mathsf {L}}$	$l$	metre	m
mass	${\mathsf {M}}$	$m$	kilogram	kg
time	${\mathsf {T}}$	$t$	second	s
electric current	${\mathsf {I}}$	$I$	ampere	A
thermodynamic temperature	${\mathsf {\Theta }}$	$T$	kelvin	K
amount of substance	${\mathsf {N}}$	$n$	mole	mol
luminous intensity	${\mathsf {J}}$	$I_{\text{v}}$	candela	cd

Derived quantities

A derived quantity is a quantity in a system of quantities that is defined in terms of only the base quantities of that system. The ISQ defines many derived quantities and corresponding derived units.

Dimensional expression of derived quantities

The conventional symbolic representation of the dimension of a derived quantity is the product of powers of the dimensions of the base quantities according to the definition of the derived quantity. The dimension of a quantity is denoted by ${\mathsf {L}}^{a}{\mathsf {M}}^{b}{\mathsf {T}}^{c}{\mathsf {I}}^{d}{\mathsf {\Theta }}^{e}{\mathsf {N}}^{f}{\mathsf {J}}^{g}$ , where the dimensional exponents are positive, negative, or zero. The dimension symbol may be omitted if its exponent is zero. For example, in the ISQ, the quantity dimension of velocity is denoted ${\mathsf {LT}}^{-1}$ . The following table lists some quantities defined by the ISQ.

Derived quantity	Expression in SI base dimensions
frequency	${\mathsf {T}}^{-1}$
force	${\mathsf {LMT}}^{-2}$
pressure	${\mathsf {L}}^{-1}{\mathsf {MT}}^{-2}$
velocity	${\mathsf {LT}}^{-1}$
area	${\mathsf {L}}^{2}$
volume	${\mathsf {L}}^{3}$
acceleration	${\mathsf {LT}}^{-2}$

Dimensionless quantities

A quantity of dimension one is historically known as a dimensionless quantity (a term that is still commonly used); all its dimensional exponents are zero and its dimension symbol is $1$ . Such a quantity can be regarded as a derived quantity in the form of the ratio of two quantities of the same dimension. The named dimensionless units "radian" (rad) and "steradian" (sr) are acceptable for distinguishing dimensionless quantities of different kind, respectively plane angle and solid angle.^[3]

Logarithmic quantities

Level

The level of a quantity is defined as the logarithm of the ratio of the quantity with a stated reference value of that quantity. Within the ISQ it is differently defined for a root-power quantity (also known by the deprecated term field quantity) and for a power quantity. It is not defined for ratios of quantities of other kinds. Within the ISQ, all levels are treated as derived quantities of dimension 1.^{[ citation needed ]} Several units for levels are defined by the SI and classified as "non-SI units accepted for use with the SI units".^[4] An example of level is sound pressure level, with the unit of decibel.

Other logarithmic quantities

Units of logarithmic frequency ratio include the octave, corresponding to a factor of 2 in frequency (precisely) and the decade, corresponding to a factor 10.

The ISQ recognizes another logarithmic quantity, information entropy, for which the coherent unit is the natural unit of information (symbol nat).^{[ citation needed ]}

Documentation

The system is formally described in a multi-part ISO standard ISO/IEC 80000, first completed in 2009 but subsequently revised and expanded, which replaced standards published in 1992, ISO 31 and ISO 1000. Working jointly, ISO and IEC have formalized parts of the ISQ by giving information and definitions concerning quantities, systems of quantities, units, quantity and unit symbols, and coherent unit systems, with particular reference to the ISQ. ISO/IEC 80000 defines physical quantities that are measured with the SI units ^[5] and also includes many other quantities in modern science and technology.^[1] The name "International System of Quantities" is used by the General Conference on Weights and Measures (CGPM) to describe the system of quantities that underlie the International System of Units.

Notes

↑ "The system of quantities, including the relations among them the quantities used as the basis of the units of the SI, is named the International System of Quantities, denoted 'ISQ', in all languages. [...] It should be realized, however, that ISQ is simply a convenient notation to assign to the essentially infinite and continually evolving and expanding system of quantities and equations on which all of modern science and technology rests. ISQ is a shorthand notation for the 'system of quantities on which the SI is based', which was the phrase used for this system in ISO 31."^[1]
↑ "The revised harmonized standard will be known as ISO/IEC 80000, Quantities and Units, in which it is proposed that the quantities and equations used with the SI will be known as the International System of Quantities."^[2]
↑ The status of the requirement for sans-serif is not as clear, since ISO 80000-1:2009 makes no mention of it ("The conventional symbolic representation of the dimension of a base quantity is a single upper case letter in roman (upright) type.") whereas the secondary source BIPM JCGM 200:2012 does ("The conventional symbolic representation of the dimension of a base quantity is a single upper case letter in roman (upright) sans-serif type.").
1 2 3 The associated quantity symbol, the SI unit name and SI unit symbol are given here for reference only; they do not form part of the ISQ.

Related Research Articles

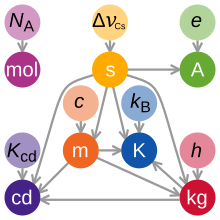
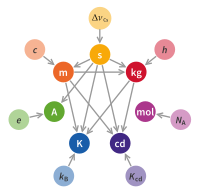
In engineering and science, dimensional analysis is the analysis of the relationships between different physical quantities by identifying their base quantities and units of measurement and tracking these dimensions as calculations or comparisons are performed. The term dimensional analysis is also used to refer to conversion of units from one dimensional unit to another, which can be used to evaluate scientific formulae.

In chemistry, the mole fraction or molar fraction, also called mole proportion or molar proportion, is a quantity defined as the ratio between the amount of a constituent substance, n_i, and the total amount of all constituents in a mixture, n_tot :

<span class="mw-page-title-main">Physical quantity</span> Measurable property of a material or system

A physical quantity is a property of a material or system that can be quantified by measurement. A physical quantity can be expressed as a value, which is the algebraic multiplication of a numerical value and a unit of measurement. For example, the physical quantity mass, symbol m, can be quantified as m=n kg, where n is the numerical value and kg is the unit symbol. Quantities that are vectors have, besides numerical value and unit, direction or orientation in space.

The radian, denoted by the symbol rad, is the unit of angle in the International System of Units (SI) and is the standard unit of angular measure used in many areas of mathematics. It is defined such that one radian is the angle subtended at the centre of a circle by an arc that is equal in length to the radius. The unit was formerly an SI supplementary unit and is currently a dimensionless SI derived unit, defined in the SI as 1 rad = 1 and expressed in terms of the SI base unit metre (m) as rad = m/m. Angles without explicitly specified units are generally assumed to be measured in radians, especially in mathematical writing.

The International System of Units, internationally known by the abbreviation SI, is the modern form of the metric system and the world's most widely used system of measurement. Coordinated by the International Bureau of Weights and Measures it is the only system of measurement with official status in nearly every country in the world, employed in science, technology, industry, and everyday commerce.

SI derived units are units of measurement derived from the seven SI base units specified by the International System of Units (SI). They can be expressed as a product of one or more of the base units, possibly scaled by an appropriate power of exponentiation. Some are dimensionless, as when the units cancel out in ratios of like quantities. SI coherent derived units involve only a trivial proportionality factor, not requiring conversion factors.

<span class="mw-page-title-main">Neper</span> Logarithmic unit for ratios of measurements of physical field and power quantities

The neper is a logarithmic unit for ratios of measurements of physical field and power quantities, such as gain and loss of electronic signals. The unit's name is derived from the name of John Napier, the inventor of logarithms. As is the case for the decibel and bel, the neper is a unit defined in the international standard ISO 80000. It is not part of the International System of Units (SI), but is accepted for use alongside the SI.

Dimensionless quantities, or quantities of dimension one, are quantities implicitly defined in a manner that prevents their aggregation into units of measurement. Typically expressed as ratios that align with another system, these quantities do not necessitate explicitly defined units. For instance, alcohol by volume (ABV) represents a volumetric ratio; its value remains independent of the specific units of volume used, such as in milliliters per milliliter (mL/mL).

A base unit of measurement is a unit of measurement adopted for a base quantity. A base quantity is one of a conventionally chosen subset of physical quantities, where no quantity in the subset can be expressed in terms of the others. The SI base units, or Systéme International d'unités, consists of the metre, kilogram, second, ampere, kelvin, mole and candela.

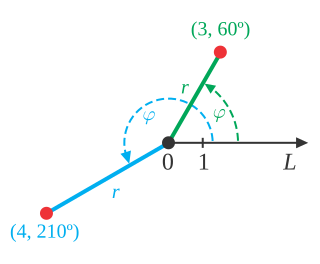
The angular displacement – also called angle of rotation, rotational displacement, or rotary displacement – of a physical body is the angle through which the body rotates around a centre or axis of rotation. Angular displacement may be signed, indicating the sense of rotation ; it may also be greater than a full turn.

<span class="mw-page-title-main">Quotient</span> Mathematical result of division

In arithmetic, a quotient is a quantity produced by the division of two numbers. The quotient has widespread use throughout mathematics. It has two definitions: either the integer part of a division or a fraction or ratio. For example, when dividing 20 by 3, the quotient is 6 in the first sense and $in the second sense.$

ISO 31 is a superseded international standard concerning physical quantities, units of measurement, their interrelationships and their presentation. It was revised and replaced by ISO/IEC 80000.

<span class="mw-page-title-main">Turn (angle)</span> Unit of plane angle where a full circle equals 1

The turn is a unit of plane angle measurement that is the measure of a complete angle—the angle subtended by a complete circle at its center. One turn is equal to $2 π$ radians, 360 degrees or 400 gradians. As an angular unit, one turn also corresponds to one cycle or to one revolution. Common related units of frequency are cycles per second (cps) and revolutions per minute (rpm). The angular unit of the turn is useful in connection with, among other things, electromagnetic coils, rotating objects, and the winding number of curves. Subdivisions of a turn include the half-turn and quarter-turn, spanning a straight angle and a right angle, respectively; metric prefixes can also be used as in, e.g., centiturns (ctr), milliturns (mtr), etc.

Gaussian units constitute a metric system of physical units. This system is the most common of the several electromagnetic unit systems based on cgs (centimetre–gram–second) units. It is also called the Gaussian unit system, Gaussian-cgs units, or often just cgs units. The term "cgs units" is ambiguous and therefore to be avoided if possible: there are several variants of cgs with conflicting definitions of electromagnetic quantities and units.

International standard ISO 1000 is the ISO standard describing the International System of Units (SI).

IEEE 1541-2002 is a standard issued in 2002 by the Institute of Electrical and Electronics Engineers (IEEE) concerning the use of prefixes for binary multiples of units of measurement related to digital electronics and computing. IEEE 1541-2021 revises and supersedes IEEE 1541–2002, which is 'inactive'.

ISO/IEC 80000, Quantities and units, is an international standard describing the International System of Quantities (ISQ). It was developed and promulgated jointly by the International Organization for Standardization (ISO) and the International Electrotechnical Commission (IEC). It serves as a style guide for using physical quantities and units of measurement, formulas involving them, and their corresponding units, in scientific and educational documents for worldwide use. The ISO/IEC 80000 family of standards was completed with the publication of the first edition of Part 1 in November 2009.

A unit of measurement, or unit of measure, is a definite magnitude of a quantity, defined and adopted by convention or by law, that is used as a standard for measurement of the same kind of quantity. Any other quantity of that kind can be expressed as a multiple of the unit of measurement.

Quantity calculus is the formal method for describing the mathematical relations between abstract physical quantities.

In science and engineering, a power level and a field level are logarithmic magnitudes of certain quantities referenced to a standard reference value of the same type.

References

1 2 3 ISO 80000-1:2009 Quantities and units. Part 1: General (1st ed.), Switzerland: ISO (the International Organization for Standardization), 2009-11-15, p. vi, retrieved 23 May 2015
↑ Taylor, Barry N. (April 2008), "NIST Special Publication 330 – 2008 edition", NIST, p. 10
↑ "ISO 80000-3:2019". International Organization for Standardization . Retrieved 2019-10-23.
↑ The International System of Units (PDF) (9th ed.), International Bureau of Weights and Measures, Dec 2022, p. 145, ISBN 978-92-822-2272-0
↑ "1.16" (PDF). International vocabulary of metrology – Basic and general concepts and associated terms (VIM) (3rd ed.). International Bureau of Weights and Measures (BIPM):Joint Committee for Guides in Metrology. 2012. Retrieved 28 March 2015.