In combinatorics, a branch of mathematics, a matroid is a structure that abstracts and generalizes the notion of linear independence in vector spaces. There are many equivalent ways to define a matroid axiomatically, the most significant being in terms of: independent sets; bases or circuits; rank functions; closure operators; and closed sets or flats. In the language of partially ordered sets, a finite simple matroid is equivalent to a geometric lattice.
In mathematics, a transcendental extension is a field extension such that there exists an element in the field that is transcendental over the field ; that is, an element that is not a root of any univariate polynomial with coefficients in . In other words, a transcendental extension is a field extension that is not algebraic. For example, and are both transcendental extensions of
In abstract algebra, a subset of a field is algebraically independent over a subfield if the elements of do not satisfy any non-trivial polynomial equation with coefficients in .
In mathematics, a closure operator on a set S is a function :{\mathcal {P}}(S)\rightarrow {\mathcal {P}}(S)} from the power set of S to itself that satisfies the following conditions for all sets
In mathematics, a duality translates concepts, theorems or mathematical structures into other concepts, theorems or structures in a one-to-one fashion, often by means of an involution operation: if the dual of A is B, then the dual of B is A. In other cases the dual of the dual – the double dual or bidual – is not necessarily identical to the original. Such involutions sometimes have fixed points, so that the dual of A is A itself. For example, Desargues' theorem is self-dual in this sense under the standard duality in projective geometry.
Pregeometry, and in full combinatorial pregeometry, are essentially synonyms for "matroid". They were introduced by Gian-Carlo Rota with the intention of providing a less "ineffably cacophonous" alternative term. Also, the term combinatorial geometry, sometimes abbreviated to geometry, was intended to replace "simple matroid". These terms are now infrequently used in the study of matroids.

In linear algebra, a cone—sometimes called a linear cone for distinguishing it from other sorts of cones—is a subset of a vector space that is closed under positive scalar multiplication; that is, C is a cone if implies for every positive scalar s. A cone need not be convex, or even look like a cone in Euclidean space.
In mathematics, a regular matroid is a matroid that can be represented over all fields.
Algebraic combinatorics is an area of mathematics that employs methods of abstract algebra, notably group theory and representation theory, in various combinatorial contexts and, conversely, applies combinatorial techniques to problems in algebra.
In matroid theory, the dual of a matroid is another matroid that has the same elements as , and in which a set is independent if and only if has a basis set disjoint from it.
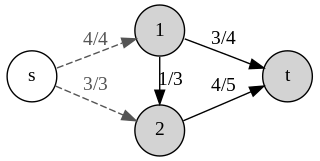
An oriented matroid is a mathematical structure that abstracts the properties of directed graphs, vector arrangements over ordered fields, and hyperplane arrangements over ordered fields. In comparison, an ordinary matroid abstracts the dependence properties that are common both to graphs, which are not necessarily directed, and to arrangements of vectors over fields, which are not necessarily ordered.
In matroid theory, a field within mathematics, a gammoid is a certain kind of matroid, describing sets of vertices that can be reached by vertex-disjoint paths in a directed graph.
In mathematics, a uniform matroid is a matroid in which the independent sets are exactly the sets containing at most r elements, for some fixed integer r. An alternative definition is that every permutation of the elements is a symmetry.
Rota's excluded minors conjecture is one of a number of conjectures made by the mathematician Gian-Carlo Rota. It is considered an important problem by some members of the structural combinatorics community. Rota conjectured in 1971 that, for every finite field, the family of matroids that can be represented over that field has only finitely many excluded minors. A proof of the conjecture was announced, but not published, in 2014 by Geelen, Gerards, and Whittle.
In matroid theory, a binary matroid is a matroid that can be represented over the finite field GF(2). That is, up to isomorphism, they are the matroids whose elements are the columns of a (0,1)-matrix and whose sets of elements are independent if and only if the corresponding columns are linearly independent in GF(2).
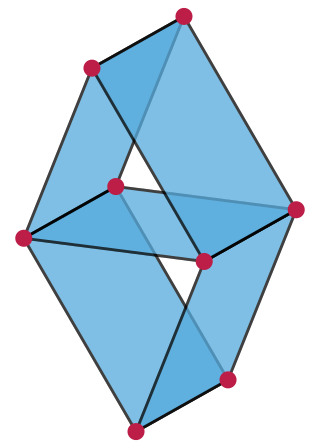
In mathematics, the Vámos matroid or Vámos cube is a matroid over a set of eight elements that cannot be represented as a matrix over any field. It is named after English mathematician Peter Vámos, who first described it in an unpublished manuscript in 1968.
In the mathematical theory of matroids, the rank of a matroid is the maximum size of an independent set in the matroid. The rank of a subset S of elements of the matroid is, similarly, the maximum size of an independent subset of S, and the rank function of the matroid maps sets of elements to their ranks.
In mathematics, an algebraic matroid is a matroid, a combinatorial structure, that expresses an abstraction of the relation of algebraic independence.

In the mathematical theory of matroids, a paving matroid is a matroid in which every circuit has size at least as large as the matroid's rank. In a matroid of rank every circuit has size at most , so it is equivalent to define paving matroids as the matroids in which the size of every circuit belongs to the set . It has been conjectured that almost all matroids are paving matroids.
In mathematics, a basis of a matroid is a maximal independent set of the matroid—that is, an independent set that is not contained in any other independent set.





















