Related Research Articles
In physics and mathematics, the dimension of a mathematical space is informally defined as the minimum number of coordinates needed to specify any point within it. Thus, a line has a dimension of one (1D) because only one coordinate is needed to specify a point on it – for example, the point at 5 on a number line. A surface, such as the boundary of a cylinder or sphere, has a dimension of two (2D) because two coordinates are needed to specify a point on it – for example, both a latitude and longitude are required to locate a point on the surface of a sphere. A two-dimensional Euclidean space is a two-dimensional space on the plane. The inside of a cube, a cylinder or a sphere is three-dimensional (3D) because three coordinates are needed to locate a point within these spaces.
In mathematics, a metric space is a set together with a notion of distance between its elements, usually called points. The distance is measured by a function called a metric or distance function. Metric spaces are the most general setting for studying many of the concepts of mathematical analysis and geometry.
A sphere is a geometrical object that is a three-dimensional analogue to a two-dimensional circle. Formally, a sphere is the set of points that are all at the same distance r from a given point in three-dimensional space. That given point is the centre of the sphere, and r is the sphere's radius. The earliest known mentions of spheres appear in the work of the ancient Greek mathematicians.
Distance is a numerical or occasionally qualitative measurement of how far apart objects or points are. In physics or everyday usage, distance may refer to a physical length or an estimation based on other criteria. Since spatial cognition is a rich source of conceptual metaphors in human thought, the term is also frequently used metaphorically to mean a measurement of the amount of difference between two similar objects or a degree of separation. Most such notions of distance, both physical and metaphorical, are formalized in mathematics using the notion of a metric space.
In mathematics, the Euclidean distance between two points in Euclidean space is the length of a line segment between the two points. It can be calculated from the Cartesian coordinates of the points using the Pythagorean theorem, therefore occasionally being called the Pythagorean distance. These names come from the ancient Greek mathematicians Euclid and Pythagoras, although Euclid did not represent distances as numbers, and the connection from the Pythagorean theorem to distance calculation was not made until the 18th century.
In geometry, a geodesic is a curve representing in some sense the shortest path (arc) between two points in a surface, or more generally in a Riemannian manifold. The term also has meaning in any differentiable manifold with a connection. It is a generalization of the notion of a "straight line".
Elliptic geometry is an example of a geometry in which Euclid's parallel postulate does not hold. Instead, as in spherical geometry, there are no parallel lines since any two lines must intersect. However, unlike in spherical geometry, two lines are usually assumed to intersect at a single point. Because of this, the elliptic geometry described in this article is sometimes referred to as single elliptic geometry whereas spherical geometry is sometimes referred to as double elliptic geometry.
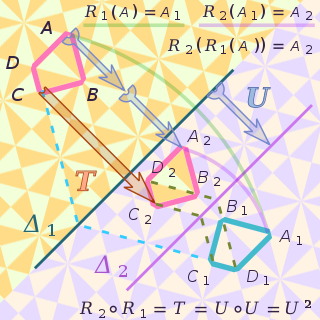
In mathematics, an isometry is a distance-preserving transformation between metric spaces, usually assumed to be bijective. The word isometry is derived from the Ancient Greek: ἴσος isos meaning "equal", and μέτρον metron meaning "measure".
This is a glossary of some terms used in Riemannian geometry and metric geometry — it doesn't cover the terminology of differential topology.
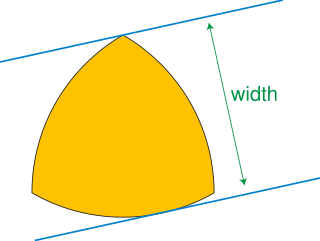
In geometry, a curve of constant width is a simple closed curve in the plane whose width is the same in all directions. The shape bounded by a curve of constant width is a body of constant width or an orbiform, the name given to these shapes by Leonhard Euler. Standard examples are the circle and the Reuleaux triangle. These curves can also be constructed using circular arcs centered at crossings of an arrangement of lines, as the involutes of certain curves, or by intersecting circles centered on a partial curve.
In mathematics, especially in functional analysis, the Tsirelson space is the first example of a Banach space in which neither an ℓ p space nor a c0 space can be embedded. The Tsirelson space is reflexive.
A Euclidean minimum spanning tree of a finite set of points in the Euclidean plane or higher-dimensional Euclidean space connects the points by a system of line segments with the points as endpoints, minimizing the total length of the segments. In it, any two points can reach each other along a path through the line segments. It can be found as the minimum spanning tree of a complete graph with the points as vertices and the Euclidean distances between points as edge weights.
In mathematics, Hilbert's fourth problem in the 1900 list of Hilbert's problems is a foundational question in geometry. In one statement derived from the original, it was to find — up to an isomorphism — all geometries that have an axiomatic system of the classical geometry, with those axioms of congruence that involve the concept of the angle dropped, and `triangle inequality', regarded as an axiom, added.
In mathematics, a space is a set with some added structure. While modern mathematics uses many types of spaces, such as Euclidean spaces, linear spaces, topological spaces, Hilbert spaces, or probability spaces, it does not define the notion of "space" itself.
The coastline paradox is the counterintuitive observation that the coastline of a landmass does not have a well-defined length. This results from the fractal curve–like properties of coastlines; i.e., the fact that a coastline typically has a fractal dimension. Although the "paradox of length" was previously noted by Hugo Steinhaus, the first systematic study of this phenomenon was by Lewis Fry Richardson, and it was expanded upon by Benoit Mandelbrot.
Riesz's lemma is a lemma in functional analysis. It specifies conditions that guarantee that a subspace in a normed vector space is dense. The lemma may also be called the Riesz lemma or Riesz inequality. It can be seen as a substitute for orthogonality when the normed space is not an inner product space.
In differential geometry, Mikhail Gromov's filling area conjecture asserts that the hemisphere has minimum area among the orientable surfaces that fill a closed curve of given length without introducing shortcuts between its points.
In mathematics, the equilateral dimension of a metric space is the maximum size of any subset of the space whose points are all at equal distances to each other. Equilateral dimension has also been called "metric dimension", but the term "metric dimension" also has many other inequivalent usages. The equilateral dimension of a -dimensional Euclidean space is , achieved by a regular simplex, and the equilateral dimension of a -dimensional vector space with the Chebyshev distance is , achieved by a hypercube. However, the equilateral dimension of a space with the Manhattan distance is not known; Kusner's conjecture, named after Robert B. Kusner, states that it is exactly , achieved by a cross polytope.
In functional analysis, a branch of mathematics, the distortion problem is to determine by how much one can distort the unit sphere in a given Banach space using an equivalent norm. Specifically, a Banach space X is called λ-distortable if there exists an equivalent norm |x| on X such that, for all infinite-dimensional subspaces Y in X,
In geometry of normed spaces, the Holmes–Thompson volume is a notion of volume that allows to compare sets contained in different normed spaces. It was introduced by Raymond D. Holmes and Anthony Charles Thompson.
References
- 1 2 Schäffer, Juan Jorge (1967), "Inner diameter, perimeter, and girth of spheres", Mathematische Annalen , 173: 79–82, doi:10.1007/BF01351519, MR 0218875, S2CID 116325652 .
- 1 2 3 Álvarez Paiva, J. C. (2006), "Some problems on Finsler geometry", Handbook of differential geometry. Vol. II, Elsevier/North-Holland, Amsterdam, pp. 1–33, doi:10.1016/S1874-5741(06)80004-X, MR 2194667 . See in particular p. 16.
- ↑ Harrell, R. E.; Karlovitz, L. A. (1970), "Girths and flat Banach spaces", Bulletin of the American Mathematical Society, 76 (6): 1288–1291, doi: 10.1090/S0002-9904-1970-12643-X , MR 0267383 .
- ↑ Álvarez Paiva, J. C. (2006), "Dual spheres have the same girth" (PDF), American Journal of Mathematics, 128 (2): 361–371, arXiv: math/0408414 , doi:10.1353/ajm.2006.0015, MR 2214896, S2CID 201749975 .
| Spaces |
| ||||
|---|---|---|---|---|---|
| Theorems | |||||
| Operators | |||||
| Algebras | |||||
| Open problems | |||||
| Applications | |||||
| Advanced topics | |||||
 | This mathematical analysis–related article is a stub. You can help Wikipedia by expanding it. |