| Green Point Hobart, Tasmania | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
 Green Point visible on the other side of the Jordan River Bridge from Herdsmans Cove | |||||||||||||||
  Green Point | |||||||||||||||
 | |||||||||||||||
| Coordinates | 42°44′26″S147°14′45″E / 42.74056°S 147.24583°E | ||||||||||||||
| Postcode(s) | 7030 | ||||||||||||||
| LGA(s) | Municipality of Brighton | ||||||||||||||
| |||||||||||||||
Green Point, Tasmania, is a far northern suburb of Hobart. It is generally considered by locals to be a part of Bridgewater.
 | This article about Hobart is a stub. You can help Wikipedia by expanding it. |
 | This Brighton, Tasmania geography article is a stub. You can help Wikipedia by expanding it. |
In mathematics, any vector space has a corresponding dual vector space consisting of all linear forms on together with the vector space structure of pointwise addition and scalar multiplication by constants.
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space, such as in a parametric curve. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it.
In mathematics, the exponential function is the unique real function which maps zero to one and has a derivative equal to its value. The exponential of a variable is denoted or , with the two notations used interchangeably. It is called exponential because its argument can be seen as an exponent to which a constant number e ≈ 2.718, the base, is raised. There are several other definitions of the exponential function, which are all equivalent although being of very different nature.
In mathematics, specifically category theory, a functor is a mapping between categories. Functors were first considered in algebraic topology, where algebraic objects are associated to topological spaces, and maps between these algebraic objects are associated to continuous maps between spaces. Nowadays, functors are used throughout modern mathematics to relate various categories. Thus, functors are important in all areas within mathematics to which category theory is applied.
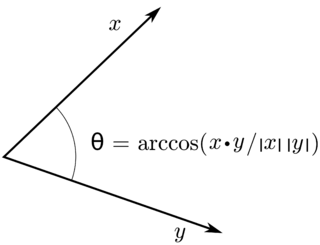
In mathematics, an inner product space is a real vector space or a complex vector space with an operation called an inner product. The inner product of two vectors in the space is a scalar, often denoted with angle brackets such as in . Inner products allow formal definitions of intuitive geometric notions, such as lengths, angles, and orthogonality of vectors. Inner product spaces generalize Euclidean vector spaces, in which the inner product is the dot product or scalar product of Cartesian coordinates. Inner product spaces of infinite dimension are widely used in functional analysis. Inner product spaces over the field of complex numbers are sometimes referred to as unitary spaces. The first usage of the concept of a vector space with an inner product is due to Giuseppe Peano, in 1898.
In mathematics, and more specifically in linear algebra, a linear map is a mapping between two vector spaces that preserves the operations of vector addition and scalar multiplication. The same names and the same definition are also used for the more general case of modules over a ring; see Module homomorphism.
Linear algebra is the branch of mathematics concerning linear equations such as:
In mathematics, a tensor is an algebraic object that describes a multilinear relationship between sets of algebraic objects related to a vector space. Tensors may map between different objects such as vectors, scalars, and even other tensors. There are many types of tensors, including scalars and vectors, dual vectors, multilinear maps between vector spaces, and even some operations such as the dot product. Tensors are defined independent of any basis, although they are often referred to by their components in a basis related to a particular coordinate system; those components form an array, which can be thought of as a high-dimensional matrix.
In mathematics, the tensor product of two vector spaces V and W is a vector space to which is associated a bilinear map that maps a pair to an element of denoted .
In linear algebra, the trace of a square matrix A, denoted tr(A), is the sum of the elements on its main diagonal, . It is only defined for a square matrix.
In algebra, the kernel of a homomorphism is generally the inverse image of 0. An important special case is the kernel of a linear map. The kernel of a matrix, also called the null space, is the kernel of the linear map defined by the matrix.
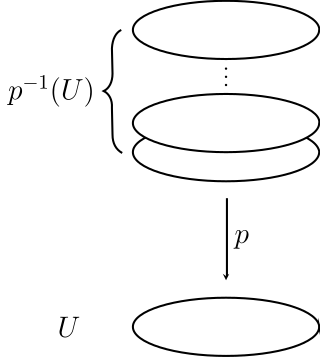
In topology, a covering or covering projection is a map between topological spaces that, intuitively, locally acts like a projection of multiple copies of a space onto itself. In particular, coverings are special types of local homeomorphisms. If is a covering, is said to be a covering space or cover of , and is said to be the base of the covering, or simply the base. By abuse of terminology, and may sometimes be called covering spaces as well. Since coverings are local homeomorphisms, a covering space is a special kind of étalé space.
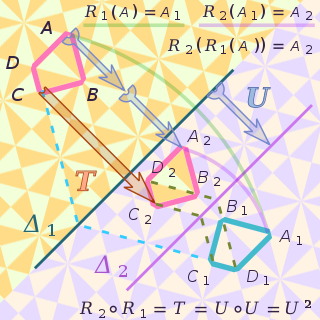
In mathematics, an isometry is a distance-preserving transformation between metric spaces, usually assumed to be bijective. The word isometry is derived from the Ancient Greek: ἴσος isos meaning "equal", and μέτρον metron meaning "measure". If the transformation is from a metric space to itself, it is a kind of geometric transformation known as a motion.
In mathematics, a vector bundle is a topological construction that makes precise the idea of a family of vector spaces parameterized by another space : to every point of the space we associate a vector space in such a way that these vector spaces fit together to form another space of the same kind as , which is then called a vector bundle over .
The President Range is a mountain range of the Canadian Rockies, located in the northwestern section of Yoho National Park. The range is named for the highest peak in the range, The President.
Dometiopolis was a city of Cilicia Trachea, and in the later Roman province of Isauria in Asia Minor. Its ruins are found in the village of Katranlı, Ermenek, Karaman Province, Turkey.
Altimir Glacier is a 4.8-kilometre (3.0 mi) long and 5.5-kilometre (3.4 mi) wide glacier draining the north slopes of the Osterrieth Range on Anvers Island in the Palmer Archipelago, Antarctica. It flows northwards to enter Dalchev Cove in Fournier Bay east of Studena Point.
In the theory of Lie groups, the exponential map is a map from the Lie algebra of a Lie group to the group, which allows one to recapture the local group structure from the Lie algebra. The existence of the exponential map is one of the primary reasons that Lie algebras are a useful tool for studying Lie groups.
The Ōshima Strait is a strait between the islands of Amami Ōshima and Kakeromajima in Japan. It is part of the Amami Guntō National Park. From the western most side to the eastern most side of the strait, the strait measures 17.5 miles (28.2 km) long.
The R746 road is a regional road in Ireland. It connects the R702 road at Doran's Cross, County Wexford with the R725 road at Croneyhorn, County Wicklow, via Bunclody. The R746 is 22.2 kilometres (13.8 mi) long.
