Modern Arabic mathematical notation

Last updated November 08, 2024

Modern Arabic mathematical notation is a mathematical notation based on the Arabic script, used especially at pre-university levels of education. Its form is mostly derived from Western notation, but has some notable features that set it apart from its Western counterpart. The most remarkable of those features is the fact that it is written from right to left following the normal direction of the Arabic script. Other differences include the replacement of the Greek and Latin alphabet letters for symbols with Arabic letters and the use of Arabic names for functions and relations.

Features

It is written from right to left following the normal direction of the Arabic script. Other differences include the replacement of the Latin alphabet letters for symbols with Arabic letters and the use of Arabic names for functions and relations.
The notation exhibits one of the very few remaining vestiges of non-dotted Arabic scripts, as dots over and under letters ( i'jam ) are usually omitted.
Letter cursivity (connectedness) of Arabic is also taken advantage of, in a few cases, to define variables using more than one letter. The most widespread example of this kind of usage is the canonical symbol for the radius of a circle نق (Arabic pronunciation: [nɑq] ), which is written using the two letters nūn and qāf. When variable names are juxtaposed (as when expressing multiplication) they are written non-cursively.

Variations

Notation differs slightly from one region to another. In tertiary education, most regions use the Western notation. The notation mainly differs in numeral system used, and in mathematical symbols used.

Numeral systems

There are three numeral systems used in right to left mathematical notation.

"Western Arabic numerals" (sometimes called European) are used in western Arabic regions (e.g. Morocco)
"Eastern Arabic numerals" are used in middle and eastern Arabic regions (e.g. Egypt and Syria)
"Eastern Arabic-Indic numerals" are used in Persian and Urdu speaking regions (e.g. Iran, Pakistan, India)

European (descended from Western Arabic)	0	1	2	3	4	5	6	7	8	9
Arabic-Indic (Eastern Arabic)	٠	١	٢	٣	٤	٥	٦	٧	٨	٩
Perso-Arabic variant	۰	۱	۲	۳	۴	۵	۶	۷	۸	۹
Urdu variant

Written numerals are arranged with their lowest-value digit to the right, with higher value positions added to the left. That is identical to the arrangement used by Western texts using Hindu-Arabic numerals even though Arabic script is read from right to left: Indeed, Western texts are written with the ones digit on the right because when the arithmetical manuals were translated from the Arabic, the numerals were treated as figures (like in a Euclidean diagram), and so were not flipped to match the Left-Right order of Latin text^[1]. The symbols "٫" and "٬" may be used as the decimal mark and the thousands separator respectively when writing with Eastern Arabic numerals, e.g. ٣٫١٤١٥٩٢٦٥٣٥٨3.14159265358, ١٬٠٠٠٬٠٠٠٬٠٠٠1,000,000,000. Negative signs are written to the left of magnitudes, e.g. ٣−−3. In-line fractions are written with the numerator and denominator on the left and right of the fraction slash respectively, e.g. ٢/٧2/7.^{[ citation needed ]}

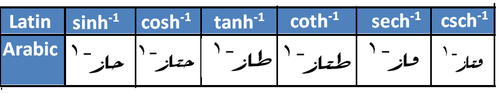
Symbols

Sometimes, symbols used in Arabic mathematical notation differ according to the region:

Latin	Arabic	Persian

$lim x \to\infty x 4$	$س ٤ نهــــــــــــا س\leftarrow\infty ‏$ ^[a]	$س ۴ حــــــــــــد س\leftarrow\infty ‏$ ^[b]

^a نهــــاnūn-hāʾ-ʾalif is derived from the first three letters of Arabic نهايةnihāya "limit".
^b حدḥadd is Persian for "limit".

Sometimes, mirrored Latin and Greek symbols are used in Arabic mathematical notation (especially in western Arabic regions):

Latin	Arabic	Mirrored Latin and Greek

$n \sum x =0 3 \sqrt x$	$٣ ‭ \sqrt ‬ س ں مجــــــــــــ س=٠$ ^[c]	$‪ \sqrt 3 ‬ س ں ‭ \sum ‬ س=0$

^c مجــــ is derived from Arabic مجموعmaǧmūʿ "sum".

However, in Iran, usually Latin and Greek symbols are used.

Examples

Mathematical letters

Latin		Notes
$a$	$ا$	From the Arabic letter اʾalif; a and اʾalif are the first letters of the Latin alphabet and the Arabic alphabet's ʾabjadī sequence respectively, and the letters also share a common ancestor and the same sound
$b$	$ٮ$	A dotless بbāʾ; b and بbāʾ are the second letters of the Latin alphabet and the ʾabjadī sequence respectively
$c$	$حــــ$	From the initial form of حḥāʾ, or that of a dotless جjīm; c and جjīm are the third letters of the Latin alphabet and the ʾabjadī sequence respectively, and the letters also share a common ancestor and the same sound
$d$	$د$	From the Arabic letter دdāl; d and دdāl are the fourth letters of the Latin alphabet and the ʾabjadī sequence respectively, and the letters also share a common ancestor and the same sound
$x$	$س$	From the Arabic letter سsīn. It is contested that the usage of Latin x in maths is derived from the first letter شšīn (without its dots) of the Arabic word شيءšayʾ(un) [ʃajʔ(un)] , meaning thing.^[2] (X was used in old Spanish for the sound /ʃ/). However, according to others there is no historical evidence for this.^[3]^[4]
$y$	$ص$	From the Arabic letter صṣād
$z$	$ع$	From the Arabic letter عʿayn

Mathematical constants and units

Description	Latin		Notes
Euler's number	$e$	$ھ$	Initial form of the Arabic letter هhāʾ. Both Latin letter e and Arabic letter هhāʾ are descendants of Phoenician letter hē.
imaginary unit	$i$	$ت$	From تtāʾ, which is in turn derived from the first letter of the second word of وحدة تخيليةwaḥdaẗun taḫīliyya "imaginary unit"
pi	$\pi$	$ط$	From طṭāʾ; also $\pi$ in some regions
radius	$r$	$نٯ$	From نnūn followed by a dotless قqāf, which is in turn derived from نصف القطرnuṣfu l-quṭr "radius"
kilogram	kg	$كجم$	From كجمkāf-jīm-mīm. In some regions alternative symbols like ( $كغ$ kāf-ġayn) or ( $كلغ$ kāf-lām-ġayn) are used. All three abbreviations are derived from كيلوغرامkīlūġrām "kilogram" and its variant spellings.
gram	g	$جم$	From جمjīm-mīm, which is in turn derived from جرامjrām, a variant spelling of غرامġrām "gram"
metre	m	$م$	From مmīm, which is in turn derived from مترmitr "metre"
centimetre	cm	$سم$	From سمsīn-mīm, which is in turn derived from سنتيمتر "centimetre"
millimetre	mm	$مم$	From ممmīm-mīm, which is in turn derived from مليمترmillīmitr "millimetre"
kilometre	km	$كم$	From كمkāf-mīm; also ( $كلم$ kāf-lām-mīm) in some regions; both are derived from كيلومترkīlūmitr "kilometre".
second	s	$ث$	From ثṯāʾ, which is in turn derived from ثانيةṯāniya "second"
minute	min	$د$	From دdālʾ, which is in turn derived from دقيقةdaqīqa "minute"; also ( $ٯ$ , i.e. dotless قqāf) in some regions
hour	h	$س$	From سsīnʾ, which is in turn derived from ساعةsāʿa "hour"
kilometre per hour	km/h	$كم/س$	From the symbols for kilometre and hour
degree Celsius	°C	$°س$	From سsīn, which is in turn derived from the second word of درجة سيلسيوسdarajat sīlsīūs "degree Celsius"; also ( $°م$ ) from مmīmʾ, which is in turn derived from the first letter of the third word of درجة حرارة مئوية "degree centigrade"
degree Fahrenheit	°F	$°ف$	From فfāʾ, which is in turn derived from the second word of درجة فهرنهايتdarajat fahranhāyt "degree Fahrenheit"
millimetres of mercury	mmHg	$مم ‌ ز$	From مم‌زmīm-mīmzayn, which is in turn derived from the initial letters of the words مليمتر زئبق "millimetres of mercury"
Ångström	Å	$أْ$	From أْʾalif with hamzah and ring above, which is in turn derived from the first letter of "Ångström", variously spelled أنغستروم or أنجستروم

Sets and number systems

Description	Latin	Arabic		Notes
Natural numbers	$\mathbb {N}$		$ط$	From طṭāʾ, which is in turn derived from the first letter of the second word of عدد طبيعيʿadadun ṭabīʿiyyun "natural number"
Integers	$\mathbb {Z}$		$ص$	From صṣād, which is in turn derived from the first letter of the second word of عدد صحيحʿadadun ṣaḥīḥun "integer"
Rational numbers	$\mathbb {Q}$		$ن$	From نnūn, which is in turn derived from the first letter of نسبةnisba "ratio"
Real numbers	$\mathbb {R}$		$ح$	From حḥāʾ, which is in turn derived from the first letter of the second word of عدد حقيقيʿadadun ḥaqīqiyyun "real number"
Imaginary numbers	$\mathbb {I}$		$ت$	From تtāʾ, which is in turn derived from the first letter of the second word of عدد تخيليʿadadun taḫīliyyun "imaginary number"
Complex numbers	$\mathbb {C}$		$م$	From مmīm, which is in turn derived from the first letter of the second word of عدد مركبʿadadun murakkabun "complex number"
Empty set	$\varnothing$	$\varnothing$	∅
Is an element of	$\in$	$\ni$	∈	A mirrored ∈
Subset	$\subset$	$\supset$	⊂	A mirrored ⊂
Superset	$\supset$	$\subset$	⊃	A mirrored ⊃
Universal set	$\mathbf {S}$		$ش$	From شšīn, which is in turn derived from the first letter of the second word of مجموعة شاملةmajmūʿatun šāmila "universal set"

Arithmetic and algebra

Description	Latin/Greek		Notes
Percent	%	$٪$	e.g. 100% "٪١٠٠"
Permille	‰	$؉$	$؊$ is an Arabic equivalent of the per ten thousand sign ‱.
Is proportional to	$\propto$	∝	A mirrored ∝
n ^th root	${\sqrt[{n}]{\,\,\,}}$	$ں ‭ \sqrt ‬ ‏$	ں is a dotless نnūn while √ is a mirrored radical sign √
Logarithm	$\log$	$لو$	From لوlām-wāw, which is in turn derived from لوغاريتم lūġārītm "logarithm"
Logarithm to base b	$\log _{b}$	$لو ٮ$
Natural logarithm	$\ln$	$لو ھ$	From the symbols of logarithm and Euler's number
Summation	$\sum$	$مجــــ$	مجـــmīm-medial form of jīm is derived from the first two letters of مجموعmajmūʿ "sum"; also (∑, a mirrored summation sign ∑) in some regions
Product	$\prod$	$جــــذ$	From جذjīm-ḏāl. The Arabic word for "product" is جداء jadāʾun. Also $\prod$ in some regions.
Factorial	$n!$	$ں$	Also ( $ں!$ ) in some regions
Permutations	$^{n}\mathbf {P} _{r}$	$ں ل ر$	Also ( $ل(ں، ر)$ ) is used in some regions as $\mathbf {P} (n,r)$
Combinations	$^{n}\mathbf {C} _{k}$	$ں ٯ ك$	Also ( $ٯ(ں، ك)$ ) is used in some regions as $\mathbf {C} (n,k)$ and ( $⎛ ⎝ ں ك ⎞ ⎠$ ) as the binomial coefficient $n \choose k$

Trigonometric and hyperbolic functions

Trigonometric functions

Description	Latin		Notes
Sine	$\sin$	$حا$	from حاءḥāʾ (i.e. dotless جjīm)-ʾalif; also ( $جب$ jīm-bāʾ) is used in some regions (e.g. Syria); Arabic for "sine" is جيبjayb
Cosine	$\cos$	$حتا$	from حتاḥāʾ (i.e. dotless جjīm)-tāʾ-ʾalif; also ( $تجب$ tāʾ-jīm-bāʾ) is used in some regions (e.g. Syria); Arabic for "cosine" is جيب تمام
Tangent	$\tan$	$طا$	from طاṭāʾ (i.e. dotless ظẓāʾ)-ʾalif; also ( $ظل$ ẓāʾ-lām) is used in some regions (e.g. Syria); Arabic for "tangent" is ظلẓill
Cotangent	$\cot$	$طتا$	from طتاṭāʾ (i.e. dotless ظẓāʾ)-tāʾ-ʾalif; also ( $تظل$ tāʾ-ẓāʾ-lām) is used in some regions (e.g. Syria); Arabic for "cotangent" is ظل تمام
Secant	$\sec$	$ٯا$	from ٯا dotless قqāf-ʾalif; Arabic for "secant" is قاطع
Cosecant	$\csc$	$ٯتا$	from ٯتا dotless قqāf-tāʾ-ʾalif; Arabic for "cosecant" is قاطع تمام

Hyperbolic functions

The letter ( $ز$ zayn, from the first letter of the second word of دالة زائدية "hyperbolic function") is added to the end of trigonometric functions to express hyperbolic functions. This is similar to the way $\operatorname {h}$ is added to the end of trigonometric functions in Latin-based notation.


Description	Hyperbolic sine	Hyperbolic cosine	Hyperbolic tangent	Hyperbolic cotangent	Hyperbolic secant	Hyperbolic cosecant
Latin	$\sinh$	$\cosh$	$\tanh$	$\coth$	$\operatorname {sech}$	$\operatorname {csch}$
Arabic	$حاز$	$حتاز$	$طاز$	$طتاز$	$ٯاز$	$ٯتاز$

Inverse trigonometric functions

For inverse trigonometric functions, the superscript $-١$ in Arabic notation is similar in usage to the superscript $-1$ in Latin-based notation.


Description	Inverse sine	Inverse cosine	Inverse tangent	Inverse cotangent	Inverse secant	Inverse cosecant
Latin	$\sin ^{-1}$	$\cos ^{-1}$	$\tan ^{-1}$	$\cot ^{-1}$	$\sec ^{-1}$	$\csc ^{-1}$
Arabic	$حا -١$	$حتا -١$	$طا -١$	$طتا -١$	$ٯا -١$	$ٯتا -١$

Inverse hyperbolic functions


Description	Inverse hyperbolic sine	Inverse hyperbolic cosine	Inverse hyperbolic tangent	Inverse hyperbolic cotangent	Inverse hyperbolic secant	Inverse hyperbolic cosecant
Latin	$\sinh ^{-1}$	$\cosh ^{-1}$	$\tanh ^{-1}$	$\coth ^{-1}$	$\operatorname {sech} ^{-1}$	$\operatorname {csch} ^{-1}$
Arabic	$حاز -١$	$حتاز -١$	$طاز -١$	$طتاز -١$	$ٯاز -١$	$ٯتاز -١$

Calculus

Description	Latin		Notes
Limit	$\lim$	$نهــــا$	نهــــاnūn-hāʾ-ʾalif is derived from the first three letters of Arabic نهايةnihāya "limit"
Function	$\mathbf {f} (x)$	$د(س)$	دdāl is derived from the first letter of دالة "function". Also called تابع, تا for short, in some regions.
Derivatives	$\mathbf {f'} (x),{\dfrac {dy}{dx}},{\dfrac {d^{2}y}{dx^{2}}},{\dfrac {\partial {y}}{\partial {x}}}$	$.mw-parser-output .sfrac{white-space:nowrap}.mw-parser-output .sfrac.tion,.mw-parser-output .sfrac .tion{display:inline-block;vertical-align:-0.5em;font-size:85%;text-align:center}.mw-parser-output .sfrac .num{display:block;line-height:1em;margin:0.0em 0.1em;border-bottom:1px solid}.mw-parser-output .sfrac .den{display:block;line-height:1em;margin:0.1em 0.1em}.mw-parser-output .sr-only{border:0;clip:rect(0,0,0,0);clip-path:polygon(0px 0px,0px 0px,0px 0px);height:1px;margin:-1px;overflow:hidden;padding:0;position:absolute;width:1px}⁠ص∂/س∂⁠ ،⁠د٢ص/ د‌س٢⁠ ،⁠د‌ص/ د‌س ⁠ ،(س)‵د$	‵ is a mirrored prime ′ while ، is an Arabic comma. The $\partial$ signs should be mirrored: ∂.
Integrals	$\int {},\iint {},\iiint {},\oint {}$	‪∮ ،∭ ،∬ ،∫‬	Mirrored ∫, ∬, ∭ and ∮

Complex analysis

Latin/Greek	Arabic
$z=x+iy=r(\cos {\varphi }+i\sin {\varphi })=re^{i\varphi }=r\angle {\varphi }$
	$ع = س + ت ص = ل(حتا ى + ت حا ى) = ل ھ ت ‌ ى = ل ∠ ى$

Related Research Articles

A, or a, is the first letter and the first vowel letter of the Latin alphabet, used in the modern English alphabet, and others worldwide. Its name in English is a, plural aes.

The Arabic alphabet, or the Arabic abjad, is the Arabic script as specifically codified for writing the Arabic language. It is written from right-to-left in a cursive style, and includes 28 letters, of which most have contextual letterforms. The Arabic alphabet is considered an abjad, with only consonants required to be written; due to its optional use of diacritics to notate vowels, it is considered an impure abjad.

Gamma is the third letter of the Greek alphabet. In the system of Greek numerals it has a value of 3. In Ancient Greek, the letter gamma represented a voiced velar stop IPA:[ɡ]. In Modern Greek, this letter normally represents a voiced velar fricative IPA:[ɣ], except before either of the two front vowels, where it represents a voiced palatal fricative IPA:[ʝ]; while /g/ in foreign words is instead commonly transcribed as γκ).

<span class="mw-page-title-main">Roman numerals</span> Numbers in the Roman numeral system

Roman numerals are a numeral system that originated in ancient Rome and remained the usual way of writing numbers throughout Europe well into the Late Middle Ages. Numbers are written with combinations of letters from the Latin alphabet, each letter with a fixed integer value. Modern style uses only these seven:

Although people in many parts of the world share common alphabets and numeral systems, styles of handwritten letterforms vary between individuals, and sometimes also vary systematically between regions.

Greek numerals, also known as Ionic, Ionian, Milesian, or Alexandrian numerals, is a system of writing numbers using the letters of the Greek alphabet. In modern Greece, they are still used for ordinal numbers and in contexts similar to those in which Roman numerals are still used in the Western world. For ordinary cardinal numbers, however, modern Greece uses Arabic numerals.

Pi is the sixteenth letter of the Greek alphabet, meaning units united, and representing the voiceless bilabial plosive IPA:[p]. In the system of Greek numerals it has a value of 80. It was derived from the Phoenician letter Pe. Letters that arose from pi include Latin P, Cyrillic Pe, Coptic pi, and Gothic pairthra (𐍀).

Theta uppercase Θ or ϴ; lowercase θ or ϑ; Ancient Greek: θῆταthē̂ta ; Modern: θήταthī́ta ) is the eighth letter of the Greek alphabet, derived from the Phoenician letter Teth . In the system of Greek numerals, it has a value of 9.

The plus sign and the minus sign are mathematical symbols used to denote positive and negative functions, respectively. In addition, + represents the operation of addition, which results in a sum, while − represents subtraction, resulting in a difference. Their use has been extended to many other meanings, more or less analogous. Plus and minus are Latin terms meaning "more" and "less", respectively.

Mathematical notation consists of using symbols for representing operations, unspecified numbers, relations, and any other mathematical objects and assembling them into expressions and formulas. Mathematical notation is widely used in mathematics, science, and engineering for representing complex concepts and properties in a concise, unambiguous, and accurate way.

Iteration marks are characters or punctuation marks that represent a duplicated character or word.

There are various stylistic and typographic variations to the Arabic numeral system.

<span class="mw-page-title-main">Scribal abbreviation</span> Abbreviations used by ancient and medieval scribes

Scribal abbreviations, or sigla, are abbreviations used by ancient and medieval scribes writing in various languages, including Latin, Greek, Old English and Old Norse.

Shin is the twenty-first and penultimate letter of the Semitic abjads, including Arabic šīnش‎‎‎, Aramaic šīn 𐡔, Hebrew šīnש‎, Phoenician šīn 𐤔 and Syriac šīn ܫ.

Aleph is the first letter of the Semitic abjads, including Arabic ʾalifا‎, Aramaic ʾālap 𐡀, Hebrew ʾālefא‎, North Arabian 𐪑, Phoenician ʾālep 𐤀, Syriac ʾālap̄ ܐ. It also appears as South Arabian 𐩱 and Ge'ez ʾälef አ.

<span class="mw-page-title-main">Eastern Arabic numerals</span> Numerals used in the eastern Arab world and Asia

The Eastern Arabic numerals, also called Indo-Arabic numerals, are the symbols used to represent numerical digits in conjunction with the Arabic alphabet in the countries of the Mashriq, the Arabian Peninsula, and its variant in other countries that use the Persian numerals on the Iranian plateau and in Asia.

L, or l, is the twelfth letter of the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is el, plural els.

The history of mathematical notation includes the commencement, progress, and cultural diffusion of mathematical symbols and the conflict of the methods of notation confronted in a notation's move to popularity or inconspicuousness. Mathematical notation comprises the symbols used to write mathematical equations and formulas. Notation generally implies a set of well-defined representations of quantities and symbols operators. The history includes Hindu–Arabic numerals, letters from the Roman, Greek, Hebrew, and German alphabets, and a host of symbols invented by mathematicians over the past several centuries.

A numeral is a character that denotes a number. The decimal number digits 0–9 are used widely in various writing systems throughout the world, however the graphemes representing the decimal digits differ widely. Therefore Unicode includes 22 different sets of graphemes for the decimal digits, and also various decimal points, thousands separators, negative signs, etc. Unicode also includes several non-decimal numerals such as Aegean numerals, Roman numerals, counting rod numerals, Mayan numerals, Cuneiform numerals and ancient Greek numerals. There is also a large number of typographical variations of the Western Arabic numerals provided for specialized mathematical use and for compatibility with earlier character sets, such as ² or ②, and composite characters such as ½.

The Unicode Standard assigns various properties to each Unicode character and code point.

References

↑ Oaks (2012). "Algebraic symbolism in medieval Arabic algebra" Philosophia, 87 27--83.
↑ Moore, Terry. "Why is X the Unknown". Ted Talk. Archived from the original on 2014-02-22. Retrieved 2012-10-11.
↑ Cajori, Florian (1993). A History of Mathematical Notation . Courier Dover Publications. pp. 382–383. ISBN 9780486677668 . Retrieved 11 October 2012. Nor is there historical evidence to support the statement found in Noah Webster's Dictionary, under the letter x, to the effect that 'x was used as an abbreviation of Ar. shei (a thing), something, which, in the Middle Ages, was used to designate the unknown, and was then prevailingly transcribed as xei.'
↑ Oxford Dictionary, 2nd Edition. There is no evidence in support of the hypothesis that x is derived ultimately from the mediaeval transliteration xei of shei "thing", used by the Arabs to denote the unknown quantity, or from the compendium for L. res "thing" or radix "root" (resembling a loosely-written x), used by mediaeval mathematicians.

External links

Multilingual mathematical e-document processing
Arabic mathematical notation - W3C Interest Group Note.
Arabic math editor - by WIRIS.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Oaks (2012). "Algebraic symbolism in medieval Arabic algebra" Philosophia, 87 27--83.

[2] Moore, Terry. "Why is X the Unknown". Ted Talk. Archived from the original on 2014-02-22. Retrieved 2012-10-11.

[3] Cajori, Florian (1993). A History of Mathematical Notation . Courier Dover Publications. pp. 382–383. ISBN 9780486677668 . Retrieved 11 October 2012. Nor is there historical evidence to support the statement found in Noah Webster's Dictionary, under the letter x, to the effect that 'x was used as an abbreviation of Ar. shei (a thing), something, which, in the Middle Ages, was used to designate the unknown, and was then prevailingly transcribed as xei.'

[4] Oxford Dictionary, 2nd Edition. There is no evidence in support of the hypothesis that x is derived ultimately from the mediaeval transliteration xei of shei "thing", used by the Arabs to denote the unknown quantity, or from the compendium for L. res "thing" or radix "root" (resembling a loosely-written x), used by mediaeval mathematicians.

[1]

[2]

[3]

[4]