The musical operation of scalar transposition shifts every note in a melody by the same number of scale steps. The musical operation of chromatic transposition shifts every note in a melody by the same distance in pitch class space. In general, for a given scale S, the scalar transpositions of a line L can be grouped into categories, or transpositional set classes, whose members are related by chromatic transposition. In diatonic set theory cardinality equals variety when, for any melodic line L in a particular scale S, the number of these classes is equal to the number of distinct pitch classes in the line L.
In music transposition refers to the process, or operation, of moving a collection of notes up or down in pitch by a constant interval.
The shifting of a melody, a harmonic progression or an entire musical piece to another key, while maintaining the same tone structure, i.e. the same succession of whole tones and semitones and remaining melodic intervals.

In music, a pitch class (p.c. or pc) is a set of all pitches that are a whole number of octaves apart, e.g., the pitch class C consists of the Cs in all octaves. "The pitch class C stands for all possible Cs, in whatever octave position." Important to musical set theory, a pitch class is, "all pitches related to each other by octave, enharmonic equivalence, or both." Thus, using scientific pitch notation, the pitch class "C" is the set

Musical set theory provides concepts for categorizing musical objects and describing their relationships. Howard Hanson first elaborated many of the concepts for analyzing tonal music. Other theorists, such as Allen Forte, further developed the theory for analyzing atonal music, drawing on the twelve-tone theory of Milton Babbitt. The concepts of musical set theory are very general and can be applied to tonal and atonal styles in any equal temperament tuning system, and to some extent more generally than that.
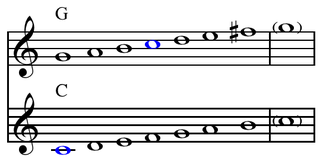
For example, the melodic line C-D-E has three distinct pitch classes. When transposed diatonically to all scale degrees in the C major scale, we obtain three interval patterns: M2-M2, M2-m2, m2-M2.

Melodic lines in the C major scale with n distinct pitch classes always generate n distinct patterns.
The property was first described by John Clough and Gerald Myerson in "Variety and Multiplicity in Diatonic Systems" (1985) (Johnson 2003, p. 68, 151). Cardinality equals variety in the diatonic collection and the pentatonic scale, and, more generally, what Carey and Clampitt (1989) call "nondegenerate well-formed scales." "Nondegenerate well-formed scales" are those that possess Myhill's property.
John Clough is a rugby league footballer playing for Salford City Reds (2004–06), London Broncos, Halifax (2006), Leigh Centurions (2007), Blackpool Panthers (2010) (captain), and Oldham (2011) as a hooker. John Clough is a former Lancashire Academy representative.
A pentatonic scale is a musical scale with five notes per octave, in contrast to the more familiar heptatonic scale that has seven notes per octave.
In western music theory, a diatonic scale is a heptatonic scale that includes five whole steps and two half steps (semitones) in each octave, in which the two half steps are separated from each other by either two or three whole steps, depending on their position in the scale. This pattern ensures that, in a diatonic scale spanning more than one octave, all the half steps are maximally separated from each other.
In music theory, a scale is any set of musical notes ordered by fundamental frequency or pitch. A scale ordered by increasing pitch is an ascending scale, and a scale ordered by decreasing pitch is a descending scale. Some scales contain different pitches when ascending than when descending, for example, the melodic minor scale.
In music theory, an interval is the difference in pitch between two sounds.
An interval may be described as horizontal, linear, or melodic if it refers to successively sounding tones, such as two adjacent pitches in a melody, and vertical or harmonic if it pertains to simultaneously sounding tones, such as in a chord.
An octatonic scale is any eight-note musical scale. However, the term most often refers to the symmetric scale composed of alternating whole and half steps, as shown at right. In classical theory, this scale is commonly simply called the octatonic scale although there are a total of 42 non-enharmonically equivalent, non-transpositionally equivalent eight-note sets.

A semitone, also called a half step or a half tone, is the smallest musical interval commonly used in Western tonal music, and it is considered the most dissonant when sounded harmonically.
It is defined as the interval between two adjacent notes in a 12-tone scale. For example, C is adjacent to C♯; the interval between them is a semitone.
Chromaticism is a compositional technique interspersing the primary diatonic pitches and chords with other pitches of the chromatic scale. Chromaticism is in contrast or addition to tonality or diatonicism. Chromatic elements are considered "elaborations of or substitutions for diatonic scale members".
Chromaticism is almost by definition an alteration of, an interpolation in or deviation from this basic diatonic organization.
Genus is a term used in the Ancient Greek and Roman theory of music to describe certain classes of intonations of the two movable notes within a tetrachord.
In diatonic set theory structure implies multiplicity is a quality of a collection or scale. This is that for the interval series formed by the shortest distance around a diatonic circle of fifths between members of a series indicates the number of unique interval patterns formed by diatonic transpositions of that series. Structure being the intervals in relation to the circle of fifths, multiplicity being the number of times each different (adjacent) interval pattern occurs. The property was first described by John Clough and Gerald Myerson in "Variety and Multiplicity in Diatonic Systems" (1985).
In diatonic set theory, a generated collection is a collection or scale formed by repeatedly adding a constant interval in integer notation, the generator, also known as an interval cycle, around the chromatic circle until a complete collection or scale is formed. All scales with the deep scale property can be generated by any interval coprime with twelve.
Diatonic set theory is a subdivision or application of musical set theory which applies the techniques and insights of discrete mathematics to properties of the diatonic collection such as maximal evenness, Myhill's property, well formedness, the deep scale property, cardinality equals variety, and structure implies multiplicity. The name is something of a misnomer as the concepts involved usually apply much more generally, to any periodically repeating scale.

The chromatic circle is a geometrical space that shows relationships among the 12 equal-tempered pitch classes making up the familiar chromatic scale on a circle.

In diatonic set theory, maximal evenness is a quality of a collection or scale in which every generic interval has either one or two consecutive (adjacent) specific intervals—in other words a scale that is "spread out as much as possible." This property was first described by music theorist John Clough and mathematician Jack Douthett in "Maximally Even Sets" (1991).

In diatonic set theory a generic interval is the number of scale steps between notes of a collection or scale. The largest generic interval is one less than the number of scale members.

In diatonic set theory, a bisector divides the octave approximately in half and may be used in place of a generator to derive collections for which structure implies multiplicity is not true such as the ascending melodic minor, harmonic minor, and octatonic scales. Well formed generated collections generators and bisectors coincide, such as the perfect fifth in the diatonic collection. The term was introduced by Jay Rahn (1977), who considers any division between one and two thirds as approximately half and who applied the term only the equally spaced collections. Clough and Johnson both adapt the term to apply to generic scale steps. Rahn also uses aliquant bisector for bisectors which may be used to generate every note in a collection, in which case the bisector and the number of notes must be coprime. Bisectors may be used to produce the diatonic, harmonic minor, and ascending melodic minor collections.
In music, polymodal chromaticism is the use of any and all musical modes sharing the same tonic simultaneously or in succession and thus creating a texture involving all twelve notes of the chromatic scale. Alternately it is the free alteration of the other notes in a mode once its tonic has been established.
Jaroslav Volek was a Czech musicologist, semiotician who developed a theory of modal music. His theory included ideas of poly-modality and alteration of notes that he called "flex," which result in what he called the system of flexible diatonics. He applied this theory to the work of Béla Bartók and Leoš Janáček. He wrote General Theory of Art based on semiotic concepts in 1968.

Diatonic and chromatic are terms in music theory that are most often used to characterize scales, and are also applied to musical instruments, intervals, chords, notes, musical styles, and kinds of harmony. They are very often used as a pair, especially when applied to contrasting features of the common practice music of the period 1600–1900.
In music, a symmetric scale is a music scale which equally divides the octave. The concept and term appears to have been introduced by Joseph Schillinger and further developed by Nicolas Slonimsky as part of his famous "Thesaurus of Scales and Melodic Patterns". In twelve-tone equal temperament, the octave can only be equally divided into two, three, four, six, or twelve parts, which consequently may be filled in by adding the same exact interval or sequence of intervals to each resulting note.
In music, a common tone is a pitch class that is a member of, or common to two or more scales or sets.










