In music theory, a diatonic scale is any heptatonic scale that includes five whole steps and two half steps (semitones) in each octave, in which the two half steps are separated from each other by either two or three whole steps, depending on their position in the scale. This pattern ensures that, in a diatonic scale spanning more than one octave, all the half steps are maximally separated from each other.

The major scale is one of the most commonly used musical scales, especially in Western music. It is one of the diatonic scales. Like many musical scales, it is made up of seven notes: the eighth duplicates the first at double its frequency so that it is called a higher octave of the same note.
In music theory, an interval is a difference in pitch between two sounds. An interval may be described as horizontal, linear, or melodic if it refers to successively sounding tones, such as two adjacent pitches in a melody, and vertical or harmonic if it pertains to simultaneously sounding tones, such as in a chord.
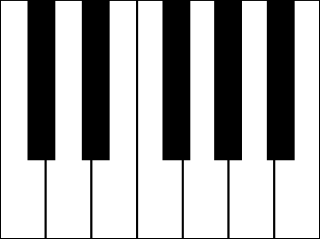
The chromatic scale is a set of twelve pitches used in tonal music, with notes separated by the interval of a semitone. Chromatic instruments, such as the piano, are made to produce the chromatic scale, while other instruments capable of continuously variable pitch, such as the trombone and violin, can also produce microtones, or notes between those available on a piano.
In music theory, a tetrachord is a series of four notes separated by three intervals. In traditional music theory, a tetrachord always spanned the interval of a perfect fourth, a 4:3 frequency proportion —but in modern use it means any four-note segment of a scale or tone row, not necessarily related to a particular tuning system.

A semitone, also called a half step or a half tone, is the smallest musical interval commonly used in Western tonal music, and it is considered the most dissonant when sounded harmonically. It is defined as the interval between two adjacent notes in a 12-tone scale. For example, C is adjacent to C♯; the interval between them is a semitone.
Chromaticism is a compositional technique interspersing the primary diatonic pitches and chords with other pitches of the chromatic scale. In simple terms, within each octave, diatonic music uses only seven different notes, rather than the twelve available on a standard piano keyboard. Music is chromatic when it uses more than just these seven notes.

The musical operation of scalar transposition shifts every note in a melody by the same number of scale steps. The musical operation of chromatic transposition shifts every note in a melody by the same distance in pitch class space. In general, for a given scale S, the scalar transpositions of a line L can be grouped into categories, or transpositional set classes, whose members are related by chromatic transposition. In diatonic set theory cardinality equals variety when, for any melodic line L in a particular scale S, the number of these classes is equal to the number of distinct pitch classes in the line L.
In diatonic set theory structure implies multiplicity is a quality of a collection or scale. For collections or scales which have this property, the interval series formed by the shortest distance around a diatonic circle of fifths between members of a series indicates the number of unique interval patterns formed by diatonic transpositions of that series. Structure refers to the intervals in relation to the circle of fifths; multiplicity refers to the number of times each different (adjacent) interval pattern occurs. The property was first described by John Clough and Gerald Myerson in "Variety and Multiplicity in Diatonic Systems" (1985).
In diatonic set theory, a generated collection is a collection or scale formed by repeatedly adding a constant interval in integer notation, the generator, also known as an interval cycle, around the chromatic circle until a complete collection or scale is formed. All scales with the deep scale property can be generated by any interval coprime with twelve.
Diatonic set theory is a subdivision or application of musical set theory which applies the techniques and analysis of discrete mathematics to properties of the diatonic collection such as maximal evenness, Myhill's property, well formedness, the deep scale property, cardinality equals variety, and structure implies multiplicity. The name is something of a misnomer as the concepts involved usually apply much more generally, to any periodically repeating scale.

In diatonic set theory a generic interval is the number of scale steps between notes of a collection or scale. The largest generic interval is one less than the number of scale members.

A heptatonic scale is a musical scale that has seven pitches, or tones, per octave. Examples include:

In music theory, pitch-class space is the circular space representing all the notes in a musical octave. In this space, there is no distinction between tones that are separated by an integral number of octaves. For example, C4, C5, and C6, though different pitches, are represented by the same point in pitch class space.

Transformational theory is a branch of music theory developed by David Lewin in the 1980s, and formally introduced in his 1987 work, Generalized Musical Intervals and Transformations. The theory—which models musical transformations as elements of a mathematical group—can be used to analyze both tonal and atonal music.

Diatonic and chromatic are terms in music theory that are most often used to characterize scales, and are also applied to musical instruments, intervals, chords, notes, musical styles, and kinds of harmony. They are very often used as a pair, especially when applied to contrasting features of the common practice music of the period 1600–1900.
In music, a symmetric scale is a music scale which equally divides the octave. The concept and term appears to have been introduced by Joseph Schillinger and further developed by Nicolas Slonimsky as part of his famous Thesaurus of Scales and Melodic Patterns. In twelve-tone equal temperament, the octave can only be equally divided into two, three, four, six, or twelve parts, which consequently may be filled in by adding the same exact interval or sequence of intervals to each resulting note.

Neo-Riemannian theory is a loose collection of ideas present in the writings of music theorists such as David Lewin, Brian Hyer, Richard Cohn, and Henry Klumpenhouwer. What binds these ideas is a central commitment to relating harmonies directly to each other, without necessary reference to a tonic. Initially, those harmonies were major and minor triads; subsequently, neo-Riemannian theory was extended to standard dissonant sonorities as well. Harmonic proximity is characteristically gauged by efficiency of voice leading. Thus, C major and E minor triads are close by virtue of requiring only a single semitonal shift to move from one to the other. Motion between proximate harmonies is described by simple transformations. For example, motion between a C major and E minor triad, in either direction, is executed by an "L" transformation. Extended progressions of harmonies are characteristically displayed on a geometric plane, or map, which portrays the entire system of harmonic relations. Where consensus is lacking is on the question of what is most central to the theory: smooth voice leading, transformations, or the system of relations that is mapped by the geometries. The theory is often invoked when analyzing harmonic practices within the Late Romantic period characterized by a high degree of chromaticism, including work of Schubert, Liszt, Wagner and Bruckner.
In music, a common tone is a pitch class that is a member of, or common to two or more chords or sets. Typically, it refers to a note shared between two chords in a chord progression. According to H.E. Woodruff:
Any tone contained in two successive chords is a common tone. Chords written upon two consecutive degrees of the [diatonic] scale can have no tones in common. All other chords [in the diatonic scale] have common tones. Common tones are also called connecting tones, and in part-writing, are to be retained in the same voice. Chords which are four or five degrees apart have one common tone. Chords which are three or six degrees apart have two common tones. Chords which are one or seven degrees apart have no tone in common.
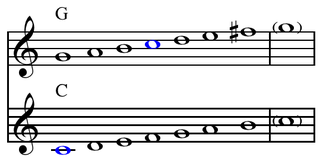
In music, a common tone is a pitch class that is a member of, or common to two or more scales or sets.














