Boron is a chemical element with the symbol B and atomic number 5. In its crystalline form it is a brittle, dark, lustrous metalloid; in its amorphous form it is a brown powder. As the lightest element of the boron group it has three valence electrons for forming covalent bonds, resulting in many compounds such as boric acid, the mineral sodium borate, and the ultra-hard crystals of boron carbide and boron nitride.

William Nunn Lipscomb Jr. was a Nobel Prize-winning American inorganic and organic chemist working in nuclear magnetic resonance, theoretical chemistry, boron chemistry, and biochemistry.
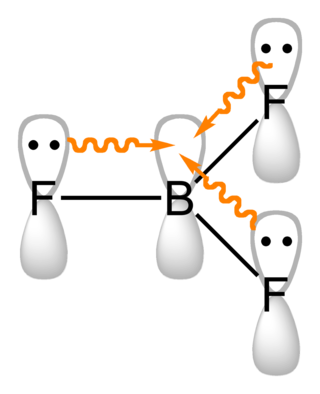
In coordination chemistry, a coordinate covalent bond, also known as a dative bond, dipolar bond, or coordinate bond is a kind of two-center, two-electron covalent bond in which the two electrons derive from the same atom. The bonding of metal ions to ligands involves this kind of interaction. This type of interaction is central to Lewis acid–base theory.

A borane is a compound with the formula BxHy or a related anion. Many such boranes are known. Most common are those with 1 to 12 boron atoms. Although they have few practical applications, the boranes exhibit structures and bonding that differs strongly from the patterns seen in hydrocarbons. Hybrids of boranes and hydrocarbons, the carboranes are also well developed.

Decaborane, also called decaborane(14), is the borane with the chemical formula B10H14. This white crystalline compound is one of the principal boron hydride clusters, both as a reference structure and as a precursor to other boron hydrides. It is toxic and volatile, giving off a foul odor, like that of burnt rubber or chocolate.
Carboranes are electron-delocalized clusters composed of boron, carbon and hydrogen atoms. Like many of the related boron hydrides, these clusters are polyhedra or fragments of polyhedra. Carboranes are one class of heteroboranes.
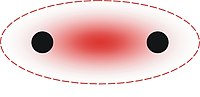
A three-center two-electron (3c–2e) bond is an electron-deficient chemical bond where three atoms share two electrons. The combination of three atomic orbitals form three molecular orbitals: one bonding, one non-bonding, and one anti-bonding. The two electrons go into the bonding orbital, resulting in a net bonding effect and constituting a chemical bond among all three atoms. In many common bonds of this type, the bonding orbital is shifted towards two of the three atoms instead of being spread equally among all three. Example molecules with 3c–2e bonds are the trihydrogen cation and diborane. In these two structures, the three atoms in each 3c-2e bond form an angular geometry, leading to a bent bond.

Organoboron chemistry or organoborane chemistry studies organoboron compounds, also called organoboranes. These chemical compounds combine boron and carbon; typically, they are organic derivatives of borane (BH3), as in the trialkyl boranes.

Borazine, also known as borazole, is an inorganic compound with the chemical formula B3H6N3. In this cyclic compound, the three BH units and three NH units alternate. The compound is isoelectronic and isostructural with benzene. For this reason borazine is sometimes referred to as “inorganic benzene”. Like benzene, borazine is a colourless liquid with an aromatic odor.
In chemistry the polyhedral skeletal electron pair theory (PSEPT) provides electron counting rules useful for predicting the structures of clusters such as borane and carborane clusters. The electron counting rules were originally formulated by Kenneth Wade, and were further developed by others including Michael Mingos; they are sometimes known as Wade's rules or the Wade–Mingos rules. The rules are based on a molecular orbital treatment of the bonding. These rules have been extended and unified in the form of the Jemmis mno rules.
Boron compounds are compounds containing the element boron. In the most familiar compounds, boron has the formal oxidation state +3. These include oxides, sulfides, nitrides, and halides.
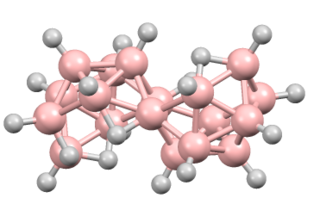
Octadecaborane is an inorganic compound, a borane with chemical formula B18H22. It is a colorless flammable solid, like many higher boron hydrides. Although the compound has no practical applications, its structure is of theoretical and pedagogical interest.

Caesium dodecaborate is an inorganic compound with the formula Cs2B12H12. It is a salt composed of caesium and dodecaborate(12) ions. The [B12H12]2− anion has been of great theoretical interest to the chemistry community.
John David Kennedy is a chemist and emeritus professor of inorganic chemistry at the University of Leeds. He works in the area of polyhedral borane chemistry.

Boron can be prepared in several crystalline and amorphous forms. Well known crystalline forms are α-rhombohedral (α-R), β-rhombohedral (β-R), and β-tetragonal (β-T). In special circumstances, boron can also be synthesized in the form of its α-tetragonal (α-T) and γ-orthorhombic (γ) allotropes. Two amorphous forms, one a finely divided powder and the other a glassy solid, are also known. Although at least 14 more allotropes have been reported, these other forms are based on tenuous evidence or have not been experimentally confirmed, or are thought to represent mixed allotropes, or boron frameworks stabilized by impurities. Whereas the β-rhombohedral phase is the most stable and the others are metastable, the transformation rate is negligible at room temperature, and thus all five phases can exist at ambient conditions. Amorphous powder boron and polycrystalline β-rhombohedral boron are the most common forms. The latter allotrope is a very hard grey material, about ten percent lighter than aluminium and with a melting point (2080 °C) several hundred degrees higher than that of steel.

Eluvathingal Devassy Jemmis is a professor of theoretical chemistry at the Indian Institute of Science, Bangalore, India. He was the founding director of Indian Institute of Science Education and Research, Thiruvananthapuram (IISER-TVM). His primary area of research is applied theoretical chemistry with emphasis on structure, bonding and reactivity, across the periodic table of the elements. Apart from many of his contributions to applied theoretical chemistry, an equivalent of the structural chemistry of carbon, as exemplified by the Huckel 4n+2 Rule, benzenoid aromatics and graphite, and tetrahedral carbon and diamond, is brought in the structural chemistry of boron by the Jemmis mno rules which relates polyhedral and macropolyhedral boranes to allotropes of boron and boron-rich solids. He has been awarded Padma Shri in Science and Engineering category by the Government of India.
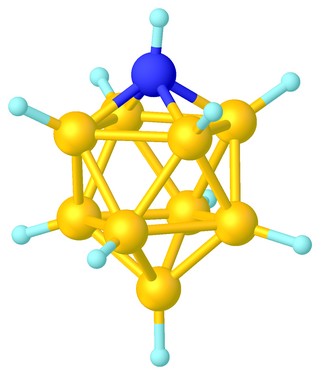
Azaborane usually refers a borane cluster where BH vertices are replaced by N or NR. Like many of the related boranes, these clusters are polyhedra and can be classified as closo-, nido-, arachno-, etc.
Heteroboranes are classes of boranes in which at least one boron atom is replaced by another element. Like many of the related boranes, these clusters are polyhedra and are similarly classified as closo-, nido-, arachno-, and hypho-, according to the so-called electron count. Closo- represents a complete polyhedron, while nido-, arachno- and hypho- stand for polyhedrons that are missing one, two and three vertices.

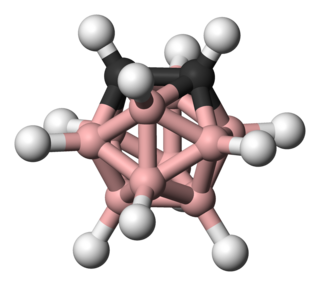
ortho-Carborane is the organoboron compound with the formula C2B10H12. The prefix ortho is derived from ortho. It is the most prominent carborane. This derivative has been considered for a wide range of applications from heat-resistant polymers to medical applications. It is a colorless solid that melts, without decomposition, at 320 °C.

In chemistry, a metallaborane is a compound that contains one or more metal atoms and one or more boron hydride. These compounds are related conceptually and often synthetically to the boron-hydride clusters by replacement of BHn units with metal-containing fragments. Often these metal fragments are derived from metal carbonyls or cyclopentadienyl complexes. Their structures can often be rationalized by polyhedral skeletal electron pair theory. The inventory of these compounds is large, and their structures can be quite complex.