The Mach number, often only Mach, is a dimensionless quantity in fluid dynamics representing the ratio of flow velocity past a boundary to the local speed of sound. It is named after the Czech physicist and philosopher Ernst Mach.
Standard temperature and pressure (STP) or Standard conditions for temperature and pressure are various standard sets of conditions for experimental measurements used to allow comparisons to be made between different sets of data. The most used standards are those of the International Union of Pure and Applied Chemistry (IUPAC) and the National Institute of Standards and Technology (NIST), although these are not universally accepted. Other organizations have established a variety of other definitions.
Atmospheric pressure, also known as air pressure or barometric pressure, is the pressure within the atmosphere of Earth. The standard atmosphere is a unit of pressure defined as 101,325 Pa (1,013.25 hPa), which is equivalent to 1,013.25 millibars, 760 mm Hg, 29.9212 inches Hg, or 14.696 psi. The atm unit is roughly equivalent to the mean sea-level atmospheric pressure on Earth; that is, the Earth's atmospheric pressure at sea level is approximately 1 atm.

The speed of sound is the distance travelled per unit of time by a sound wave as it propagates through an elastic medium. At 20 °C (68 °F), the speed of sound in air is about 343 m/s, or 1 km in 2.91 s or one mile in 4.69 s. It depends strongly on temperature as well as the medium through which a sound wave is propagating. At 0 °C (32 °F), the speed of sound in air is about 331 m/s. More simply, the speed of sound is how fast vibrations travel.
Geopotential height or geopotential altitude is a vertical coordinate referenced to Earth's mean sea level that represents the work involved in lifting one unit of mass over one unit of length through a hypothetical space in which the acceleration of gravity is assumed constant. In SI units, a geopotential height difference of one meter implies the vertical transport of a parcel of one kilogram; adopting the standard gravity value, it corresponds to a constant work or potential energy difference of 9.80665 joules.
In fluid dynamics, the drag equation is a formula used to calculate the force of drag experienced by an object due to movement through a fully enclosing fluid. The equation is:
The primitive equations are a set of nonlinear partial differential equations that are used to approximate global atmospheric flow and are used in most atmospheric models. They consist of three main sets of balance equations:
- A continuity equation: Representing the conservation of mass.
- Conservation of momentum: Consisting of a form of the Navier–Stokes equations that describe hydrodynamical flow on the surface of a sphere under the assumption that vertical motion is much smaller than horizontal motion (hydrostasis) and that the fluid layer depth is small compared to the radius of the sphere
- A thermal energy equation: Relating the overall temperature of the system to heat sources and sinks
The Knudsen number (Kn) is a dimensionless number defined as the ratio of the molecular mean free path length to a representative physical length scale. This length scale could be, for example, the radius of a body in a fluid. The number is named after Danish physicist Martin Knudsen (1871–1949).
The density of air or atmospheric density, denoted ρ, is the mass per unit volume of Earth's atmosphere. Air density, like air pressure, decreases with increasing altitude. It also changes with variations in atmospheric pressure, temperature and humidity. At 101.325 kPa (abs) and 20 °C, air has a density of approximately 1.204 kg/m3 (0.0752 lb/cu ft), according to the International Standard Atmosphere (ISA). At 101.325 kPa (abs) and 15 °C (59 °F), air has a density of approximately 1.225 kg/m3 (0.0765 lb/cu ft), which is about 1⁄800 that of water, according to the International Standard Atmosphere (ISA). Pure liquid water is 1,000 kg/m3 (62 lb/cu ft).

In aviation, airspeed is the speed of an aircraft relative to the air it is flying through. It is difficult to measure the exact airspeed of the aircraft, but other measures of airspeed, such as indicated airspeed and Mach number give useful information about the capabilities and limitations of airplane performance. The common measures of airspeed are:

The true airspeed of an aircraft is the speed of the aircraft relative to the air mass through which it is flying. The true airspeed is important information for accurate navigation of an aircraft. Traditionally it is measured using an analogue TAS indicator, but as the Global Positioning System has become available for civilian use, the importance of such air-measuring instruments has decreased. Since indicated, as opposed to true, airspeed is a better indicator of margin above the stall, true airspeed is not used for controlling the aircraft; for these purposes the indicated airspeed – IAS or KIAS – is used. However, since indicated airspeed only shows true speed through the air at standard sea level pressure and temperature, a TAS meter is necessary for navigation purposes at cruising altitude in less dense air. The IAS meter reads very nearly the TAS at lower altitude and at lower speed. On jet airliners the TAS meter is usually hidden at speeds below 200 knots (370 km/h). Neither provides for accurate speed over the ground, since surface winds or winds aloft are not taken into account.

Indicated airspeed (IAS) is the airspeed of an aircraft as measured by its pitot-static system and displayed by the airspeed indicator (ASI). This is the pilots' primary airspeed reference.

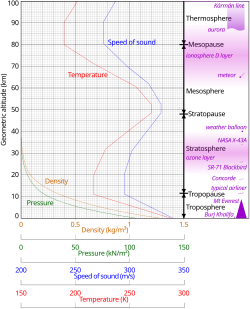
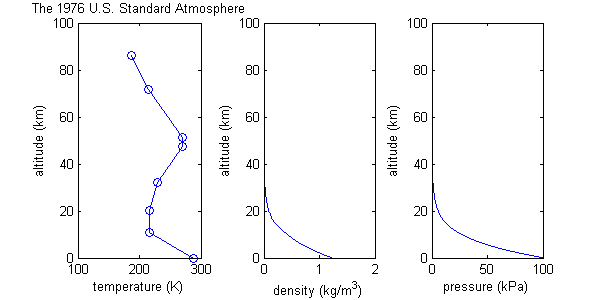
The International Standard Atmosphere (ISA) is a static atmospheric model of how the pressure, temperature, density, and viscosity of the Earth's atmosphere change over a wide range of altitudes or elevations. It has been established to provide a common reference for temperature and pressure and consists of tables of values at various altitudes, plus some formulas by which those values were derived. The International Organization for Standardization (ISO) publishes the ISA as an international standard, ISO 2533:1975. Other standards organizations, such as the International Civil Aviation Organization (ICAO) and the United States Government, publish extensions or subsets of the same atmospheric model under their own standards-making authority.
The barometric formula is a formula used to model how the pressure of the air changes with altitude.

In fluid dynamics, a barotropic fluid is a fluid whose density is a function of pressure only. The barotropic fluid is a useful model of fluid behavior in a wide variety of scientific fields, from meteorology to astrophysics.

In atmospheric, earth, and planetary sciences, a scale height, usually denoted by the capital letter H, is a distance over which a physical quantity decreases by a factor of e.
A reference atmospheric model describes how the ideal gas properties of an atmosphere change, primarily as a function of altitude, and sometimes also as a function of latitude, day of year, etc. A static atmospheric model has a more limited domain, excluding time. A standard atmosphere is defined by the World Meteorological Organization as "a hypothetical vertical distribution of atmospheric temperature, pressure and density which, by international agreement, is roughly representative of year-round, midlatitude conditions."
In fluid mechanics, potential vorticity (PV) is a quantity which is proportional to the dot product of vorticity and stratification. This quantity, following a parcel of air or water, can only be changed by diabatic or frictional processes. It is a useful concept for understanding the generation of vorticity in cyclogenesis, especially along the polar front, and in analyzing flow in the ocean.
The term standard day is used throughout meteorology, aviation, and other sciences and disciplines as a way of defining certain properties of the atmosphere in a manner which allows those who use our atmosphere to effectively calculate and communicate its properties at any given time. For example, a temperature deviation of +8 °C means that the air at any given altitude is 8 °C (14 °F) warmer than what standard day conditions and the measurement altitude would predict, and would indicate a higher density altitude. These variations are extremely important to both meteorologists and aviators, as they strongly determine the different properties of the atmosphere.
Standard sea-level conditions (SSL), also known as sea-level standard (SLS), defines a set of atmospheric conditions for physical calculations. The term "standard sea level" is used to indicate that values of properties are to be taken to be the same as those standard at sea level, and is done to define values for use in general calculations.