Centered triangular number

Last updated August 09, 2024

A centered (or centred) triangular number is a centered figurate number that represents an equilateral triangle with a dot in the center and all its other dots surrounding the center in successive equilateral triangular layers.

Properties

The gnomon of the n-th centered triangular number, corresponding to the (n + 1)-th triangular layer, is:

C_{3,n+1}-C_{3,n}=3(n+1).

The n-th centered triangular number, corresponding to n layers plus the center, is given by the formula:

C_{3,n}=1+3{\frac {n(n+1)}{2}}={\frac {3n^{2}+3n+2}{2}}.

Each centered triangular number has a remainder of 1 when divided by 3, and the quotient (if positive) is the previous regular triangular number.

Each centered triangular number from 10 onwards is the sum of three consecutive regular triangular numbers.

For n > 2, the sum of the first n centered triangular numbers is the magic constant for an n by n normal magic square.

Relationship with centered square numbers

The centered triangular numbers can be expressed in terms of the centered square numbers:

C_{3,n}={\frac {3C_{4,n}+1}{4}},

where

C_{4,n}=n^{2}+(n+1)^{2}.

Lists of centered triangular numbers

The first centered triangular numbers (C_3,n < 3000) are:

1, 4, 10, 19, 31, 46, 64, 85, 109, 136, 166, 199, 235, 274, 316, 361, 409, 460, 514, 571, 631, 694, 760, 829, 901, 976, 1054, 1135, 1219, 1306, 1396, 1489, 1585, 1684, 1786, 1891, 1999, 2110, 2224, 2341, 2461, 2584, 2710, 2839, 2971, … (sequence A005448 in the OEIS ).

The first simultaneously triangular and centered triangular numbers (C_3,n = T_N < 10⁹) are:

1, 10, 136, 1 891, 26 335, 366 796, 5 108 806, 71 156 485, 991 081 981, … (sequence A128862 in the OEIS ).

The generating function

If the centered triangular numbers are treated as the coefficients of the McLaurin series of a function, that function converges for all $|x|<1$ , in which case it can be expressed as the meromorphic generating function

1+4x+10x^{2}+19x^{3}+31x^{4}+~...={\frac {1-x^{3}}{(1-x)^{4}}}={\frac {x^{2}+x+1}{(1-x)^{3}}}~.

Related Research Articles

In mathematics, Pascal's triangle is an infinite triangular array of the binomial coefficients which play a crucial role in probability theory, combinatorics, and algebra. In much of the Western world, it is named after the French mathematician Blaise Pascal, although other mathematicians studied it centuries before him in Persia, India, China, Germany, and Italy.

2 (two) is a number, numeral and digit. It is the natural number following 1 and preceding 3. It is the smallest and the only even prime number.

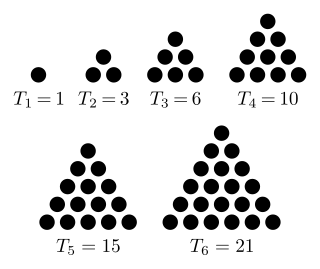
A triangular number or triangle number counts objects arranged in an equilateral triangle. Triangular numbers are a type of figurate number, other examples being square numbers and cube numbers. The $n$ th triangular number is the number of dots in the triangular arrangement with $n$ dots on each side, and is equal to the sum of the $n$ natural numbers from 1 to $n$ . The sequence of triangular numbers, starting with the 0th triangular number, is

In mathematics, a polygonal number is a number that counts dots arranged in the shape of a regular polygon. These are one type of 2-dimensional figurate numbers.

In mathematics, a square triangular number is a number which is both a triangular number and a square number. There are infinitely many square triangular numbers; the first few are:

1000 or one thousand is the natural number following 999 and preceding 1001. In most English-speaking countries, it can be written with or without a comma or sometimes a period separating the thousands digit: 1,000.

700 is the natural number following 699 and preceding 701.

A hexagonal number is a figurate number. The nth hexagonal number h_n is the number of distinct dots in a pattern of dots consisting of the outlines of regular hexagons with sides up to n dots, when the hexagons are overlaid so that they share one vertex.

In mathematics and combinatorics, a centered hexagonal number, or hex number, is a centered figurate number that represents a hexagon with a dot in the center and all other dots surrounding the center dot in a hexagonal lattice. The following figures illustrate this arrangement for the first four centered hexagonal numbers:

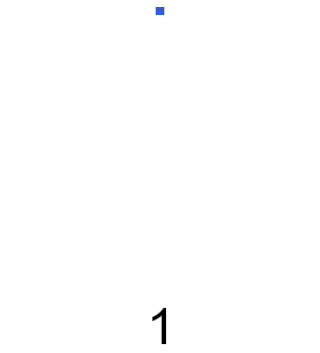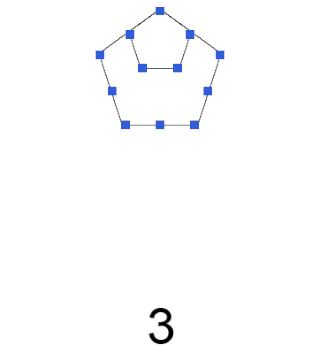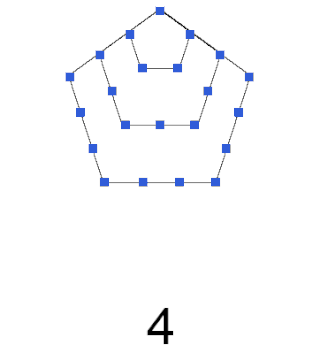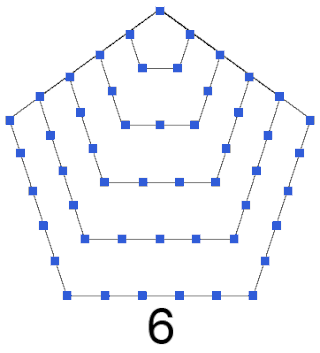
A pentagonal number is a figurate number that extends the concept of triangular and square numbers to the pentagon, but, unlike the first two, the patterns involved in the construction of pentagonal numbers are not rotationally symmetrical. The nth pentagonal number p_n is the number of distinct dots in a pattern of dots consisting of the outlines of regular pentagons with sides up to n dots, when the pentagons are overlaid so that they share one vertex. For instance, the third one is formed from outlines comprising 1, 5 and 10 dots, but the 1, and 3 of the 5, coincide with 3 of the 10 – leaving 12 distinct dots, 10 in the form of a pentagon, and 2 inside.

A heptagonal number is a figurate number that is constructed by combining heptagons with ascending size. The n-th heptagonal number is given by the formula

An octagonal number is a figurate number that gives the number of points in a certain octagonal arrangement. The octagonal number for n is given by the formula 3n² − 2n, with n > 0. The first few octagonal numbers are

In mathematics, a pyramid number, or square pyramidal number, is a natural number that counts the stacked spheres in a pyramid with a square base. The study of these numbers goes back to Archimedes and Fibonacci. They are part of a broader topic of figurate numbers representing the numbers of points forming regular patterns within different shapes.

In elementary number theory, a centered square number is a centered figurate number that gives the number of dots in a square with a dot in the center and all other dots surrounding the center dot in successive square layers. That is, each centered square number equals the number of dots within a given city block distance of the center dot on a regular square lattice. While centered square numbers, like figurate numbers in general, have few if any direct practical applications, they are sometimes studied in recreational mathematics for their elegant geometric and arithmetic properties.

The centered polygonal numbers are a class of series of figurate numbers, each formed by a central dot, surrounded by polygonal layers of dots with a constant number of sides. Each side of a polygonal layer contains one more dot than each side in the previous layer; so starting from the second polygonal layer, each layer of a centered k-gonal number contains k more dots than the previous layer.

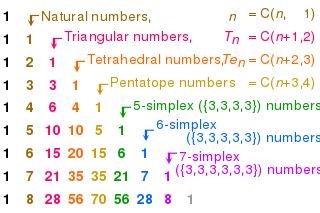
In number theory, a pentatope number is a number in the fifth cell of any row of Pascal's triangle starting with the 5-term row 1 4 6 4 1, either from left to right or from right to left. It is named because it represents the number of 3-dimensional unit spheres which can be packed into a pentatope of increasing side lengths.

A centered nonagonal number is a centered figurate number that represents a nonagon with a dot in the center and all other dots surrounding the center dot in successive nonagonal layers. The centered nonagonal number for n layers is given by the formula

A centered decagonal number is a centered figurate number that represents a decagon with a dot in the center and all other dots surrounding the center dot in successive decagonal layers. The centered decagonal number for n is given by the formula

14 (fourteen) is the natural number following 13 and preceding 15.

A dodecahedral number is a figurate number that represents a dodecahedron. The nth dodecahedral number is given by the formula

References

Lancelot Hogben: Mathematics for the Million (1936), republished by W. W. Norton & Company (September 1993), ISBN 978-0-393-31071-9
Weisstein, Eric W. "Centered Triangular Number". MathWorld .

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.