Centered hexagonal number

Last updated December 12, 2023

In mathematics and combinatorics, a centered hexagonal number, or hex number,^[1]^[2] is a centered figurate number that represents a hexagon with a dot in the center and all other dots surrounding the center dot in a hexagonal lattice. The following figures illustrate this arrangement for the first four centered hexagonal numbers:

Formula

The $n$ th centered hexagonal number is given by the formula^[2]

H(n)=n^{3}-(n-1)^{3}=3n(n-1)+1=3n^{2}-3n+1.\,

Expressing the formula as

H(n)=1+6\left({\frac {n(n-1)}{2}}\right)

shows that the centered hexagonal number for $n$ is 1 more than 6 times the $(n - 1)$ th triangular number.

In the opposite direction, the index $n$ corresponding to the centered hexagonal number $H=H(n)$ can be calculated using the formula

n={\frac {3+{\sqrt {12H-3}}}{6}}.

This can be used as a test for whether a number $H$ is centered hexagonal: it will be if and only if the above expression is an integer.

Recurrence and generating function

The centered hexagonal numbers $H(n)$ satisfy the recurrence relation ^[2]

H(n+1)=H(n)+6n.

From this we can calculate the generating function $F(x)=\sum _{n\geq 0}H(x)x^{n}$ . The generating function satisfies

F(x)=x+xF(x)+\sum _{n\geq 2}6nx^{n}.

The latter term is the Taylor series of ${\frac {6x}{(1-x)^{2}}}-6x$ , so we get

(1-x)F(x)=x+{\frac {6x}{(1-x)^{2}}}-6x={\frac {x+4x^{2}+x^{3}}{(1-x)^{2}}}

and end up at

F(x)={\frac {x+4x^{2}+x^{3}}{(1-x)^{3}}}.

Properties

In base 10 one can notice that the hexagonal numbers' rightmost (least significant) digits follow the pattern 1–7–9–7–1 (repeating with period 5). This follows from the last digit of the triangle numbers (sequence A008954 in the OEIS ) which repeat 0-1-3-1-0 when taken modulo 5. In base 6 the rightmost digit is always 1: 1₆, 11₆, 31₆, 101₆, 141₆, 231₆, 331₆, 441₆... This follows from the fact that every centered hexagonal number modulo 6 (=10₆) equals 1.

The sum of the first $n$ centered hexagonal numbers is $n 3$ . That is, centered hexagonal pyramidal numbers and cubes are the same numbers, but they represent different shapes. Viewed from the opposite perspective, centered hexagonal numbers are differences of two consecutive cubes, so that the centered hexagonal numbers are the gnomon of the cubes. (This can be seen geometrically from the diagram.) In particular, prime centered hexagonal numbers are cuban primes.

The difference between $(2 n) 2$ and the $n$ th centered hexagonal number is a number of the form $3 n 2 + 3 n - 1$ , while the difference between $(2 n - 1) 2$ and the $n$ th centered hexagonal number is a pronic number.

Applications

Centered hexagonal numbers have practical applications in packing problems. They arise when packing round items into larger round containers, such as Vienna sausages into round cans, or combining individual wire strands into a cable.^{[ citation needed ]}

Many segmented mirror reflecting telescopes have primary mirrors comprising a centered hexagonal number of segments (neglecting the central segment removed to allow passage of light) to simplify the control system.^[3] Some examples:

Telescope	Number of segments	Number missing	Total	n-th centered hexagonal number
Giant Magellan Telescope	7	0	7	2
James Webb Space Telescope	18	1	19	3
Gran Telescopio Canarias	36	1	37	4
Guido Horn d'Arturo's prototype	61	0	61	5
Southern African Large Telescope	91	0	91	6

Related Research Articles

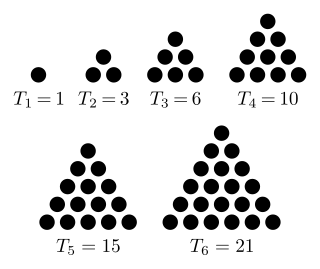
A triangular number or triangle number counts objects arranged in an equilateral triangle. Triangular numbers are a type of figurate number, other examples being square numbers and cube numbers. The $n$ th triangular number is the number of dots in the triangular arrangement with $n$ dots on each side, and is equal to the sum of the $n$ natural numbers from 1 to $n$ . The sequence of triangular numbers, starting with the 0th triangular number, is

In mathematics, a square number or perfect square is an integer that is the square of an integer; in other words, it is the product of some integer with itself. For example, 9 is a square number, since it equals $32$ and can be written as $3 \times 3$ .

A hexagonal number is a figurate number. The nth hexagonal number h_n is the number of distinct dots in a pattern of dots consisting of the outlines of regular hexagons with sides up to n dots, when the hexagons are overlaid so that they share one vertex.

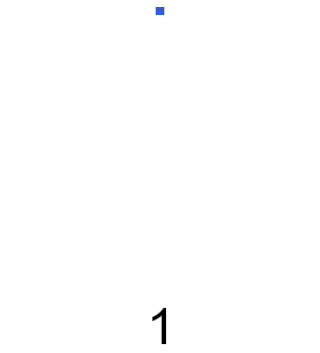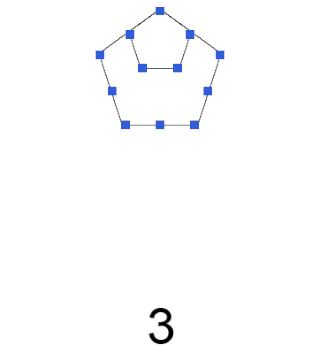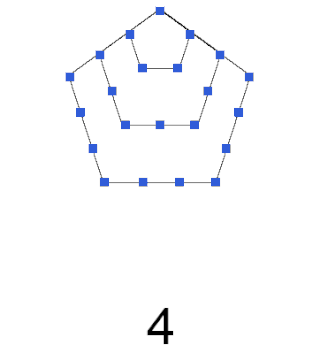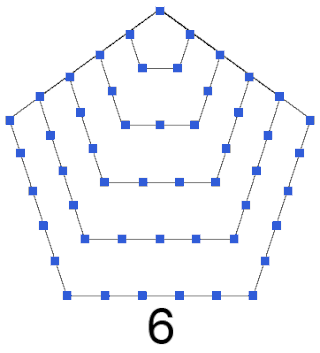
A pentagonal number is a figurate number that extends the concept of triangular and square numbers to the pentagon, but, unlike the first two, the patterns involved in the construction of pentagonal numbers are not rotationally symmetrical. The nth pentagonal number p_n is the number of distinct dots in a pattern of dots consisting of the outlines of regular pentagons with sides up to n dots, when the pentagons are overlaid so that they share one vertex. For instance, the third one is formed from outlines comprising 1, 5 and 10 dots, but the 1, and 3 of the 5, coincide with 3 of the 10 – leaving 12 distinct dots, 10 in the form of a pentagon, and 2 inside.

A heptagonal number is a figurate number that is constructed by combining heptagons with ascending size. The n-th heptagonal number is given by the formula

A pyramidal number is the number of points in a pyramid with a polygonal base and triangular sides. The term often refers to square pyramidal numbers, which have a square base with four sides, but it can also refer to a pyramid with any number of sides. The numbers of points in the base and in layers parallel to the base are given by polygonal numbers of the given number of sides, while the numbers of points in each triangular side is given by a triangular number. It is possible to extend the pyramidal numbers to higher dimensions.

A cuban prime is a prime number that is also a solution to one of two different specific equations involving differences between third powers of two integers x and y.

An octagonal number is a figurate number that gives the number of points in a certain octagonal arrangement. The octagonal number for n is given by the formula 3n² − 2n, with n > 0. The first few octagonal numbers are

A star number is a centered figurate number, a centered hexagram, such as the Star of David, or the board Chinese checkers is played on.

In elementary number theory, a centered square number is a centered figurate number that gives the number of dots in a square with a dot in the center and all other dots surrounding the center dot in successive square layers. That is, each centered square number equals the number of dots within a given city block distance of the center dot on a regular square lattice. While centered square numbers, like figurate numbers in general, have few if any direct practical applications, they are sometimes studied in recreational mathematics for their elegant geometric and arithmetic properties.

A centeredtriangular number is a centered figurate number that represents an equilateral triangle with a dot in the center and all its other dots surrounding the center in successive equilateral triangular layers.

The centered polygonal numbers are a class of series of figurate numbers, each formed by a central dot, surrounded by polygonal layers of dots with a constant number of sides. Each side of a polygonal layer contains one more dot than each side in the previous layer; so starting from the second polygonal layer, each layer of a centered k-gonal number contains k more dots than the previous layer.

A nonagonal number is a figurate number that extends the concept of triangular and square numbers to the nonagon. However, unlike the triangular and square numbers, the patterns involved in the construction of nonagonal numbers are not rotationally symmetrical. Specifically, the nth nonagonal number counts the number of dots in a pattern of n nested nonagons, all sharing a common corner, where the ith nonagon in the pattern has sides made of i dots spaced one unit apart from each other. The nonagonal number for n is given by the formula:

A centered cube number is a centered figurate number that counts the number of points in a three-dimensional pattern formed by a point surrounded by concentric cubical layers of points, with $i 2$ points on the square faces of the $i$ th layer. Equivalently, it is the number of points in a body-centered cubic pattern within a cube that has $n + 1$ points along each of its edges.

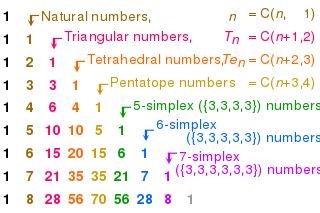
In number theory, a pentatope number is a number in the fifth cell of any row of Pascal's triangle starting with the 5-term row 1 4 6 4 1, either from left to right or from right to left. It is named because it represents the number of 3-dimensional unit spheres which can be packed into a pentatope of increasing side lengths.

A centered nonagonal number is a centered figurate number that represents a nonagon with a dot in the center and all other dots surrounding the center dot in successive nonagonal layers. The centered nonagonal number for n layers is given by the formula

A centered decagonal number is a centered figurate number that represents a decagon with a dot in the center and all other dots surrounding the center dot in successive decagonal layers. The centered decagonal number for n is given by the formula

288 is the natural number following 287 and preceding 289. Because 288 = 2 · 12 · 12, it may also be called "two gross" or "two dozen dozen".

A centered octahedral number or Haüy octahedral number is a figurate number that counts the number of points of a three-dimensional integer lattice that lie inside an octahedron centered at the origin. The same numbers are special cases of the Delannoy numbers, which count certain two-dimensional lattice paths. The Haüy octahedral numbers are named after René Just Haüy.

A dodecahedral number is a figurate number that represents a dodecahedron. The nth dodecahedral number is given by the formula

References

↑ Hindin, H. J. (1983). "Stars, hexes, triangular numbers and Pythagorean triples". J. Rec. Math. 16: 191–193.
1 2 3 Deza, Elena; Deza, M. (2012). Figurate Numbers. World Scientific. pp. 47–55. ISBN 978-981-4355-48-3.
↑ Mast, T S, and Nelson, J E. Figure control for a segmented telescope mirror. United States: N. p., 1979. Web. doi:10.2172/6194407.