The Möbius function is a multiplicative function in number theory introduced by the German mathematician August Ferdinand Möbius in 1832. It is ubiquitous in elementary and analytic number theory and most often appears as part of its namesake the Möbius inversion formula. Following work of Gian-Carlo Rota in the 1960s, generalizations of the Möbius function were introduced into combinatorics, and are similarly denoted .
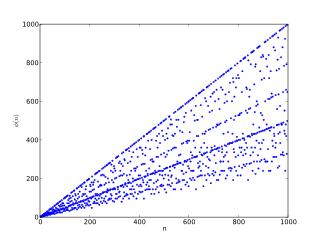
In number theory, Euler's totient function counts the positive integers up to a given integer n that are relatively prime to n. It is written using the Greek letter phi as or , and may also be called Euler's phi function. In other words, it is the number of integers k in the range 1 ≤ k ≤ n for which the greatest common divisor gcd(n, k) is equal to 1. The integers k of this form are sometimes referred to as totatives of n.
19 (nineteen) is the natural number following 18 and preceding 20. It is a prime number.
A highly composite number is a positive integer that has more divisors than all smaller positive integers. If d(n) denotes the number of divisors of a positive integer n, then a positive integer N is highly composite if d(N) > d(n) for all n < N. For example, 6 is highly composite because d(6)=4 and d(n)=1,2,2,3,2 for n=1,2,3,4,5 respectively.
In recreational mathematics, a repunit is a number like 11, 111, or 1111 that contains only the digit 1 — a more specific type of repdigit. The term stands for "repeated unit" and was coined in 1966 by Albert H. Beiler in his book Recreations in the Theory of Numbers.
In recreational mathematics, a repdigit or sometimes monodigit is a natural number composed of repeated instances of the same digit in a positional number system. The word is a portmanteau of "repeated" and "digit". Examples are 11, 666, 4444, and 999999. All repdigits are palindromic numbers and are multiples of repunits. Other well-known repdigits include the repunit primes and in particular the Mersenne primes.
The tables contain the prime factorization of the natural numbers from 1 to 1000.

In mathematics, a multiply perfect number is a generalization of a perfect number.

In number theory, a weird number is a natural number that is abundant but not semiperfect. In other words, the sum of the proper divisors of the number is greater than the number, but no subset of those divisors sums to the number itself.
In number theory, a k-hyperperfect number is a natural number n for which the equality holds, where σ(n) is the divisor function (i.e., the sum of all positive divisors of n). A hyperperfect number is a k-hyperperfect number for some integer k. Hyperperfect numbers generalize perfect numbers, which are 1-hyperperfect.
84 (eighty-four) is the natural number following 83 and preceding 85. It is seven dozens.
58 (fifty-eight) is the natural number following 57 and preceding 59.
In mathematics, a harshad number in a given number base is an integer that is divisible by the sum of its digits when written in that base. Harshad numbers in base n are also known as n-harshad numbers. Because being a Harshad number is determined based on the base the number is expressed in, a number can be a Harshad number many times over. So-called Trans-Harshad numbers are Harshad numbers in every base.
In number theory, a Wagstaff prime is a prime number of the form
In number theory, a self number or Devlali number in a given number base is a natural number that cannot be written as the sum of any other natural number and the individual digits of . 20 is a self number, because no such combination can be found. 21 is not, because it can be written as 15 + 1 + 5 using n = 15. These numbers were first described in 1949 by the Indian mathematician D. R. Kaprekar.
1728 is the natural number following 1727 and preceding 1729. It is a dozen gross, or one great gross. It is also the number of cubic inches in a cubic foot.
In number theory, an extravagant number (also known as a wasteful number) is a natural number in a given number base that has fewer digits than the number of digits in its prime factorization in the given number base (including exponents). For example, in base 10, 4 = 22, 6 = 2×3, 8 = 23, and 9 = 32 are extravagant numbers (sequence A046760 in the OEIS).
In number theory, an equidigital number is a natural number in a given number base that has the same number of digits as the number of digits in its prime factorization in the given number base, including exponents but excluding exponents equal to 1. For example, in base 10, 1, 2, 3, 5, 7, and 10 are equidigital numbers. All prime numbers are equidigital numbers in any base.
In number theory, a frugal number is a natural number in a given number base that has more digits than the number of digits in its prime factorization in the given number base (including exponents). For example, in base 10, 125 = 53, 128 = 27, 243 = 35, and 256 = 28 are frugal numbers (sequence A046759 in the OEIS). The first frugal number which is not a prime power is 1029 = 3 × 73. In base 2, thirty-two is a frugal number, since 32 = 25 is written in base 2 as 100000 = 10101.
In number theory, the Lagarias arithmetic derivative or number derivative is a function defined for integers, based on prime factorization, by analogy with the product rule for the derivative of a function that is used in mathematical analysis.











