
In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space, such as in a parametric curve. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it.

In the mathematical field of dynamical systems, an attractor is a set of states toward which a system tends to evolve, for a wide variety of starting conditions of the system. System values that get close enough to the attractor values remain close even if slightly disturbed.

In mathematics, an affine space is a geometric structure that generalizes some of the properties of Euclidean spaces in such a way that these are independent of the concepts of distance and measure of angles, keeping only the properties related to parallelism and ratio of lengths for parallel line segments. Affine space is the setting for affine geometry.
This article describes periodic points of some complex quadratic maps. A map is a formula for computing a value of a variable based on its own previous value or values; a quadratic map is one that involves the previous value raised to the powers one and two; and a complex map is one in which the variable and the parameters are complex numbers. A periodic point of a map is a value of the variable that occurs repeatedly after intervals of a fixed length.
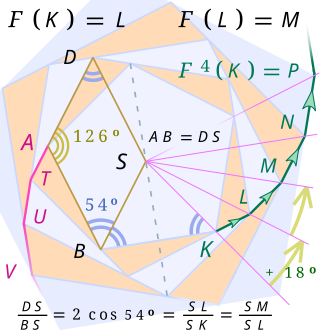
In mathematics, an iterated function is a function X → X which is obtained by composing another function f : X → X with itself a certain number of times. The process of repeatedly applying the same function is called iteration. In this process, starting from some initial object, the result of applying a given function is fed again in the function as input, and this process is repeated. For example on the image on the right:

The Rössler attractor is the attractor for the Rössler system, a system of three non-linear ordinary differential equations originally studied by Otto Rössler in the 1970s. These differential equations define a continuous-time dynamical system that exhibits chaotic dynamics associated with the fractal properties of the attractor. Rössler interpreted it as a formalization of a taffy-pulling machine.

A cobweb plot, or Verhulst diagram is a visual tool used in the dynamical systems field of mathematics to investigate the qualitative behaviour of one-dimensional iterated functions, such as the logistic map. Using a cobweb plot, it is possible to infer the long term status of an initial condition under repeated application of a map.

In mathematics, the tent map with parameter μ is the real-valued function fμ defined by

Bifurcation theory is the mathematical study of changes in the qualitative or topological structure of a given family of curves, such as the integral curves of a family of vector fields, and the solutions of a family of differential equations. Most commonly applied to the mathematical study of dynamical systems, a bifurcation occurs when a small smooth change made to the parameter values of a system causes a sudden 'qualitative' or topological change in its behavior. Bifurcations occur in both continuous systems and discrete systems.
In dynamical systems theory, a subset Λ of a smooth manifold M is said to have a hyperbolic structure with respect to a smooth map f if its tangent bundle may be split into two invariant subbundles, one of which is contracting and the other is expanding under f, with respect to some Riemannian metric on M. An analogous definition applies to the case of flows.

In the study of dynamical systems, a homoclinic orbit is a path through phase space which joins a saddle equilibrium point to itself. More precisely, a homoclinic orbit lies in the intersection of the stable manifold and the unstable manifold of an equilibrium. It is a heteroclinic orbit–a path between any two equilibrium points–in which the endpoints are one and the same.
In mathematics, in the study of iterated functions and dynamical systems, a periodic point of a function is a point which the system returns to after a certain number of function iterations or a certain amount of time.
In mathematics, Smale's axiom A defines a class of dynamical systems which have been extensively studied and whose dynamics is relatively well understood. A prominent example is the Smale horseshoe map. The term "axiom A" originates with Stephen Smale. The importance of such systems is demonstrated by the chaotic hypothesis, which states that, 'for all practical purposes', a many-body thermostatted system is approximated by an Anosov system.

In mathematics, Arnold's cat map is a chaotic map from the torus into itself, named after Vladimir Arnold, who demonstrated its effects in the 1960s using an image of a cat, hence the name.

In mathematics, the tricorn, sometimes called the Mandelbar set, is a fractal defined in a similar way to the Mandelbrot set, but using the mapping instead of used for the Mandelbrot set. It was introduced by W. D. Crowe, R. Hasson, P. J. Rippon, and P. E. D. Strain-Clark. John Milnor found tricorn-like sets as a prototypical configuration in the parameter space of real cubic polynomials, and in various other families of rational maps.

In mathematics, a Misiurewicz point is a parameter value in the Mandelbrot set and also in real quadratic maps of the interval for which the critical point is strictly pre-periodic. By analogy, the term Misiurewicz point is also used for parameters in a multibrot set where the unique critical point is strictly pre-periodic. This term makes less sense for maps in greater generality that have more than one free critical point because some critical points might be periodic and others not. These points are named after the Polish-American mathematician Michał Misiurewicz, who was the first to study them.
Numerical continuation is a method of computing approximate solutions of a system of parameterized nonlinear equations,
A complex quadratic polynomial is a quadratic polynomial whose coefficients and variable are complex numbers.
A Siegel disc or Siegel disk is a connected component in the Fatou set where the dynamics is analytically conjugate to an irrational rotation.
Fubini's nightmare is a seeming violation of Fubini's theorem, where a nice space, such as the square is foliated by smooth fibers, but there exists a set of positive measure whose intersection with each fiber is singular. There is no real contradiction to Fubini's theorem because despite smoothness of the fibers, the foliation is not absolutely continuous, and neither are the conditional measures on fibers.



























