Nina Snaith | |
|---|---|
 Snaith in 2009 | |
| Born | Nina Claire Snaith |
| Awards | Suffrage Science award (2018) Whitehead Prize (2008) |
| Scientific career | |
| Institutions | University of Bristol |
| Thesis | Random Matrix Theory and zeta functions (2000) |
| Doctoral advisor | Jonathan Keating [1] |
| Website | https://people.maths.bris.ac.uk/~mancs/ |
Nina Claire Snaith is a British mathematician at the University of Bristol working in random matrix theory and quantum chaos.
Snaith was educated at the University of Bristol where she received her PhD in 2000 [2] for research supervised by Jonathan Keating. [1]
In 1998, Snaith and her then adviser Jonathan Keating conjectured a value for the leading coefficient of the asymptotics of the moments of the Riemann zeta function. Keating and Snaith's guessed value for the constant was based on random-matrix theory, following a trend that started with Montgomery's pair correlation conjecture. Keating's and Snaith's work extended works [3] by Brian Conrey, Ghosh, and Gonek, also conjectural, based on number theoretic heuristics; Conrey, Farmer, Keating, Rubinstein, and Snaith later conjectured the lower terms in the asymptotics of the moments. [4] Snaith's work appeared in her doctoral thesis Random Matrix Theory and zeta functions. [1]
Snaith is currently Professor of Mathematical Physics at the University of Bristol. [5] [6]
In 2008, Snaith was awarded the London Mathematical Society's Whitehead Prize.
In 2014, she delivered the annual Hanna Neumann Lecture to honour the achievements of women in mathematics. [7]
Snaith is the daughter of mathematician Victor Snaith and sister of mathematician and musician Dan Snaith, mostly known by his artistic names Manitoba, Caribou, and Daphni. [8]
Atle Selberg was a Norwegian mathematician known for his work in analytic number theory and the theory of automorphic forms, and in particular for bringing them into relation with spectral theory. He was awarded the Fields Medal in 1950 and an honorary Abel Prize in 2002.
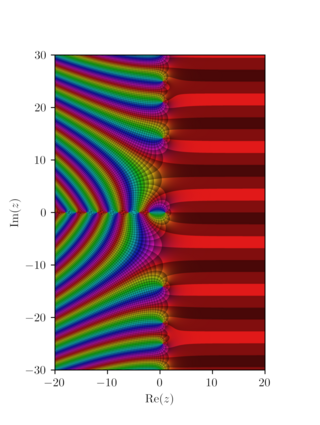
The Riemann zeta function or Euler–Riemann zeta function, denoted by the Greek letter ζ (zeta), is a mathematical function of a complex variable defined as
Lars Valerian Ahlfors was a Finnish mathematician, remembered for his work in the field of Riemann surfaces and his textbook on complex analysis.
Louis de Branges de Bourcia is a French-American mathematician. He was the Edward C. Elliott Distinguished Professor of Mathematics at Purdue University in West Lafayette, Indiana, retiring in 2023. He is best known for proving the long-standing Bieberbach conjecture in 1984, now called de Branges's theorem. He claims to have proved several important conjectures in mathematics, including the generalized Riemann hypothesis.
John Edensor Littlewood was a British mathematician. He worked on topics relating to analysis, number theory, and differential equations and had lengthy collaborations with G. H. Hardy, Srinivasa Ramanujan and Mary Cartwright.
In mathematics, an L-function is a meromorphic function on the complex plane, associated to one out of several categories of mathematical objects. An L-series is a Dirichlet series, usually convergent on a half-plane, that may give rise to an L-function via analytic continuation. The Riemann zeta function is an example of an L-function, and one important conjecture involving L-functions is the Riemann hypothesis and its generalization.
In number theory, Skewes's number is any of several large numbers used by the South African mathematician Stanley Skewes as upper bounds for the smallest natural number for which
In mathematics, analytic number theory is a branch of number theory that uses methods from mathematical analysis to solve problems about the integers. It is often said to have begun with Peter Gustav Lejeune Dirichlet's 1837 introduction of Dirichlet L-functions to give the first proof of Dirichlet's theorem on arithmetic progressions. It is well known for its results on prime numbers and additive number theory.
In mathematics, the Hilbert–Pólya conjecture states that the non-trivial zeros of the Riemann zeta function correspond to eigenvalues of a self-adjoint operator. It is a possible approach to the Riemann hypothesis, by means of spectral theory.
In mathematics, the Lindelöf hypothesis is a conjecture by Finnish mathematician Ernst Leonard Lindelöf about the rate of growth of the Riemann zeta function on the critical line. This hypothesis is implied by the Riemann hypothesis. It says that for any ε > 0,
In mathematics, the Selberg class is an axiomatic definition of a class of L-functions. The members of the class are Dirichlet series which obey four axioms that seem to capture the essential properties satisfied by most functions that are commonly called L-functions or zeta functions. Although the exact nature of the class is conjectural, the hope is that the definition of the class will lead to a classification of its contents and an elucidation of its properties, including insight into their relationship to automorphic forms and the Riemann hypothesis. The class was defined by Atle Selberg in, who preferred not to use the word "axiom" that later authors have employed.
In mathematics, Riemann–Hilbert problems, named after Bernhard Riemann and David Hilbert, are a class of problems that arise in the study of differential equations in the complex plane. Several existence theorems for Riemann–Hilbert problems have been produced by Mark Krein, Israel Gohberg and others.
John Brian Conrey is an American mathematician and the executive director of the American Institute of Mathematics. His research interests are in number theory, specifically analysis of L-functions and the Riemann zeta function.
In mathematics, the Riemann hypothesis is the conjecture that the Riemann zeta function has its zeros only at the negative even integers and complex numbers with real part 1/2. Many consider it to be the most important unsolved problem in pure mathematics. It is of great interest in number theory because it implies results about the distribution of prime numbers. It was proposed by Bernhard Riemann, after whom it is named.
In mathematics, Montgomery's pair correlation conjecture is a conjecture made by Hugh Montgomery that the pair correlation between pairs of zeros of the Riemann zeta function is
Kohji Matsumoto is a Japanese mathematician. He is professor of mathematics at Nagoya University in Nagoya, Japan.
Zeev Rudnick or Ze'ev Rudnick is a mathematician, specializing in number theory and in mathematical physics, notably quantum chaos. Rudnick is a professor at the School of Mathematical Sciences and the Cissie and Aaron Beare Chair in Number Theory at Tel Aviv University.
Jonathan Peter Keating is a British mathematician. As of September 2019, he is the Sedleian Professor of Natural Philosophy at the University of Oxford, and from 2012 to 2019 was the Henry Overton Wills Professor of Mathematics at the University of Bristol, where he served as Dean of the Faculty of Science (2009–2013). He has made contributions to applied mathematics and mathematical physics, in particular to quantum chaos, random matrix theory and number theory.
Samuel James Patterson is a Northern Irish mathematician specializing in analytic number theory. He has been a professor at the University of Göttingen since 1981.
Ashkan Nikeghbali Cisakht is a French mathematician and university professor of Iranian descent. He holds the chair of Financial Mathematics at the University of Zurich.
| International | |
|---|---|
| National | |
| Academics | |
| Other | |
