| Uniform octagonal prism | |
|---|---|
 | |
| Type | Prismatic uniform polyhedron |
| Elements | F = 10, E = 24, V = 16 (χ = 2) |
| Faces by sides | 8{4}+2{8} |
| Schläfli symbol | t{2,8} or {8}×{} |
| Wythoff symbol | 2 8 | 2 2 2 4 | |
| Coxeter diagrams |        |
| Symmetry | D8h, [8,2], (*822), order 32 |
| Rotation group | D8, [8,2]+, (822), order 16 |
| References | U 76(f) |
| Dual | Octagonal dipyramid |
| Properties | convex, zonohedron |
 Vertex figure 4.4.8 | |
In geometry, the octagonal prism is a prism comprising eight rectangular sides joining two regular octagon caps.
| Name | Ditetragonal prism | Ditetragonal trapezoprism |
|---|---|---|
| Image |  |  |
| Symmetry | D4h, [2,4], (*422) | D4d, [2+,8], (2*4) |
| Construction | tr{4,2} or t{4}×{}, | s2{2,8}, |
The octagonal prism can also be seen as a tiling on a sphere:
In optics, octagonal prisms are used to generate flicker-free images in movie projectors.
It is an element of three uniform honeycombs:
Truncated square prismatic honeycomb  | Omnitruncated cubic honeycomb  | Runcitruncated cubic honeycomb |
 |  |  |
It is also an element of two four-dimensional uniform 4-polytopes:
| Runcitruncated tesseract | Omnitruncated tesseract |
 |  |
| Family of uniform n-gonal prisms | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Prism name | Digonal prism | (Trigonal) Triangular prism | (Tetragonal) Square prism | Pentagonal prism | Hexagonal prism | Heptagonal prism | Octagonal prism | Enneagonal prism | Decagonal prism | Hendecagonal prism | Dodecagonal prism | ... | Apeirogonal prism |
| Polyhedron image |  |  |  |  |  |  |  |  |  |  |  | ... | |
| Spherical tiling image |  |  |  |  |  |  |  |  | Plane tiling image |  | |||
| Vertex config. | 2.4.4 | 3.4.4 | 4.4.4 | 5.4.4 | 6.4.4 | 7.4.4 | 8.4.4 | 9.4.4 | 10.4.4 | 11.4.4 | 12.4.4 | ... | ∞.4.4 |
| Coxeter diagram | | | |  |  |  | |  |  |  |  | ... | |
| *n42 symmetry mutation of omnitruncated tilings: 4.8.2n | ||||||||
|---|---|---|---|---|---|---|---|---|
| Symmetry *n42 [n,4] | Spherical | Euclidean | Compact hyperbolic | Paracomp. | ||||
| *242 [2,4] | *342 [3,4] | *442 [4,4] | *542 [5,4] | *642 [6,4] | *742 [7,4] | *842 [8,4]... | *∞42 [∞,4] | |
| Omnitruncated figure |  4.8.4 |  4.8.6 |  4.8.8 |  4.8.10 |  4.8.12 |  4.8.14 |  4.8.16 |  4.8.∞ |
| Omnitruncated duals |  V4.8.4 |  V4.8.6 |  V4.8.8 |  V4.8.10 |  V4.8.12 |  V4.8.14 |  V4.8.16 |  V4.8.∞ |
 | This polyhedron-related article is a stub. You can help Wikipedia by expanding it. |
In geometry, a cube is a three-dimensional solid object bounded by six square faces, facets, or sides, with three meeting at each vertex. Viewed from a corner, it is a hexagon and its net is usually depicted as a cross.
In geometry, a prism is a polyhedron comprising an n-sided polygon base, a second base which is a translated copy of the first, and n other faces, necessarily all parallelograms, joining corresponding sides of the two bases. All cross-sections parallel to the bases are translations of the bases. Prisms are named after their bases, e.g. a prism with a pentagonal base is called a pentagonal prism. Prisms are a subclass of prismatoids.
In geometry, the square orthobicupola is one of the Johnson solids. As the name suggests, it can be constructed by joining two square cupolae along their octagonal bases, matching like faces. A 45-degree rotation of one cupola before the joining yields a square gyrobicupola.
In geometry, a uniform 4-polytope is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.
In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an n-polytope and an m-polytope is an (n+m)-polytope, where n and m are dimensions of 2 (polygon) or higher.
In four-dimensional geometry, a runcinated tesseract is a convex uniform 4-polytope, being a runcination of the regular tesseract.
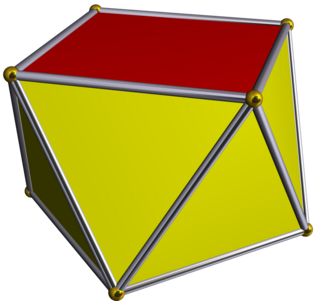
In geometry, the square antiprism is the second in an infinite family of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. It is also known as an anticube.
In four-dimensional geometry, a cantellated tesseract is a convex uniform 4-polytope, being a cantellation of the regular tesseract.
The cubic honeycomb or cubic cellulation is the only proper regular space-filling tessellation in Euclidean 3-space made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a regular octahedron. It is a self-dual tessellation with Schläfli symbol {4,3,4}. John Horton Conway called this honeycomb a cubille.
In hyperbolic geometry, the order-5 cubic honeycomb is one of four compact regular space-filling tessellations in hyperbolic 3-space. With Schläfli symbol {4,3,5}, it has five cubes {4,3} around each edge, and 20 cubes around each vertex. It is dual with the order-4 dodecahedral honeycomb.
In four-dimensional geometry, a runcinated 24-cell is a convex uniform 4-polytope, being a runcination of the regular 24-cell.
In 4-dimensional geometry, a uniform antiprismatic prism or antiduoprism is a uniform 4-polytope with two uniform antiprism cells in two parallel 3-space hyperplanes, connected by uniform prisms cells between pairs of faces. The symmetry of a p-gonal antiprismatic prism is [2p,2+,2], order 8p.
In geometry, a truncated cuboctahedral prism or great rhombicuboctahedral prism is a convex uniform polychoron.
In four-dimensional geometry, a prismatic uniform 4-polytope is a uniform 4-polytope with a nonconnected Coxeter diagram symmetry group. These figures are analogous to the set of prisms and antiprism uniform polyhedra, but add a third category called duoprisms, constructed as a product of two regular polygons.
A pseudo-uniform polyhedron is a polyhedron which has regular polygons as faces and has the same vertex configuration at all vertices but is not vertex-transitive: it is not true that for any two vertices, there exists a symmetry of the polyhedron mapping the first isometrically onto the second. Thus, although all the vertices of a pseudo-uniform polyhedron appear the same, it is not isogonal. They are called pseudo-uniform polyhedra due to their resemblance to some true uniform polyhedra.
In geometry, an octadecahedron is a polyhedron with 18 faces. No octadecahedron is regular; hence, the name does not commonly refer to one specific polyhedron.
In the geometry of hyperbolic 3-space, the square tiling honeycomb is one of 11 paracompact regular honeycombs. It is called paracompact because it has infinite cells, whose vertices exist on horospheres and converge to a single ideal point at infinity. Given by Schläfli symbol {4,4,3}, it has three square tilings, {4,4}, around each edge, and six square tilings around each vertex, in a cubic {4,3} vertex figure.
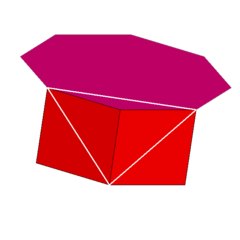
In geometry, the crossed square cupola is one of the nonconvex Johnson solid isomorphs, being topologically identical to the convex square cupola. It can be obtained as a slice of the nonconvex great rhombicuboctahedron or quasirhombicuboctahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; in this case the base polygon is an octagram.