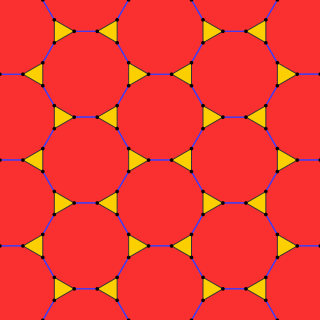
In geometry, the truncated hexagonal tiling is a semiregular tiling of the Euclidean plane. There are 2 dodecagons (12-sides) and one triangle on each vertex.

In geometry, the truncated trihexagonal tiling is one of eight semiregular tilings of the Euclidean plane. There are one square, one hexagon, and one dodecagon on each vertex. It has Schläfli symbol of tr{3,6}.

In geometry, the truncated square tiling is a semiregular tiling by regular polygons of the Euclidean plane with one square and two octagons on each vertex. This is the only edge-to-edge tiling by regular convex polygons which contains an octagon. It has Schläfli symbol of t{4,4}.

In geometry, the rhombitrihexagonal tiling is a semiregular tiling of the Euclidean plane. There are one triangle, two squares, and one hexagon on each vertex. It has Schläfli symbol of rr{3,6}.
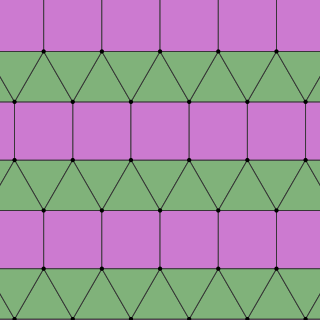
In geometry, the elongated triangular tiling is a semiregular tiling of the Euclidean plane. There are three triangles and two squares on each vertex. It is named as a triangular tiling elongated by rows of squares, and given Schläfli symbol {3,6}:e.

The cubic honeycomb or cubic cellulation is the only proper regular space-filling tessellation in Euclidean 3-space made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a regular octahedron. It is a self-dual tessellation with Schläfli symbol {4,3,4}. John Horton Conway called this honeycomb a cubille.

In geometry, the triheptagonal tiling is a semiregular tiling of the hyperbolic plane, representing a rectified Order-3 heptagonal tiling. There are two triangles and two heptagons alternating on each vertex. It has Schläfli symbol of r{7,3}.
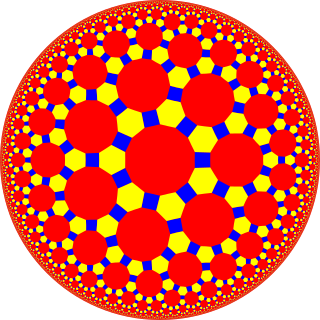
In geometry, the truncated triheptagonal tiling is a semiregular tiling of the hyperbolic plane. There is one square, one hexagon, and one tetradecagon (14-sides) on each vertex. It has Schläfli symbol of tr{7,3}.

In geometry, the truncated heptagonal tiling is a semiregular tiling of the hyperbolic plane. There are one triangle and two tetradecagons on each vertex. It has Schläfli symbol of t{7,3}. The tiling has a vertex configuration of 3.14.14.

In geometry, the rhombitriheptagonal tiling is a semiregular tiling of the hyperbolic plane. At each vertex of the tiling there is one triangle and one heptagon, alternating between two squares. The tiling has Schläfli symbol rr{7, 3}. It can be seen as constructed as a rectified triheptagonal tiling, r{7,3}, as well as an expanded heptagonal tiling or expanded order-7 triangular tiling.
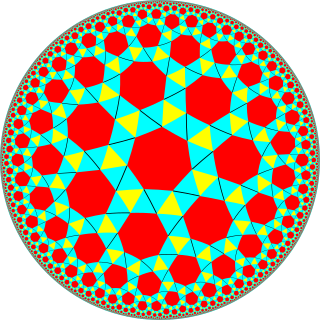
In geometry, the order-3 snub heptagonal tiling is a semiregular tiling of the hyperbolic plane. There are four triangles and one heptagon on each vertex. It has Schläfli symbol of sr{7,3}. The snub tetraheptagonal tiling is another related hyperbolic tiling with Schläfli symbol sr{7,4}.

In geometry, the order-7 truncated triangular tiling, sometimes called the hyperbolic soccerball, is a semiregular tiling of the hyperbolic plane. There are two hexagons and one heptagon on each vertex, forming a pattern similar to a conventional soccer ball with heptagons in place of pentagons. It has Schläfli symbol of t{3,7}.
In geometry, a uniform tiling is a tessellation of the plane by regular polygon faces with the restriction of being vertex-transitive.
In hyperbolic geometry, a uniform hyperbolic tiling is an edge-to-edge filling of the hyperbolic plane which has regular polygons as faces and is vertex-transitive. It follows that all vertices are congruent, and the tiling has a high degree of rotational and translational symmetry.

In geometry, the 3-7 kisrhombille tiling is a semiregular dual tiling of the hyperbolic plane. It is constructed by congruent right triangles with 4, 6, and 14 triangles meeting at each vertex.

In geometry, the truncated trioctagonal tiling is a semiregular tiling of the hyperbolic plane. There are one square, one hexagon, and one hexadecagon (16-sides) on each vertex. It has Schläfli symbol of tr{8,3}.
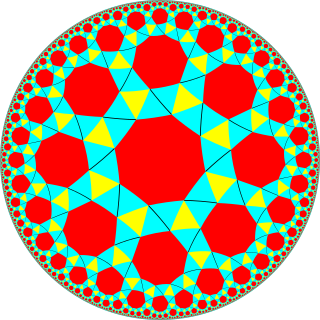
In geometry, the order-3 snub octagonal tiling is a semiregular tiling of the hyperbolic plane. There are four triangles, one octagon on each vertex. It has Schläfli symbol of sr{8,3}.

In geometry, the trioctagonal tiling is a semiregular tiling of the hyperbolic plane, representing a rectified Order-3 octagonal tiling. There are two triangles and two octagons alternating on each vertex. It has Schläfli symbol of r{8,3}.
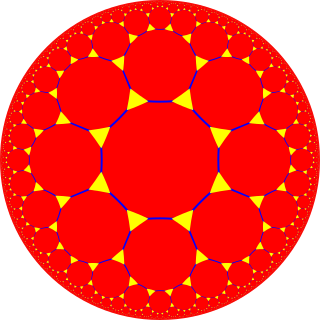
In geometry, the truncated octagonal tiling is a semiregular tiling of the hyperbolic plane. There is one triangle and two hexakaidecagons on each vertex. It has Schläfli symbol of t{8,3}.



















































