| hexaoctagonal tiling | |
|---|---|
 Poincaré disk model of the hyperbolic plane | |
| Type | Hyperbolic uniform tiling |
| Vertex configuration | (6.8)2 |
| Schläfli symbol | r{8,6} or |
| Wythoff symbol | 2 | 8 6 |
| Coxeter diagram |     |
| Symmetry group | [8,6], (*862) |
| Dual | Order-8-6 quasiregular rhombic tiling |
| Properties | Vertex-transitive edge-transitive |
In geometry, the hexaoctagonal tiling is a uniform tiling of the hyperbolic plane.
There are four uniform constructions of this tiling, three of them as constructed by mirror removal from the [8,6] kaleidoscope. Removing the mirror between the order 2 and 4 points, [8,6,1+], gives [(8,8,3)], (*883). Removing the mirror between the order 2 and 8 points, [1+,8,6], gives [(4,6,6)], (*664). Removing two mirrors as [8,1+,6,1+], leaves remaining mirrors (*4343).
| Uniform Coloring |  |  |  | |
|---|---|---|---|---|
| Symmetry | [8,6] (*862)    | [(8,3,8)] = [8,6,1+] (*883)   | [(6,4,6)] = [1+,8,6] (*664)   | [1+,8,6,1+] (*4343)  |
| Symbol | r{8,6} | r{(8,3,8)} | r{(6,4,6)} | |
| Coxeter diagram | |  =  | = | = |
The dual tiling has face configuration V6.8.6.8, and represents the fundamental domains of a quadrilateral kaleidoscope, orbifold (*4343), shown here. Adding a 2-fold gyration point at the center of each rhombi defines a (2*43) orbifold. These are subsymmetries of [8,6].
 [1+,8,4,1+], (*4343) |  [(8,4,2+)], (2*43) |
|---|
| Uniform octagonal/hexagonal tilings | ||||||
|---|---|---|---|---|---|---|
| Symmetry: [8,6], (*862) | ||||||
| | | | | | |
 |  |  |  |  |  |  |
| {8,6} | t{8,6} | r{8,6} | 2t{8,6}=t{6,8} | 2r{8,6}={6,8} | rr{8,6} | tr{8,6} |
| Uniform duals | ||||||
 | | | | | | |
 |  |  |  |  |  |  |
| V86 | V6.16.16 | V(6.8)2 | V8.12.12 | V68 | V4.6.4.8 | V4.12.16 |
| Alternations | ||||||
| [1+,8,6] (*466) | [8+,6] (8*3) | [8,1+,6] (*4232) | [8,6+] (6*4) | [8,6,1+] (*883) | [(8,6,2+)] (2*43) | [8,6]+ (862) |
 |  | | | | | |
 |  |  | ||||
| h{8,6} | s{8,6} | hr{8,6} | s{6,8} | h{6,8} | hrr{8,6} | sr{8,6} |
| Alternation duals | ||||||
 | | | | | | |
 | ||||||
| V(4.6)6 | V3.3.8.3.8.3 | V(3.4.4.4)2 | V3.4.3.4.3.6 | V(3.8)8 | V3.45 | V3.3.6.3.8 |
| Symmetry mutation of quasiregular tilings: 6.n.6.n | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry *6n2 [n,6] | Euclidean | Compact hyperbolic | Paracompact | Noncompact | |||||||
| *632 [3,6] | *642 [4,6] | *652 [5,6] | *662 [6,6] | *762 [7,6] | *862 [8,6]... | *∞62 [∞,6] | [iπ/λ,6] | ||||
| Quasiregular figures configuration |  6.3.6.3 |  6.4.6.4 |  6.5.6.5 |  6.6.6.6 |  6.7.6.7 |  6.8.6.8 |  6.∞.6.∞ | 6.∞.6.∞ | |||
| Dual figures | |||||||||||
| Rhombic figures configuration |  V6.3.6.3 |  V6.4.6.4 |  V6.5.6.5 |  V6.6.6.6 | V6.7.6.7 |  V6.8.6.8 |  V6.∞.6.∞ | ||||
| Dimensional family of quasiregular polyhedra and tilings: (8.n)2 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry *8n2 [n,8] | Hyperbolic... | Paracompact | Noncompact | ||||||||
| *832 [3,8] | *842 [4,8] | *852 [5,8] | *862 [6,8] | *872 [7,8] | *882 [8,8]... | *∞82 [∞,8] | [iπ/λ,8] | ||||
| Coxeter |  |  |  | |  | |  |  | |||
| Quasiregular figures configuration |  3.8.3.8 |  4.8.4.8 |  8.5.8.5 | 8.6.8.6 |  8.7.8.7 |  8.8.8.8 |  8.∞.8.∞ | 8.∞.8.∞ | |||
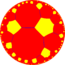
In geometry, the order-4 pentagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {5,4}. It can also be called a pentapentagonal tiling in a bicolored quasiregular form.
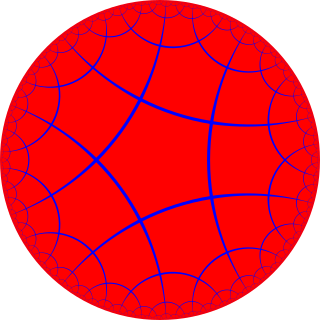
In geometry, the order-4 hexagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {6,4}.
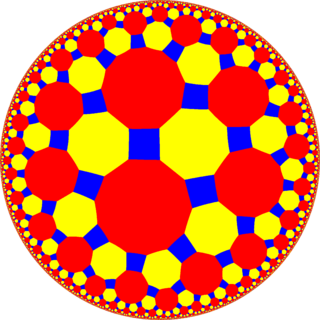
In geometry, the truncated tetrahexagonal tiling is a semiregular tiling of the hyperbolic plane. There are one square, one octagon, and one dodecagon on each vertex. It has Schläfli symbol of tr{6,4}.
In geometry, the tetrahexagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol r{6,4}.
In geometry, the rhombitetrahexagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of rr{6,4}. It can be seen as constructed as a rectified tetrahexagonal tiling, r{6,4}, as well as an expanded order-4 hexagonal tiling or expanded order-6 square tiling.
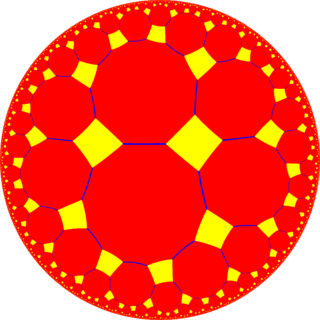
In geometry, the truncated order-4 hexagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t{6,4}. A secondary construction tr{6,6} is called a truncated hexahexagonal tiling with two colors of dodecagons.
In geometry, the truncated order-6 square tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t{4,6}.
In geometry, the truncated tetrapentagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t0,1,2{4,5} or tr{4,5}.
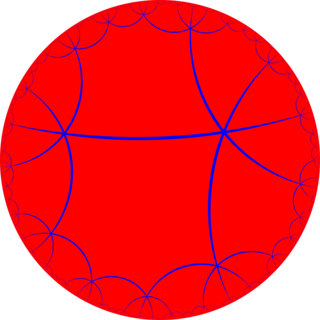
In geometry, the order-6 hexagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {6,6} and is self-dual.
In geometry, the order-4 heptagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {7,4}.
In geometry, the order-4 octagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {8,4}. Its checkerboard coloring can be called a octaoctagonal tiling, and Schläfli symbol of r{8,8}.
In geometry, the truncated order-4 octagonal tiling is a uniform tiling of the hyperbolic plane. It has Schläfli symbol of t0,1{8,4}. A secondary construction t0,1,2{8,8} is called a truncated octaoctagonal tiling with two colors of hexakaidecagons.
In geometry, the tetraoctagonal tiling is a uniform tiling of the hyperbolic plane.
In geometry, the order-8 square tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {4,8}.
In geometry, the order-8 octagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {8,8} and is self-dual.
In geometry, the order-6 pentagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {5,6}.
In geometry, the order-6 octagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {8,6}.
In geometry, the truncated hexaoctagonal tiling is a semiregular tiling of the hyperbolic plane. There are one square, one dodecagon, and one hexakaidecagon on each vertex. It has Schläfli symbol of tr{8,6}.
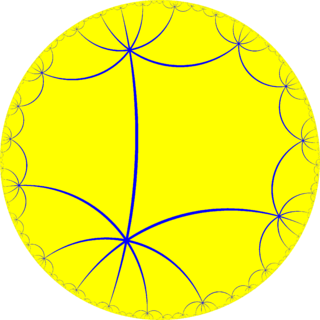
In geometry, the order-8 hexagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {6,8}.
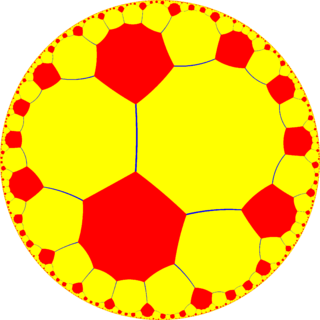
In geometry, the truncated order-8 hexagonal tiling is a semiregular tiling of the hyperbolic plane. It has Schläfli symbol of t{6,8}.
