| Ref. [1] indices | Symmetry | Architectonic tessellation | Catoptric tessellation |
|---|
Name
Coxeter diagram
Image | Vertex figure
Image | Cells | Name | Cell | Vertex figures |
|---|
J11,15
A1
W1
G22
δ4 | nc
[4,3,4]
       | Cubille
(Cubic honeycomb)
      
  | Octahedron,     
 |  | Cubille
      
 | 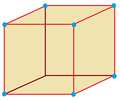
Cube,      | 
     |
|---|
J12,32
A15
W14
G7
t1δ4 | nc
[4,3,4]
       | Cuboctahedrille
(Rectified cubic honeycomb)
      
  | Cuboid,     
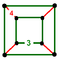 |   | Oblate octahedrille
      
 | 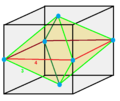
Isosceles square bipyramid
     |  
     , ,      |
|---|
J13
A14
W15
G8
t0,1δ4 | nc
[4,3,4]
       | Truncated cubille
(Truncated cubic honeycomb)
      
  | Isosceles square pyramid
 |   | Pyramidille
      
 | 
Isosceles square pyramid |  
     , ,      |
|---|
J14
A17
W12
G9
t0,2δ4 | nc
[4,3,4]
       | 2-RCO-trille
(Cantellated cubic honeycomb)
      
  | Wedge
 |    | Quarter oblate octahedrille
       | 
irr. Triangular bipyramid |   
     , ,      , ,      |
|---|
J16
A3
W2
G28
t1,2δ4 | bc
[[4,3,4]]
   | Truncated octahedrille
(Bitruncated cubic honeycomb)
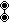  
  | Tetragonal disphenoid
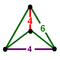 |  | Oblate tetrahedrille
      
 | 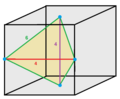
Tetragonal disphenoid | 
     |
|---|
J17
A18
W13
G25
t0,1,2δ4 | nc
[4,3,4]
       | n-tCO-trille
(Cantitruncated cubic honeycomb)
      
  | Mirrored sphenoid
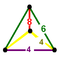 |    | Triangular pyramidille
       | 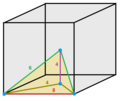
Mirrored sphenoid |   
     , ,      , ,      |
|---|
J18
A19
W19
G20
t0,1,3δ4 | nc
[4,3,4]
       | 1-RCO-trille
(Runcitruncated cubic honeycomb)
      
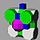  | Trapezoidal pyramid
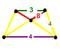 |     | Square quarter pyramidille
       | 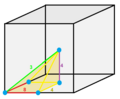
Irr. pyramid |    
     , ,      , ,      , ,      |
|---|
J19
A22
W18
G27
t0,1,2,3δ4 | bc
[[4,3,4]]
   | b-tCO-trille
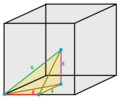
(Omnitruncated cubic honeycomb)
  | Phyllic disphenoid
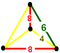 |   | Eighth pyramidille
       | 
Phyllic disphenoid |  
     , ,      |
|---|
J21,31,51
A2
W9
G1
hδ4 | fc
[4,31,1]
     | Tetroctahedrille
(Tetrahedral-octahedral honeycomb)
     or or       
  | Cuboctahedron,     
 |   | Dodecahedrille
     or or       
 | 
Rhombic dodecahedron,      |  
     , ,      |
|---|
J22,34
A21
W17
G10
h2δ4 | fc
[4,31,1]
     | truncated tetraoctahedrille
(Truncated tetrahedral-octahedral honeycomb)
     or or       
  | Rectangular pyramid
 |    | Half oblate octahedrille
     or or        | 
rhombic pyramid |   
     , ,      , ,      |
|---|
J23
A16
W11
G5
h3δ4 | fc
[4,31,1]
     | 3-RCO-trille
(Cantellated tetrahedral-octahedral honeycomb)
     or or       
  | Truncated triangular pyramid
 |    | Quarter cubille
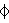       |  
irr. triangular bipyramid |    |
|---|
J24
A20
W16
G21
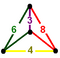
h2,3δ4 | fc
[4,31,1]
     | f-tCO-trille
(Cantitruncated tetrahedral-octahedral honeycomb)
     or or       
  | Mirrored sphenoid
 |    | Half pyramidille
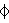       |  
Mirrored sphenoid |    |
|---|
J25,33
A13
W10
G6
qδ4 | d
[[3[4]]]
   | Truncated tetrahedrille
(Cyclotruncated tetrahedral-octahedral honeycomb)
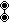   or or       
  | Isosceles triangular prism
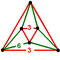 |   | Oblate cubille
         | 
Trigonal trapezohedron |   |
|---|
































