Bitruncated cubic honeycomb

Last updated November 06, 2024

Bitruncated cubic honeycomb

Type	Uniform honeycomb
Schläfli symbol	2t{4,3,4} t_1,2{4,3,4}
Coxeter-Dynkin diagram
Cell type	(4.6.6)
Face types	square {4} hexagon {6}
Edge figure	isosceles triangle {3}
Vertex figure	(tetragonal disphenoid)
Space group Fibrifold notation Coxeter notation	Im3m (229) 8^o:2 [[4,3,4]]
Coxeter group	${\tilde {C}}_{3}$ , [4,3,4]
Dual	Oblate tetrahedrille Disphenoid tetrahedral honeycomb Cell:
Properties	isogonal, isotoxal, isochoric

The bitruncated cubic honeycomb is a space-filling tessellation (or honeycomb) in Euclidean 3-space made up of truncated octahedra (or, equivalently, bitruncated cubes). It has 4 truncated octahedra around each vertex. Being composed entirely of truncated octahedra, it is cell-transitive. It is also edge-transitive, with 2 hexagons and one square on each edge, and vertex-transitive. It is one of 28 uniform honeycombs.

John Horton Conway calls this honeycomb a truncated octahedrille in his Architectonic and catoptric tessellation list, with its dual called an oblate tetrahedrille, also called a disphenoid tetrahedral honeycomb. Although a regular tetrahedron can not tessellate space alone, this dual has identical disphenoid tetrahedron cells with isosceles triangle faces.

Geometry

It can be realized as the Voronoi tessellation of the body-centred cubic lattice. Lord Kelvin conjectured that a variant of the bitruncated cubic honeycomb (with curved faces and edges, but the same combinatorial structure) was the optimal soap bubble foam. However, a number of less symmetrical structures have later been found to be more efficient foams of soap bubbles, among which the Weaire–Phelan structure appears to be the best.

The honeycomb represents the permutohedron tessellation for 3-space. The coordinates of the vertices for one octahedron represent a hyperplane of integers in 4-space, specifically permutations of (1,2,3,4). The tessellation is formed by translated copies within the hyperplane.

The tessellation is the highest tessellation of parallelohedrons in 3-space.

Projections

The bitruncated cubic honeycomb can be orthogonally projected into the euclidean plane with various symmetry arrangements. The highest (hexagonal) symmetry form projects into a nonuniform rhombitrihexagonal tiling. A square symmetry projection forms two overlapping truncated square tiling, which combine together as a chamfered square tiling.

Orthogonal projections
Symmetry	p6m (*632)	p4m (*442)	pmm (*2222)
Solid
Frame

Symmetry

The vertex figure for this honeycomb is a disphenoid tetrahedron, and it is also the Goursat tetrahedron (fundamental domain) for the ${\tilde {A}}_{3}$ Coxeter group. This honeycomb has four uniform constructions, with the truncated octahedral cells having different Coxeter groups and Wythoff constructions. These uniform symmetries can be represented by coloring differently the cells in each construction.

Five uniform colorings by cell
Space group	Im3m (229)	Pm3m (221)	Fm3m (225)	F43m (216)	Fd3m (227)
Fibrifold	8^o:2	4⁻:2	2⁻:2	1^o:2	2⁺:2
Coxeter group	${\tilde {C}}_{3}$ ×2 [[4,3,4]] =[4[3^[4]]] =	${\tilde {C}}_{3}$ [4,3,4] =[2[3^[4]]] =	${\tilde {B}}_{3}$ [4,3^1,1] =<[3^[4]]> =	${\tilde {A}}_{3}$ [3^[4]]	${\tilde {A}}_{3}$ ×2 [[3^[4]]] =[[3^[4]]]
Coxeter diagram
truncated octahedra	1	1:1 :	2:1:1 : :	1:1:1:1 : : :	1:1 :
Vertex figure
Vertex figure symmetry	[2⁺,4] (order 8)	[2] (order 4)	[ ] (order 2)	[ ]⁺ (order 1)	[2]⁺ (order 2)
Image Colored by cell

Related polyhedra and honeycombs

The regular skew apeirohedron {6,4|4} contains the hexagons of this honeycomb. Muoctahedron.png — The regular skew apeirohedron {6,4|4} contains the hexagons of this honeycomb.

The [4,3,4], , Coxeter group generates 15 permutations of uniform tessellations, 9 with distinct geometry including the alternated cubic honeycomb. The expanded cubic honeycomb (also known as the runcinated tesseractic honeycomb) is geometrically identical to the cubic honeycomb.

C3 honeycombs
Space group	Fibrifold	Extended symmetry	Extended diagram	Order	Honeycombs
Pm3m (221)	4⁻:2	[4,3,4]		×1	₁, ₂, ₃, ₄, ₅, ₆
Fm3m (225)	2⁻:2	[1⁺,4,3,4] ↔ [4,3^1,1]	↔	Half	₇, ₁₁, ₁₂, ₁₃
I43m (217)	4^o:2	[[(4,3,4,2⁺)]]		Half × 2	₍₇₎,
Fd3m (227)	2⁺:2	[[1⁺,4,3,4,1⁺]] ↔ [[3^[4]]]	↔	Quarter × 2	₁₀,
Im3m (229)	8^o:2	[[4,3,4]]		×2	₍₁₎, ₈, ₉

The [4,3^1,1], , Coxeter group generates 9 permutations of uniform tessellations, 4 with distinct geometry including the alternated cubic honeycomb.

B3 honeycombs
Space group	Fibrifold	Extended symmetry	Extended diagram	Order	Honeycombs
Fm3m (225)	2⁻:2	[4,3^1,1] ↔ [4,3,4,1⁺]	↔	×1	₁, ₂, ₃, ₄
Fm3m (225)	2⁻:2	<[1⁺,4,3^1,1]> ↔ <[3^[4]]>	↔	×2	₍₁₎, ₍₃₎
Pm3m (221)	4⁻:2	<[4,3^1,1]>		×2	₅, ₆, ₇, ₍₆₎, ₉, ₁₀, ₁₁

This honeycomb is one of five distinct uniform honeycombs ^[1] constructed by the ${\tilde {A}}_{3}$ Coxeter group. The symmetry can be multiplied by the symmetry of rings in the Coxeter–Dynkin diagrams:

A3 honeycombs
Space group	Fibrifold	Square symmetry	Extended symmetry	Extended diagram	Extended group	Honeycomb diagrams
F43m (216)	1^o:2	a1	[3^[4]]		${\tilde {A}}_{3}$	(None)
Fm3m (225)	2⁻:2	d2	<[3^[4]]> ↔ [4,3^1,1]	↔	${\tilde {A}}_{3}$ ×2₁ ↔ ${\tilde {B}}_{3}$	₁, ₂
Fd3m (227)	2⁺:2	g2	[[3^[4]]] or [2⁺[3^[4]]]	↔	${\tilde {A}}_{3}$ ×2₂	₃
Pm3m (221)	4⁻:2	d4	<2[3^[4]]> ↔ [4,3,4]	↔	${\tilde {A}}_{3}$ ×4₁ ↔ ${\tilde {C}}_{3}$	₄
I3 (204)	8^−o	r8	[4[3^[4]]]⁺ ↔ [[4,3⁺,4]]	↔	½ ${\tilde {A}}_{3}$ ×8 ↔ ½ ${\tilde {C}}_{3}$ ×2	_(*)
Im3m (229)	8^o:2	r8	[4[3^[4]]] ↔ [[4,3,4]]	↔	${\tilde {A}}_{3}$ ×8 ↔ ${\tilde {C}}_{3}$ ×2	₅

Alternated form

Alternated bitruncated cubic honeycomb
Type	Convex honeycomb
Schläfli symbol	2s{4,3,4} 2s{4,3^1,1} sr{3^[4]}
Coxeter diagrams	= = =
Cells	tetrahedron icosahedron
Vertex figure
Coxeter group	[[4,3⁺,4]], ${\tilde {C}}_{3}$
Dual	Ten-of-diamonds honeycomb Cell:
Properties	vertex-transitive

This honeycomb can be alternated, creating pyritohedral icosahedra from the truncated octahedra with disphenoid tetrahedral cells created in the gaps. There are three constructions from three related Coxeter-Dynkin diagrams: , , and . These have symmetry [4,3⁺,4], [4,(3^1,1)⁺] and [3^[4]]⁺ respectively. The first and last symmetry can be doubled as [[4,3⁺,4]] and [[3^[4]]]⁺.

The dual honeycomb is made of cells called ten-of-diamonds decahedra.

Five uniform colorings
Space group	I3 (204)	Pm3 (200)	Fm3 (202)	Fd3 (203)	F23 (196)
Fibrifold	8^−o	4⁻	2⁻	2^o+	1^o
Coxeter group	[[4,3⁺,4]]	[4,3⁺,4]	[4,(3^1,1)⁺]	[[3^[4]]]⁺	[3^[4]]⁺
Coxeter diagram
Order	double	full	half	quarter double	quarter
Image colored by cells

This honeycomb is represented in the boron atoms of the α-rhombohedral crystal. The centers of the icosahedra are located at the fcc positions of the lattice.^[2]

Related polytopes

Nonuniform variants with [4,3,4] symmetry and two types of truncated octahedra can be doubled by placing the two types of truncated octahedra to produce a nonuniform honeycomb with truncated octahedra and hexagonal prisms (as ditrigonal trapezoprisms). Its vertex figure is a C_2v-symmetric triangular bipyramid.

Continuously morphing a bitruncated cubic honeycomb from a cubic honeycomb, one obtains chamfered cubes, which are non-uniform and therefore consistute near-miss Johnson solids.

This honeycomb can then be alternated to produce another nonuniform honeycomb with pyritohedral icosahedra, octahedra (as triangular antiprisms), and tetrahedra (as sphenoids). Its vertex figure has C_2v symmetry and consists of 2 pentagons, 4 rectangles, 4 isosceles triangles (divided into two sets of 2), and 4 scalene triangles.

Notes

↑ , A000029 6-1 cases, skipping one with zero marks
↑ Williams, 1979, p 199, Figure 5-38.

Related Research Articles

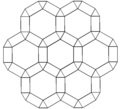
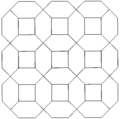
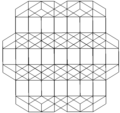
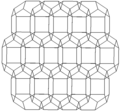
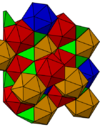
In geometry, a convex uniform honeycomb is a uniform tessellation which fills three-dimensional Euclidean space with non-overlapping convex uniform polyhedral cells.

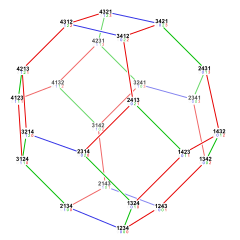
In four-dimensional geometry, a runcinated 5-cell is a convex uniform 4-polytope, being a runcination of the regular 5-cell.

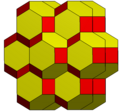
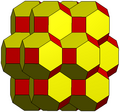
The cubic honeycomb or cubic cellulation is the only proper regular space-filling tessellation in Euclidean 3-space made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a regular octahedron. It is a self-dual tessellation with Schläfli symbol {4,3,4}. John Horton Conway called this honeycomb a cubille.

The tetrahedral-octahedral honeycomb, alternated cubic honeycomb is a quasiregular space-filling tessellation in Euclidean 3-space. It is composed of alternating regular octahedra and tetrahedra in a ratio of 1:2.

The quarter cubic honeycomb, quarter cubic cellulation or bitruncated alternated cubic honeycomb is a space-filling tessellation in Euclidean 3-space. It is composed of tetrahedra and truncated tetrahedra in a ratio of 1:1. It is called "quarter-cubic" because its symmetry unit – the minimal block from which the pattern is developed by reflections – is four times that of the cubic honeycomb.

The tetragonal disphenoid tetrahedral honeycomb is a space-filling tessellation in Euclidean 3-space made up of identical tetragonal disphenoidal cells. Cells are face-transitive with 4 identical isosceles triangle faces. John Horton Conway calls it an oblate tetrahedrille or shortened to obtetrahedrille.

In hyperbolic geometry, the order-5 cubic honeycomb is one of four compact regular space-filling tessellations in hyperbolic 3-space. With Schläfli symbol ${4,3,5},$ it has five cubes ${4,3}$ around each edge, and 20 cubes around each vertex. It is dual with the order-4 dodecahedral honeycomb.

In geometry, the icosahedral honeycomb is one of four compact, regular, space-filling tessellations in hyperbolic 3-space. With Schläfli symbol ${3,5,3},$ there are three icosahedra around each edge, and 12 icosahedra around each vertex, in a regular dodecahedral vertex figure.

In geometry, a truncated 24-cell is a uniform 4-polytope formed as the truncation of the regular 24-cell.

In geometry, a truncated 5-cell is a uniform 4-polytope formed as the truncation of the regular 5-cell.

In four-dimensional geometry, a runcinated 24-cell is a convex uniform 4-polytope, being a runcination of the regular 24-cell.

The triangular prismatic honeycomb or triangular prismatic cellulation is a space-filling tessellation in Euclidean 3-space. It is composed entirely of triangular prisms.

In four-dimensional Euclidean geometry, the 24-cell honeycomb, or icositetrachoric honeycomb is a regular space-filling tessellation of 4-dimensional Euclidean space by regular 24-cells. It can be represented by Schläfli symbol {3,4,3,3}.

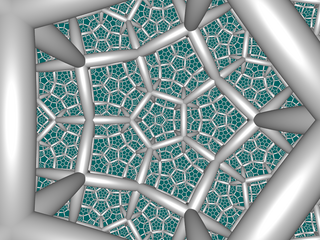
In hyperbolic geometry, a uniform honeycomb in hyperbolic space is a uniform tessellation of uniform polyhedral cells. In 3-dimensional hyperbolic space there are nine Coxeter group families of compact convex uniform honeycombs, generated as Wythoff constructions, and represented by permutations of rings of the Coxeter diagrams for each family.

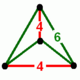
In four-dimensional Euclidean geometry, the 4-simplex honeycomb, 5-cell honeycomb or pentachoric-dispentachoric honeycomb is a space-filling tessellation honeycomb. It is composed of 5-cells and rectified 5-cells facets in a ratio of 1:1.

In four-dimensional Euclidean geometry, the snub 24-cell honeycomb, or snub icositetrachoric honeycomb is a uniform space-filling tessellation by snub 24-cells, 16-cells, and 5-cells. It was discovered by Thorold Gosset with his 1900 paper of semiregular polytopes. It is not semiregular by Gosset's definition of regular facets, but all of its cells (ridges) are regular, either tetrahedra or icosahedra.

In geometry, John Horton Conway defines architectonic and catoptric tessellations as the uniform tessellations of Euclidean 3-space with prime space groups and their duals, as three-dimensional analogue of the Platonic, Archimedean, and Catalan tiling of the plane. The singular vertex figure of an architectonic tessellation is the dual of the cell of the corresponding catoptric tessellation, and vice versa. The cubille is the only Platonic (regular) tessellation of 3-space, and is self-dual. There are other uniform honeycombs constructed as gyrations or prismatic stacks which are excluded from these categories.

In the field of hyperbolic geometry, the order-4 hexagonal tiling honeycomb arises as one of 11 regular paracompact honeycombs in 3-dimensional hyperbolic space. It is paracompact because it has cells composed of an infinite number of faces. Each cell is a hexagonal tiling whose vertices lie on a horosphere: a flat plane in hyperbolic space that approaches a single ideal point at infinity.

The triangular tiling honeycomb is one of 11 paracompact regular space-filling tessellations in hyperbolic 3-space. It is called paracompact because it has infinite cells and vertex figures, with all vertices as ideal points at infinity. It has Schläfli symbol {3,6,3}, being composed of triangular tiling cells. Each edge of the honeycomb is surrounded by three cells, and each vertex is ideal with infinitely many cells meeting there. Its vertex figure is a hexagonal tiling.

In the geometry of hyperbolic 3-space, the order-4 square tiling honeycomb is one of 11 paracompact regular honeycombs. It is paracompact because it has infinite cells and vertex figures, with all vertices as ideal points at infinity. Given by Schläfli symbol {4,4,4}, it has four square tilings around each edge, and infinite square tilings around each vertex in a square tiling vertex figure.

References

John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 21, Naming the Archimedean and Catalan polyhedra and tilings, Architectonic and Catoptric tessellations, p 292-298, includes all the nonprismatic forms)
George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs)
Branko Grünbaum, Uniform tilings of 3-space. Geombinatorics 4(1994), 49 - 56.
Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10] (1.9 Uniform space-fillings)
A. Andreini, Sulle reti di poliedri regolari e semiregolari e sulle corrispondenti reti correlative (On the regular and semiregular nets of polyhedra and on the corresponding correlative nets), Mem. Società Italiana della Scienze, Ser.3, 14 (1905) 75–129.
Klitzing, Richard. "3D Euclidean Honeycombs o4x3x4o - batch - O16".
Uniform Honeycombs in 3-Space: 05-Batch
Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X.

External links

Weisstein, Eric W. "Space-filling polyhedron". MathWorld .

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] , A000029 6-1 cases, skipping one with zero marks

[2] Williams, 1979, p 199, Figure 5-38.

[1]

[2]