Stars (M. C. Escher)

Last updated
Stars
Escher Stars.JPG
Artist M. C. Escher
Year1948
Type wood engraving
Dimensions32 cm× 26 cm(13 in× 10 in)

Stars is a wood engraving print created by the Dutch artist M. C. Escher in 1948, depicting two chameleons in a polyhedral cage floating through space.

Contents

The compound of three octahedra used for the central cage in Stars had been studied before in mathematics, and Escher likely learned of it from the book Vielecke und Vielflache by Max Brückner. Escher used similar compound polyhedral forms in several other works, including Crystal (1947), Study for Stars (1948), Double Planetoid (1949), and Waterfall (1961).

The design for Stars was likely influenced by Escher's own interest in both geometry and astronomy, by a long history of using geometric forms to model the heavens, and by a drawing style used by Leonardo da Vinci. Commentators have interpreted the cage's compound shape as a reference to double and triple stars in astronomy, or to twinned crystals in crystallography. The image contrasts the celestial order of its polyhedral shapes with the more chaotic forms of biology.

Prints of Stars belong to the permanent collections of major museums including the Rijksmuseum, the National Gallery of Art, and the National Gallery of Canada.

Description

Stars is a wood engraving print; that is, it was produced by carving the artwork into the end grain of a block of wood (unlike a woodcut which uses the side grain), and then using this block to print the image. It was created by Escher in October 1948. [1] [2] Although most published copies of Stars are monochromatic, with white artwork against a black background, the copy in the National Gallery of Canada is tinted in different shades of turquoise, yellow, green, and pale pink. [3]

The print depicts a hollowed-out compound of three octahedra, a polyhedral compound composed of three interlocking regular octahedra, floating in space. Numerous other polyhedra and polyhedral compounds float in the background; the four largest are, on the upper left, the compound of cube and octahedron; on the upper right, the stella octangula; on the lower left, a compound of two cubes; and on the lower right, a solid version of the same octahedron 3-compound. The smaller polyhedra visible within the print also include all of the five Platonic solids and the rhombic dodecahedron. [4] [5] In order to depict polyhedra accurately, Escher made models of them from cardboard. [2]

Two chameleons are contained within the cage-like shape of the central compound; Escher writes that they were chosen as its inhabitants "because they are able to cling by their legs and tails to the beams of their cage as it swirls through space". [6] The chameleon on the left sticks out his tongue, perhaps in commentary; H. S. M. Coxeter observes that the tongue has an unusual spiral-shaped tip. [5]

Influences

A rhombicuboctahedron drawn by Leonardo da Vinci, 1509, four centuries before Escher Leonardo polyhedra.png
A rhombicuboctahedron drawn by Leonardo da Vinci, 1509, four centuries before Escher

Escher's interest in geometry is well known, but he was also an avid amateur astronomer, and in the early 1940s he became a member of the Dutch Association for Meteorology and Astronomy. He owned a 6 cm refracting telescope, and recorded several observations of binary stars. [2]

The use of polyhedra to model heavenly bodies can be traced back to Plato, who in the Timaeus identified the regular dodecahedron with the shape of the heavens and its 12 faces with the constellations of the zodiac. [7] Later, Johannes Kepler theorized that the distribution of distances of the planets from the sun could be explained by the shapes of the five Platonic solids, nested within each other. Escher kept a model of this system of nested polyhedra, and regularly depicted polyhedra in his artworks relating to astronomy and other worlds. [2]

Escher learned his wood engraving technique from Samuel Jessurun de Mesquita. [6] He illustrated the octahedral compound of Stars in the beveled wire-frame style that had been used by Leonardo da Vinci in his illustrations for Luca Pacioli's 1509 book, De divina proportione . [4] [5] [8]

The stella octangula (Latin for "eight-pointed star") in the upper right of Stars was first described by Pacioli, and later rediscovered by Kepler, who gave it its astronomical name. [9] H. S. M. Coxeter reports that the shape of the central chameleon cage in Stars had previously been described in 1900 by Max Brückner, whose book Vielecke und Vielflache includes a photograph of a model of the same shape. Coxeter, believing that Escher was not aware of this reference, wrote "It is remarkable that Escher, without any knowledge of algebra or analytic geometry, was able to rediscover this highly symmetrical figure." [5] However, George W. Hart has documented that Escher was familiar with Brückner's book and based much of his knowledge of stellated polyhedra and polyhedral compounds on it. [10]

Analysis

Martin Beech interprets the many polyhedral compounds within Stars as corresponding to double stars and triple star systems in astronomy. [2] Beech writes that, for Escher, the mathematical orderliness of polyhedra depicts the "stability and timeless quality" of the heavens, and similarly Marianne L. Teuber writes that Stars "celebrates Escher's identification with Johannes Kepler's neo-Platonic belief in an underlying mathematical order in the universe". [11]

Alternatively, Howard W. Jaffe interprets the polyhedral forms in Stars crystallographically, as "brilliantly faceted jewels" floating through space, with its compound polyhedra representing crystal twinning. [12] However, R. A. Dunlap points out the contrast between the order of the polyhedral forms and the more chaotic biological nature of the chameleons inhabiting them. [13] In the same vein, Beech observes that the stars themselves convey tension between order and chaos: despite their symmetric shapes, the stars are scattered apparently at random, and vary haphazardly from each other. [2] As Escher himself wrote about the central chameleon cage, "I shouldn't be surprised if it wobbles a bit." [2]

A closely related woodcut, Study for Stars, completed in August 1948, [2] [14] depicts wireframe versions of several of the same polyhedra and polyhedral compounds, floating in black within a square composition, but without the chameleons. The largest polyhedron shown in Study for Stars, a stellated rhombic dodecahedron, is also one of two polyhedra depicted prominently in Escher's 1961 print Waterfall . [4]

The stella octangula, a compound of two tetrahedra that appears in the upper right of Stars, also forms the central shape of another of Escher's astronomical works, Double Planetoid (1949). [5] The compound of cube and octahedron in the upper left was used earlier by Escher, in Crystal (1947). [9]

Escher's later work Four Regular Solids (Stereometric Figure) returned to the theme of polyhedral compounds, depicting a more explicitly Keplerian form in which the compound of the cube and octahedron is nested within the compound of the dodecahedron and icosahedron. [13]

Collections and publications

Stars was used as cover art for the 1962 anthology Best Fantasy Stories edited by Brian Aldiss, [15] and for a 1971 Italian edition of occult guidebook The Morning of the Magicians . [16] It also formed the frontispiece for a 1996 textbook on crystallography. [12]

As well as being exhibited in the Escher Museum, copies of Stars are in the permanent collections of the Rijksmuseum, [17] National Gallery of Art, [18] Mildred Lane Kemper Art Museum, [19] Boston Public Library, [20] and the National Gallery of Canada. [3]

Related Research Articles

<span class="mw-page-title-main">Regular icosahedron</span> Convex polyhedron with 20 triangular faces

In geometry, the regular icosahedron is a convex polyhedron that can be constructed from pentagonal antiprism by attaching two pentagonal pyramids with regular faces to each of its pentagonal faces, or by putting points onto the cube. The resulting polyhedron has 20 equilateral triangles as its faces, 30 edges, and 12 vertices. It is an example of a Platonic solid and of a deltahedron. The icosahedral graph represents the skeleton of a regular icosahedron.

In geometry, an octahedron is a polyhedron with eight faces. An octahedron can be considered as a square bipyramid. When the edges of a square bipyramid are all equal in length, it produces a regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex. It is also an example of a deltahedron. An octahedron is the three-dimensional case of the more general concept of a cross polytope.

<span class="mw-page-title-main">Polyhedron</span> 3D shape with flat faces, straight edges and sharp corners

In geometry, a polyhedron is a three-dimensional figure with flat polygonal faces, straight edges and sharp corners or vertices.

In geometry, a polyhedral compound is a figure that is composed of several polyhedra sharing a common centre. They are the three-dimensional analogs of polygonal compounds such as the hexagram.

In geometry, a Platonic solid is a convex, regular polyhedron in three-dimensional Euclidean space. Being a regular polyhedron means that the faces are congruent regular polygons, and the same number of faces meet at each vertex. There are only five such polyhedra:

<span class="mw-page-title-main">Stellation</span> Extending the elements of a polytope to form a new figure

In geometry, stellation is the process of extending a polygon in two dimensions, a polyhedron in three dimensions, or, in general, a polytope in n dimensions to form a new figure. Starting with an original figure, the process extends specific elements such as its edges or face planes, usually in a symmetrical way, until they meet each other again to form the closed boundary of a new figure. The new figure is a stellation of the original. The word stellation comes from the Latin stellātus, "starred", which in turn comes from the Latin stella, "star". Stellation is the reciprocal or dual process to faceting.

A regular polyhedron is a polyhedron whose symmetry group acts transitively on its flags. A regular polyhedron is highly symmetrical, being all of edge-transitive, vertex-transitive and face-transitive. In classical contexts, many different equivalent definitions are used; a common one is that the faces are congruent regular polygons which are assembled in the same way around each vertex.

<span class="mw-page-title-main">Rhombic dodecahedron</span> Catalan solid with 12 faces

In geometry, the rhombic dodecahedron is a convex polyhedron with 12 congruent rhombic faces. It has 24 edges, and 14 vertices of 2 types. It is a Catalan solid, and the dual polyhedron of the cuboctahedron.

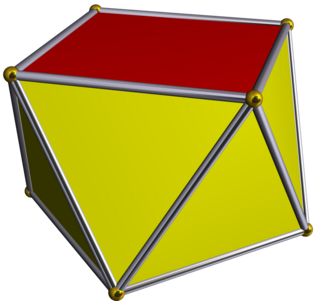
<span class="mw-page-title-main">Uniform polyhedron</span> Isogonal polyhedron with regular faces

In geometry, a uniform polyhedron has regular polygons as faces and is vertex-transitive—there is an isometry mapping any vertex onto any other. It follows that all vertices are congruent. Uniform polyhedra may be regular, quasi-regular, or semi-regular. The faces and vertices don't need to be convex, so many of the uniform polyhedra are also star polyhedra.

<span class="mw-page-title-main">Stellated octahedron</span> Polyhedral compound

The stellated octahedron is the only stellation of the octahedron. It is also called the stella octangula, a name given to it by Johannes Kepler in 1609, though it was known to earlier geometers. It was depicted in Pacioli's De Divina Proportione, 1509.

<span class="mw-page-title-main">Regular dodecahedron</span> Convex polyhedron with 12 regular pentagonal faces

A regular dodecahedron or pentagonal dodecahedron is a dodecahedron composed of regular pentagonal faces, three meeting at each vertex. It is an example of Platonic solids, described as cosmic stellation by Plato in his dialogues, and it was used as part of Solar System proposed by Johannes Kepler. However, the regular dodecahedron, including the other Platonic solids, has already been described by other philosophers since antiquity.

<span class="mw-page-title-main">Compound of five cubes</span> Polyhedral compound

The compound of five cubes is one of the five regular polyhedral compounds. It was first described by Edmund Hess in 1876.

<span class="mw-page-title-main">Faceting</span> Removing parts of a polytope without creating new vertices

In geometry, faceting is the process of removing parts of a polygon, polyhedron or polytope, without creating any new vertices.

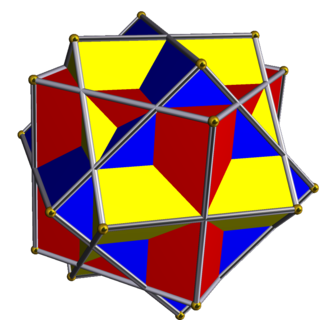
<span class="mw-page-title-main">Compound of three cubes</span> Polyhedral compound

In geometry, the compound of three cubes is a uniform polyhedron compound formed from three cubes arranged with octahedral symmetry. It has been depicted in works by Max Brückner and M.C. Escher.

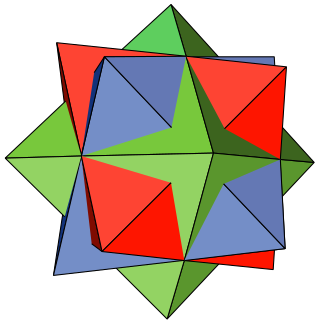
<span class="mw-page-title-main">Compound of three octahedra</span> Polyhedral compound

In mathematics, the compound of three octahedra or octahedron 3-compound is a polyhedral compound formed from three regular octahedra, all sharing a common center but rotated with respect to each other. Although appearing earlier in the mathematical literature, it was rediscovered and popularized by M. C. Escher, who used it in the central image of his 1948 woodcut Stars.

<span class="mw-page-title-main">First stellation of the rhombic dodecahedron</span> Self I intersecting polyhedron with 12 faces

In geometry, the first stellation of the rhombic dodecahedron is a self-intersecting polyhedron with 12 faces, each of which is a non-convex hexagon. It is a stellation of the rhombic dodecahedron and has the same outer shell and the same visual appearance as two other shapes: a solid, Escher's solid, with 48 triangular faces, and a polyhedral compound of three flattened octahedra with 24 overlapping triangular faces.

<span class="mw-page-title-main">Icosahedron</span> Polyhedron with 20 faces

In geometry, an icosahedron is a polyhedron with 20 faces. The name comes from Ancient Greek εἴκοσι (eíkosi) 'twenty' and ἕδρα (hédra) 'seat'. The plural can be either "icosahedra" or "icosahedrons".

<span class="mw-page-title-main">Max Brückner</span> German geometer (1860–1934)

Johannes Max Brückner was a German geometer, known for his collection of polyhedral models.

<i>Perspectiva corporum regularium</i> 1568 book on polyhedra by Wenzel Jamnitzer

Perspectiva corporum regularium is a book of perspective drawings of polyhedra by German Renaissance goldsmith Wenzel Jamnitzer, with engravings by Jost Amman, published in 1568.

<span class="mw-page-title-main">Double Planetoid</span> Escher print of a stellated octahedron shaped planetoid

Double Planetoid is a wood engraving print by the Dutch artist M. C. Escher, first printed in 1949.

References

  1. ↑ Locher, J. L. (2000), The Magic of M. C. Escher, Harry N. Abrams, Inc., p. 100, ISBN   0-8109-6720-0
  2. 1 2 3 4 5 6 7 8 Beech, Martin (1992), "Escher's Stars", Journal of the Royal Astronomical Society of Canada, 86: 169–177, Bibcode:1992JRASC..86..169B
  3. 1 2 Stars, National Gallery of Canada, archived from the original on 22 October 2013, retrieved 19 November 2011
  4. 1 2 3 Hart, George W. (1996), "The Polyhedra of M.C. Escher", Virtual Polyhedra, archived from the original on 2019-01-15, retrieved 2005-04-05
  5. 1 2 3 4 5 Coxeter, H. S. M. (1985), "A special book review: M. C. Escher: His life and complete graphic work", The Mathematical Intelligencer, 7 (1): 59–69, doi:10.1007/BF03023010, S2CID   189887063 Coxeter's analysis of Stars is on pp. 61–62.
  6. 1 2 Escher, M. C. (1992), M.C. Escher, the graphic work, Taschen, pp.  5, 14, ISBN   978-3-8228-5864-6
  7. ↑ Runia, David T. (1986), Philo of Alexandria and The "Timaeus" of Plato, Philosophia antiqua, vol. 44, Leiden: E. J. Brill, p. 295, ISBN   978-90-04-07477-4 .
  8. ↑ Calter, Paul (1998), "The Platonic Solids", Lecture Notes: Geometry in Art and Architecture, Dartmouth College, archived from the original on 2019-09-16, retrieved 2011-11-19
  9. 1 2 Barnes, John (2009), "Shapes and Solids", Gems of Geometry, Springer, pp. 25–56, doi:10.1007/978-3-642-05092-3_2, ISBN   978-3-642-05091-6
  10. ↑ Hart, George W., "Max Brücknerʼs Wunderkammer of Paper Polyhedra", Bridges 2019 Conference Proceedings (PDF), pp. 59–66, archived (PDF) from the original on 2022-01-21, retrieved 2021-02-22
  11. ↑ Teuber, M. L. (July 1974), "Sources of ambiguity in the prints of Maurits C. Escher", Scientific American, 231 (1): 90–104, Bibcode:1974SciAm.231a..90T, doi:10.1038/scientificamerican0774-90, PMID   4603121
  12. 1 2 Jaffe, Howard W. (1996), "About the frontispiece", Crystal Chemistry and Refractivity, Dover, p. vi, ISBN   978-0-486-69173-2
  13. 1 2 Dunlap, R. A. (1992), Hargittai, István (ed.), "Fivefold symmetry in the graphic art of M. C. Escher", Fivefold Symmetry (2nd ed.), World Scientific, pp. 489–504, ISBN   978-981-02-0600-0
  14. ↑ Locher (2000), p. 99.
  15. ↑ Clute, John; Grant, John (1999), The encyclopedia of fantasy (2nd ed.), Macmillan, p. 322, ISBN   978-0-312-19869-5
  16. ↑ Coulthart, John (October 17, 2015), "MC Escher book covers", feuilleton, archived from the original on 2015-12-08, retrieved 2015-12-05.
  17. ↑ Stars, Maurits Cornelis Escher, Rijksmuseum, 1948, hdl:10934/RM0001.COLLECT.494193
  18. ↑ "Stars", The Collection, National Gallery of Art, Rosenwald Collection 1980.45.493, archived from the original on 2015-12-22, retrieved 2015-12-13.
  19. ↑ Artwork detail, Kemper Museum, archived from the original on 6 April 2012, retrieved 19 November 2011
  20. ↑ Stars, Boston Public Library Print Department, archived from the original on 9 May 2018, retrieved 8 May 2018

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.