The triple-alpha process is a set of nuclear fusion reactions by which three helium-4 nuclei (alpha particles) are transformed into carbon. [1] [2]
The triple-alpha process is a set of nuclear fusion reactions by which three helium-4 nuclei (alpha particles) are transformed into carbon. [1] [2]
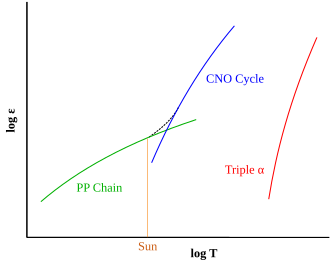
Helium accumulates in the cores of stars as a result of the proton–proton chain reaction and the carbon–nitrogen–oxygen cycle.
Nuclear fusion reaction of two helium-4 nuclei produces beryllium-8, which is highly unstable, and decays back into smaller nuclei with a half-life of 8.19×10−17 s, unless within that time a third alpha particle fuses with the beryllium-8 nucleus [3] to produce an excited resonance state of carbon-12, [4] called the Hoyle state, which nearly always decays back into three alpha particles, but once in about 2421.3 times releases energy and changes into the stable base form of carbon-12. [5] When a star runs out of hydrogen to fuse in its core, it begins to contract and heat up. If the central temperature rises to 108 K, [6] six times hotter than the Sun's core, alpha particles can fuse fast enough to get past the beryllium-8 barrier and produce significant amounts of stable carbon-12.
The net energy release of the process is 7.275 MeV.
As a side effect of the process, some carbon nuclei fuse with additional helium to produce a stable isotope of oxygen and energy:
Nuclear fusion reactions of helium with hydrogen produces lithium-5, which also is highly unstable, and decays back into smaller nuclei with a half-life of 3.7×10−22 s.
Fusing with additional helium nuclei can create heavier elements in a chain of stellar nucleosynthesis known as the alpha process, but these reactions are only significant at higher temperatures and pressures than in cores undergoing the triple-alpha process. This creates a situation in which stellar nucleosynthesis produces large amounts of carbon and oxygen, but only a small fraction of those elements are converted into neon and heavier elements. Oxygen and carbon are the main "ash" of helium-4 burning.
The triple-alpha process is ineffective at the pressures and temperatures early in the Big Bang. One consequence of this is that no significant amount of carbon was produced in the Big Bang.
Ordinarily, the probability of the triple-alpha process is extremely small. However, the beryllium-8 ground state has almost exactly the energy of two alpha particles. In the second step, 8Be + 4He has almost exactly the energy of an excited state of 12C. This resonance greatly increases the probability that an incoming alpha particle will combine with beryllium-8 to form carbon. The existence of this resonance was predicted by Fred Hoyle before its actual observation, based on the physical necessity for it to exist, in order for carbon to be formed in stars. The prediction and then discovery of this energy resonance and process gave very significant support to Hoyle's hypothesis of stellar nucleosynthesis, which posited that all chemical elements had originally been formed from hydrogen, the true primordial substance. The anthropic principle has been cited to explain the fact that nuclear resonances are sensitively arranged to create large amounts of carbon and oxygen in the universe. [7] [8]
With further increases of temperature and density, fusion processes produce nuclides only up to nickel-56 (which decays later to iron); heavier elements (those beyond Ni) are created mainly by neutron capture. The slow capture of neutrons, the s-process, produces about half of elements beyond iron. The other half are produced by rapid neutron capture, the r-process, which probably occurs in core-collapse supernovae and neutron star mergers. [9]
The triple-alpha steps are strongly dependent on the temperature and density of the stellar material. The power released by the reaction is approximately proportional to the temperature to the 40th power, and the density squared. [10] In contrast, the proton–proton chain reaction produces energy at a rate proportional to the fourth power of temperature, the CNO cycle at about the 17th power of the temperature, and both are linearly proportional to the density. This strong temperature dependence has consequences for the late stage of stellar evolution, the red-giant stage.
For lower mass stars on the red-giant branch, the helium accumulating in the core is prevented from further collapse only by electron degeneracy pressure. The entire degenerate core is at the same temperature and pressure, so when its density becomes high enough, fusion via the triple-alpha process rate starts throughout the core. The core is unable to expand in response to the increased energy production until the pressure is high enough to lift the degeneracy. As a consequence, the temperature increases, causing an increased reaction rate in a positive feedback cycle that becomes a runaway reaction. This process, known as the helium flash, lasts a matter of seconds but burns 60–80% of the helium in the core. During the core flash, the star's energy production can reach approximately 1011 solar luminosities which is comparable to the luminosity of a whole galaxy, [11] although no effects will be immediately observed at the surface, as the whole energy is used up to lift the core from the degenerate to normal, gaseous state. Since the core is no longer degenerate, hydrostatic equilibrium is once more established and the star begins to "burn" helium at its core and hydrogen in a spherical layer above the core. The star enters a steady helium-burning phase which lasts about 10% of the time it spent on the main sequence (the Sun is expected to burn helium at its core for about a billion years after the helium flash). [12]
In higher mass stars, which evolve along the asymptotic giant branch, carbon and oxygen accumulate in the core as helium is burned, while hydrogen burning shifts to further-out layers, resulting in an intermediate helium shell. However, the boundaries of these shells do not shift outward at the same rate due to differing critical temperatures and temperature sensitivities for hydrogen and helium burning. When the temperature at the inner boundary of the helium shell is no longer high enough to sustain helium burning, the core contracts and heats up, while the hydrogen shell (and thus the star's radius) expand outward. Core contraction and shell expansion continue until the core becomes hot enough to reignite the surrounding helium. This process continues cyclically – with a period on the order of 1000 years – and stars undergoing this process have periodically variable luminosity. These stars also lose material from their outer layers in a stellar wind driven by radiation pressure, which ultimately becomes a superwind as the star enters the planetary nebula phase. [13]
The triple-alpha process is highly dependent on carbon-12 and beryllium-8 having resonances with slightly more energy than helium-4. Based on known resonances, by 1952 it seemed impossible for ordinary stars to produce carbon as well as any heavier element. [14] Nuclear physicist William Alfred Fowler had noted the beryllium-8 resonance, and Edwin Salpeter had calculated the reaction rate for 8Be, 12C, and 16O nucleosynthesis taking this resonance into account. [15] [16] However, Salpeter calculated that red giants burned helium at temperatures of 2·108 K or higher, whereas other recent work hypothesized temperatures as low as 1.1·108 K for the core of a red giant.
Salpeter's paper mentioned in passing the effects that unknown resonances in carbon-12 would have on his calculations, but the author never followed up on them. It was instead astrophysicist Fred Hoyle who, in 1953, used the abundance of carbon-12 in the universe as evidence for the existence of a carbon-12 resonance. The only way Hoyle could find that would produce an abundance of both carbon and oxygen was through a triple-alpha process with a carbon-12 resonance near 7.68 MeV, which would also eliminate the discrepancy in Salpeter's calculations. [14]
Hoyle went to Fowler's lab at Caltech and said that there had to be a resonance of 7.68 MeV in the carbon-12 nucleus. (There had been reports of an excited state at about 7.5 MeV. [14] ) Fred Hoyle's audacity in doing this is remarkable, and initially, the nuclear physicists in the lab were skeptical. Finally, a junior physicist, Ward Whaling, fresh from Rice University, who was looking for a project decided to look for the resonance. Fowler permitted Whaling to use an old Van de Graaff generator that was not being used. Hoyle was back in Cambridge when Fowler's lab discovered a carbon-12 resonance near 7.65 MeV a few months later, validating his prediction. The nuclear physicists put Hoyle as first author on a paper delivered by Whaling at the summer meeting of the American Physical Society. A long and fruitful collaboration between Hoyle and Fowler soon followed, with Fowler even coming to Cambridge. [17]
The final reaction product lies in a 0+ state (spin 0 and positive parity). Since the Hoyle state was predicted to be either a 0+ or a 2+ state, electron–positron pairs or gamma rays were expected to be seen. However, when experiments were carried out, the gamma emission reaction channel was not observed, and this meant the state must be a 0+ state. This state completely suppresses single gamma emission, since single gamma emission must carry away at least 1 unit of angular momentum. Pair production from an excited 0+ state is possible because their combined spins (0) can couple to a reaction that has a change in angular momentum of 0. [18]
Carbon is a necessary component of all known life. 12C, a stable isotope of carbon, is abundantly produced in stars due to three factors:
Some scholars argue the 7.656 MeV Hoyle resonance, in particular, is unlikely to be the product of mere chance. Fred Hoyle argued in 1982 that the Hoyle resonance was evidence of a "superintellect"; [14] Leonard Susskind in The Cosmic Landscape rejects Hoyle's intelligent design argument. [22] Instead, some scientists believe that different universes, portions of a vast "multiverse", have different fundamental constants: [23] according to this controversial fine-tuning hypothesis, life can only evolve in the minority of universes where the fundamental constants happen to be fine-tuned to support the existence of life. Other scientists reject the hypothesis of the multiverse on account of the lack of independent evidence. [24]
The CNO cycle is one of the two known sets of fusion reactions by which stars convert hydrogen to helium, the other being the proton–proton chain reaction, which is more efficient at the Sun's core temperature. The CNO cycle is hypothesized to be dominant in stars that are more than 1.3 times as massive as the Sun.
The proton–proton chain, also commonly referred to as the p–p chain, is one of two known sets of nuclear fusion reactions by which stars convert hydrogen to helium. It dominates in stars with masses less than or equal to that of the Sun, whereas the CNO cycle, the other known reaction, is suggested by theoretical models to dominate in stars with masses greater than about 1.3 solar masses.
In physical cosmology, Big Bang nucleosynthesis is the production of nuclei other than those of the lightest isotope of hydrogen during the early phases of the universe. This type of nucleosynthesis is thought by most cosmologists to have occurred from 10 seconds to 20 minutes after the Big Bang. It is thought to be responsible for the formation of most of the universe's helium, along with small fractions of the hydrogen isotope deuterium, the helium isotope helium-3 (3He), and a very small fraction of the lithium isotope lithium-7 (7Li). In addition to these stable nuclei, two unstable or radioactive isotopes were produced: the heavy hydrogen isotope tritium and the beryllium isotope beryllium-7 (7Be). These unstable isotopes later decayed into 3He and 7Li, respectively, as above.
Nucleosynthesis is the process that creates new atomic nuclei from pre-existing nucleons and nuclei. According to current theories, the first nuclei were formed a few minutes after the Big Bang, through nuclear reactions in a process called Big Bang nucleosynthesis. After about 20 minutes, the universe had expanded and cooled to a point at which these high-energy collisions among nucleons ended, so only the fastest and simplest reactions occurred, leaving our universe containing hydrogen and helium. The rest is traces of other elements such as lithium and the hydrogen isotope deuterium. Nucleosynthesis in stars and their explosions later produced the variety of elements and isotopes that we have today, in a process called cosmic chemical evolution. The amounts of total mass in elements heavier than hydrogen and helium remains small, so that the universe still has approximately the same composition.
In astrophysics, stellar nucleosynthesis is the creation of chemical elements by nuclear fusion reactions within stars. Stellar nucleosynthesis has occurred since the original creation of hydrogen, helium and lithium during the Big Bang. As a predictive theory, it yields accurate estimates of the observed abundances of the elements. It explains why the observed abundances of elements change over time and why some elements and their isotopes are much more abundant than others. The theory was initially proposed by Fred Hoyle in 1946, who later refined it in 1954. Further advances were made, especially to nucleosynthesis by neutron capture of the elements heavier than iron, by Margaret and Geoffrey Burbidge, William Alfred Fowler and Fred Hoyle in their famous 1957 B2FH paper, which became one of the most heavily cited papers in astrophysics history.
A neutron source is any device that emits neutrons, irrespective of the mechanism used to produce the neutrons. Neutron sources are used in physics, engineering, medicine, nuclear weapons, petroleum exploration, biology, chemistry, and nuclear power. Neutron source variables include the energy of the neutrons emitted by the source, the rate of neutrons emitted by the source, the size of the source, the cost of owning and maintaining the source, and government regulations related to the source.
The carbon-burning process or carbon fusion is a set of nuclear fusion reactions that take place in the cores of massive stars (at least 8 at birth) that combines carbon into other elements. It requires high temperatures (> 5×108 K or 50 keV) and densities (> 3×109 kg/m3).
The oxygen-burning process is a set of nuclear fusion reactions that take place in massive stars that have used up the lighter elements in their cores. Oxygen-burning is preceded by the neon-burning process and succeeded by the silicon-burning process. As the neon-burning process ends, the core of the star contracts and heats until it reaches the ignition temperature for oxygen burning. Oxygen burning reactions are similar to those of carbon burning; however, they must occur at higher temperatures and densities due to the larger Coulomb barrier of oxygen.
In astrophysics, silicon burning is a very brief sequence of nuclear fusion reactions that occur in massive stars with a minimum of about 8–11 solar masses. Silicon burning is the final stage of fusion for massive stars that have run out of the fuels that power them for their long lives in the main sequence on the Hertzsprung–Russell diagram. It follows the previous stages of hydrogen, helium, carbon, neon and oxygen burning processes.
Neutron emission is a mode of radioactive decay in which one or more neutrons are ejected from a nucleus. It occurs in the most neutron-rich/proton-deficient nuclides, and also from excited states of other nuclides as in photoneutron emission and beta-delayed neutron emission. As only a neutron is lost by this process the number of protons remains unchanged, and an atom does not become an atom of a different element, but a different isotope of the same element.
Carbon-12 (12C) is the most abundant of the two stable isotopes of carbon, amounting to 98.93% of element carbon on Earth; its abundance is due to the triple-alpha process by which it is created in stars. Carbon-12 is of particular importance in its use as the standard from which atomic masses of all nuclides are measured, thus, its atomic mass is exactly 12 daltons by definition. Carbon-12 is composed of 6 protons, 6 neutrons, and 6 electrons.
Helium-4 is a stable isotope of the element helium. It is by far the more abundant of the two naturally occurring isotopes of helium, making up about 99.99986% of the helium on Earth. Its nucleus is identical to an alpha particle, and consists of two protons and two neutrons.
The neon-burning process is a set of nuclear fusion reactions that take place in evolved massive stars with at least 8 Solar masses. Neon burning requires high temperatures and densities (around 1.2×109 K or 100 keV and 4×109 kg/m3).
The Oddo–Harkins rule holds that an element with an even atomic number is more abundant than the elements with immediately adjacent atomic numbers. For example, carbon, with atomic number 6, is more abundant than boron (5) and nitrogen (7). Generally, the relative abundance of an even atomic numbered element is roughly two orders of magnitude greater than the relative abundances of the immediately adjacent odd atomic numbered elements to either side. This pattern was first reported by Giuseppe Oddo in 1914 and William Draper Harkins in 1917. The Oddo–Harkins rule is true for all elements beginning with carbon produced by stellar nucleosynthesis but not true for the lightest elements below carbon produced by big bang nucleosynthesis and cosmic ray spallation.
Supernova nucleosynthesis is the nucleosynthesis of chemical elements in supernova explosions.
Cosmic ray spallation, also known as the x-process, is a set of naturally occurring nuclear reactions causing nucleosynthesis; it refers to the formation of chemical elements from the impact of cosmic rays on an object. Cosmic rays are highly energetic charged particles from beyond Earth, ranging from protons, alpha particles, and nuclei of many heavier elements. About 1% of cosmic rays also consist of free electrons.
Helium (2He) has nine known isotopes, but only helium-3 (3He) and helium-4 (4He) are stable. All radioisotopes are short-lived; the longest-lived is 6He with half-life 806.92(24) milliseconds. The least stable is 10He, with half-life 260(40) yoctoseconds, though 2He may have an even shorter half-life.
Nuclear binding energy in experimental physics is the minimum energy that is required to disassemble the nucleus of an atom into its constituent protons and neutrons, known collectively as nucleons. The binding energy for stable nuclei is always a positive number, as the nucleus must gain energy for the nucleons to move apart from each other. Nucleons are attracted to each other by the strong nuclear force. In theoretical nuclear physics, the nuclear binding energy is considered a negative number. In this context it represents the energy of the nucleus relative to the energy of the constituent nucleons when they are infinitely far apart. Both the experimental and theoretical views are equivalent, with slightly different emphasis on what the binding energy means.
Nuclear astrophysics is an interdisciplinary part of both nuclear physics and astrophysics, involving close collaboration among researchers in various subfields of each of these fields. This includes, notably, nuclear reactions and their rates as they occur in cosmic environments, and modeling of astrophysical objects where these nuclear reactions may occur, but also considerations of cosmic evolution of isotopic and elemental composition (often called chemical evolution). Constraints from observations involve multiple messengers, all across the electromagnetic spectrum (nuclear gamma-rays, X-rays, optical, and radio/sub-mm astronomy), as well as isotopic measurements of solar-system materials such as meteorites and their stardust inclusions, cosmic rays, material deposits on Earth and Moon). Nuclear physics experiments address stability (i.e., lifetimes and masses) for atomic nuclei well beyond the regime of stable nuclides into the realm of radioactive/unstable nuclei, almost to the limits of bound nuclei (the drip lines), and under high density (up to neutron star matter) and high temperature (plasma temperatures up to 109 K). Theories and simulations are essential parts herein, as cosmic nuclear reaction environments cannot be realized, but at best partially approximated by experiments. In general terms, nuclear astrophysics aims to understand the origin of the chemical elements and isotopes, and the role of nuclear energy generation, in cosmic sources such as stars, supernovae, novae, and violent binary-star interactions.
Beryllium-8 is a radionuclide with 4 neutrons and 4 protons. It is an unbound resonance and nominally an isotope of beryllium. It decays into two alpha particles with a half-life on the order of 8.19×10−17 seconds. This has important ramifications in stellar nucleosynthesis as it creates a bottleneck in the creation of heavier chemical elements. The properties of 8Be have also led to speculation on the fine tuning of the Universe, and theoretical investigations on cosmological evolution had 8Be been stable.
