
In mathematics, the Lyapunov exponent or Lyapunov characteristic exponent of a dynamical system is a quantity that characterizes the rate of separation of infinitesimally close trajectories. Quantitatively, two trajectories in phase space with initial separation vector diverge at a rate given by

Quantum chaos is a branch of physics which studies how chaotic classical dynamical systems can be described in terms of quantum theory. The primary question that quantum chaos seeks to answer is: "What is the relationship between quantum mechanics and classical chaos?" The correspondence principle states that classical mechanics is the classical limit of quantum mechanics, specifically in the limit as the ratio of Planck's constant to the action of the system tends to zero. If this is true, then there must be quantum mechanisms underlying classical chaos. If quantum mechanics does not demonstrate an exponential sensitivity to initial conditions, how can exponential sensitivity to initial conditions arise in classical chaos, which must be the correspondence principle limit of quantum mechanics?

In the study of dynamical systems, the van der Pol oscillator is a non-conservative, oscillating system with non-linear damping. It evolves in time according to the second-order differential equation

Bifurcation theory is the mathematical study of changes in the qualitative or topological structure of a given family of curves, such as the integral curves of a family of vector fields, and the solutions of a family of differential equations. Most commonly applied to the mathematical study of dynamical systems, a bifurcation occurs when a small smooth change made to the parameter values of a system causes a sudden 'qualitative' or topological change in its behavior. Bifurcations occur in both continuous systems and discrete systems.

The ADM formalism is a Hamiltonian formulation of general relativity that plays an important role in canonical quantum gravity and numerical relativity. It was first published in 1959.
In dynamical systems theory, a period-doubling bifurcation occurs when a slight change in a system's parameters causes a new periodic trajectory to emerge from an existing periodic trajectory—the new one having double the period of the original. With the doubled period, it takes twice as long for the numerical values visited by the system to repeat themselves.

The Landau–Zener formula is an analytic solution to the equations of motion governing the transition dynamics of a two-state quantum system, with a time-dependent Hamiltonian varying such that the energy separation of the two states is a linear function of time. The formula, giving the probability of a diabatic transition between the two energy states, was published separately by Lev Landau, Clarence Zener, Ernst Stueckelberg, and Ettore Majorana, in 1932.
Vector field reconstruction is a method of creating a vector field from experimental or computer generated data, usually with the goal of finding a differential equation model of the system.
The Ghirardi–Rimini–Weber theory (GRW) is a spontaneous collapse theory in quantum mechanics, proposed in 1986 by Giancarlo Ghirardi, Alberto Rimini, and Tullio Weber.
Quantum dissipation is the branch of physics that studies the quantum analogues of the process of irreversible loss of energy observed at the classical level. Its main purpose is to derive the laws of classical dissipation from the framework of quantum mechanics. It shares many features with the subjects of quantum decoherence and quantum theory of measurement.

The light-front quantization of quantum field theories provides a useful alternative to ordinary equal-time quantization. In particular, it can lead to a relativistic description of bound systems in terms of quantum-mechanical wave functions. The quantization is based on the choice of light-front coordinates, where plays the role of time and the corresponding spatial coordinate is . Here, is the ordinary time, is one Cartesian coordinate, and is the speed of light. The other two Cartesian coordinates, and , are untouched and often called transverse or perpendicular, denoted by symbols of the type . The choice of the frame of reference where the time and -axis are defined can be left unspecified in an exactly soluble relativistic theory, but in practical calculations some choices may be more suitable than others.
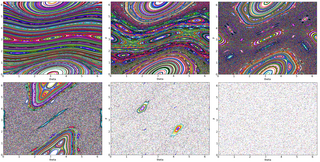
The kicked rotator, also spelled as kicked rotor, is a paradigmatic model for both Hamiltonian chaos and quantum chaos. It describes a free rotating stick in an inhomogeneous "gravitation like" field that is periodically switched on in short pulses. The model is described by the Hamiltonian
The term file dynamics is the motion of many particles in a narrow channel.

Oreste Piro is a dynamical systems theorist and biophysicist. He is at the Universitat de les Illes Balears (UIB) in Palma de Mallorca.
The Koopman–von Neumann (KvN) theory is a description of classical mechanics as an operatorial theory similar to quantum mechanics, based on a Hilbert space of complex, square-integrable wavefunctions. As its name suggests, the KvN theory is loosely related to work by Bernard Koopman and John von Neumann in 1931 and 1932, respectively. As explained in this entry, however, the historical origins of the theory and its name are complicated.

Quantum thermodynamics is the study of the relations between two independent physical theories: thermodynamics and quantum mechanics. The two independent theories address the physical phenomena of light and matter. In 1905, Albert Einstein argued that the requirement of consistency between thermodynamics and electromagnetism leads to the conclusion that light is quantized, obtaining the relation . This paper is the dawn of quantum theory. In a few decades quantum theory became established with an independent set of rules. Currently quantum thermodynamics addresses the emergence of thermodynamic laws from quantum mechanics. It differs from quantum statistical mechanics in the emphasis on dynamical processes out of equilibrium. In addition, there is a quest for the theory to be relevant for a single individual quantum system.
Dynamic scaling is a litmus test that shows whether an evolving system exhibits self-similarity. In general a function is said to exhibit dynamic scaling if it satisfies:
The Dicke model is a fundamental model of quantum optics, which describes the interaction between light and matter. In the Dicke model, the light component is described as a single quantum mode, while the matter is described as a set of two-level systems. When the coupling between the light and matter crosses a critical value, the Dicke model shows a mean-field phase transition to a superradiant phase. This transition belongs to the Ising universality class and was realized in cavity quantum electrodynamics experiments. Although the superradiant transition bears some analogy with the lasing instability, these two transitions belong to different universality classes.
Julyan Cartwright is an interdisciplinary physicist working in Granada, Spain at the Andalusian Earth Sciences Institute of the CSIC and affiliated with the Carlos I Institute of Theoretical and Computational Physics at the University of Granada.
Quantum computational chemistry is an emerging field that exploits quantum computing to simulate chemical systems. Despite quantum mechanics' foundational role in understanding chemical behaviors, traditional computational approaches face significant challenges, largely due to the complexity and computational intensity of quantum mechanical equations. This complexity arises from the exponential growth of a quantum system's wave function with each added particle, making exact simulations on classical computers inefficient.












