Related Research Articles
Amicable numbers are two different numbers so related that the sum of the proper divisors of each is equal to the other number. A pair of amicable numbers constitutes an aliquot sequence of period 2. A related concept is that of a perfect number, which is a number that equals the sum of its own proper divisors, in other words a number which forms an aliquot sequence of period 1. Numbers that are members of an aliquot sequence with period greater than 2 are known as sociable numbers.

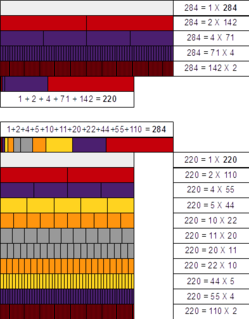
In number theory, a perfect number is a positive integer that is equal to the sum of its positive divisors, excluding the number itself. For instance, 6 has divisors 1, 2 and 3, and 1 + 2 + 3 = 6, so 6 is a perfect number.
The tables contain the prime factorization of the natural numbers from 1 to 1000.
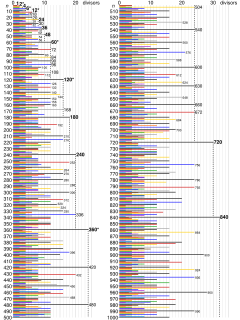
The tables below list all of the divisors of the numbers 1 to 1000.
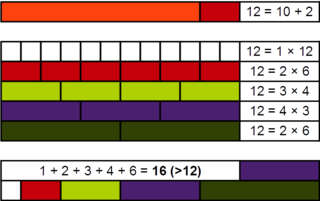
In number theory, an abundant number or excessive number is a number for which the sum of its proper divisors is greater than the number itself. The integer 12 is the first abundant number. Its proper divisors are 1, 2, 3, 4 and 6 for a total of 16. The amount by which the sum exceeds the number is the abundance. The number 12 has an abundance of 4, for example.

In number theory, a deficient number or defective number is a number n for which the sum of divisors σ(n)<2n, or, equivalently, the sum of proper divisors s(n)<n. The value 2n − σ(n) is called the number's deficiency.

In number theory, a weird number is a natural number that is abundant but not semiperfect.

In mathematics, an almost perfect number (sometimes also called slightly defective or least deficientnumber) is a natural number n such that the sum of all divisors of n (the sum-of-divisors function σ(n)) is equal to 2n − 1, the sum of all proper divisors of n, s(n) = σ(n) − n, then being equal to n − 1. The only known almost perfect numbers are powers of 2 with non-negative exponents (sequence A000079 in the OEIS). Therefore the only known odd almost perfect number is 20 = 1, and the only known even almost perfect numbers are those of the form 2k for some positive number k; however, it has not been shown that all almost perfect numbers are of this form. It is known that an odd almost perfect number greater than 1 would have at least six prime factors.
70 (seventy) is the natural number following 69 and preceding 71.
300 is the natural number following 299 and preceding 301.
500 is the natural number following 499 and preceding 501.
In mathematics, an aliquot sequence is a sequence of positive integers in which each term is the sum of the proper divisors of the previous term. If the sequence reaches the number 1, it ends, since the sum of the proper divisors of 1 is 0.
260 is the natural number following 259 and preceding 261.
An untouchable number is a positive integer that cannot be expressed as the sum of all the proper divisors of any positive integer. That is, these numbers are not in the image of the aliquot sum function. Their study goes back at least to Abu Mansur al-Baghdadi, who observed that both 2 and 5 are untouchable.
124 is the natural number following 123 and preceding 125.
248 is the natural number following 247 and preceding 249.
276 is the natural number following 275 and preceding 277.
288 is a pentagonal pyramidal number, is 4 superfactorial since 288 = 1!·2!·3!·4!
In number theory, the aliquot sums(n) of a positive integer n is the sum of all proper divisors of n, that is, all divisors of n other than n itself. It can be used to characterize the prime numbers, perfect numbers, deficient numbers, abundant numbers, and untouchable numbers, and to define the aliquot sequence of a number.

In mathematics a primitive abundant number is an abundant number whose proper divisors are all deficient numbers.
References
- ↑ "Sloane's A006037 : Weird numbers". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2016-06-02.
| This number article is a stub. You can help Wikipedia by expanding it. |