In mathematics, a measure on a real vector space is said to be transverse to a given set if it assigns measure zero to every translate of that set, while assigning finite and positive (i.e. non-zero) measure to some compact set.
Let V be a real vector space together with a metric space structure with respect to which it is a complete space. A Borel measure μ is said to be transverse to a Borel-measurable subset S of V if
The first requirement ensures that, for example, the trivial measure is not considered to be a transverse measure.
As an example, take V to be the Euclidean plane R2 with its usual Euclidean norm/metric structure. Define a measure μ on R2 by setting μ(E) to be the one-dimensional Lebesgue measure of the intersection of E with the first coordinate axis:
An example of a compact set K with positive and finite μ-measure is K = B1(0), the closed unit ball about the origin, which has μ(K) = 2. Now take the set S to be the second coordinate axis. Any translate (v1, v2) + S of S will meet the first coordinate axis in precisely one point, (v1, 0). Since a single point has Lebesgue measure zero, μ((v1, v2) + S) = 0, and so μ is transverse to S.
In mathematics, specifically in measure theory, a Borel measure on a topological space is a measure that is defined on all open sets. Some authors require additional restrictions on the measure, as described below.
In measure theory, a branch of mathematics, the Lebesgue measure, named after French mathematician Henri Lebesgue, is the standard way of assigning a measure to subsets of n-dimensional Euclidean space. For n = 1, 2, or 3, it coincides with the standard measure of length, area, or volume. In general, it is also called n-dimensional volume, n-volume, or simply volume. It is used throughout real analysis, in particular to define Lebesgue integration. Sets that can be assigned a Lebesgue measure are called Lebesgue-measurable; the measure of the Lebesgue-measurable set A is here denoted by λ(A).
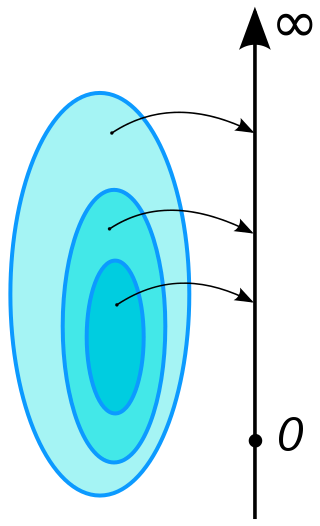
In mathematics, the concept of a measure is a generalization and formalization of geometrical measures and other common notions, such as mass and probability of events. These seemingly distinct concepts have many similarities and can often be treated together in a single mathematical context. Measures are foundational in probability theory, integration theory, and can be generalized to assume negative values, as with electrical charge. Far-reaching generalizations of measure are widely used in quantum physics and physics in general.
In mathematical analysis, a null set is a measurable set that has measure zero. This can be characterized as a set that can be covered by a countable union of intervals of arbitrarily small total length.
In mathematical analysis, the Haar measure assigns an "invariant volume" to subsets of locally compact topological groups, consequently defining an integral for functions on those groups.
In mathematics, a complete measure (or, more precisely, a complete measure space) is a measure space in which every subset of every null set is measurable (having measure zero). More formally, a measure space (X, Σ, μ) is complete if and only if
In mathematics, the Lp spaces are function spaces defined using a natural generalization of the p-norm for finite-dimensional vector spaces. They are sometimes called Lebesgue spaces, named after Henri Lebesgue, although according to the Bourbaki group they were first introduced by Frigyes Riesz.
In real analysis the Heine–Borel theorem, named after Eduard Heine and Émile Borel, states:
In calculus, absolute continuity is a smoothness property of functions that is stronger than continuity and uniform continuity. The notion of absolute continuity allows one to obtain generalizations of the relationship between the two central operations of calculus—differentiation and integration. This relationship is commonly characterized in the framework of Riemann integration, but with absolute continuity it may be formulated in terms of Lebesgue integration. For real-valued functions on the real line, two interrelated notions appear: absolute continuity of functions and absolute continuity of measures. These two notions are generalized in different directions. The usual derivative of a function is related to the Radon–Nikodym derivative, or density, of a measure. We have the following chains of inclusions for functions over a compact subset of the real line:
In mathematics, Pontryagin duality is a duality between locally compact abelian groups that allows generalizing Fourier transform to all such groups, which include the circle group, the finite abelian groups, and the additive group of the integers, the real numbers, and every finite dimensional vector space over the reals or a p-adic field.
In mathematics, a Radon measure, named after Johann Radon, is a measure on the σ-algebra of Borel sets of a Hausdorff topological space X that is finite on all compact sets, outer regular on all Borel sets, and inner regular on open sets. These conditions guarantee that the measure is "compatible" with the topology of the space, and most measures used in mathematical analysis and in number theory are indeed Radon measures.
In mathematics, a regular measure on a topological space is a measure for which every measurable set can be approximated from above by open measurable sets and from below by compact measurable sets.
In mathematics, Gaussian measure is a Borel measure on finite-dimensional Euclidean space Rn, closely related to the normal distribution in statistics. There is also a generalization to infinite-dimensional spaces. Gaussian measures are named after the German mathematician Carl Friedrich Gauss. One reason why Gaussian measures are so ubiquitous in probability theory is the central limit theorem. Loosely speaking, it states that if a random variable X is obtained by summing a large number N of independent random variables of order 1, then X is of order and its law is approximately Gaussian.
In mathematics, the Cameron–Martin theorem or Cameron–Martin formula is a theorem of measure theory that describes how abstract Wiener measure changes under translation by certain elements of the Cameron–Martin Hilbert space.
In mathematics, there is a folklore claim that there is no analogue of Lebesgue measure on an infinite-dimensional Banach space. The theorem this refers to states that there is no translationally invariant measure on a separable Banach space - because if any ball has nonzero non-infinite volume, a slightly smaller ball has zero volume, and countable many such smaller balls cover the space. The folklore statement, however, is entirely false. The countable product of Lebesgue measure is translationally invariant and gives the intuitive notion of volume as the infinite product of lengths, only the domain on which this product measure is defined must necessarily be non-separable, and the measure itself is not sigma finite.
In mathematics, an inner regular measure is one for which the measure of a set can be approximated from within by compact subsets.
In mathematics, the disintegration theorem is a result in measure theory and probability theory. It rigorously defines the idea of a non-trivial "restriction" of a measure to a measure zero subset of the measure space in question. It is related to the existence of conditional probability measures. In a sense, "disintegration" is the opposite process to the construction of a product measure.
In mathematics, an invariant measure is a measure that is preserved by some function. The function may be a geometric transformation. For examples, circular angle is invariant under rotation, hyperbolic angle is invariant under squeeze mapping, and a difference of slopes is invariant under shear mapping.
In mathematics, the notions of prevalence and shyness are notions of "almost everywhere" and "measure zero" that are well-suited to the study of infinite-dimensional spaces and make use of the translation-invariant Lebesgue measure on finite-dimensional real spaces. The term "shy" was suggested by the American mathematician John Milnor.
In mathematics, the integral of a non-negative function of a single variable can be regarded, in the simplest case, as the area between the graph of that function and the x-axis. The Lebesgue integral, named after French mathematician Henri Lebesgue, extends the integral to a larger class of functions. It also extends the domains on which these functions can be defined.
{{cite journal}}: CS1 maint: multiple names: authors list (link)