In mathematics, specifically in measure theory, a Borel measure on a topological space is a measure that is defined on all open sets. Some authors require additional restrictions on the measure, as described below.
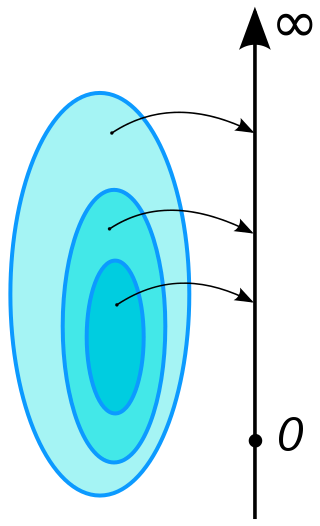
In mathematics, the concept of a measure is a generalization and formalization of geometrical measures and other common notions, such as magnitude, mass, and probability of events. These seemingly distinct concepts have many similarities and can often be treated together in a single mathematical context. Measures are foundational in probability theory, integration theory, and can be generalized to assume negative values, as with electrical charge. Far-reaching generalizations of measure are widely used in quantum physics and physics in general.
In mathematics, a topological space is called separable if it contains a countable, dense subset; that is, there exists a sequence of elements of the space such that every nonempty open subset of the space contains at least one element of the sequence.
In mathematical analysis and in probability theory, a σ-algebra on a set X is a nonempty collection Σ of subsets of X closed under complement, countable unions, and countable intersections. The ordered pair is called a measurable space.
In mathematics, the Lp spaces are function spaces defined using a natural generalization of the p-norm for finite-dimensional vector spaces. They are sometimes called Lebesgue spaces, named after Henri Lebesgue, although according to the Bourbaki group they were first introduced by Frigyes Riesz.
In the mathematical field of measure theory, an outer measure or exterior measure is a function defined on all subsets of a given set with values in the extended real numbers satisfying some additional technical conditions. The theory of outer measures was first introduced by Constantin Carathéodory to provide an abstract basis for the theory of measurable sets and countably additive measures. Carathéodory's work on outer measures found many applications in measure-theoretic set theory, and was used in an essential way by Hausdorff to define a dimension-like metric invariant now called Hausdorff dimension. Outer measures are commonly used in the field of geometric measure theory.
In mathematics, the ba space of an algebra of sets is the Banach space consisting of all bounded and finitely additive signed measures on . The norm is defined as the variation, that is
In mathematics, the total variation identifies several slightly different concepts, related to the (local or global) structure of the codomain of a function or a measure. For a real-valued continuous function f, defined on an interval [a, b] ⊂ R, its total variation on the interval of definition is a measure of the one-dimensional arclength of the curve with parametric equation x ↦ f(x), for x ∈ [a, b]. Functions whose total variation is finite are called functions of bounded variation.
In mathematics, an additive set function is a function mapping sets to numbers, with the property that its value on a union of two disjoint sets equals the sum of its values on these sets, namely, If this additivity property holds for any two sets, then it also holds for any finite number of sets, namely, the function value on the union of k disjoint sets equals the sum of its values on the sets. Therefore, an additive set function is also called a finitely additive set function. However, a finitely additive set function might not have the additivity property for a union of an infinite number of sets. A σ-additive set function is a function that has the additivity property even for countably infinite many sets, that is,
In mathematics, signed measure is a generalization of the concept of (positive) measure by allowing the set function to take negative values, i.e., to acquire sign.
In mathematics, specifically measure theory, a complex measure generalizes the concept of measure by letting it have complex values. In other words, one allows for sets whose size is a complex number.
In mathematics, more precisely in measure theory, an atom is a measurable set which has positive measure and contains no set of smaller positive measure. A measure which has no atoms is called non-atomic or atomless.
In mathematics, the Bochner integral, named for Salomon Bochner, extends the definition of Lebesgue integral to functions that take values in a Banach space, as the limit of integrals of simple functions.
In measure theory, Carathéodory's extension theorem states that any pre-measure defined on a given ring of subsets R of a given set Ω can be extended to a measure on the σ-ring generated by R, and this extension is unique if the pre-measure is σ-finite. Consequently, any pre-measure on a ring containing all intervals of real numbers can be extended to the Borel algebra of the set of real numbers. This is an extremely powerful result of measure theory, and leads, for example, to the Lebesgue measure.
In mathematics, a positive (or signed) measure μ defined on a σ-algebra Σ of subsets of a set X is called a finite measure if μ(X) is a finite real number (rather than ∞). A set A in Σ is of finite measure if μ(A) < ∞. The measure μ is called σ-finite if X is a countable union of measurable sets each with finite measure. A set in a measure space is said to have σ-finite measure if it is a countable union of measurable sets with finite measure. A measure being σ-finite is a weaker condition than being finite, i.e. all finite measures are σ-finite but there are (many) σ-finite measures that are not finite.
In mathematics, cylinder set measure is a kind of prototype for a measure on an infinite-dimensional vector space. An example is the Gaussian cylinder set measure on Hilbert space.
In mathematics, in particular in measure theory, a content is a real-valued function defined on a collection of subsets such that
In real analysis and measure theory, the Vitali convergence theorem, named after the Italian mathematician Giuseppe Vitali, is a generalization of the better-known dominated convergence theorem of Henri Lebesgue. It is a characterization of the convergence in Lp in terms of convergence in measure and a condition related to uniform integrability.
In mathematics, especially measure theory, a set function is a function whose domain is a family of subsets of some given set and that (usually) takes its values in the extended real number line which consists of the real numbers and
In mathematics, lifting theory was first introduced by John von Neumann in a pioneering paper from 1931, in which he answered a question raised by Alfréd Haar. The theory was further developed by Dorothy Maharam (1958) and by Alexandra Ionescu Tulcea and Cassius Ionescu Tulcea (1961). Lifting theory was motivated to a large extent by its striking applications. Its development up to 1969 was described in a monograph of the Ionescu Tulceas. Lifting theory continued to develop since then, yielding new results and applications.































