The cant of a railway track or camber of a road (also referred to as superelevation, cross slope or cross fall) is the rate of change in elevation (height) between the two rails or edges of the road. This is normally greater where the railway or road is curved; raising the outer rail or the outer edge of the road creates a banked turn, thus allowing vehicles to travel round the curve at faster speeds which would otherwise not be possible if the surface is flat or level.
On railways, cant helps a train steer around a curve, keeping the wheel flanges from touching the rails, minimizing friction, wear and rail squeal.
The main functions of cant are the following:
The necessary cant in a curve depends on the expected speed of the trains and the radius. However, it may be necessary to select a compromise value at design time, for example if slow-moving trains may occasionally use tracks intended for high-speed trains.
Generally the aim is for trains to run without flange contact, which also depends on the tire profile of the wheels. Allowance has to be made for the different speeds of trains. Slower trains will tend to make flange contact with the inner rail on curves, while faster trains will tend to ride outwards and make contact with the outer rail. Either contact causes wear and tear and may lead to derailment. Many high-speed lines do not permit slower freight trains, particularly with heavier axle loads. In some cases, the impact is reduced by the use of flange lubrication.
Ideally, the track should have sleepers (railroad ties) at a closer spacing and a greater depth of ballast to accommodate the increased forces exerted in the curve.
At the ends of a curve, the amount of cant cannot change from zero to its maximum immediately. It must change (ramp) gradually in a track transition curve. The length of the transition depends on the maximum allowable speed; the higher the speed, the greater length is required.
For the United States, with a standard maximum unbalanced superelevation of 75 mm (3 in), the formula is this:
where is the superelevation in inches, is the curvature of the track in degrees per 100 feet, and the maximum speed in MPH.
The maximum value of cant (the height of the outer rail above the inner rail) for a standard gauge railway is approximately 150 mm (6 in).[ citation needed ] For high-speed railways in Europe, maximum cant is 180 mm (7 in) when slow freight trains are not allowed. [1]
Track unbalanced superelevation (cant deficiency) in the United States is restricted to 75 mm (3 in), though 102 mm (4.0 in) is permissible by waiver. The maximum value for European railways varies by country, some of which have curves with over 280 mm (11 in) of unbalanced superelevation to permit high-speed transportation. The highest values are only for tilting trains, because it would be too uncomfortable for passengers in conventional train cars. [2]
Ideally, the amount of cant , given the speed of a train, the radius of curvature and the gauge of the track, the relation
must be fulfilled, with the gravitational acceleration. This follows simply from a balance between weight, centrifugal force, and normal force. In the approximation it is assumed that the cant is small compared to the gauge of the track. It is often convenient to define the unbalanced cant as the maximum allowed additional amount of cant that would be required by a train moving faster than the speed for which the cant was designed, setting the maximum allowed speed . In a formula this becomes
with the curvature of the track, which is also the turn in radians per unit length of track.
In the United States, maximum speed is subject to specific rules. When filling in , and the conversion factors for US customary units, the maximum speed of a train on curved track for a given cant deficiency or unbalanced superelevation is determined by the following formula:
with and in inches, the degree of curvature in degrees per 100 feet and in MPH.
In Australia, the Australian Rail Track Corporation is increasing speed around curves sharper than an 800-metre (2,625 ft) radius by replacing wooden sleepers with concrete ones so that the cant can be increased. [3]
The rails themselves are now usually canted inwards by about 5 to 10 percent.
In 1925 about 15 of 36 major American railways had adopted this practice. [4]
In civil engineering, cant is often referred to as cross slope or camber. It helps rainwater drain from the road surface. Along straight or gently curved sections, the middle of the road is normally higher than the edges. This is called "normal crown" and helps shed rainwater off the sides of the road. During road works that involve lengths of temporary carriageway, the slope may be the opposite to normal – for example, with the outer edge higher – which causes vehicles to lean towards oncoming traffic. In the UK, this is indicated on warning signs as "adverse camber".
On more severe bends, the outside edge of the curve is raised, or superelevated, to help vehicles around the curve. The amount of superelevation increases with its design speed and with curve sharpness.
An off-camber corner is described as the opposite of a banked turn, or a negative-bank turn, which is lower on the outside of a turn than on the inside. [5] [6] Off-camber corners are both feared and celebrated by skilled drivers. [7] [8] Handling them is a major factor in skilled vehicle control, both single-track and automotive; both engine-powered and human-powered vehicles; both on and off closed courses; and both on and off paved surfaces.[ citation needed ]
On race courses, they are one of a handful of engineering factors at the disposal of a course designer in order to challenge and test drivers' skills. [9] Off-camber corners were described by a training guide for prospective racers as "the hardest corners you will encounter" on the track. [10] Many notable courses such as Riverside International Raceway combine off-camber corners with elevation and link corners for extra driver challenge. [11]
On the street, they are a feature of some of the world's most celebrated paved roads, such as The "Dragon" (US 129) through Deals Gap [12] and the "Diamondback" (NC 226A) in North Carolina, [13] Route 78 in Ohio, [14] Route 125 in Pennsylvania, [15] Route 33 in California, [16] and Betws-y-Coed Triangle at Snowdonia National Park in Wales. [17]
To mountain bikers and motorcyclists on trails and dirt tracks, off-camber corners are also challenging, and can be either an engineered course feature, or a natural feature of single-track trails. [18] [19] [20] [21] In cyclocross, off-camber sections are very common as the courses snake around ridges, adding difficulty.
Camber in virtual race circuits is carefully controlled by video game race simulators to achieve the designer's desired level of difficulty. [9]
A bogie is a chassis or framework that carries a wheelset, attached to a vehicle—a modular subassembly of wheels and axles. Bogies take various forms in various modes of transport. A bogie may remain normally attached or be quickly detachable ; it may contain a suspension within it, or be solid and in turn be suspended ; it may be mounted on a swivel, as traditionally on a railway carriage or locomotive, additionally jointed and sprung, or held in place by other means.
A railroad switch (AE), turnout, or [set of] points (CE) is a mechanical installation enabling railway trains to be guided from one track to another, such as at a railway junction or where a spur or siding branches off.
In structural engineering, buckling is the sudden change in shape (deformation) of a structural component under load, such as the bowing of a column under compression or the wrinkling of a plate under shear. If a structure is subjected to a gradually increasing load, when the load reaches a critical level, a member may suddenly change shape and the structure and component is said to have buckled. Euler's critical load and Johnson's parabolic formula are used to determine the buckling stress of a column.
In rail transport, a derailment occurs when a rail vehicle such as a train comes off its rails. Although many derailments are minor, all result in temporary disruption of the proper operation of the railway system and they are a potentially serious hazard.
An adhesion railway relies on adhesion traction to move the train. Adhesion traction is the friction between the drive wheels and the steel rail. The term "adhesion railway" is used only when it is necessary to distinguish adhesion railways from railways moved by other means, such as by a stationary engine pulling on a cable attached to the cars or by railways that are moved by a pinion meshing with a rack.
A banked turn is a turn or change of direction in which the vehicle banks or inclines, usually towards the inside of the turn. For a road or railroad this is usually due to the roadbed having a transverse down-slope towards the inside of the curve. The bank angle is the angle at which the vehicle is inclined about its longitudinal axis with respect to the horizontal.
A transition curve is a spiral-shaped length of highway or railroad track that is used between sections having different profiles and radii, such as between straightaways (tangents) and curves, or between two different curves.
Hunting oscillation is a self-oscillation, usually unwanted, about an equilibrium. The expression came into use in the 19th century and describes how a system "hunts" for equilibrium. The expression is used to describe phenomena in such diverse fields as electronics, aviation, biology, and railway engineering.
In railway engineering, cant deficiency is defined in the context of travel of a rail vehicle at constant speed on a constant-radius curve. Cant itself refers to the superelevation of the curve, that is, the difference between the elevations of the outside and inside rails. Cant deficiency is present when a rail vehicle's speed on the curve is greater than the speed at which the components of wheel to rail force are normal to the plane of the track. In that case, the resultant force exerts on the outside rail more than the inside rail, in which it creates lateral acceleration toward the outside of the curve. In order to reduce cant deficiency, the speed can be reduced or the superelevation can be increased. The amount of cant deficiency is expressed in terms of required superelevation to be added in order to bring the resultant force into balance between the two rails.
Cross slope, cross fall or camber is a geometric feature of pavement surfaces: the transverse slope with respect to the horizon. It is a very important safety factor. Cross slope is provided to provide a drainage gradient so that water will run off the surface to a drainage system such as a street gutter or ditch. Inadequate cross slope will contribute to aquaplaning. On straight sections of normal two-lane roads, the pavement cross section is usually highest in the center and drains to both sides. In horizontal curves, the cross slope is banked into superelevation to reduce steering effort and lateral force required to go around the curve. All water drains to the inside of the curve. If the cross slope magnitude oscillates within 1–25 metres (3–82 ft), the body and payload of high (heavy) vehicles will experience high roll vibration.
The Ewing System is a balancing monorail system developed in the late 19th century by British inventor W. J. Ewing. It is not to be confused with the much later system patented by Robert W. Ewing.
In railway engineering, curve resistance is a part of train resistance, namely the additional rolling resistance a train must overcome when travelling on a curved section of track. Curve resistance is typically measured in per mille, with the correct physical unit being Newton per kilo-Newton (N/kN). Older texts still use the wrong unit of kilogram-force per tonne (kgf/t).
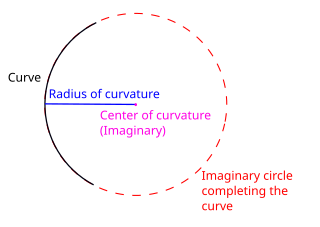
In differential geometry, the radius of curvature (Rc), R, is the reciprocal of the curvature. For a curve, it equals the radius of the circular arc which best approximates the curve at that point. For surfaces, the radius of curvature is the radius of a circle that best fits a normal section or combinations thereof.
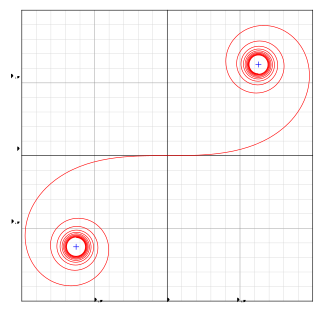
An Euler spiral is a curve whose curvature changes linearly with its curve length. Euler spirals are also commonly referred to as spiros, clothoids, or Cornu spirals.
The minimum railway curve radius is the shortest allowable design radius for the centerline of railway tracks under a particular set of conditions. It has an important bearing on construction costs and operating costs and, in combination with superelevation in the case of train tracks, determines the maximum safe speed of a curve. The minimum radius of a curve is one parameter in the design of railway vehicles as well as trams; monorails and automated guideways are also subject to a minimum radius.
Rail speed limits in the United States are regulated by the Federal Railroad Administration. Railroads also implement their own limits and enforce speed limits. Speed restrictions are based on a number of factors including curvature, signaling, track condition, and the presence of grade crossings. Like road speed limits in the United States, speed limits for tracks and trains are measured in miles per hour (mph).
The geometric design of roads is the branch of highway engineering concerned with the positioning of the physical elements of the roadway according to standards and constraints. The basic objectives in geometric design are to optimize efficiency and safety while minimizing cost and environmental damage. Geometric design also affects an emerging fifth objective called "livability," which is defined as designing roads to foster broader community goals, including providing access to employment, schools, businesses and residences, accommodate a range of travel modes such as walking, bicycling, transit, and automobiles, and minimizing fuel use, emissions and environmental damage.
A railway or railroad is a track where the vehicle travels over two parallel steel bars, called rails. The rails support and guide the wheels of the vehicles, which are traditionally either trains or trams. Modern light rail is a relatively new innovation which combines aspects of those two modes of transport. However fundamental differences in the track and wheel design are important, especially where trams or light railways and trains have to share a section of track, as sometimes happens in congested areas.
Track geometry is concerned with the properties and relations of points, lines, curves, and surfaces in the three-dimensional positioning of railroad track. The term is also applied to measurements used in design, construction and maintenance of track. Track geometry involves standards, speed limits and other regulations in the areas of track gauge, alignment, elevation, curvature and track surface. Standards are usually separately expressed for horizontal and vertical layouts although track geometry is three-dimensional.
Rail squeal is a screeching train-track friction sound, commonly occurring on sharp curves.
Concrete re-sleepering of all curves of less than an 810-metre radius, using some 220,000 sleepers to increase cant deficiency and super-elevation, will be undertaken allowing for increased train speeds and further reducing transit times.
Off camber turn: An off camber turn is the opposite of a banked turn. It is lower on the outside of a turn than on the inside.
Tight turns and some off-camber curves make this road a delight.
Off camber corners: These corners are the hardest corners you will encounter...
Riverside International Raceway is a good example of a course with no isolated textbook turns: Every corner is either combined with another, or banked, off-camber, rising or falling.
[One of] the two worst corners [is] "Guardrail cliff", a sharp off-camber left ...
[It] offers all types of curves—off-camber tight stuff, sweepers and esses that make me scramble from one side of the bike to the other while my foot stabs for the right gear.
Changing elevation a thousand feet at a time as it snakes over six mountain passes it offers no rest from decreasing radius, off-camber, blind and switchback curves.
Route 33 has everything. It rolls across the Santa Ynez Mountains and plunges into the Cuyama Valley in relentlessly interesting ways. That includes midcorner elevation changes, off-camber hairpins, tightening-radius sweepers and straights long enough to hit terminal velocity. It's 72 miles of pure entertainment.
