See also
Look up instant in Wiktionary, the free dictionary.
In physics and the philosophy of science, instant refers to an infinitesimal interval in time, whose passage is instantaneous. In ordinary speech, an instant has been defined as "a point or very short space of time," a notion deriving from its etymological source, the Latin verb instare, from in- + stare ('to stand'), meaning 'to stand upon or near.' [1]
The continuous nature of time and its infinite divisibility was addressed by Aristotle in his Physics , where he wrote on Zeno's paradoxes. The philosopher and mathematician Bertrand Russell was still seeking to define the exact nature of an instant thousands of years later. [2] In 2024, John William Stafford used algorithms to demonstrate that a time difference of zero could theoretically continue to expand (in various ways) to infinity, and subsequently described a new concept that he referred to as instantaneous. He concluded by stating that instantaneous is, with respect to the measurement of time, mutually exclusive. [3] In addition, a theoretical model of multiple Universes was proposed which exist within the context of instantaneous.
As of October 2020 [update] , the smallest time interval certified in regulated measurements is on the order of 397 zeptoseconds (397 × 10−21 seconds). [4]
Instant (usually abbreviated in print to inst.) can be used to indicate "Of the current month". For example, "the 11th inst." means the 11th day of the current month, whether that date is in the past, or the future, from the date of publication. [5] [6] [7]
Calculus is the mathematical study of continuous change, in the same way that geometry is the study of shape, and algebra is the study of generalizations of arithmetic operations.
ISO 8601 is an international standard covering the worldwide exchange and communication of date and time-related data. It is maintained by the International Organization for Standardization (ISO) and was first published in 1988, with updates in 1991, 2000, 2004, and 2019, and an amendment in 2022. The standard provides a well-defined, unambiguous method of representing calendar dates and times in worldwide communications, especially to avoid misinterpreting numeric dates and times when such data is transferred between countries with different conventions for writing numeric dates and times.
Measurement is the quantification of attributes of an object or event, which can be used to compare with other objects or events. In other words, measurement is a process of determining how large or small a physical quantity is as compared to a basic reference quantity of the same kind. The scope and application of measurement are dependent on the context and discipline. In natural sciences and engineering, measurements do not apply to nominal properties of objects or events, which is consistent with the guidelines of the International vocabulary of metrology published by the International Bureau of Weights and Measures. However, in other fields such as statistics as well as the social and behavioural sciences, measurements can have multiple levels, which would include nominal, ordinal, interval and ratio scales.
The speed of light in vacuum, commonly denoted c, is a universal physical constant that is exactly equal to 299,792,458 metres per second. According to the special theory of relativity, c is the upper limit for the speed at which conventional matter or energy can travel through space.
In kinematics, the speed of an object is the magnitude of the change of its position over time or the magnitude of the change of its position per unit of time; it is thus a non-negative scalar quantity. The average speed of an object in an interval of time is the distance travelled by the object divided by the duration of the interval; the instantaneous speed is the limit of the average speed as the duration of the time interval approaches zero. Speed is the magnitude of velocity, which indicates additionally the direction of motion.
Superluminal communication is a hypothetical process in which information is conveyed at faster-than-light speeds. The current scientific consensus is that faster-than-light communication is not possible, and to date it has not been achieved in any experiment.
Time is the continued sequence of existence and events that occurs in an apparently irreversible succession from the past, through the present, and into the future. It is a component quantity of various measurements used to sequence events, to compare the duration of events or the intervals between them, and to quantify rates of change of quantities in material reality or in the conscious experience. Time is often referred to as a fourth dimension, along with three spatial dimensions.
Zeno's paradoxes are a series of philosophical arguments presented by the ancient Greek philosopher Zeno of Elea, primarily known through the works of Plato, Aristotle, and later commentators like Simplicius of Cilicia. Zeno devised these paradoxes to support his teacher Parmenides's philosophy of monism, which posits that despite our sensory experiences, reality is singular and unchanging. The paradoxes famously challenge the notions of plurality, motion, space, and time by suggesting they lead to logical contradictions.
A pendulum is a device made of a weight suspended from a pivot so that it can swing freely. When a pendulum is displaced sideways from its resting, equilibrium position, it is subject to a restoring force due to gravity that will accelerate it back toward the equilibrium position. When released, the restoring force acting on the pendulum's mass causes it to oscillate about the equilibrium position, swinging back and forth. The time for one complete cycle, a left swing and a right swing, is called the period. The period depends on the length of the pendulum and also to a slight degree on the amplitude, the width of the pendulum's swing.
Newton's laws of motion are three physical laws that describe the relationship between the motion of an object and the forces acting on it. These laws, which provide the basis for Newtonian mechanics, can be paraphrased as follows:
In philosophy, the philosophy of physics deals with conceptual and interpretational issues in physics, many of which overlap with research done by certain kinds of theoretical physicists. Historically, philosophers of physics have engaged with questions such as the nature of space, time, matter and the laws that govern their interactions, as well as the epistemological and ontological basis of the theories used by practicing physicists. The discipline draws upon insights from various areas of philosophy, including metaphysics, epistemology, and philosophy of science, while also engaging with the latest developments in theoretical and experimental physics.
In mathematics, an infinitesimal number is a non-zero quantity that is closer to 0 than any non-zero real number is. The word infinitesimal comes from a 17th-century Modern Latin coinage infinitesimus, which originally referred to the "infinity-eth" item in a sequence.
Quantity or amount is a property that can exist as a multitude or magnitude, which illustrate discontinuity and continuity. Quantities can be compared in terms of "more", "less", or "equal", or by assigning a numerical value multiple of a unit of measurement. Mass, time, distance, heat, and angle are among the familiar examples of quantitative properties.
A shake is an informal metric unit of time equal to 10 nanoseconds, or 10−8 seconds. It was originally coined for use in nuclear physics, helping to conveniently express the timing of various events in a nuclear reaction.
A unit of time is any particular time interval, used as a standard way of measuring or expressing duration. The base unit of time in the International System of Units (SI), and by extension most of the Western world, is the second, defined as about 9 billion oscillations of the caesium atom. The exact modern SI definition is "[The second] is defined by taking the fixed numerical value of the cesium frequency, ΔνCs, the unperturbed ground-state hyperfine transition frequency of the cesium 133 atom, to be 9192631770 when expressed in the unit Hz, which is equal to s−1."
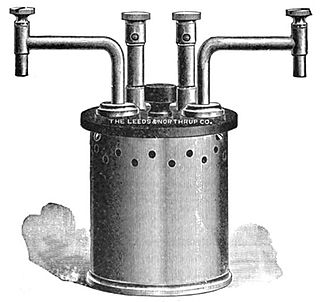
The ohm is the unit of electrical resistance in the International System of Units (SI). It is named after German physicist Georg Ohm. Various empirically derived standard units for electrical resistance were developed in connection with early telegraphy practice, and the British Association for the Advancement of Science proposed a unit derived from existing units of mass, length and time, and of a convenient scale for practical work as early as 1861.
Continuum theories or models explain variation as involving gradual quantitative transitions without abrupt changes or discontinuities. In contrast, categorical theories or models explain variation using qualitatively different states.
Jiffy can be an informal term for any unspecified short period, as in "I will be back in a jiffy". From this, it has acquired a number of more precise applications as the name of multiple units of measurement, each used to express or measure very brief durations of time. First attested in 1780, the word's origin is unclear, though one suggestion is that it was thieves' cant for lightning. It was common in a number of Scots English dialects and in John Jamieson's Etymological Dictionary of the Scottish Language (1808) it is suggested that it is a corruption of 'gliff' (glimpse) or 'gliffin' (glance) and may ultimately derive from Gothic or Teutonic words for 'shine'.
In mathematical dynamics, discrete time and continuous time are two alternative frameworks within which variables that evolve over time are modeled.
{{cite book}}: CS1 maint: location missing publisher (link)| Key concepts | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Measurement and standards |
| ||||||||
| Philosophy of time | |||||||||
| Human experience and use of time | |||||||||
| Time in science |
| ||||||||
| Related | |||||||||
| International standards |
|   |
|---|---|---|
| Obsolete standards | ||
| Time in physics | ||
| Horology | ||
| Calendar | ||
| Archaeology and geology | ||
| Astronomical chronology | ||
| Other units of time | ||
| Related topics | ||
 | This philosophy of science-related article is a stub. You can help Wikipedia by expanding it. |
 | This time-related article is a stub. You can help Wikipedia by expanding it. |
