Financial economics is the branch of economics characterized by a "concentration on monetary activities", in which "money of one type or another is likely to appear on both sides of a trade". Its concern is thus the interrelation of financial variables, such as share prices, interest rates and exchange rates, as opposed to those concerning the real economy. It has two main areas of focus: asset pricing and corporate finance; the first being the perspective of providers of capital, i.e. investors, and the second of users of capital. It thus provides the theoretical underpinning for much of finance.
New Keynesian economics is a school of macroeconomics that strives to provide microeconomic foundations for Keynesian economics. It developed partly as a response to criticisms of Keynesian macroeconomics by adherents of new classical macroeconomics.
The Black–Scholes or Black–Scholes–Merton model is a mathematical model for the dynamics of a financial market containing derivative investment instruments, using various underlying assumptions. From the parabolic partial differential equation in the model, known as the Black–Scholes equation, one can deduce the Black–Scholes formula, which gives a theoretical estimate of the price of European-style options and shows that the option has a unique price given the risk of the security and its expected return. The equation and model are named after economists Fischer Black and Myron Scholes. Robert C. Merton, who first wrote an academic paper on the subject, is sometimes also credited.
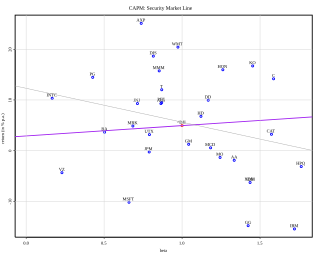
In finance, the capital asset pricing model (CAPM) is a model used to determine a theoretically appropriate required rate of return of an asset, to make decisions about adding assets to a well-diversified portfolio.

Prospect theory is a theory of behavioral economics, judgment and decision making that was developed by Daniel Kahneman and Amos Tversky in 1979. The theory was cited in the decision to award Kahneman the 2002 Nobel Memorial Prize in Economics.
In mathematical finance, a risk-neutral measure is a probability measure such that each share price is exactly equal to the discounted expectation of the share price under this measure. This is heavily used in the pricing of financial derivatives due to the fundamental theorem of asset pricing, which implies that in a complete market, a derivative's price is the discounted expected value of the future payoff under the unique risk-neutral measure. Such a measure exists if and only if the market is arbitrage-free.
The equity premium puzzle refers to the inability of an important class of economic models to explain the average equity risk premium (ERP) provided by a diversified portfolio of equities over that of government bonds, which has been observed for more than 100 years. There is a significant disparity between returns produced by stocks compared to returns produced by government treasury bills. The equity premium puzzle addresses the difficulty in understanding and explaining this disparity. This disparity is calculated using the equity risk premium:
In economics, a puzzle is a situation where the implication of theory is inconsistent with observed economic data.
In economics, incomplete markets are markets in which there does not exist an Arrow–Debreu security for every possible state of nature. In contrast with complete markets, this shortage of securities will likely restrict individuals from transferring the desired level of wealth among states.
Martingale pricing is a pricing approach based on the notions of martingale and risk neutrality. The martingale pricing approach is a cornerstone of modern quantitative finance and can be applied to a variety of derivatives contracts, e.g. options, futures, interest rate derivatives, credit derivatives, etc.

Cumulative prospect theory (CPT) is a model for descriptive decisions under risk and uncertainty which was introduced by Amos Tversky and Daniel Kahneman in 1992. It is a further development and variant of prospect theory. The difference between this version and the original version of prospect theory is that weighting is applied to the cumulative probability distribution function, as in rank-dependent expected utility theory but not applied to the probabilities of individual outcomes. In 2002, Daniel Kahneman received the Bank of Sweden Prize in Economic Sciences in Memory of Alfred Nobel for his contributions to behavioral economics, in particular the development of Cumulative Prospect Theory (CPT).
Rajnish Mehra is an Indian American financial economist. He currently holds the E.N. Basha Arizona Heritage Endowed Chair at the Arizona State University and is a Professor of Economics Emeritus at the University of California, Santa Barbara.
Consumption smoothing is an economic concept for the practice of optimizing a person's standard of living through an appropriate balance between savings and consumption over time. An optimal consumption rate should be relatively similar at each stage of a person's life rather than fluctuate wildly. Luxurious consumption at an old age does not compensate for an impoverished existence at other stages in one's life.
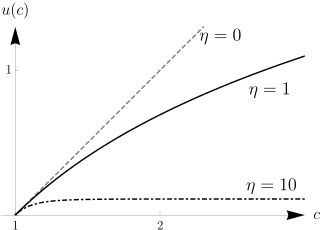
In economics, the isoelastic function for utility, also known as the isoelastic utility function, or power utility function, is used to express utility in terms of consumption or some other economic variable that a decision-maker is concerned with. The isoelastic utility function is a special case of hyperbolic absolute risk aversion and at the same time is the only class of utility functions with constant relative risk aversion, which is why it is also called the CRRA utility function. In statistics, the same function is called the Box-Cox transformation.
The real exchange-rate puzzles is a common term for two much-discussed anomalies of real exchange rates: that real exchange rates are more volatile and show more persistence than what most models can account for. These two anomalies are sometimes referred to as the purchasing power parity puzzles.
In macroeconomics, the cost of business cycles is the decrease in social welfare, if any, caused by business cycle fluctuations.
The random walk model of consumption was introduced by economist Robert Hall. This model uses the Euler numerical method to model consumption. He created his consumption theory in response to the Lucas critique. Using Euler equations to model the random walk of consumption has become the dominant approach to modeling consumption.
Financial correlations measure the relationship between the changes of two or more financial variables over time. For example, the prices of equity stocks and fixed interest bonds often move in opposite directions: when investors sell stocks, they often use the proceeds to buy bonds and vice versa. In this case, stock and bond prices are negatively correlated.
Ravi Bansal is an economist and an academic. He is J.B. Fuqua Professor at the Fuqua School of Business at Duke University and Research Associate at the National Bureau of Economic Research. He is most known for his research work in the fields of financial economics and macroeconomics.











