
A centripetal force is a force that makes a body follow a curved path. The direction of the centripetal force is always orthogonal to the motion of the body and towards the fixed point of the instantaneous center of curvature of the path. Isaac Newton described it as "a force by which bodies are drawn or impelled, or in any way tend, towards a point as to a centre". In Newtonian mechanics, gravity provides the centripetal force causing astronomical orbits.

In celestial mechanics, an orbit is the curved trajectory of an object such as the trajectory of a planet around a star, or of a natural satellite around a planet, or of an artificial satellite around an object or position in space such as a planet, moon, asteroid, or Lagrange point. Normally, orbit refers to a regularly repeating trajectory, although it may also refer to a non-repeating trajectory. To a close approximation, planets and satellites follow elliptic orbits, with the center of mass being orbited at a focal point of the ellipse, as described by Kepler's laws of planetary motion.

In mathematics and physics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace, who first studied its properties. This is often written as
In mathematics, the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a scalar function on Euclidean space. It is usually denoted by the symbols , (where is the nabla operator), or . In a Cartesian coordinate system, the Laplacian is given by the sum of second partial derivatives of the function with respect to each independent variable. In other coordinate systems, such as cylindrical and spherical coordinates, the Laplacian also has a useful form. Informally, the Laplacian Δf (p) of a function f at a point p measures by how much the average value of f over small spheres or balls centered at p deviates from f (p).
Linear elasticity is a mathematical model of how solid objects deform and become internally stressed due to prescribed loading conditions. It is a simplification of the more general nonlinear theory of elasticity and a branch of continuum mechanics.
The Kerr–Newman metric is the most general asymptotically flat and stationary solution of the Einstein–Maxwell equations in general relativity that describes the spacetime geometry in the region surrounding an electrically charged and rotating mass. It generalizes the Kerr metric by taking into account the field energy of an electromagnetic field, in addition to describing rotation. It is one of a large number of various different electrovacuum solutions; that is, it is a solution to the Einstein–Maxwell equations that account for the field energy of an electromagnetic field. Such solutions do not include any electric charges other than that associated with the gravitational field, and are thus termed vacuum solutions.

In modern models of physical cosmology, a dark matter halo is a basic unit of cosmological structure. It is a hypothetical region that has decoupled from cosmic expansion and contains gravitationally bound matter. A single dark matter halo may contain multiple virialized clumps of dark matter bound together by gravity, known as subhalos. Modern cosmological models, such as ΛCDM, propose that dark matter halos and subhalos may contain galaxies. The dark matter halo of a galaxy envelops the galactic disc and extends well beyond the edge of the visible galaxy. Thought to consist of dark matter, halos have not been observed directly. Their existence is inferred through observations of their effects on the motions of stars and gas in galaxies and gravitational lensing. Dark matter halos play a key role in current models of galaxy formation and evolution. Theories that attempt to explain the nature of dark matter halos with varying degrees of success include cold dark matter (CDM), warm dark matter, and massive compact halo objects (MACHOs).
In theoretical physics, Nordström's theory of gravitation was a predecessor of general relativity. Strictly speaking, there were actually two distinct theories proposed by the Finnish theoretical physicist Gunnar Nordström, in 1912 and 1913 respectively. The first was quickly dismissed, but the second became the first known example of a metric theory of gravitation, in which the effects of gravitation are treated entirely in terms of the geometry of a curved spacetime.
In the mathematical description of general relativity, the Boyer–Lindquist coordinates are a generalization of the coordinates used for the metric of a Schwarzschild black hole that can be used to express the metric of a Kerr black hole.
In physical cosmology, cosmological perturbation theory is the theory by which the evolution of structure is understood in the Big Bang model. Cosmological perturbation theory may be broken into two categories: Newtonian or general relativistic. Each case uses its governing equations to compute gravitational and pressure forces which cause small perturbations to grow and eventually seed the formation of stars, quasars, galaxies and clusters. Both cases apply only to situations where the universe is predominantly homogeneous, such as during cosmic inflation and large parts of the Big Bang. The universe is believed to still be homogeneous enough that the theory is a good approximation on the largest scales, but on smaller scales more involved techniques, such as N-body simulations, must be used. When deciding whether to use general relativity for perturbation theory, note that Newtonian physics is only applicable in some cases such as for scales smaller than the Hubble horizon, where spacetime is sufficiently flat, and for which speeds are non-relativistic.
The Newman–Penrose (NP) formalism is a set of notation developed by Ezra T. Newman and Roger Penrose for general relativity (GR). Their notation is an effort to treat general relativity in terms of spinor notation, which introduces complex forms of the usual variables used in GR. The NP formalism is itself a special case of the tetrad formalism, where the tensors of the theory are projected onto a complete vector basis at each point in spacetime. Usually this vector basis is chosen to reflect some symmetry of the spacetime, leading to simplified expressions for physical observables. In the case of the NP formalism, the vector basis chosen is a null tetrad: a set of four null vectors—two real, and a complex-conjugate pair. The two real members often asymptotically point radially inward and radially outward, and the formalism is well adapted to treatment of the propagation of radiation in curved spacetime. The Weyl scalars, derived from the Weyl tensor, are often used. In particular, it can be shown that one of these scalars— in the appropriate frame—encodes the outgoing gravitational radiation of an asymptotically flat system.
In physics, the Green's function for the Laplacian in three variables is used to describe the response of a particular type of physical system to a point source. In particular, this Green's function arises in systems that can be described by Poisson's equation, a partial differential equation (PDE) of the form
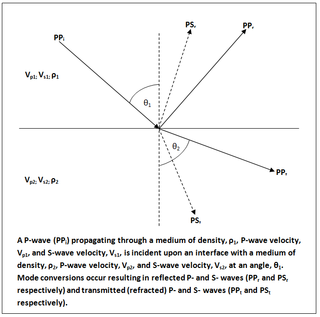
In geophysics and reflection seismology, the Zoeppritz equations are a set of equations that describe the partitioning of seismic wave energy at an interface, due to mode conversion. They are named after their author, the German geophysicist Karl Bernhard Zoeppritz, who died before they were published in 1919.
In mathematics, vector spherical harmonics (VSH) are an extension of the scalar spherical harmonics for use with vector fields. The components of the VSH are complex-valued functions expressed in the spherical coordinate basis vectors.
In astronomy, rotational Brownian motion is the random walk in orientation of a binary star's orbital plane, induced by gravitational perturbations from passing stars.
The Press–Schechter formalism is a mathematical model for predicting the number of objects of a certain mass within a given volume of the Universe. It was described in an academic paper by William H. Press and Paul Schechter in 1974.
In astrophysics, the virial mass is the mass of a gravitationally bound astrophysical system, assuming the virial theorem applies. In the context of galaxy formation and dark matter halos, the virial mass is defined as the mass enclosed within the virial radius of a gravitationally bound system, a radius within which the system obeys the virial theorem. The virial radius is determined using a "top-hat" model. A spherical "top hat" density perturbation destined to become a galaxy begins to expand, but the expansion is halted and reversed due to the mass collapsing under gravity until the sphere reaches equilibrium – it is said to be virialized. Within this radius, the sphere obeys the virial theorem which says that the average kinetic energy is equal to minus one half times the average potential energy, , and this radius defines the virial radius.

The Jeans equations are a set of partial differential equations that describe the motion of a collection of stars in a gravitational field. The Jeans equations relate the second-order velocity moments to the density and potential of a stellar system for systems without collision. They are analogous to the Euler equations for fluid flow and may be derived from the collisionless Boltzmann equation. The Jeans equations can come in a variety of different forms, depending on the structure of what is being modelled. Most utilization of these equations has been found in simulations with large number of gravitationally bound objects.
In optics, the Fraunhofer diffraction equation is used to model the diffraction of waves when the diffraction pattern is viewed at a long distance from the diffracting object, and also when it is viewed at the focal plane of an imaging lens.
Blade element momentum theory is a theory that combines both blade element theory and momentum theory. It is used to calculate the local forces on a propeller or wind-turbine blade. Blade element theory is combined with momentum theory to alleviate some of the difficulties in calculating the induced velocities at the rotor.






























