This article has no lead section .(March 2020) |
This article needs attention from an expert in computing. The specific problem is: Add a lead and prune the article to reduce the detail.(January 2022) |
This article has no lead section .(March 2020) |
This article needs attention from an expert in computing. The specific problem is: Add a lead and prune the article to reduce the detail.(January 2022) |

When a visual display with non-vanishing size is seen by an observer, every point of the display area is seen from a different direction as illustrated in fig. 1. No two spots on the display are seen from the same direction. The larger the display is and the closer the observer is to the display the more the viewing direction varies over the surface area of the display.
Colloquially, the viewing direction is often called "viewing angle". This is an ill-chosen expression which should be avoided, because the viewing direction is specified by two polar angles: the angle of inclination, θ (measured from the surface normal of the display) and the azimuth angle, Φ, measured in the plane of the display as shown in figure 3.

In fig. 2, the eyeball represents the observer that is looking at a specific spot on the display which is identical to the origin of the polar coordinate system. The green arrow is the viewing direction (i.e. direction of observation). The viewing direction is specified by the angle of inclination, θ, measured from the surface normal of the display (blue vertical arrow) while the azimuth angle, Φ, is the angle that the projection of the viewing direction onto the surface of the display makes with the x-axis (red arrow). The projection of the viewing direction is shown here as the shadow of the green arrow. The azimuth angle Φ increases counterclockwise as illustrated in figure 3.

The multitude of directions, from which a display can be seen without artifacts and distortions that would render its intended use impossible (e.g. computerized office work, television, entertainment) is called the viewing cone (even though its shape might be that of a generalized cone).

The concept of the viewing cone has been introduced for the first time in the international standard ISO 13406-2:2001 "Ergonomic requirements for work with visual displays based on flat panels – Part 2: Ergonomic requirements for flat panel displays". This standard provides a classification for computer monitors with LCDs according to the range of viewing directions that can safely be used for the intended task (here: office work) without "reduced visual performance". The classification is according to "Viewing Direction Range Classes" with the "range of viewing directions" being equivalent to the viewing cone.
ISO 13406-2 describes a complex procedure according to which the usable viewing cone can be evaluated from measurements of luminance and chromaticity versus direction of observation. ISO 13406-2 introduces 4 viewing direction range classes of which the first (class I) is a wide viewing cone for many simultaneous observers and the last (class IV) is a so-called "privacy display" with a severely limited viewing cone.
Depending on the actual task to be performed with a certain display device (e.g. office work, entertainment, home theater. etc.) the requirements for the display are different. Compliance routes for different display applications can now be found in the successor standard ISO 9241-300.
Viewing directions are conveniently represented in a polar coordinate system with the angle of inclination, θ, being represented by the radial distance from the origin and the azimuth, Φ, increasing counterclockwise as shown in figure 4. In this coordinate system every point corresponds to one viewing direction. A viewing cone is thus defined by a locus (a closed line) in this coordinate system as indicated by the rectangle and the ellipse in fig. 4.
If a viewing cone is specified by four directions only (e.g. in the horizontal and the vertical plane), it does not become clear if it is the rectangle or the elliptical cone according to fig. 4. In order to resolve this ambiguity, the viewing cone should be specified by at least 8 directions, located in the horizontal and vertical plane and in the two diagonal planes (Φ = 45° and 135°).
Each direction in the polar coordinate system of fig. 4 can be assigned a (scalar) physical quantity, e.g. luminance, contrast, etc.. This quantity can then be represented by lines of equal values (contour lines), by shades of gray or by pseudo-colors (as shown in fig. 4).
A viewing cone can be defined starting from a certain application and the related geometry of observation, from which a range of directions can be obtained that specify the viewing cone required for that task. Inside this viewing cone certain physical parameters that are related to the visual performance of the display device must remain within certain (task dependent) limits.
A viewing cone can also result from measurements (versus viewing direction) carried out with a certain display device under specified operating conditions. Then the viewing cone is obtained by limiting values of a visual quantity (e.g. contrast) which for a certain application is required to be above e.g. 10 (compare e.g. Vesa FPDM2 307-4 Viewing-cone thresholds). Then the line for which the contrast equals 10 defines the viewing cone.
Recent experiments [1] have shown that the acceptable viewing cone is rather determined by decrease of luminance and change of chromaticity than by the decrease of contrast. Comprehensive comparisons between experiments and measurements have been carried out in order to identify the quantities and the corresponding limiting values that define the apparent viewing cone for television screens with LCDs and PDPs. One of the results is that "the luminance at intermediate-to-high gray levels determines the viewingdirection dependent quality and not the contrast ratio." This is found to be in agreement with other research results that "find a low correlation between contrast ratio and visual assessment value". Furthermore, "not only the chromaticity coordinates of the primaries, but even more those of the white point play an important role and need to be included in a viewing direction dependent metric". The authors conclude that "for LCDs, this new metric results in a viewing cone, which is on the order of 70°–90° (subtended angle), and thus, considerably lower than what is usually specified based on a minimum contrast of 10. For PDPs, this new metric yields the same viewing direction range as the present specification that uses a luminance decrease to 50%". In the terminology as introduced above (and illustrated in Figure 2) a viewing cone of 70°–90° subtended angle means (for a rotationally symmetric viewing cone) a maximum angle of inclination of 35°-45°.

Fig. 5 shows luminance and contrast versus viewing direction in a polar coordinate system. The left column shows the directional luminance distribution of the dark state of the display (here: IPS-LCD), the center column shows the bright state and the right column shows the (luminance) contrast (ratio) resulting from the preceding two luminance distributions. The value is coded by (pseudo) colors. The graphs below the polar coordinate systems each show a cross section in the horizontal plane and indicate the values for luminance and for the contrast.
Each borderline between two (shades of) colors represents a line of constant value, in the case of contrast an iso-contrast (contour) line. Note, that "iso" is used here in the sense of "equal", it does NOT establish a relation to the International Organization for Standardization, ISO.
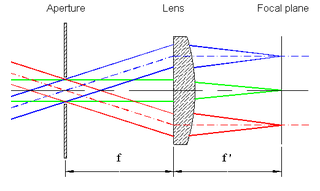
This way of representing the variation of a quantity of a display with direction of observation originates from an optical technique called conoscopy. Conoscopy, originally proposed and used by Maugin for examination of the state of liquid crystal alignment in 1911 [2] has been used in every LCD-laboratory in the late seventies and throughout the eighties for measurement and evaluation of the optical properties of LCDs and for estimation of LCD-contrast as a function of viewing direction. In the conoscopic mode of observation, in the old days often realized with a polarizing microscope, a directions image is generated in the rear focal plane of the objective lens. [3] This directions image [4] is based on the same coordinates as the representation in the polar coordinate system shown in figs. 4 and 5.
The first publication of the variation of the contrast of reflective LCDs measured with a motorized mechanically scanning gonioscopic apparatus and represented as a conoscopic directions figure was published in 1979. [5]

In geography, latitude is a geographic coordinate that specifies the north–south position of a point on the Earth's surface. Latitude is an angle which ranges from 0° at the Equator to 90° at the poles. Lines of constant latitude, or parallels, run east–west as circles parallel to the equator. Latitude is used together with longitude to specify the precise location of features on the surface of the Earth. On its own, the term latitude should be taken to be the geodetic latitude as defined below. Briefly, geodetic latitude at a point is the angle formed by the vector perpendicular to the ellipsoidal surface from that point, and the equatorial plane. Also defined are six auxiliary latitudes that are used in special applications.

Luminance is a photometric measure of the luminous intensity per unit area of light travelling in a given direction. It describes the amount of light that passes through, is emitted from, or is reflected from a particular area, and falls within a given solid angle.
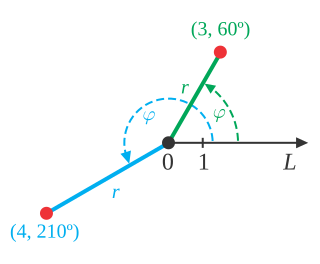
In mathematics, the polar coordinate system is a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction. The reference point is called the pole, and the ray from the pole in the reference direction is the polar axis. The distance from the pole is called the radial coordinate, radial distance or simply radius, and the angle is called the angular coordinate, polar angle, or azimuth. Angles in polar notation are generally expressed in either degrees or radians.

In mathematics, a spherical coordinate system is a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the radial distance of that point from a fixed origin, its polar angle measured from a fixed zenith direction, and the azimuthal angle of its orthogonal projection on a reference plane that passes through the origin and is orthogonal to the zenith, measured from a fixed reference direction on that plane. It can be seen as the three-dimensional version of the polar coordinate system.
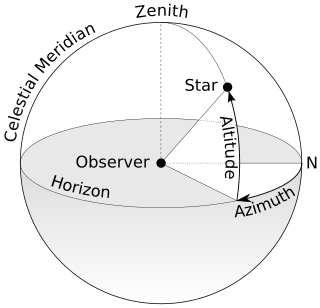
An azimuth is an angular measurement in a spherical coordinate system. The vector from an observer (origin) to a point of interest is projected perpendicularly onto a reference plane; the angle between the projected vector and a reference vector on the reference plane is called the azimuth.

The horizontal coordinate system is a celestial coordinate system that uses the observer's local horizon as the fundamental plane to define two angles: altitude and azimuth. Therefore, the horizontal coordinate system is sometimes called as the az/el system, the alt/az system, or the alt-azimuth system, among others. In the telescope altazimuth mount, the instrument's two axes follow altitude and azimuth.

In cartography, a map projection is a way to flatten a globe's surface into a plane in order to make a map. This requires a systematic transformation of the latitudes and longitudes of locations from the surface of the globe into locations on a plane.

In mathematics, a unit vector in a normed vector space is a vector of length 1. A unit vector is often denoted by a lowercase letter with a circumflex, or "hat", as in .
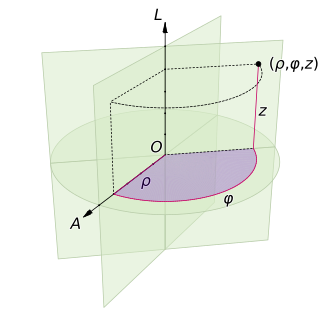
A cylindrical coordinate system is a three-dimensional coordinate system that specifies point positions by the distance from a chosen reference axis (axis L in the image opposite), the direction from the axis relative to a chosen reference direction (axis A), and the distance from a chosen reference plane perpendicular to the axis (plane containing the purple section). The latter distance is given as a positive or negative number depending on which side of the reference plane faces the point.
ISO 9241 is a multi-part standard from the International Organization for Standardization (ISO) covering ergonomics of human-computer interaction. It is managed by the ISO Technical Committee 159. It was originally titled Ergonomic requirements for office work with visual display terminals (VDTs). From 2006 on, the standards were retitled to the more generic Ergonomics of Human System Interaction.
In radiometry, radiance is the radiant flux emitted, reflected, transmitted or received by a given surface, per unit solid angle per unit projected area. Spectral radiance is the radiance of a surface per unit frequency or wavelength, depending on whether the spectrum is taken as a function of frequency or of wavelength. These are directional quantities. The SI unit of radiance is the watt per steradian per square metre, while that of spectral radiance in frequency is the watt per steradian per square metre per hertz and that of spectral radiance in wavelength is the watt per steradian per square metre per metre —commonly the watt per steradian per square metre per nanometre. The microflick is also used to measure spectral radiance in some fields. Radiance is used to characterize diffuse emission and reflection of electromagnetic radiation, or to quantify emission of neutrinos and other particles. Historically, radiance is called "intensity" and spectral radiance is called "specific intensity". Many fields still use this nomenclature. It is especially dominant in heat transfer, astrophysics and astronomy. "Intensity" has many other meanings in physics, with the most common being power per unit area.

The azimuthal equidistant projection is an azimuthal map projection. It has the useful properties that all points on the map are at proportionally correct distances from the center point, and that all points on the map are at the correct azimuth (direction) from the center point. A useful application for this type of projection is a polar projection which shows all meridians as straight, with distances from the pole represented correctly. The flag of the United Nations contains an example of a polar azimuthal equidistant projection.
In display technology parlance, viewing angle is the angle at which a display can be viewed with acceptable visual performance. In a technical context, the angular range is called viewing cone defined by a multitude of viewing directions. The viewing angle can be an angular range over which the display view is acceptable, or it can be the angle of generally acceptable viewing, such as a twelve o'clock viewing angle for a display optimized or viewing from the top.

The Lambert azimuthal equal-area projection is a particular mapping from a sphere to a disk. It accurately represents area in all regions of the sphere, but it does not accurately represent angles. It is named for the Swiss mathematician Johann Heinrich Lambert, who announced it in 1772. "Zenithal" being synonymous with "azimuthal", the projection is also known as the Lambert zenithal equal-area projection.

In electromagnetics, directivity is a parameter of an antenna or optical system which measures the degree to which the radiation emitted is concentrated in a single direction. It measures the power density the antenna radiates in the direction of its strongest emission, versus the power density radiated by an ideal isotropic radiator radiating the same total power.
In mathematics and physics, vector notation is a commonly used notation for representing vectors, which may be Euclidean vectors, or more generally, members of a vector space.
ISO 13406-2 is an ISO standard, with the full title "Ergonomic requirements for work with visual displays based on flat panels -- Part 2: Ergonomic requirements for flat panel displays". It is best known to end consumers for defining a series of flat-panel display "classes" with different numbers of permitted defects. ISO 13406-2 also provides a classification of Viewing Direction Range Classes and Reflection Classes.
Contrast in visual perception is the difference in appearance of two or more parts of a field seen simultaneously or successively.
Conoscopy is an optical technique to make observations of a transparent specimen in a cone of converging rays of light. The various directions of light propagation are observable simultaneously.

In technical drawing and computer graphics, a multiview projection is a technique of illustration by which a standardized series of orthographic two-dimensional pictures are constructed to represent the form of a three-dimensional object. Up to six pictures of an object are produced, with each projection plane parallel to one of the coordinate axes of the object. The views are positioned relative to each other according to either of two schemes: first-angle or third-angle projection. In each, the appearances of views may be thought of as being projected onto planes that form a six-sided box around the object. Although six different sides can be drawn, usually three views of a drawing give enough information to make a three-dimensional object. These views are known as front view, top view and end view. Other names for these views include plan, elevation and section. When the plane or axis of the object depicted is not parallel to the projection plane, and where multiple sides of an object are visible in the same image, it is called an auxiliary view.