
Euclidean geometry is a mathematical system attributed to ancient Greek mathematician Euclid, which he described in his textbook on geometry, Elements. Euclid's approach consists in assuming a small set of intuitively appealing axioms (postulates) and deducing many other propositions (theorems) from these. Although many of Euclid's results had been stated earlier, Euclid was the first to organize these propositions into a logical system in which each result is proved from axioms and previously proved theorems.

In geometry, straightedge-and-compass construction – also known as ruler-and-compass construction, Euclidean construction, or classical construction – is the construction of lengths, angles, and other geometric figures using only an idealized ruler and a pair of compasses.

A ruler, sometimes called a rule, line gauge, or scale, is an instrument used to make length measurements, whereby a user estimates a length by reading from a series of markings called "rules" along an edge of the device. Commonly the instrument is rigid and the edge itself is a straightedge which additionally allows one to draw straight lines. Some rulers, such as cloth or paper tape measures, are non-rigid. Specialty rulers exist that have flexible edges that retain a chosen shape; these find use in sewing, arts, and crafts.

In elementary geometry, two geometric objects are perpendicular if their intersection forms right angles at the point of intersection called a foot. The condition of perpendicularity may be represented graphically using the perpendicular symbol, ⟂. Perpendicular intersections can happen between two lines, between a line and a plane, and between two planes.

Doubling the cube, also known as the Delian problem, is an ancient geometric problem. Given the edge of a cube, the problem requires the construction of the edge of a second cube whose volume is double that of the first. As with the related problems of squaring the circle and trisecting the angle, doubling the cube is now known to be impossible to construct by using only a compass and straightedge, but even in ancient times solutions were known that employed other tools.

Angle trisection is a classical problem of straightedge and compass construction of ancient Greek mathematics. It concerns construction of an angle equal to one third of a given arbitrary angle, using only two tools: an unmarked straightedge and a compass.
The Huzita–Justin axioms or Huzita–Hatori axioms are a set of rules related to the mathematical principles of origami, describing the operations that can be made when folding a piece of paper. The axioms assume that the operations are completed on a plane, and that all folds are linear. These are not a minimal set of axioms but rather the complete set of possible single folds.
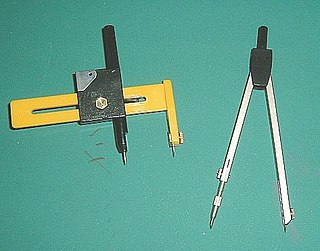
A compass, more accurately known as a pair of compasses, is a technical drawing instrument that can be used for inscribing circles or arcs. As dividers, it can also be used as a tool to mark out distances, in particular, on maps. Compasses can be used for mathematics, drafting, navigation and other purposes.

In the branch of mathematics known as Euclidean geometry, the Poncelet–Steiner theorem is one of several results concerning compass and straightedge constructions having additional restrictions imposed on the traditional rules. This result states that whatever can be constructed by straightedge and compass together can be constructed by straightedge alone, provided that a single circle and its centre are given. This theorem is related to the rusty compass equivalence.

In geometry, the cissoid of Diocles is a cubic plane curve notable for the property that it can be used to construct two mean proportionals to a given ratio. In particular, it can be used to double a cube. It can be defined as the cissoid of a circle and a line tangent to it with respect to the point on the circle opposite to the point of tangency. In fact, the curve family of cissoids is named for this example and some authors refer to it simply as the cissoid. It has a single cusp at the pole, and is symmetric about the diameter of the circle which is the line of tangency of the cusp. The line is an asymptote. It is a member of the conchoid of de Sluze family of curves and in form it resembles a tractrix.
In mathematics, the Mohr–Mascheroni theorem states that any geometric construction that can be performed by a compass and straightedge can be performed by a compass alone.

In Euclidean plane geometry, Apollonius's problem is to construct circles that are tangent to three given circles in a plane (Figure 1). Apollonius of Perga posed and solved this famous problem in his work Ἐπαφαί ; this work has been lost, but a 4th-century AD report of his results by Pappus of Alexandria has survived. Three given circles generically have eight different circles that are tangent to them (Figure 2), a pair of solutions for each way to divide the three given circles in two subsets.
In geometry, a quadratrix is a curve having ordinates which are a measure of the area of another curve. The two most famous curves of this class are those of Dinostratus and E. W. Tschirnhaus, which are both related to the circle.

The coastline paradox is the counterintuitive observation that the coastline of a landmass does not have a well-defined length. This results from the fractal curve–like properties of coastlines; i.e., the fact that a coastline typically has a fractal dimension. Although the "paradox of length" was previously noted by Hugo Steinhaus, the first systematic study of this phenomenon was by Lewis Fry Richardson, and it was expanded upon by Benoit Mandelbrot.

In hyperbolic geometry, a horocycle, sometimes called an oricycle, oricircle, or limit circle, is a curve whose normal or perpendicular geodesics all converge asymptotically in the same direction. It is the two-dimensional case of a horosphere.
In geometry, the compass equivalence theorem is an important statement in compass and straightedge constructions. The tool advocated by Plato in these constructions is a divider or collapsing compass, that is, a compass that "collapses" whenever it is lifted from a page, so that it may not be directly used to transfer distances. The modern compass with its fixable aperture can be used to transfer distances directly and so appears to be a more powerful instrument. However, the compass equivalence theorem states that any construction via a "modern compass" may be attained with a collapsing compass. This can be shown by establishing that with a collapsing compass, given a circle in the plane, it is possible to construct another circle of equal radius, centered at any given point on the plane. This theorem is Proposition II of Book I of Euclid's Elements. The proof of this theorem has had a chequered history.
This is a timeline of mathematicians in ancient Greece.
In Euclidean geometry, Apollonius' problem is to construct all the circles that are tangent to three given circles. Special cases of Apollonius' problem are those in which at least one of the given circles is a point or line, i.e., is a circle of zero or infinite radius. The nine types of such limiting cases of Apollonius' problem are to construct the circles tangent to:
- three points
- three lines
- one line and two points
- two lines and a point
- one circle and two points
- one circle, one line, and a point
- two circles and a point
- one circle and two lines
- two circles and a line

Drafting tools may be used for measurement and layout of drawings, or to improve the consistency and speed of creation of standard drawing elements. Tools such as pens and pencils mark the drawing medium. Other tools such as straight edges, assist the operator in drawing straight lines, or assist the operator in drawing complicated shapes repeatedly. Various scales and the protractor are used to measure the lengths of lines and angles, allowing accurate scale drawing to be carried out. The compass is used to draw arcs and circles. A drawing board was used to hold the drawing media in place; later boards included drafting machines that sped the layout of straight lines and angles. Tools such as templates and lettering guides assisted in the drawing of repetitive elements such as circles, ellipses, schematic symbols and text. Other auxiliary tools were used for special drawing purposes or for functions related to the preparation and revision of drawings. The tools used for manual technical drawing have been displaced by the advent of computer-aided drawing, drafting and design (CADD).
A schema for horizontal dials is a set of instructions used to construct horizontal sundials using compass and straightedge construction techniques, which were widely used in Europe from the late fifteenth century to the late nineteenth century. The common horizontal sundial is a geometric projection of an equatorial sundial onto a horizontal plane.














