Validity (logic)

Last updated July 27, 2024

In logic, specifically in deductive reasoning, an argument is valid if and only if it takes a form that makes it impossible for the premises to be true and the conclusion nevertheless to be false.^[1] It is not required for a valid argument to have premises that are actually true,^[2] but to have premises that, if they were true, would guarantee the truth of the argument's conclusion. Valid arguments must be clearly expressed by means of sentences called well-formed formulas (also called wffs or simply formulas).

Arguments

In logic, an argument is a set of related statements expressing the premises (which may consists of non-emperical evidence, empirical evidence or may contain some axiomatic truths) and an necessary conclusion based on the relationship of the premises.

An argument is valid if and only if it would be contradictory for the conclusion to be false if all of the premises are true.^[3] Validity does not require the truth of the premises, instead it merely necessitates that conclusion follows from the premises without violating the correctness of the logical form. If also the premises of a valid argument are proven true, this is said to be sound.^[3]

The corresponding conditional of a valid argument is a logical truth and the negation of its corresponding conditional is a contradiction. The conclusion is a necessary consequence of its premises.

An argument that is not valid is said to be "invalid".

An example of a valid (and sound) argument is given by the following well-known syllogism:

All men are mortal. (True)

Socrates is a man. (True)

Therefore, Socrates is mortal. (True)

What makes this a valid argument is not that it has true premises and a true conclusion. Validity is about the tie in relationship between the two premises the necessity of the conclusion. There needs to be a relationship established between the premises i.e., a middle term between the premises. If you just have two unrelated premises there is no argument. Notice some of the terms repeat: men is a variation man in premises one and two, Socrates and the term mortal repeats in the conclusion. The argument would be just as valid if both premises and conclusion were false. The following argument is of the same logical form but with false premises and a false conclusion, and it is equally valid:

All cups are green. (False)

Socrates is a cup. (False)

Therefore, Socrates is green. (False)

No matter how the universe might be constructed, it could never be the case that these arguments should turn out to have simultaneously true premises but a false conclusion. The above arguments may be contrasted with the following invalid one:

All men are immortal. (False)

Socrates is a man. (True)

Therefore, Socrates is mortal. (True)

In this case, the conclusion contradicts the deductive logic of the preceding premises, rather than deriving from it. Therefore, the argument is logically 'invalid', even though the conclusion could be considered 'true' in general terms. The premise 'All men are immortal' would likewise be deemed false outside of the framework of classical logic. However, within that system 'true' and 'false' essentially function more like mathematical states such as binary 1s and 0s than the philosophical concepts normally associated with those terms. Formal arguments that are invalid are often associated with at least one fallacy which should be verifiable.

A standard view is that whether an argument is valid is a matter of the argument's logical form. Many techniques are employed by logicians to represent an argument's logical form. A simple example, applied to two of the above illustrations, is the following: Let the letters 'P', 'Q', and 'S' stand, respectively, for the set of men, the set of mortals, and Socrates. Using these symbols, the first argument may be abbreviated as:

All P are Q.

S is a P.

Therefore, S is a Q.

Similarly, the third argument becomes:

All P's are not Q.

S is a P.

Therefore, S is a Q.

An argument is termed formally valid if it has structural self-consistency, i.e. if when the operands between premises are all true, the derived conclusion is always also true. In the third example, the initial premises cannot logically result in the conclusion and is therefore categorized as an invalid argument.

Valid formula

A formula of a formal language is a valid formula if and only if it is true under every possible interpretation of the language. In propositional logic, they are tautologies.

Statements

A statement can be called valid, i.e. logical truth, in some systems of logic like in Modal logic if the statement is true in all interpretations. In Aristotelian logic statements are not valid per se. Validity refers to entire arguments. The same is true in propositional logic (statements can be true or false but not called valid or invalid).

Soundness

Validity of deduction is not affected by the truth of the premise or the truth of the conclusion. The following deduction is perfectly valid:

All animals live on Mars. (False)

All humans are animals. (True)

Therefore, all humans live on Mars. (False)

The problem with the argument is that it is not sound. In order for a deductive argument to be sound, the argument must be valid and all the premises must be true.^[3]

Satisfiability

Model theory analyzes formulae with respect to particular classes of interpretation in suitable mathematical structures. On this reading, formula is valid if all such interpretations make it true. An inference is valid if all interpretations that validate the premises validate the conclusion. This is known as semantic validity.^[4]

Preservation

In truth-preserving validity, the interpretation under which all variables are assigned a truth value of 'true' produces a truth value of 'true'.

In a false-preserving validity, the interpretation under which all variables are assigned a truth value of 'false' produces a truth value of 'false'.^[5]

Preservation properties	Logical connective sentences
True and false preserving:	Proposition • Logical conjunction (AND, $\land$ ) • Logical disjunction (OR, $\lor$ )
True preserving only:	Tautology ( $\top$ ) • Biconditional (XNOR, $\leftrightarrow$ ) • Implication ( $\rightarrow$ ) • Converse implication ( $\leftarrow$ )
False preserving only:	Contradiction ( $\bot$ ) • Exclusive disjunction (XOR, $\oplus$ ) • Nonimplication ( $\nrightarrow$ ) • Converse nonimplication ( $\nleftarrow$ )
Non-preserving:	Negation ( $\neg$ ) • Alternative denial (NAND, $\uparrow$ ) • Joint denial (NOR, $\downarrow$ )

Related Research Articles

A false dilemma, also referred to as false dichotomy or false binary, is an informal fallacy based on a premise that erroneously limits what options are available. The source of the fallacy lies not in an invalid form of inference but in a false premise. This premise has the form of a disjunctive claim: it asserts that one among a number of alternatives must be true. This disjunction is problematic because it oversimplifies the choice by excluding viable alternatives, presenting the viewer with only two absolute choices when, in fact, there could be many.

The propositional calculus is a branch of logic. It is also called propositional logic, statement logic, sentential calculus, sentential logic, or sometimes zeroth-order logic. It deals with propositions and relations between propositions, including the construction of arguments based on them. Compound propositions are formed by connecting propositions by logical connectives representing the truth functions of conjunction, disjunction, implication, biconditional, and negation. Some sources include other connectives, as in the table below.

In propositional logic, modus ponens, also known as modus ponendo ponens, implication elimination, or affirming the antecedent, is a deductive argument form and rule of inference. It can be summarized as "P implies Q.P is true. Therefore, Q must also be true."

In propositional logic, modus tollens (MT), also known as modus tollendo tollens and denying the consequent, is a deductive argument form and a rule of inference. Modus tollens is a mixed hypothetical syllogism that takes the form of "If P, then Q. Not Q. Therefore, not P." It is an application of the general truth that if a statement is true, then so is its contrapositive. The form shows that inference from P implies Q to the negation of Q implies the negation of P is a valid argument.

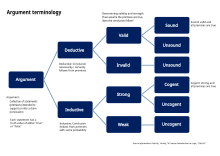
In logic and deductive reasoning, an argument is sound if it is both valid in form and has no false premises. Soundness has a related meaning in mathematical logic, wherein a formal system of logic is sound if and only if every well-formed formula that can be proven in the system is logically valid with respect to the logical semantics of the system.

A syllogism is a kind of logical argument that applies deductive reasoning to arrive at a conclusion based on two propositions that are asserted or assumed to be true.

A fallacy is the use of invalid or otherwise faulty reasoning in the construction of an argument that may appear to be well-reasoned if unnoticed. The term was introduced in the Western intellectual tradition by the Aristotelian De Sophisticis Elenchis.

Deductive reasoning is the process of drawing valid inferences. An inference is valid if its conclusion follows logically from its premises, meaning that it is impossible for the premises to be true and the conclusion to be false.

Inferences are steps in reasoning, moving from premises to logical consequences; etymologically, the word infer means to "carry forward". Inference is theoretically traditionally divided into deduction and induction, a distinction that in Europe dates at least to Aristotle. Deduction is inference deriving logical conclusions from premises known or assumed to be true, with the laws of valid inference being studied in logic. Induction is inference from particular evidence to a universal conclusion. A third type of inference is sometimes distinguished, notably by Charles Sanders Peirce, contradistinguishing abduction from induction.

Inductive reasoning is any of various methods of reasoning in which broad generalizations or principles are derived from a body of observations. This article is concerned with the inductive reasoning other than deductive reasoning, where the conclusion of a deductive argument is certain given the premises are correct; in contrast, the truth of the conclusion of an inductive argument is at best probable, based upon the evidence given.

Logical reasoning is a mental activity that aims to arrive at a conclusion in a rigorous way. It happens in the form of inferences or arguments by starting from a set of premises and reasoning to a conclusion supported by these premises. The premises and the conclusion are propositions, i.e. true or false claims about what is the case. Together, they form an argument. Logical reasoning is norm-governed in the sense that it aims to formulate correct arguments that any rational person would find convincing. The main discipline studying logical reasoning is logic.

Informal fallacies are a type of incorrect argument in natural language. The source of the error is not just due to the form of the argument, as is the case for formal fallacies, but can also be due to their content and context. Fallacies, despite being incorrect, usually appear to be correct and thereby can seduce people into accepting and using them. These misleading appearances are often connected to various aspects of natural language, such as ambiguous or vague expressions, or the assumption of implicit premises instead of making them explicit.

In logic, the logical form of a statement is a precisely-specified semantic version of that statement in a formal system. Informally, the logical form attempts to formalize a possibly ambiguous statement into a statement with a precise, unambiguous logical interpretation with respect to a formal system. In an ideal formal language, the meaning of a logical form can be determined unambiguously from syntax alone. Logical forms are semantic, not syntactic constructs; therefore, there may be more than one string that represents the same logical form in a given language.

In logic and philosophy, a formal fallacy, deductive fallacy, logical fallacy or non sequitur is a pattern of reasoning rendered invalid by a flaw in its logical structure that can neatly be expressed in a standard logic system, for example propositional logic. It is defined as a deductive argument that is invalid. The argument itself could have true premises, but still have a false conclusion. Thus, a formal fallacy is a fallacy in which deduction goes wrong, and is no longer a logical process. This may not affect the truth of the conclusion, since validity and truth are separate in formal logic.

A premise or premiss is a proposition—a true or false declarative statement—used in an argument to prove the truth of another proposition called the conclusion. Arguments consist of a set of premises and a conclusion.

An argument is a series of sentences, statements, or propositions some of which are called premises and one is the conclusion. The purpose of an argument is to give reasons for one's conclusion via justification, explanation, and/or persuasion.

Philosophy of logic is the area of philosophy that studies the scope and nature of logic. It investigates the philosophical problems raised by logic, such as the presuppositions often implicitly at work in theories of logic and in their application. This involves questions about how logic is to be defined and how different logical systems are connected to each other. It includes the study of the nature of the fundamental concepts used by logic and the relation of logic to other disciplines. According to a common characterisation, philosophical logic is the part of the philosophy of logic that studies the application of logical methods to philosophical problems, often in the form of extended logical systems like modal logic. But other theorists draw the distinction between the philosophy of logic and philosophical logic differently or not at all. Metalogic is closely related to the philosophy of logic as the discipline investigating the properties of formal logical systems, like consistency and completeness.

Logical consequence is a fundamental concept in logic which describes the relationship between statements that hold true when one statement logically follows from one or more statements. A valid logical argument is one in which the conclusion is entailed by the premises, because the conclusion is the consequence of the premises. The philosophical analysis of logical consequence involves the questions: In what sense does a conclusion follow from its premises? and What does it mean for a conclusion to be a consequence of premises? All of philosophical logic is meant to provide accounts of the nature of logical consequence and the nature of logical truth.

Logic is the study of correct reasoning. It includes both formal and informal logic. Formal logic is the study of deductively valid inferences or logical truths. It examines how conclusions follow from premises due to the structure of arguments alone, independent of their topic and content. Informal logic is associated with informal fallacies, critical thinking, and argumentation theory. Informal logic examines arguments expressed in natural language while formal logic uses formal language. When used as a countable noun, the term "a logic" refers to a logical formal system that articulates a proof system. Logic plays a central role in many fields, such as philosophy, mathematics, computer science, and linguistics.

This is a glossary of logic. Logic is the study of the principles of valid reasoning and argumentation.

References

↑ Validity and Soundness – Internet Encyclopedia of Philosophy
↑ Jc Beall and Greg Restall, "Logical Consequence", The Stanford Encyclopedia of Philosophy (Fall 2014 Edition).
1 2 3 4 Gensler, Harry J. (January 6, 2017). Introduction to logic (Third ed.). New York: Routledge. ISBN 978-1-138-91058-4. OCLC 957680480.
↑ L. T. F. Gamut, Logic, Language, and Meaning: Introduction to Logic, University of Chicago Press, 1991, p. 115.
↑ Robert Cogan, Critical Thinking: Step by Step, University Press of America, 1998, p. 48.