In music, just intonation or pure intonation is the tuning of musical intervals as whole number ratios of frequencies. An interval tuned in this way is said to be pure, and is called a just interval. Just intervals consist of tones from a single harmonic series of an implied fundamental. For example, in the diagram, if the notes G3 and C4 are tuned as members of the harmonic series of the lowest C, their frequencies will be 3 and 4 times the fundamental frequency. The interval ratio between C4 and G3 is therefore 4:3, a just fourth.
In music, there are two common meanings for tuning:
The piano is a keyboard instrument that produces sound when the keys are pressed. Most modern pianos have a row of 88 black and white keys: 52 white keys for the notes of the C major scale and 36 shorter black keys raised above the white keys and set further back, for sharps and flats. This means that the piano can play 88 different pitches, spanning a range of a bit over seven octaves. The black keys are for the "accidentals", which are needed to play in all twelve keys.
The violin, colloquially known as a fiddle, is a wooden chordophone, and is the smallest, and thus highest-pitched instrument (soprano) in regular use in the violin family. Smaller violin-type instruments exist, including the violino piccolo and the pochette, but these are virtually unused. Most violins have a hollow wooden body, and commonly have four strings, usually tuned in perfect fifths with notes G3, D4, A4, E5, and are most commonly played by drawing a bow across the strings. The violin can also be played by plucking the strings with the fingers (pizzicato) and, in specialized cases, by striking the strings with the wooden side of the bow.
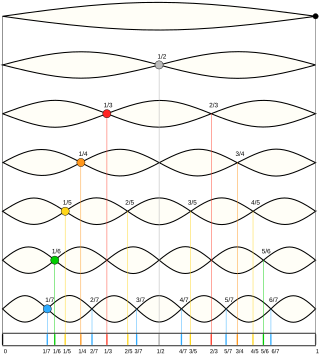
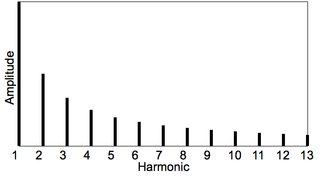
In physics, acoustics, and telecommunications, a harmonic is a sinusoidal wave with a frequency that is a positive integer multiple of the fundamental frequency of a periodic signal. The fundamental frequency is also called the 1st harmonic; the other harmonics are known as higher harmonics. As all harmonics are periodic at the fundamental frequency, the sum of harmonics is also periodic at that frequency. The set of harmonics forms a harmonic series.
In musical instrument classification, string instruments or chordophones, are musical instruments that produce sound from vibrating strings when a performer plays or sounds the strings in some manner.
Piano acoustics is the set of physical properties of the piano that affect its sound. It is an area of study within musical acoustics.
Piano tuning is the act of adjusting the tension of the strings of an acoustic piano so that the musical intervals between strings are in tune. The meaning of the term 'in tune', in the context of piano tuning, is not simply a particular fixed set of pitches. Fine piano tuning requires an assessment of the vibration interaction among notes, which is different for every piano, thus in practice requiring slightly different pitches from any theoretical standard. Pianos are usually tuned to a modified version of the system called equal temperament.
According to legend, Pythagoras discovered the foundations of musical tuning by listening to the sounds of four blacksmith's hammers, which produced consonance and dissonance when they were struck simultaneously. According to Nicomachus in his 2nd-century CE Enchiridion harmonices, Pythagoras noticed that hammer A produced consonance with hammer B when they were struck together, and hammer C produced consonance with hammer A, but hammers B and C produced dissonance with each other. Hammer D produced such perfect consonance with hammer A that they seemed to be "singing" the same note. Pythagoras rushed into the blacksmith shop to discover why, and found that the explanation was in the weight ratios. The hammers weighed 12, 9, 8, and 6 pounds respectively. Hammers A and D were in a ratio of 2:1, which is the ratio of the octave. Hammers B and C weighed 8 and 9 pounds. Their ratios with hammer D were and. The space between B and C is a ratio of 9:8, which is equal to the musical whole tone, or whole step interval.
Stretched tuning is a detail of musical tuning, applied to wire-stringed musical instruments, older, non-digital electric pianos, and some sample-based synthesizers based on these instruments, to accommodate the natural inharmonicity of their vibrating elements. In stretched tuning, two notes an octave apart, whose fundamental frequencies theoretically have an exact 2:1 ratio, are tuned slightly farther apart. "For a stretched tuning the octave is greater than a factor of 2; for a compressed tuning the octave is smaller than a factor of 2."
12 equal temperament (12-ET) is the musical system that divides the octave into 12 parts, all of which are equally tempered on a logarithmic scale, with a ratio equal to the 12th root of 2. That resulting smallest interval, 1⁄12 the width of an octave, is called a semitone or half step.
Music theory analyzes the pitch, timing, and structure of music. It uses mathematics to study elements of music such as tempo, chord progression, form, and meter. The attempt to structure and communicate new ways of composing and hearing music has led to musical applications of set theory, abstract algebra and number theory.
In musical tuning, a temperament is a tuning system that slightly compromises the pure intervals of just intonation to meet other requirements. Most modern Western musical instruments are tuned in the equal temperament system. Tempering is the process of altering the size of an interval by making it narrower or wider than pure. "Any plan that describes the adjustments to the sizes of some or all of the twelve fifth intervals in the circle of fifths so that they accommodate pure octaves and produce certain sizes of major thirds is called a temperament." Temperament is especially important for keyboard instruments, which typically allow a player to play only the pitches assigned to the various keys, and lack any way to alter pitch of a note in performance. Historically, the use of just intonation, Pythagorean tuning and meantone temperament meant that such instruments could sound "in tune" in one key, or some keys, but would then have more dissonance in other keys.
Werckmeister temperaments are the tuning systems described by Andreas Werckmeister in his writings. The tuning systems are numbered in two different ways: the first refers to the order in which they were presented as "good temperaments" in Werckmeister's 1691 treatise, the second to their labelling on his monochord. The monochord labels start from III since just intonation is labelled I and quarter-comma meantone is labelled II. The temperament commonly known as "Werckmeister III" is referred to in this article as "Werckmeister I (III)".
In music, the undertone series or subharmonic series is a sequence of notes that results from inverting the intervals of the overtone series. While overtones naturally occur with the physical production of music on instruments, undertones must be produced in unusual ways. While the overtone series is based upon arithmetic multiplication of frequencies, resulting in a harmonic series, the undertone series is based on arithmetic division.
The Moodswinger is a twelve-string electric zither with an additional third bridge designed by Yuri Landman. The rod which functions as the third bridge divides the strings into two sections to cause an overtone multiphonic sound. One of the copies of the instrument is part of the collection of the Musical Instrument Museum in Phoenix, Arizona.
Mersenne's laws are laws describing the frequency of oscillation of a stretched string or monochord, useful in musical tuning and musical instrument construction.
The 3rd bridge is an extended playing technique used on the electric guitar and other string instruments that allows a musician to produce distinctive timbres and overtones that are unavailable on a conventional string instrument with two bridges. The timbre created with this technique is close to that of gamelan instruments like the bonang and similar Indonesian types of pitched gongs.
A third bridge can be devised by inserting a rigid preparation object between the strings and the body or neck of the instrument, effectively dividing the string into distinct vibrating segments.
The scale of harmonics is a musical scale based on the noded positions of the natural harmonics existing on a string. This musical scale is present on the guqin, regarded as one of the first string instruments with a musical scale. Most fret positions appearing on Non-Western string instruments (lutes) are equal to positions of this scale. Unexpectedly, these fret positions are actually the corresponding undertones of the overtones from the harmonic series. The distance from the nut to the fret is an integer number lower than the distance from the fret to the bridge.
In music, an interval ratio is a ratio of the frequencies of the pitches in a musical interval. For example, a just perfect fifth is 3:2, 1.5, and may be approximated by an equal tempered perfect fifth which is 27/12. If the A above middle C is 440 Hz, the perfect fifth above it would be E, at (440*1.5=) 660 Hz, while the equal tempered E5 is 659.255 Hz.
