294 (number)

Last updated
← 293 294 295 →
Cardinal two hundred ninety-four
Ordinal 294th
(two hundred ninety-fourth)
Factorization 2 × 3 × 72
Divisors 1, 2, 3, 6, 7,14, 21, 42, 49, 98, 147, 294
Greek numeral ΣϞΔ´
Roman numeral CCXCIV
Binary 1001001102
Ternary 1012203
Senary 12106
Octal 4468
Duodecimal 20612
Hexadecimal 12616

294 is the natural number following 293 and preceding 295.

Contents

In mathematics

In technology

World Records

Other fields

Related Research Articles

In theoretical computer science, the subgraph isomorphism problem is a computational task in which two graphs G and H are given as input, and one must determine whether G contains a subgraph that is isomorphic to H. Subgraph isomorphism is a generalization of both the maximum clique problem and the problem of testing whether a graph contains a Hamiltonian cycle, and is therefore NP-complete. However certain other cases of subgraph isomorphism may be solved in polynomial time.

<span class="mw-page-title-main">Vertex (graph theory)</span> Fundamental unit of which graphs are formed

In discrete mathematics, and more specifically in graph theory, a vertex or node is the fundamental unit of which graphs are formed: an undirected graph consists of a set of vertices and a set of edges, while a directed graph consists of a set of vertices and a set of arcs. In a diagram of a graph, a vertex is usually represented by a circle with a label, and an edge is represented by a line or arrow extending from one vertex to another.

In the mathematical discipline of graph theory, the line graph of an undirected graph G is another graph L(G) that represents the adjacencies between edges of G. L(G) is constructed in the following way: for each edge in G, make a vertex in L(G); for every two edges in G that have a vertex in common, make an edge between their corresponding vertices in L(G).

<span class="mw-page-title-main">Clebsch graph</span> One of two different regular graphs with 16 vertices

In the mathematical field of graph theory, the Clebsch graph is either of two complementary graphs on 16 vertices, a 5-regular graph with 40 edges and a 10-regular graph with 80 edges. The 80-edge graph is the dimension-5 halved cube graph; it was called the Clebsch graph name by Seidel (1968) because of its relation to the configuration of 16 lines on the quartic surface discovered in 1868 by the German mathematician Alfred Clebsch. The 40-edge variant is the dimension-5 folded cube graph; it is also known as the Greenwood–Gleason graph after the work of Robert E. Greenwood and Andrew M. Gleason, who used it to evaluate the Ramsey number R(3,3,3) = 17.

<span class="mw-page-title-main">Tutte 12-cage</span>

In the mathematical field of graph theory, the Tutte 12-cage or Benson graph is a 3-regular graph with 126 vertices and 189 edges. It is named after W. T. Tutte.

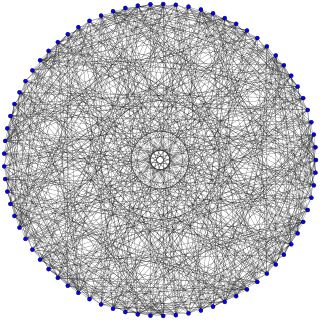
M<sub>22</sub> graph Strongly regular graph

The M22 graph, also called the Mesner graph or Witt graph is the unique strongly regular graph with parameters (77, 16, 0, 4). It is constructed from the Steiner system (3, 6, 22) by representing its 77 blocks as vertices and joining two vertices iff they have no terms in common or by deleting a vertex and its neighbors from the Higman–Sims graph.

265 is the natural number following 264 and preceding 266.

285 is the natural number following 284 and preceding 286.

293 is the natural number following 292 and preceding 294.

282 is the natural number following 281 and preceding 283.

268 is the natural number following 267 and preceding 269.

275 is the natural number following 274 and preceding 276.

278 is the natural number following 277 and preceding 279.

284 is the natural number following 283 and preceding 285.

286 is the natural number following 285 and preceding 287.

287 is the natural number following 286 and preceding 288.

292 is the natural number following 291 and preceding 293.

295 is the natural number following 294 and preceding 296.

296 is the natural number following 295 and preceding 297.

297 is the natural number following 296 and preceding 298.

References

  1. ↑ "Facts about the integer". mathworld.wolfram.com.
  2. ↑ "Biconnected graph information". mathworld.wolfram.com.
  3. ↑ Sarcone, Gianni (December 20, 2020). "Magic Inscribed Lotus information". archimedes-lab.org.
  4. ↑ "George A. Miller information" (PDF). www.nasonline.org.
  5. ↑ "Isomorphism information". mathworld.wolfram.com.
  6. ↑ "294 area code in Veracruz Mexico". en.codigosinternacionales.com. 27 August 2023.
  7. ↑ Tang, Adrian; Chang, Mau-Chung Frank (2013). A 294 GHZ 0.47mW caterpillar amplifier based transmitter in 65nm CMOS for THz data-links. pp. 46–48. doi:10.1109/RWS.2013.6486636. ISBN   978-1-4673-2932-3. S2CID   18211707.
  8. ↑ "UL Standard 294". www.barcode-automation.com.
  9. ↑ "294 signatures on a t-shirt in one hour". www.guiennessworldrecords.com.
  10. ↑ "294 Beatles impersonators". www.guinnessworldrecods.com.
  11. ↑ "294 military deaths in Afghanistan in 2008". www.guinnessworldrecords.com.
  12. ↑ "294 people in an indoor volleyball exhibition match". www.guinnessworldrecords.com.
  13. ↑ "2.94m martial arts kick". guinnessworldrecords.com.
  14. ↑ "French Republican Calendar Guide" (PDF). broadcast.lds.org.
  15. ↑ "294 Felicia information". in-the-sky.org.
  16. ↑ "Oganesson-294 information". www.acs.org.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.