Horseshoe orbit

Last updated May 30, 2023

In celestial mechanics, a horseshoe orbit is a type of co-orbital motion of a small orbiting body relative to a larger orbiting body. The osculating (instantaneous) orbital period of the smaller body remains very near that of the larger body, and if its orbit is a little more eccentric than that of the larger body, during every period it appears to trace an ellipse around a point on the larger object's orbit. However, the loop is not closed but drifts forward or backward so that the point it circles will appear to move smoothly along the larger body's orbit over a long period of time. When the object approaches the larger body closely at either end of its trajectory, its apparent direction changes. Over an entire cycle the center traces the outline of a horseshoe, with the larger body between the 'horns'.

Asteroids in horseshoe orbits with respect to Earth include 54509 YORP, 2002 AA29 , 2010 SO16 , 2015 SO2 and possibly 2001 GO2 . A broader definition includes 3753 Cruithne, which can be said to be in a compound and/or transition orbit,^[1] or (85770) 1998 UP1 and 2003 YN107 . By 2016, 12 horseshoe librators of Earth have been discovered.^[2]

Saturn's moons Epimetheus and Janus occupy horseshoe orbits with respect to each other (in their case, there is no repeated looping: each one traces a full horseshoe with respect to the other).

Explanation of horseshoe orbital cycle

Background

The following explanation relates to an asteroid which is in such an orbit around the Sun, and is also affected by the Earth.

The asteroid is in almost the same solar orbit as Earth. Both take approximately one year to orbit the Sun.

It is also necessary to grasp two rules of orbit dynamics:

A body closer to the Sun completes an orbit more quickly than a body farther away.
If a body accelerates along its orbit, its orbit moves outwards from the Sun. If it decelerates, the orbital radius decreases.

The horseshoe orbit arises because the gravitational attraction of the Earth changes the shape of the elliptical orbit of the asteroid. The shape changes are very small but result in significant changes relative to the Earth.

The horseshoe becomes apparent only when mapping the movement of the asteroid relative to both the Sun and the Earth. The asteroid always orbits the Sun in the same direction. However, it goes through a cycle of catching up with the Earth and falling behind, so that its movement relative to both the Sun and the Earth traces a shape like the outline of a horseshoe.

Stages of the orbit

Starting at point A, on the inner ring between L₅ and Earth, the satellite is orbiting faster than the Earth and is on its way toward passing between the Earth and the Sun. But Earth's gravity exerts an outward accelerating force, pulling the satellite into a higher orbit which (per Kepler's third law) decreases its angular speed.

When the satellite gets to point B, it is traveling at the same speed as Earth. Earth's gravity is still accelerating the satellite along the orbital path, and continues to pull the satellite into a higher orbit. Eventually, at Point C, the satellite reaches a high and slow enough orbit such that it starts to lag behind Earth. It then spends the next century or more appearing to drift 'backwards' around the orbit when viewed relative to the Earth. Its orbit around the Sun still takes only slightly more than one Earth year. Given enough time, the Earth and the satellite will be on opposite sides of the Sun.

Eventually the satellite comes around to point D where Earth's gravity is now reducing the satellite's orbital velocity. This causes it to fall into a lower orbit, which actually increases the angular speed of the satellite around the Sun. This continues until point E where the satellite's orbit is now lower and faster than Earth's orbit, and it begins moving out ahead of Earth. Over the next few centuries it completes its journey back to point A.

On the longer term, asteroids can transfer between horseshoe orbits and quasi-satellite orbits. Quasi-satellites aren't gravitationally bound to their planet, but appear to circle it in a retrograde direction as they circle the Sun with the same orbital period as the planet. By 2016, orbital calculations showed that four of Earth's horseshoe librators and all five of its then known quasi-satellites repeatedly transfer between horseshoe and quasi-satellite orbits.^[3]

Energy viewpoint

A somewhat different, but equivalent, view of the situation may be noted by considering conservation of energy. It is a theorem of classical mechanics that a body moving in a time-independent potential field will have its total energy, E = T + V, conserved, where E is total energy, T is kinetic energy (always non-negative) and V is potential energy, which is negative. It is apparent then, since V = -GM/R near a gravitating body of mass M and orbital radius R, that seen from a stationary frame, V will be increasing for the region behind M, and decreasing for the region in front of it. However, orbits with lower total energy have shorter periods, and so a body moving slowly on the forward side of a planet will lose energy, fall into a shorter-period orbit, and thus slowly move away, or be "repelled" from it. Bodies moving slowly on the trailing side of the planet will gain energy, rise to a higher, slower, orbit, and thereby fall behind, similarly repelled. Thus a small body can move back and forth between a leading and a trailing position, never approaching too close to the planet that dominates the region.

Tadpole orbit

See also Trojan (celestial body).

Figure 1 above shows shorter orbits around the Lagrangian points L₄ and L₅ (e.g. the lines close to the blue triangles). These are called tadpole orbits and can be explained in a similar way, except that the asteroid's distance from the Earth does not oscillate as far as the L₃ point on the other side of the Sun. As it moves closer to or farther from the Earth, the changing pull of Earth's gravitational field causes it to accelerate or decelerate, causing a change in its orbit known as libration.

An example of a tadpole orbit is Polydeuces, a small moon of Saturn which librates around the trailing L₅ point relative to a larger moon, Dione. In relation to the orbit of Earth, the 300-metre-diameter (980-foot) asteroid 2010 TK7 is in a tadpole orbit around the leading L₄ point. 2020 VT1 follows a temporary horseshoe orbit with respect to Mars.^[4]

Known and suspected companions of Earth
v
t
e
Name	Eccentricity	Diameter (m)	Discoverer	Date of Discovery	Type	Current Type
Moon	0.055	3474800	?	?	Natural satellite	Natural satellite
1913 Great Meteor Procession	?	?	?	1913-02-09	Possible Temporary satellite	Destroyed
3753 Cruithne	0.515	5000	Duncan Waldron	1986-10-10	Quasi-satellite	Horseshoe orbit
1991 VG	0.053	5–12	Spacewatch	1991-11-06	Temporary satellite	Apollo asteroid
(85770) 1998 UP1	0.345	210–470	Lincoln Lab's ETS	1998-10-18	Horseshoe orbit	Horseshoe orbit
54509 YORP	0.230	124	Lincoln Lab's ETS	2000-08-03	Horseshoe orbit	Horseshoe orbit
2001 GO2	0.168	35–85	Lincoln Lab's ETS	2001-04-13	Possible Horseshoe orbit	Possible Horseshoe orbit
2002 AA29	0.013	20–100	LINEAR	2002-01-09	Quasi-satellite	Horseshoe orbit
2003 YN107	0.014	10–30	LINEAR	2003-12-20	Quasi-satellite	Horseshoe orbit
(164207) 2004 GU9	0.136	160–360	LINEAR	2004-04-13	Quasi-satellite	Quasi-satellite
(277810) 2006 FV35	0.377	140–320	Spacewatch	2006-03-29	Quasi-satellite	Quasi-satellite
2006 JY26	0.083	6–13	Catalina Sky Survey	2006-05-06	Horseshoe orbit	Horseshoe orbit
2006 RH120	0.024	2–3	Catalina Sky Survey	2006-09-13	Temporary satellite	Apollo asteroid
(419624) 2010 SO16	0.075	357	WISE	2010-09-17	Horseshoe orbit	Horseshoe orbit
2010 TK7	0.191	150–500	WISE	2010-10-01	Earth trojan	Earth trojan
2013 BS45	0.083	20–40	Spacewatch	2010-01-20	Horseshoe orbit	Horseshoe orbit
2013 LX28	0.452	130–300	Pan-STARRS	2013-06-12	Quasi-satellite temporary	Quasi-satellite temporary
2014 OL339	0.461	70–160	EURONEAR	2014-07-29	Quasi-satellite temporary	Quasi-satellite temporary
2015 SO2	0.108	50–110	Črni Vrh Observatory	2015-09-21	Quasi-satellite	Horseshoe orbit temporary
2015 XX169	0.184	9–22	Mount Lemmon Survey	2015-12-09	Horseshoe orbit temporary	Horseshoe orbit temporary
2015 YA	0.279	9–22	Catalina Sky Survey	2015-12-16	Horseshoe orbit temporary	Horseshoe orbit temporary
2015 YQ1	0.404	7–16	Mount Lemmon Survey	2015-12-19	Horseshoe orbit temporary	Horseshoe orbit temporary
469219 Kamoʻoalewa	0.104	40-100	Pan-STARRS	2016-04-27	Quasi-satellite stable	Quasi-satellite stable
DN16082203	?	?	?	2016-08-22	Possible Temporary satellite	Destroyed
2020 CD3	0.017	1–6	Mount Lemmon Survey	2020-02-15	Temporary satellite	Temporary satellite
2020 PN1	0.127	10–50	ATLAS-HKO	2020-08-12	Horseshoe orbit temporary	Horseshoe orbit temporary
2020 PP1	0.074	10–20	Pan-STARRS	2020-08-12	Quasi-satellite stable	Quasi-satellite stable
2020 XL5	0.387	1100-1260	Pan-STARRS	2020-12-12	Earth trojan	Earth trojan
2022 NX1	0.025	5-15	Moonbase South Observatory	2020-07-02	Temporary satellite	Apollo asteroid
2023 FW13	0.177	10-20	Pan-STARRS	2023-03-28	Quasi-satellite	Quasi-satellite

Related Research Articles

2003 YN₁₀₇ is a tiny asteroid, classified as a near-Earth object of the Aten group moving in a 1:1 mean-motion resonance with Earth. Because of that, it is in a co-orbital configuration relative to Earth.

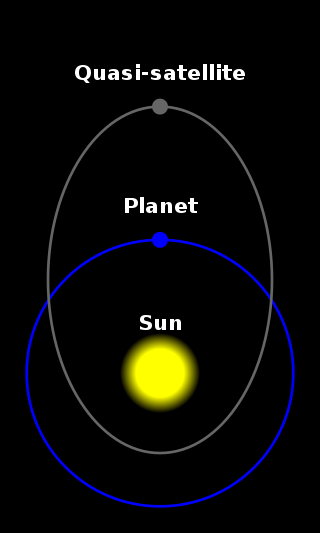
A quasi-satellite is an object in a specific type of co-orbital configuration with a planet where the object stays close to that planet over many orbital periods.

(524522) 2002 VE₆₈, provisional designation 2002 VE₆₈, is a sub-kilometer sized asteroid and temporary quasi-satellite of Venus. It was the first such object to be discovered around a major planet in the Solar System. In a frame of reference rotating with Venus, it appears to travel around it during one Venerean year but it actually orbits the Sun, not Venus.

<span class="mw-page-title-main">Claimed moons of Earth</span> Claims that Earth may have other natural satellites

Claims of the existence of other moons of Earth—that is, of one or more natural satellites with relatively stable orbits of Earth, other than the Moon—have existed for some time. Several candidates have been proposed, but none have been confirmed. Since the 19th century, scientists have made genuine searches for more moons, but the possibility has also been the subject of a number of dubious non-scientific speculations as well as a number of likely hoaxes.

In astronomy, a co-orbital configuration is a configuration of two or more astronomical objects orbiting at the same, or very similar, distance from their primary, i.e. they are in a 1:1 mean-motion resonance..

The Mars trojans are a group of trojan objects that share the orbit of the planet Mars around the Sun. They can be found around the two Lagrangian points 60° ahead of and behind Mars. The origin of the Mars trojans is not well understood. One theory suggests that they were primordial objects left over from the formation of Mars that were captured in its Lagrangian points as the Solar System was forming. However, spectral studies of the Mars trojans indicate this may not be the case. Another explanation involves asteroids chaotically wandering into the Mars Lagrangian points later in the Solar System's formation. This is also questionable considering the short dynamical lifetimes of these objects. The spectra of Eureka and two other Mars trojans indicates an olivine-rich composition. Since olivine-rich objects are rare in the asteroid belt it has been suggested that some of the Mars trojans are captured debris from a large orbit-altering impact on Mars when it encountered a planetary embryo.

54509 YORP, provisional designation 2000 PH₅, is an Earth co-orbital asteroid discovered on 3 August 2000 by the Lincoln Laboratory Near-Earth Asteroid Research (LINEAR) Team at Lincoln Laboratory Experimental Test Site in Socorro, New Mexico. Measurements of the rotation rate of this object provided the first observational evidence of the YORP effect, hence the name of the asteroid. The asteroid's rate of rotation is increasing at the rate of (2.0 ± 0.2) × 10⁻⁴ deg/day² which between 2001 and 2005 caused the asteroid to rotate about 250° further than its spin rate in 2001 would have predicted. Simulations of the asteroid suggest that it may reach a rotation period of ~20 seconds near the end of its expected lifetime, which has a 75% probability of happening within the next 35 million years. The simulations also ruled out the possibility that close encounters with the Earth have been the cause of the increased spin rate.

<span class="mw-page-title-main">Retrograde and prograde motion</span> Relative directions of orbit or rotation

Retrograde motion in astronomy is, in general, orbital or rotational motion of an object in the direction opposite the rotation of its primary, that is, the central object. It may also describe other motions such as precession or nutation of an object's rotational axis. Prograde or direct motion is more normal motion in the same direction as the primary rotates. However, "retrograde" and "prograde" can also refer to an object other than the primary if so described. The direction of rotation is determined by an inertial frame of reference, such as distant fixed stars.

2010 TK₇ is a sub-kilometer Near-Earth asteroid and the first Earth trojan discovered; it precedes Earth in its orbit around the Sun. Trojan objects are most easily conceived as orbiting at a Lagrangian point, a dynamically stable location (where the combined gravitational force acts through the Sun's and Earth's barycenter) 60 degrees ahead of or behind a massive orbiting body, in a type of 1:1 orbital resonance. In reality, they oscillate around such a point. Such objects had previously been observed in the orbits of Mars, Jupiter, Neptune, and the Saturnian moons Tethys and Dione.

Asteroid 2011 QF₉₉ is a minor planet from the outer Solar System and the first known Uranus trojan to be discovered. It measures approximately 60 kilometers (37 miles) in diameter, assuming an albedo of 0.05. It was first observed 29 August 2011 during a deep survey of trans-Neptunian objects conducted with the Canada–France–Hawaii Telescope, but its identification as Uranian trojan was not announced until 2013.

2014 OL₃₃₉ (also written 2014 OL339) is an Aten asteroid that is a temporary quasi-satellite of Earth, the fourth known Earth quasi-satellite.

2015 SO₂ (also written 2015 SO2) is an Aten asteroid that is a temporary horseshoe companion to the Earth, the ninth known Earth horseshoe librator. Prior to its most recent close encounter with our planet (2015 September 30) it was an Apollo asteroid.

2015 XX₁₆₉ (also written 2015 XX169) is an Apollo asteroid that is a temporary horseshoe companion to the Earth, the tenth known Earth horseshoe librator. A close encounter with the Earth on 14 December 2015 caused the value of the semi-major axis of 2015 XX₁₆₉ to drift slowly upwards, and the object evolved from an Aten asteroid to an Apollo asteroid about a year after this close approach.

2015 YQ₁ (also written 2015 YQ1) is an Apollo asteroid that is a temporary horseshoe companion to the Earth, the twelfth known Earth horseshoe librator. It experienced a close encounter with the Earth on 2015 December 22 at 0.0037 AU.

2015 YA is a sub-kilometer asteroid, classified as near-Earth object of the Aten group, that is a temporary horseshoe companion to the Earth. It is the 11th known Earth horseshoe librator. Prior to a close encounter with the Earth on 15 December 2015, 2015 YA was an Apollo asteroid.

2017 FZ₂ (also written 2017 FZ2) is a micro-asteroid and near-Earth object of the Apollo group that was a quasi-satellite of the Earth until March 23, 2017.

A temporary satellite is an object which has been captured by the gravitational field of a planet and thus has become the planet's natural satellite, but, unlike irregular moons of the larger outer planets of the Solar System, will eventually either leave its orbit around the planet or collide with the planet. The only observed examples are 2006 RH120, a temporary satellite of Earth for twelve months from July 2006 to July 2007, and 2020 CD3, which was discovered in 2020. Some defunct space probes or rockets have also been observed on temporary satellite orbits.

2017 SN₁₆, is a sub-kilometer asteroid, classified as a near-Earth object of the Apollo group, approximately 90 meters (300 feet) in diameter. The object was first observed on 24 September 2017, by cometary discoverer Alex Gibbs with the Mount Lemmon Survey at Mount Lemmon Observatory, Arizona, in the United States. It forms an asteroid pair with 2018 RY7 and is currently trapped in a 3:5 mean motion resonance with Venus.

2020 PN₁ is a sub-kilometer asteroid, classified as a near-Earth object of the Aten group, that is a temporary horseshoe companion to the Earth. There are dozens of known Earth horseshoe librators, some of which switch periodically between the quasi-satellite and the horseshoe co-orbital states.

2020 PP₁ is a sub-kilometer asteroid, classified as a near-Earth object of the Apollo group, that is a temporary quasi-satellite of the Earth. There are over a dozen known Earth quasi-satellites, some of which switch periodically between the quasi-satellite and horseshoe co-orbital states.

References

↑ Christou, Apostolos A.; Asher, David J. (2011). "A long-lived horseshoe companion to the Earth". Monthly Notices of the Royal Astronomical Society. 414 (4): 2965–2969. arXiv: 1104.0036 . Bibcode:2011MNRAS.414.2965C. doi:10.1111/j.1365-2966.2011.18595.x. S2CID 13832179.
↑ de la Fuente Marcos, C.; de la Fuente Marcos, R. (April 2016). "A trio of horseshoes: past, present and future dynamical evolution of Earth co-orbital asteroids 2015 XX₁₆₉, 2015 YA and 2015 YQ₁". Astrophysics and Space Science. 361 (4): 121–133. arXiv: 1603.02415 . Bibcode:2016Ap&SS.361..121D. doi:10.1007/s10509-016-2711-6. S2CID 119222384.
↑ de la Fuente Marcos, C.; de la Fuente Marcos, R. (November 11, 2016). "Asteroid (469219) (469219) 2016 HO₃, the smallest and closest Earth quasi-satellite". Monthly Notices of the Royal Astronomical Society. 462 (4): 3441–3456. arXiv: 1608.01518 . Bibcode:2016MNRAS.462.3441D. doi:10.1093/mnras/stw1972. S2CID 118580771.
↑ de la Fuente Marcos, Carlos; de la Fuente Marcos, Raúl (March 2021). "Using Mars co-orbitals to estimate the importance of rotation-induced YORP break-up events in Earth co-orbital space". Monthly Notices of the Royal Astronomical Society . 501 (4): 6007–6025. arXiv: 2101.02563 . Bibcode:2021MNRAS.501.6007D. doi:10.1093/mnras/stab062.

External links

Research paper describing Horseshoe orbits. Start at page 105.
A good description of 2002 AA29

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Christou, Apostolos A.; Asher, David J. (2011). "A long-lived horseshoe companion to the Earth". Monthly Notices of the Royal Astronomical Society. 414 (4): 2965–2969. arXiv: 1104.0036 . Bibcode:2011MNRAS.414.2965C. doi:10.1111/j.1365-2966.2011.18595.x. S2CID 13832179.

[Fuentes-horseshoe-2] Fuente Marcos, C.; de la Fuente Marcos, R. (April 2016). "A trio of horseshoes: past, present and future dynamical evolution of Earth co-orbital asteroids 2015 XX₁₆₉, 2015 YA and 2015 YQ₁". Astrophysics and Space Science. 361 (4): 121–133. arXiv: 1603.02415 . Bibcode:2016Ap&SS.361..121D. doi:10.1007/s10509-016-2711-6. S2CID 119222384.

[Fuentes-2016HO3-3] Fuente Marcos, C.; de la Fuente Marcos, R. (November 11, 2016). "Asteroid (469219) (469219) 2016 HO₃, the smallest and closest Earth quasi-satellite". Monthly Notices of the Royal Astronomical Society. 462 (4): 3441–3456. arXiv: 1608.01518 . Bibcode:2016MNRAS.462.3441D. doi:10.1093/mnras/stw1972. S2CID 118580771.

[MarsHorseshoe-4] Fuente Marcos, Carlos; de la Fuente Marcos, Raúl (March 2021). "Using Mars co-orbitals to estimate the importance of rotation-induced YORP break-up events in Earth co-orbital space". Monthly Notices of the Royal Astronomical Society . 501 (4): 6007–6025. arXiv: 2101.02563 . Bibcode:2021MNRAS.501.6007D. doi:10.1093/mnras/stab062.

[1]

[2]

[3]

[4]