| Nuclear physics |
|---|
 |
This article summarizes equations in the theory of nuclear physics and particle physics.
| Nuclear physics |
|---|
 |
This article summarizes equations in the theory of nuclear physics and particle physics.
| Quantity (common name/s) | (Common) symbol/s | Defining equation | SI units | Dimension |
|---|---|---|---|---|
| Number of atoms | N = Number of atoms remaining at time t N0 = Initial number of atoms at time t = 0 | dimensionless | dimensionless | |
| Decay rate, activity of a radioisotope | A | Bq = Hz = s−1 | [T]−1 | |
| Decay constant | λ | Bq = Hz = s−1 | [T]−1 | |
| Half-life of a radioisotope | t1/2, T1/2 | Time taken for half the number of atoms present to decay | s | [T] |
| Number of half-lives | n (no standard symbol) | dimensionless | dimensionless | |
| Radioisotope time constant, mean lifetime of an atom before decay | τ (no standard symbol) | s | [T] | |
| Absorbed dose, total ionizing dose (total energy of radiation transferred to unit mass) | D can only be found experimentally | N/A | Gy = 1 J/kg (Gray) | [L]2[T]−2 |
| Equivalent dose | H | Q = radiation quality factor (dimensionless) | Sv = J kg−1 (Sievert) | [L]2[T]−2 |
| Effective dose | E | Wj = weighting factors corresponding to radiosensitivities of matter (dimensionless) | Sv = J kg−1 (Sievert) | [L]2[T]−2 |
| Physical situation | Nomenclature | Equations |
|---|---|---|
| Mass number |
| |
| Mass in nuclei |
| |
| Nuclear radius | r0 ≈ 1.2 fm | hence (approximately)
|
| Nuclear binding energy, empirical curve | Dimensionless parameters to fit experiment:
| where (due to pairing of nuclei)
|
| Physical situation | Nomenclature | Equations |
|---|---|---|
| Radioactive decay |
| Statistical decay of a radionuclide: |
| Bateman's equations | ||
| Radiation flux |
| |
The following apply for the nuclear reaction:
in the centre of mass frame, where a and b are the initial species about to collide, c is the final species, and R is the resonant state.
| Physical situation | Nomenclature | Equations |
|---|---|---|
| Breit-Wigner formula |
| Cross-section: Spin factor: Total width: Resonance lifetime: |
| Born scattering |
| Differential cross-section: |
| Mott scattering |
| Differential cross-section (for identical particles in a coulomb potential, in centre of mass frame): Scattering potential energy (α = constant): |
| Rutherford scattering | Differential cross-section (non-identical particles in a coulomb potential): | |
These equations need to be refined such that the notation is defined as has been done for the previous sets of equations.
| Name | Equations |
|---|---|
| Strong force | |
| Electroweak interaction | |
| Quantum electrodynamics |

In physics, electromagnetism is an interaction that occurs between particles with electric charge via electromagnetic fields. The electromagnetic force is one of the four fundamental forces of nature. It is the dominant force in the interactions of atoms and molecules. Electromagnetism can be thought of as a combination of electrostatics and magnetism, which are distinct but closely intertwined phenomena. Electromagnetic forces occur between any two charged particles. Electric forces cause an attraction between particles with opposite charges and repulsion between particles with the same charge, while magnetism is an interaction that occurs between charged particles in relative motion. These two forces are described in terms of electromagnetic fields. Macroscopic charged objects are described in terms of Coulomb's law for electricity and Ampère's force law for magnetism; the Lorentz force describes microscopic charged particles.
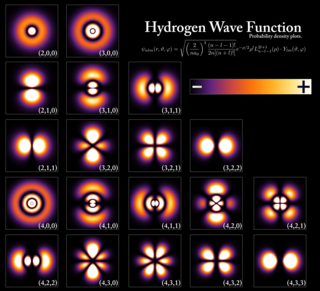
Quantum mechanics is a fundamental theory in physics that describes the behavior of nature at and below the scale of atoms. It is the foundation of all quantum physics, which includes quantum chemistry, quantum field theory, quantum technology, and quantum information science.
Quantum chemistry, also called molecular quantum mechanics, is a branch of physical chemistry focused on the application of quantum mechanics to chemical systems, particularly towards the quantum-mechanical calculation of electronic contributions to physical and chemical properties of molecules, materials, and solutions at the atomic level. These calculations include systematically applied approximations intended to make calculations computationally feasible while still capturing as much information about important contributions to the computed wave functions as well as to observable properties such as structures, spectra, and thermodynamic properties. Quantum chemistry is also concerned with the computation of quantum effects on molecular dynamics and chemical kinetics.
Atomic, molecular, and optical physics (AMO) is the study of matter–matter and light–matter interactions, at the scale of one or a few atoms and energy scales around several electron volts. The three areas are closely interrelated. AMO theory includes classical, semi-classical and quantum treatments. Typically, the theory and applications of emission, absorption, scattering of electromagnetic radiation (light) from excited atoms and molecules, analysis of spectroscopy, generation of lasers and masers, and the optical properties of matter in general, fall into these categories.

In quantum physics and chemistry, quantum numbers are quantities that characterize the possible states of the system. Quantum numbers are closely related to eigenvalues of observables. When the corresponding observable commutes with the Hamiltonian, the quantum number is said to be "good", and acts as a constant of motion in the quantum dynamics.

In physics, specifically relativistic quantum mechanics (RQM) and its applications to particle physics, relativistic wave equations predict the behavior of particles at high energies and velocities comparable to the speed of light. In the context of quantum field theory (QFT), the equations determine the dynamics of quantum fields. The solutions to the equations, universally denoted as ψ or Ψ, are referred to as "wave functions" in the context of RQM, and "fields" in the context of QFT. The equations themselves are called "wave equations" or "field equations", because they have the mathematical form of a wave equation or are generated from a Lagrangian density and the field-theoretic Euler–Lagrange equations.
In physics, semiclassical refers to a theory in which one part of a system is described quantum mechanically, whereas the other is treated classically. For example, external fields will be constant, or when changing will be classically described. In general, it incorporates a development in powers of Planck's constant, resulting in the classical physics of power 0, and the first nontrivial approximation to the power of (−1). In this case, there is a clear link between the quantum-mechanical system and the associated semi-classical and classical approximations, as it is similar in appearance to the transition from physical optics to geometric optics.

Modern physics is a branch of physics that developed in the early 20th century and onward or branches greatly influenced by early 20th century physics. Notable branches of modern physics include quantum mechanics, special relativity, and general relativity.

In physics, specifically field theory and particle physics, the Proca action describes a massive spin-1 field of mass m in Minkowski spacetime. The corresponding equation is a relativistic wave equation called the Proca equation. The Proca action and equation are named after Romanian physicist Alexandru Proca.
In theoretical physics and applied mathematics, a field equation is a partial differential equation which determines the dynamics of a physical field, specifically the time evolution and spatial distribution of the field. The solutions to the equation are mathematical functions which correspond directly to the field, as functions of time and space. Since the field equation is a partial differential equation, there are families of solutions which represent a variety of physical possibilities. Usually, there is not just a single equation, but a set of coupled equations which must be solved simultaneously. Field equations are not ordinary differential equations since a field depends on space and time, which requires at least two variables.
In physics, relativistic mechanics refers to mechanics compatible with special relativity (SR) and general relativity (GR). It provides a non-quantum mechanical description of a system of particles, or of a fluid, in cases where the velocities of moving objects are comparable to the speed of light c. As a result, classical mechanics is extended correctly to particles traveling at high velocities and energies, and provides a consistent inclusion of electromagnetism with the mechanics of particles. This was not possible in Galilean relativity, where it would be permitted for particles and light to travel at any speed, including faster than light. The foundations of relativistic mechanics are the postulates of special relativity and general relativity. The unification of SR with quantum mechanics is relativistic quantum mechanics, while attempts for that of GR is quantum gravity, an unsolved problem in physics.
In physics, relativistic quantum mechanics (RQM) is any Poincaré covariant formulation of quantum mechanics (QM). This theory is applicable to massive particles propagating at all velocities up to those comparable to the speed of light c, and can accommodate massless particles. The theory has application in high energy physics, particle physics and accelerator physics, as well as atomic physics, chemistry and condensed matter physics. Non-relativistic quantum mechanics refers to the mathematical formulation of quantum mechanics applied in the context of Galilean relativity, more specifically quantizing the equations of classical mechanics by replacing dynamical variables by operators. Relativistic quantum mechanics (RQM) is quantum mechanics applied with special relativity. Although the earlier formulations, like the Schrödinger picture and Heisenberg picture were originally formulated in a non-relativistic background, a few of them also work with special relativity.

In the physical sciences, a particle is a small localized object which can be described by several physical or chemical properties, such as volume, density, or mass. They vary greatly in size or quantity, from subatomic particles like the electron, to microscopic particles like atoms and molecules, to macroscopic particles like powders and other granular materials. Particles can also be used to create scientific models of even larger objects depending on their density, such as humans moving in a crowd or celestial bodies in motion.