| Part of a series of articles about |
| Quantum mechanics |
|---|
This article summarizes equations in the theory of quantum mechanics.
| Part of a series of articles about |
| Quantum mechanics |
|---|
This article summarizes equations in the theory of quantum mechanics.
A fundamental physical constant occurring in quantum mechanics is the Planck constant , h. A common abbreviation is ħ = h/2π, also known as the reduced Planck constant or Dirac constant.
| Quantity (common name/s) | (Common) symbol/s | Defining equation | SI unit | Dimension |
|---|---|---|---|---|
| Wavefunction | ψ, Ψ | To solve from the Schrödinger equation | varies with situation and number of particles | |
| Wavefunction probability density | ρ | m−3 | [L]−3 | |
| Wavefunction probability current | j | Non-relativistic, no external field: star * is complex conjugate | m−2⋅s−1 | [T]−1 [L]−2 |
The general form of wavefunction for a system of particles, each with position ri and z-component of spin sz i. Sums are over the discrete variable sz, integrals over continuous positions r.
For clarity and brevity, the coordinates are collected into tuples, the indices label the particles (which cannot be done physically, but is mathematically necessary). Following are general mathematical results, used in calculations.
| Property or effect | Nomenclature | Equation |
|---|---|---|
| Wavefunction for N particles in 3d |
| In function notation: in bra–ket notation: for non-interacting particles: |
| Position-momentum Fourier transform (1 particle in 3d) |
| |
| General probability distribution |
| |
| General normalization condition | ||
| Property or effect | Nomenclature | Equation |
|---|---|---|
| Planck–Einstein equation and de Broglie wavelength relations |
| |
| Schrödinger equation |
| General time-dependent case: Time-independent case: |
| Heisenberg equation |
| |
| Time evolution in Heisenberg picture (Ehrenfest theorem) |
of a particle. | For momentum and position; |
Summarized below are the various forms the Hamiltonian takes, with the corresponding Schrödinger equations and forms of wavefunction solutions. Notice in the case of one spatial dimension, for one particle, the partial derivative reduces to an ordinary derivative.
| One particle | N particles | |
| One dimension | where the position of particle n is xn. | |
There is a further restriction — the solution must not grow at infinity, so that it has either a finite L2-norm (if it is a bound state) or a slowly diverging norm (if it is part of a continuum): [1] | for non-interacting particles | |
| Three dimensions | where the position of the particle is r = (x, y, z). | where the position of particle n is rn = (xn, yn, zn), and the Laplacian for particle n using the corresponding position coordinates is |
for non-interacting particles |
Again, summarized below are the various forms the Hamiltonian takes, with the corresponding Schrödinger equations and forms of solutions.
| One particle | N particles | |
| One dimension | where the position of particle n is xn. | |
| Three dimensions | ||
This last equation is in a very high dimension, [2] so the solutions are not easy to visualize. | ||
| Property/Effect | Nomenclature | Equation |
|---|---|---|
| Photoelectric equation |
| |
| Threshold frequency and Work function |
| Can only be found by experiment. The De Broglie relations give the relation between them: |
| Photon momentum |
| The De Broglie relations give: |
| Property or effect | Nomenclature | Equation |
|---|---|---|
| Heisenberg's uncertainty principles |
| Position–momentum Energy-time Number-phase |
| Dispersion of observable | A = observables (eigenvalues of operator) | |
| General uncertainty relation | A, B = observables (eigenvalues of operator) | |
| Property or effect | Equation |
|---|---|
| Density of states | |
| Fermi–Dirac distribution (fermions) | where
|
| Bose–Einstein distribution (bosons) | |
| Property or effect | Nomenclature | Equation |
|---|---|---|
| Angular momentum quantum numbers |
| Spin: Orbital: Total: |
| Angular momentum magnitudes | angular momementa:
| Spin magnitude: Orbital magnitude: Total magnitude: |
| Angular momentum components | Spin: Orbital: | |
In what follows, B is an applied external magnetic field and the quantum numbers above are used.
| Property or effect | Nomenclature | Equation |
|---|---|---|
| orbital magnetic dipole moment |
| z-component: |
| spin magnetic dipole moment |
| z-component: |
| dipole moment potential | U = potential energy of dipole in field | |
| Property or effect | Nomenclature | Equation |
|---|---|---|
| Energy level |
| |
| Spectrum | λ = wavelength of emitted photon, during electronic transition from Ei to Ej | |
The mathematical formulations of quantum mechanics are those mathematical formalisms that permit a rigorous description of quantum mechanics. This mathematical formalism uses mainly a part of functional analysis, especially Hilbert spaces, which are a kind of linear space. Such are distinguished from mathematical formalisms for physics theories developed prior to the early 1900s by the use of abstract mathematical structures, such as infinite-dimensional Hilbert spaces, and operators on these spaces. In brief, values of physical observables such as energy and momentum were no longer considered as values of functions on phase space, but as eigenvalues; more precisely as spectral values of linear operators in Hilbert space.
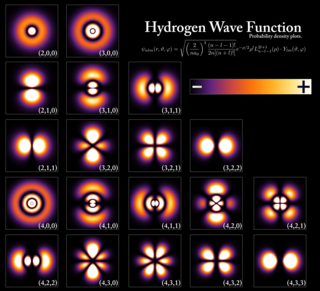
Quantum mechanics is a fundamental theory that describes the behavior of nature at and below the scale of atoms. It is the foundation of all quantum physics, which includes quantum chemistry, quantum field theory, quantum technology, and quantum information science.
The Schrödinger equation is a partial differential equation that governs the wave function of a quantum-mechanical system. Its discovery was a significant landmark in the development of quantum mechanics. It is named after Erwin Schrödinger, who postulated the equation in 1925 and published it in 1926, forming the basis for the work that resulted in his Nobel Prize in Physics in 1933.

In quantum physics, a wave function is a mathematical description of the quantum state of an isolated quantum system. The most common symbols for a wave function are the Greek letters ψ and Ψ. Wave functions are complex-valued. For example, a wave function might assign a complex number to each point in a region of space. The Born rule provides the means to turn these complex probability amplitudes into actual probabilities. In one common form, it says that the squared modulus of a wave function that depends upon position is the probability density of measuring a particle as being at a given place. The integral of a wavefunction's squared modulus over all the system's degrees of freedom must be equal to 1, a condition called normalization. Since the wave function is complex-valued, only its relative phase and relative magnitude can be measured; its value does not, in isolation, tell anything about the magnitudes or directions of measurable observables. One has to apply quantum operators, whose eigenvalues correspond to sets of possible results of measurements, to the wave function ψ and calculate the statistical distributions for measurable quantities.
In physics, action is a scalar quantity that describes how the balance of kinetic versus potential energy of a physical system changes with trajectory. Action is significant because it is an input to the principle of stationary action, an approach to classical mechanics that is simpler for multiple objects. Action and the variational principle are used in Feynman's quantum mechanics and in general relativity. For systems with small values of action similar to the Planck constant, quantum effects are significant.
In physics, the Schrödinger picture or Schrödinger representation is a formulation of quantum mechanics in which the state vectors evolve in time, but the operators are mostly constant with respect to time. This differs from the Heisenberg picture which keeps the states constant while the observables evolve in time, and from the interaction picture in which both the states and the observables evolve in time. The Schrödinger and Heisenberg pictures are related as active and passive transformations and commutation relations between operators are preserved in the passage between the two pictures.

In quantum physics and chemistry, quantum numbers are quantities that characterize the possible states of the system. Quantum numbers are closely related to eigenvalues of observables. When the corresponding observable commutes with the Hamiltonian, the quantum number is said to be "good", and acts as a constant of motion in the quantum dynamics.

In physics, specifically relativistic quantum mechanics (RQM) and its applications to particle physics, relativistic wave equations predict the behavior of particles at high energies and velocities comparable to the speed of light. In the context of quantum field theory (QFT), the equations determine the dynamics of quantum fields. The solutions to the equations, universally denoted as ψ or Ψ, are referred to as "wave functions" in the context of RQM, and "fields" in the context of QFT. The equations themselves are called "wave equations" or "field equations", because they have the mathematical form of a wave equation or are generated from a Lagrangian density and the field-theoretic Euler–Lagrange equations.
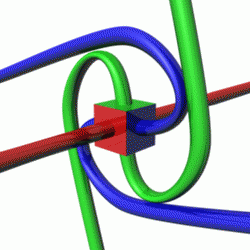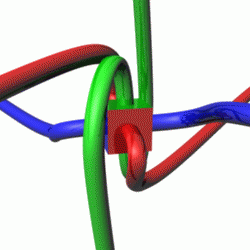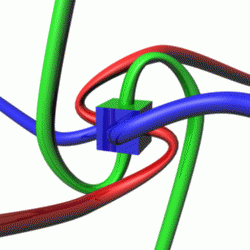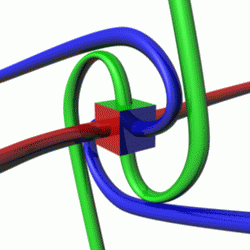
In quantum mechanics, spin is an intrinsic property of all elementary particles. All known fermions, the particles that constitute ordinary matter, have a spin of 1/2. The spin number describes how many symmetrical facets a particle has in one full rotation; a spin of 1/2 means that the particle must be rotated by two full turns before it has the same configuration as when it started.
In physics, relativistic quantum mechanics (RQM) is any Poincaré covariant formulation of quantum mechanics (QM). This theory is applicable to massive particles propagating at all velocities up to those comparable to the speed of light c, and can accommodate massless particles. The theory has application in high energy physics, particle physics and accelerator physics, as well as atomic physics, chemistry and condensed matter physics. Non-relativistic quantum mechanics refers to the mathematical formulation of quantum mechanics applied in the context of Galilean relativity, more specifically quantizing the equations of classical mechanics by replacing dynamical variables by operators. Relativistic quantum mechanics (RQM) is quantum mechanics applied with special relativity. Although the earlier formulations, like the Schrödinger picture and Heisenberg picture were originally formulated in a non-relativistic background, a few of them also work with special relativity.
In quantum mechanics, energy is defined in terms of the energy operator, acting on the wave function of the system as a consequence of time translation symmetry.
Peter R. Holland is an English theoretical physicist, known for his work on foundational problems in quantum physics and in particular his book on the pilot wave theory and the de Broglie-Bohm causal interpretation of quantum mechanics.
In general relativity, the Hamilton–Jacobi–Einstein equation (HJEE) or Einstein–Hamilton–Jacobi equation (EHJE) is an equation in the Hamiltonian formulation of geometrodynamics in superspace, cast in the "geometrodynamics era" around the 1960s, by Asher Peres in 1962 and others. It is an attempt to reformulate general relativity in such a way that it resembles quantum theory within a semiclassical approximation, much like the correspondence between quantum mechanics and classical mechanics.