In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.

In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a 5-hypercube (penteract) with alternated vertices removed.
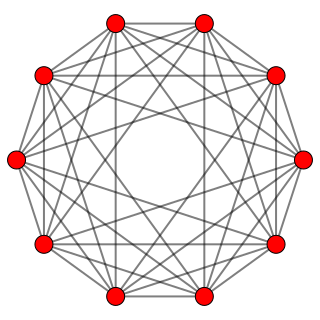
In five-dimensional geometry, a 5-orthoplex, or 5-cross polytope, is a five-dimensional polytope with 10 vertices, 40 edges, 80 triangle faces, 80 tetrahedron cells, 32 5-cell 4-faces.

In geometry, a 6-demicube or demihexeract is a uniform 6-polytope, constructed from a 6-cube (hexeract) with alternated vertices removed. It is part of a dimensionally infinite family of uniform polytopes called demihypercubes.

In geometry, a 7-cube is a seven-dimensional hypercube with 128 vertices, 448 edges, 672 square faces, 560 cubic cells, 280 tesseract 4-faces, 84 penteract 5-faces, and 14 hexeract 6-faces.

In geometry, a demihepteract or 7-demicube is a uniform 7-polytope, constructed from the 7-hypercube (hepteract) with alternated vertices removed. It is part of a dimensionally infinite family of uniform polytopes called demihypercubes.

In geometry, a demiocteract or 8-demicube is a uniform 8-polytope, constructed from the 8-hypercube, octeract, with alternated vertices removed. It is part of a dimensionally infinite family of uniform polytopes called demihypercubes.
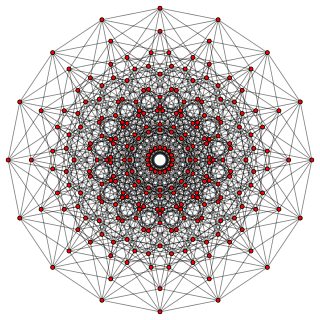
In geometry, an 8-cube is an eight-dimensional hypercube. It has 256 vertices, 1024 edges, 1792 square faces, 1792 cubic cells, 1120 tesseract 4-faces, 448 5-cube 5-faces, 112 6-cube 6-faces, and 16 7-cube 7-faces.

In geometry, a 9-cube is a nine-dimensional hypercube with 512 vertices, 2304 edges, 4608 square faces, 5376 cubic cells, 4032 tesseract 4-faces, 2016 5-cube 5-faces, 672 6-cube 6-faces, 144 7-cube 7-faces, and 18 8-cube 8-faces.
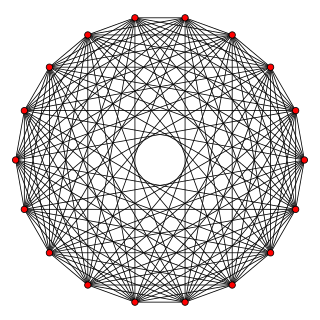
In geometry, a 9-orthoplex or 9-cross polytope, is a regular 9-polytope with 18 vertices, 144 edges, 672 triangle faces, 2016 tetrahedron cells, 4032 5-cells 4-faces, 5376 5-simplex 5-faces, 4608 6-simplex 6-faces, 2304 7-simplex 7-faces, and 512 8-simplex 8-faces.

In geometry, a 10-cube is a ten-dimensional hypercube. It has 1024 vertices, 5120 edges, 11520 square faces, 15360 cubic cells, 13440 tesseract 4-faces, 8064 5-cube 5-faces, 3360 6-cube 6-faces, 960 7-cube 7-faces, 180 8-cube 8-faces, and 20 9-cube 9-faces.
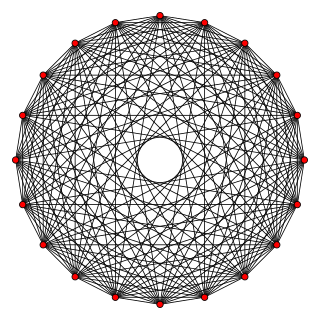
In geometry, a 10-orthoplex or 10-cross polytope, is a regular 10-polytope with 20 vertices, 180 edges, 960 triangle faces, 3360 octahedron cells, 8064 5-cells 4-faces, 13440 5-faces, 15360 6-faces, 11520 7-faces, 5120 8-faces, and 1024 9-faces.

In geometry, a 10-demicube or demidekeract is a uniform 10-polytope, constructed from the 10-cube with alternated vertices removed. It is part of a dimensionally infinite family of uniform polytopes called demihypercubes.

In geometry of five dimensions or higher, a cantic 5-cube, cantihalf 5-cube, truncated 5-demicube is a uniform 5-polytope, being a truncation of the 5-demicube. It has half the vertices of a cantellated 5-cube.

In five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation (runcination) of the regular 5-orthoplex.

In seven-dimensional geometry, a cantic 7-cube or truncated 7-demicube as a uniform 7-polytope, being a truncation of the 7-demicube.
In seven-dimensional geometry, a runcic 7-cube is a convex uniform 7-polytope, related to the uniform 7-demicube. There are 2 unique forms.

In seven-dimensional geometry, a pentic 7-cube is a convex uniform 7-polytope, related to the uniform 7-demicube. There are 8 unique forms.

In seven-dimensional geometry, a hexic 7-cube is a convex uniform 7-polytope, constructed from the uniform 7-demicube. There are 16 unique forms.

In seven-dimensional geometry, a stericated 7-cube is a convex uniform 7-polytope, being a runcination of the uniform 7-demicube. There are 4 unique runcinations for the 7-demicube including truncation and cantellation.

![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() and Schläfli symbol or {3,36,1}.
and Schläfli symbol or {3,36,1}.

























