Uniform 7-polytope

Graphs of three regular and related uniform polytopes

7-simplex				Rectified 7-simplex				Truncated 7-simplex
Cantellated 7-simplex				Runcinated 7-simplex				Stericated 7-simplex
Pentellated 7-simplex						Hexicated 7-simplex
7-orthoplex				Truncated 7-orthoplex				Rectified 7-orthoplex
Cantellated 7-orthoplex				Runcinated 7-orthoplex				Stericated 7-orthoplex
Pentellated 7-orthoplex				Hexicated 7-cube				Pentellated 7-cube
Stericated 7-cube				Cantellated 7-cube				Runcinated 7-cube
7-cube				Truncated 7-cube				Rectified 7-cube
7-demicube				Cantic 7-cube				Runcic 7-cube
Steric 7-cube				Pentic 7-cube				Hexic 7-cube
321				231				132

In seven-dimensional geometry, a 7-polytope is a polytope contained by 6-polytope facets. Each 5-polytope ridge being shared by exactly two 6-polytope facets.

A uniform 7-polytope is one whose symmetry group is transitive on vertices and whose facets are uniform 6-polytopes.

Regular 7-polytopes

Regular 7-polytopes are represented by the Schläfli symbol {p,q,r,s,t,u} with u {p,q,r,s,t} 6-polytopes facets around each 4-face.

There are exactly three such convex regular 7-polytopes:

1. {3,3,3,3,3,3} - 7-simplex
2. {4,3,3,3,3,3} - 7-cube
3. {3,3,3,3,3,4} - 7-orthoplex

There are no nonconvex regular 7-polytopes.

Characteristics

The topology of any given 7-polytope is defined by its Betti numbers and torsion coefficients. ^[1]

The value of the Euler characteristic used to characterise polyhedra does not generalize usefully to higher dimensions, whatever their underlying topology. This inadequacy of the Euler characteristic to reliably distinguish between different topologies in higher dimensions led to the discovery of the more sophisticated Betti numbers. ^[1]

Similarly, the notion of orientability of a polyhedron is insufficient to characterise the surface twistings of toroidal polytopes, and this led to the use of torsion coefficients. ^[1]

Uniform 7-polytopes by fundamental Coxeter groups

Uniform 7-polytopes with reflective symmetry can be generated by these four Coxeter groups, represented by permutations of rings of the Coxeter-Dynkin diagrams:

#	Coxeter group			Regular and semiregular forms	Uniform count
1	A7	[36]		7-simplex - {36},	71
2	B7	[4,35]		7-cube - {4,35}, 7-orthoplex - {35,4}, 7-demicube - h{4,35},	127 + 32
3	D7	[33,1,1]		7-demicube, {3,34,1}, 7-orthoplex, {34,31,1},	95 (0 unique)
4	E7	[33,2,1]		321 - 132 - 231 -	127

Prismatic finite Coxeter groups
#	Coxeter group		Coxeter diagram
6+1
1	A6A1	[35]×[ ]
2	BC6A1	[4,34]×[ ]
3	D6A1	[33,1,1]×[ ]
4	E6A1	[32,2,1]×[ ]
5+2
1	A5I2(p)	[3,3,3]×[p]
2	BC5I2(p)	[4,3,3]×[p]
3	D5I2(p)	[32,1,1]×[p]
5+1+1
1	A5A12	[3,3,3]×[ ]2
2	BC5A12	[4,3,3]×[ ]2
3	D5A12	[32,1,1]×[ ]2
4+3
1	A4A3	[3,3,3]×[3,3]
2	A4B3	[3,3,3]×[4,3]
3	A4H3	[3,3,3]×[5,3]
4	BC4A3	[4,3,3]×[3,3]
5	BC4B3	[4,3,3]×[4,3]
6	BC4H3	[4,3,3]×[5,3]
7	H4A3	[5,3,3]×[3,3]
8	H4B3	[5,3,3]×[4,3]
9	H4H3	[5,3,3]×[5,3]
10	F4A3	[3,4,3]×[3,3]
11	F4B3	[3,4,3]×[4,3]
12	F4H3	[3,4,3]×[5,3]
13	D4A3	[31,1,1]×[3,3]
14	D4B3	[31,1,1]×[4,3]
15	D4H3	[31,1,1]×[5,3]
4+2+1
1	A4I2(p)A1	[3,3,3]×[p]×[ ]
2	BC4I2(p)A1	[4,3,3]×[p]×[ ]
3	F4I2(p)A1	[3,4,3]×[p]×[ ]
4	H4I2(p)A1	[5,3,3]×[p]×[ ]
5	D4I2(p)A1	[31,1,1]×[p]×[ ]
4+1+1+1
1	A4A13	[3,3,3]×[ ]3
2	BC4A13	[4,3,3]×[ ]3
3	F4A13	[3,4,3]×[ ]3
4	H4A13	[5,3,3]×[ ]3
5	D4A13	[31,1,1]×[ ]3
3+3+1
1	A3A3A1	[3,3]×[3,3]×[ ]
2	A3B3A1	[3,3]×[4,3]×[ ]
3	A3H3A1	[3,3]×[5,3]×[ ]
4	BC3B3A1	[4,3]×[4,3]×[ ]
5	BC3H3A1	[4,3]×[5,3]×[ ]
6	H3A3A1	[5,3]×[5,3]×[ ]
3+2+2
1	A3I2(p)I2(q)	[3,3]×[p]×[q]
2	BC3I2(p)I2(q)	[4,3]×[p]×[q]
3	H3I2(p)I2(q)	[5,3]×[p]×[q]
3+2+1+1
1	A3I2(p)A12	[3,3]×[p]×[ ]2
2	BC3I2(p)A12	[4,3]×[p]×[ ]2
3	H3I2(p)A12	[5,3]×[p]×[ ]2
3+1+1+1+1
1	A3A14	[3,3]×[ ]4
2	BC3A14	[4,3]×[ ]4
3	H3A14	[5,3]×[ ]4
2+2+2+1
1	I2(p)I2(q)I2(r)A1	[p]×[q]×[r]×[ ]
2+2+1+1+1
1	I2(p)I2(q)A13	[p]×[q]×[ ]3
2+1+1+1+1+1
1	I2(p)A15	[p]×[ ]5
1+1+1+1+1+1+1
1	A17	[ ]7

The A₇ family

The A₇ family has symmetry of order 40320 (8 factorial).

There are 71 (64+8-1) forms based on all permutations of the Coxeter-Dynkin diagrams with one or more rings. All 71 are enumerated below. Norman Johnson's truncation names are given. Bowers names and acronym are also given for cross-referencing.

See also a list of A7 polytopes for symmetric Coxeter plane graphs of these polytopes.

A7 uniform polytopes
#	Coxeter-Dynkin diagram	Truncation indices	Johnson name Bowers name (and acronym)	Basepoint	Element counts
					6	5	4	3	2	1	0
1		t0	7-simplex (oca)	(0,0,0,0,0,0,0,1)	8	28	56	70	56	28	8
2		t1	Rectified 7-simplex (roc)	(0,0,0,0,0,0,1,1)	16	84	224	350	336	168	28
3		t2	Birectified 7-simplex (broc)	(0,0,0,0,0,1,1,1)	16	112	392	770	840	420	56
4		t3	Trirectified 7-simplex (he)	(0,0,0,0,1,1,1,1)	16	112	448	980	1120	560	70
5		t0,1	Truncated 7-simplex (toc)	(0,0,0,0,0,0,1,2)	16	84	224	350	336	196	56
6		t0,2	Cantellated 7-simplex (saro)	(0,0,0,0,0,1,1,2)	44	308	980	1750	1876	1008	168
7		t1,2	Bitruncated 7-simplex (bittoc)	(0,0,0,0,0,1,2,2)						588	168
8		t0,3	Runcinated 7-simplex (spo)	(0,0,0,0,1,1,1,2)	100	756	2548	4830	4760	2100	280
9		t1,3	Bicantellated 7-simplex (sabro)	(0,0,0,0,1,1,2,2)						2520	420
10		t2,3	Tritruncated 7-simplex (tattoc)	(0,0,0,0,1,2,2,2)						980	280
11		t0,4	Stericated 7-simplex (sco)	(0,0,0,1,1,1,1,2)						2240	280
12		t1,4	Biruncinated 7-simplex (sibpo)	(0,0,0,1,1,1,2,2)						4200	560
13		t2,4	Tricantellated 7-simplex (stiroh)	(0,0,0,1,1,2,2,2)						3360	560
14		t0,5	Pentellated 7-simplex (seto)	(0,0,1,1,1,1,1,2)						1260	168
15		t1,5	Bistericated 7-simplex (sabach)	(0,0,1,1,1,1,2,2)						3360	420
16		t0,6	Hexicated 7-simplex (suph)	(0,1,1,1,1,1,1,2)						336	56
17		t0,1,2	Cantitruncated 7-simplex (garo)	(0,0,0,0,0,1,2,3)						1176	336
18		t0,1,3	Runcitruncated 7-simplex (patto)	(0,0,0,0,1,1,2,3)						4620	840
19		t0,2,3	Runcicantellated 7-simplex (paro)	(0,0,0,0,1,2,2,3)						3360	840
20		t1,2,3	Bicantitruncated 7-simplex (gabro)	(0,0,0,0,1,2,3,3)						2940	840
21		t0,1,4	Steritruncated 7-simplex (cato)	(0,0,0,1,1,1,2,3)						7280	1120
22		t0,2,4	Stericantellated 7-simplex (caro)	(0,0,0,1,1,2,2,3)						10080	1680
23		t1,2,4	Biruncitruncated 7-simplex (bipto)	(0,0,0,1,1,2,3,3)						8400	1680
24		t0,3,4	Steriruncinated 7-simplex (cepo)	(0,0,0,1,2,2,2,3)						5040	1120
25		t1,3,4	Biruncicantellated 7-simplex (bipro)	(0,0,0,1,2,2,3,3)						7560	1680
26		t2,3,4	Tricantitruncated 7-simplex (gatroh)	(0,0,0,1,2,3,3,3)						3920	1120
27		t0,1,5	Pentitruncated 7-simplex (teto)	(0,0,1,1,1,1,2,3)						5460	840
28		t0,2,5	Penticantellated 7-simplex (tero)	(0,0,1,1,1,2,2,3)						11760	1680
29		t1,2,5	Bisteritruncated 7-simplex (bacto)	(0,0,1,1,1,2,3,3)						9240	1680
30		t0,3,5	Pentiruncinated 7-simplex (tepo)	(0,0,1,1,2,2,2,3)						10920	1680
31		t1,3,5	Bistericantellated 7-simplex (bacroh)	(0,0,1,1,2,2,3,3)						15120	2520
32		t0,4,5	Pentistericated 7-simplex (teco)	(0,0,1,2,2,2,2,3)						4200	840
33		t0,1,6	Hexitruncated 7-simplex (puto)	(0,1,1,1,1,1,2,3)						1848	336
34		t0,2,6	Hexicantellated 7-simplex (puro)	(0,1,1,1,1,2,2,3)						5880	840
35		t0,3,6	Hexiruncinated 7-simplex (puph)	(0,1,1,1,2,2,2,3)						8400	1120
36		t0,1,2,3	Runcicantitruncated 7-simplex (gapo)	(0,0,0,0,1,2,3,4)						5880	1680
37		t0,1,2,4	Stericantitruncated 7-simplex (cagro)	(0,0,0,1,1,2,3,4)						16800	3360
38		t0,1,3,4	Steriruncitruncated 7-simplex (capto)	(0,0,0,1,2,2,3,4)						13440	3360
39		t0,2,3,4	Steriruncicantellated 7-simplex (capro)	(0,0,0,1,2,3,3,4)						13440	3360
40		t1,2,3,4	Biruncicantitruncated 7-simplex (gibpo)	(0,0,0,1,2,3,4,4)						11760	3360
41		t0,1,2,5	Penticantitruncated 7-simplex (tegro)	(0,0,1,1,1,2,3,4)						18480	3360
42		t0,1,3,5	Pentiruncitruncated 7-simplex (tapto)	(0,0,1,1,2,2,3,4)						27720	5040
43		t0,2,3,5	Pentiruncicantellated 7-simplex (tapro)	(0,0,1,1,2,3,3,4)						25200	5040
44		t1,2,3,5	Bistericantitruncated 7-simplex (bacogro)	(0,0,1,1,2,3,4,4)						22680	5040
45		t0,1,4,5	Pentisteritruncated 7-simplex (tecto)	(0,0,1,2,2,2,3,4)						15120	3360
46		t0,2,4,5	Pentistericantellated 7-simplex (tecro)	(0,0,1,2,2,3,3,4)						25200	5040
47		t1,2,4,5	Bisteriruncitruncated 7-simplex (bicpath)	(0,0,1,2,2,3,4,4)						20160	5040
48		t0,3,4,5	Pentisteriruncinated 7-simplex (tacpo)	(0,0,1,2,3,3,3,4)						15120	3360
49		t0,1,2,6	Hexicantitruncated 7-simplex (pugro)	(0,1,1,1,1,2,3,4)						8400	1680
50		t0,1,3,6	Hexiruncitruncated 7-simplex (pugato)	(0,1,1,1,2,2,3,4)						20160	3360
51		t0,2,3,6	Hexiruncicantellated 7-simplex (pugro)	(0,1,1,1,2,3,3,4)						16800	3360
52		t0,1,4,6	Hexisteritruncated 7-simplex (pucto)	(0,1,1,2,2,2,3,4)						20160	3360
53		t0,2,4,6	Hexistericantellated 7-simplex (pucroh)	(0,1,1,2,2,3,3,4)						30240	5040
54		t0,1,5,6	Hexipentitruncated 7-simplex (putath)	(0,1,2,2,2,2,3,4)						8400	1680
55		t0,1,2,3,4	Steriruncicantitruncated 7-simplex (gecco)	(0,0,0,1,2,3,4,5)						23520	6720
56		t0,1,2,3,5	Pentiruncicantitruncated 7-simplex (tegapo)	(0,0,1,1,2,3,4,5)						45360	10080
57		t0,1,2,4,5	Pentistericantitruncated 7-simplex (tecagro)	(0,0,1,2,2,3,4,5)						40320	10080
58		t0,1,3,4,5	Pentisteriruncitruncated 7-simplex (tacpeto)	(0,0,1,2,3,3,4,5)						40320	10080
59		t0,2,3,4,5	Pentisteriruncicantellated 7-simplex (tacpro)	(0,0,1,2,3,4,4,5)						40320	10080
60		t1,2,3,4,5	Bisteriruncicantitruncated 7-simplex (gabach)	(0,0,1,2,3,4,5,5)						35280	10080
61		t0,1,2,3,6	Hexiruncicantitruncated 7-simplex (pugopo)	(0,1,1,1,2,3,4,5)						30240	6720
62		t0,1,2,4,6	Hexistericantitruncated 7-simplex (pucagro)	(0,1,1,2,2,3,4,5)						50400	10080
63		t0,1,3,4,6	Hexisteriruncitruncated 7-simplex (pucpato)	(0,1,1,2,3,3,4,5)						45360	10080
64		t0,2,3,4,6	Hexisteriruncicantellated 7-simplex (pucproh)	(0,1,1,2,3,4,4,5)						45360	10080
65		t0,1,2,5,6	Hexipenticantitruncated 7-simplex (putagro)	(0,1,2,2,2,3,4,5)						30240	6720
66		t0,1,3,5,6	Hexipentiruncitruncated 7-simplex (putpath)	(0,1,2,2,3,3,4,5)						50400	10080
67		t0,1,2,3,4,5	Pentisteriruncicantitruncated 7-simplex (geto)	(0,0,1,2,3,4,5,6)						70560	20160
68		t0,1,2,3,4,6	Hexisteriruncicantitruncated 7-simplex (pugaco)	(0,1,1,2,3,4,5,6)						80640	20160
69		t0,1,2,3,5,6	Hexipentiruncicantitruncated 7-simplex (putgapo)	(0,1,2,2,3,4,5,6)						80640	20160
70		t0,1,2,4,5,6	Hexipentistericantitruncated 7-simplex (putcagroh)	(0,1,2,3,3,4,5,6)						80640	20160
71		t0,1,2,3,4,5,6	Omnitruncated 7-simplex (guph)	(0,1,2,3,4,5,6,7)						141120	40320

The B₇ family

The B₇ family has symmetry of order 645120 (7 factorial x 2⁷).

There are 127 forms based on all permutations of the Coxeter-Dynkin diagrams with one or more rings. Johnson and Bowers names.

See also a list of B7 polytopes for symmetric Coxeter plane graphs of these polytopes.

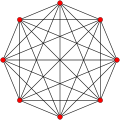
B7 uniform polytopes
#	Coxeter-Dynkin diagram t-notation	Name (BSA)	Base point	Element counts
				6	5	4	3	2	1	0
1	t0{3,3,3,3,3,4}	7-orthoplex (zee)	(0,0,0,0,0,0,1)√2	128	448	672	560	280	84	14
2	t1{3,3,3,3,3,4}	Rectified 7-orthoplex (rez)	(0,0,0,0,0,1,1)√2	142	1344	3360	3920	2520	840	84
3	t2{3,3,3,3,3,4}	Birectified 7-orthoplex (barz)	(0,0,0,0,1,1,1)√2	142	1428	6048	10640	8960	3360	280
4	t3{4,3,3,3,3,3}	Trirectified 7-cube (sez)	(0,0,0,1,1,1,1)√2	142	1428	6328	14560	15680	6720	560
5	t2{4,3,3,3,3,3}	Birectified 7-cube (bersa)	(0,0,1,1,1,1,1)√2	142	1428	5656	11760	13440	6720	672
6	t1{4,3,3,3,3,3}	Rectified 7-cube (rasa)	(0,1,1,1,1,1,1)√2	142	980	2968	5040	5152	2688	448
7	t0{4,3,3,3,3,3}	7-cube (hept)	(0,0,0,0,0,0,0)√2 + (1,1,1,1,1,1,1)	14	84	280	560	672	448	128
8	t0,1{3,3,3,3,3,4}	Truncated 7-orthoplex (Taz)	(0,0,0,0,0,1,2)√2	142	1344	3360	4760	2520	924	168
9	t0,2{3,3,3,3,3,4}	Cantellated 7-orthoplex (Sarz)	(0,0,0,0,1,1,2)√2	226	4200	15456	24080	19320	7560	840
10	t1,2{3,3,3,3,3,4}	Bitruncated 7-orthoplex (Botaz)	(0,0,0,0,1,2,2)√2						4200	840
11	t0,3{3,3,3,3,3,4}	Runcinated 7-orthoplex (Spaz)	(0,0,0,1,1,1,2)√2						23520	2240
12	t1,3{3,3,3,3,3,4}	Bicantellated 7-orthoplex (Sebraz)	(0,0,0,1,1,2,2)√2						26880	3360
13	t2,3{3,3,3,3,3,4}	Tritruncated 7-orthoplex (Totaz)	(0,0,0,1,2,2,2)√2						10080	2240
14	t0,4{3,3,3,3,3,4}	Stericated 7-orthoplex (Scaz)	(0,0,1,1,1,1,2)√2						33600	3360
15	t1,4{3,3,3,3,3,4}	Biruncinated 7-orthoplex (Sibpaz)	(0,0,1,1,1,2,2)√2						60480	6720
16	t2,4{4,3,3,3,3,3}	Tricantellated 7-cube (Strasaz)	(0,0,1,1,2,2,2)√2						47040	6720
17	t2,3{4,3,3,3,3,3}	Tritruncated 7-cube (Tatsa)	(0,0,1,2,2,2,2)√2						13440	3360
18	t0,5{3,3,3,3,3,4}	Pentellated 7-orthoplex (Staz)	(0,1,1,1,1,1,2)√2						20160	2688
19	t1,5{4,3,3,3,3,3}	Bistericated 7-cube (Sabcosaz)	(0,1,1,1,1,2,2)√2						53760	6720
20	t1,4{4,3,3,3,3,3}	Biruncinated 7-cube (Sibposa)	(0,1,1,1,2,2,2)√2						67200	8960
21	t1,3{4,3,3,3,3,3}	Bicantellated 7-cube (Sibrosa)	(0,1,1,2,2,2,2)√2						40320	6720
22	t1,2{4,3,3,3,3,3}	Bitruncated 7-cube (Betsa)	(0,1,2,2,2,2,2)√2						9408	2688
23	t0,6{4,3,3,3,3,3}	Hexicated 7-cube (Supposaz)	(0,0,0,0,0,0,1)√2 + (1,1,1,1,1,1,1)						5376	896
24	t0,5{4,3,3,3,3,3}	Pentellated 7-cube (Stesa)	(0,0,0,0,0,1,1)√2 + (1,1,1,1,1,1,1)						20160	2688
25	t0,4{4,3,3,3,3,3}	Stericated 7-cube (Scosa)	(0,0,0,0,1,1,1)√2 + (1,1,1,1,1,1,1)						35840	4480
26	t0,3{4,3,3,3,3,3}	Runcinated 7-cube (Spesa)	(0,0,0,1,1,1,1)√2 + (1,1,1,1,1,1,1)						33600	4480
27	t0,2{4,3,3,3,3,3}	Cantellated 7-cube (Sersa)	(0,0,1,1,1,1,1)√2 + (1,1,1,1,1,1,1)						16128	2688
28	t0,1{4,3,3,3,3,3}	Truncated 7-cube (Tasa)	(0,1,1,1,1,1,1)√2 + (1,1,1,1,1,1,1)	142	980	2968	5040	5152	3136	896
29	t0,1,2{3,3,3,3,3,4}	Cantitruncated 7-orthoplex (Garz)	(0,1,2,3,3,3,3)√2						8400	1680
30	t0,1,3{3,3,3,3,3,4}	Runcitruncated 7-orthoplex (Potaz)	(0,1,2,2,3,3,3)√2						50400	6720
31	t0,2,3{3,3,3,3,3,4}	Runcicantellated 7-orthoplex (Parz)	(0,1,1,2,3,3,3)√2						33600	6720
32	t1,2,3{3,3,3,3,3,4}	Bicantitruncated 7-orthoplex (Gebraz)	(0,0,1,2,3,3,3)√2						30240	6720
33	t0,1,4{3,3,3,3,3,4}	Steritruncated 7-orthoplex (Catz)	(0,0,1,1,1,2,3)√2						107520	13440
34	t0,2,4{3,3,3,3,3,4}	Stericantellated 7-orthoplex (Craze)	(0,0,1,1,2,2,3)√2						141120	20160
35	t1,2,4{3,3,3,3,3,4}	Biruncitruncated 7-orthoplex (Baptize)	(0,0,1,1,2,3,3)√2						120960	20160
36	t0,3,4{3,3,3,3,3,4}	Steriruncinated 7-orthoplex (Copaz)	(0,1,1,1,2,3,3)√2						67200	13440
37	t1,3,4{3,3,3,3,3,4}	Biruncicantellated 7-orthoplex (Boparz)	(0,0,1,2,2,3,3)√2						100800	20160
38	t2,3,4{4,3,3,3,3,3}	Tricantitruncated 7-cube (Gotrasaz)	(0,0,0,1,2,3,3)√2						53760	13440
39	t0,1,5{3,3,3,3,3,4}	Pentitruncated 7-orthoplex (Tetaz)	(0,1,1,1,1,2,3)√2						87360	13440
40	t0,2,5{3,3,3,3,3,4}	Penticantellated 7-orthoplex (Teroz)	(0,1,1,1,2,2,3)√2						188160	26880
41	t1,2,5{3,3,3,3,3,4}	Bisteritruncated 7-orthoplex (Boctaz)	(0,1,1,1,2,3,3)√2						147840	26880
42	t0,3,5{3,3,3,3,3,4}	Pentiruncinated 7-orthoplex (Topaz)	(0,1,1,2,2,2,3)√2						174720	26880
43	t1,3,5{4,3,3,3,3,3}	Bistericantellated 7-cube (Bacresaz)	(0,1,1,2,2,3,3)√2						241920	40320
44	t1,3,4{4,3,3,3,3,3}	Biruncicantellated 7-cube (Bopresa)	(0,1,1,2,3,3,3)√2						120960	26880
45	t0,4,5{3,3,3,3,3,4}	Pentistericated 7-orthoplex (Tocaz)	(0,1,2,2,2,2,3)√2						67200	13440
46	t1,2,5{4,3,3,3,3,3}	Bisteritruncated 7-cube (Bactasa)	(0,1,2,2,2,3,3)√2						147840	26880
47	t1,2,4{4,3,3,3,3,3}	Biruncitruncated 7-cube (Biptesa)	(0,1,2,2,3,3,3)√2						134400	26880
48	t1,2,3{4,3,3,3,3,3}	Bicantitruncated 7-cube (Gibrosa)	(0,1,2,3,3,3,3)√2						47040	13440
49	t0,1,6{3,3,3,3,3,4}	Hexitruncated 7-orthoplex (Putaz)	(0,0,0,0,0,1,2)√2 + (1,1,1,1,1,1,1)						29568	5376
50	t0,2,6{3,3,3,3,3,4}	Hexicantellated 7-orthoplex (Puraz)	(0,0,0,0,1,1,2)√2 + (1,1,1,1,1,1,1)						94080	13440
51	t0,4,5{4,3,3,3,3,3}	Pentistericated 7-cube (Tacosa)	(0,0,0,0,1,2,2)√2 + (1,1,1,1,1,1,1)						67200	13440
52	t0,3,6{4,3,3,3,3,3}	Hexiruncinated 7-cube (Pupsez)	(0,0,0,1,1,1,2)√2 + (1,1,1,1,1,1,1)						134400	17920
53	t0,3,5{4,3,3,3,3,3}	Pentiruncinated 7-cube (Tapsa)	(0,0,0,1,1,2,2)√2 + (1,1,1,1,1,1,1)						174720	26880
54	t0,3,4{4,3,3,3,3,3}	Steriruncinated 7-cube (Capsa)	(0,0,0,1,2,2,2)√2 + (1,1,1,1,1,1,1)						80640	17920
55	t0,2,6{4,3,3,3,3,3}	Hexicantellated 7-cube (Purosa)	(0,0,1,1,1,1,2)√2 + (1,1,1,1,1,1,1)						94080	13440
56	t0,2,5{4,3,3,3,3,3}	Penticantellated 7-cube (Tersa)	(0,0,1,1,1,2,2)√2 + (1,1,1,1,1,1,1)						188160	26880
57	t0,2,4{4,3,3,3,3,3}	Stericantellated 7-cube (Carsa)	(0,0,1,1,2,2,2)√2 + (1,1,1,1,1,1,1)						161280	26880
58	t0,2,3{4,3,3,3,3,3}	Runcicantellated 7-cube (Parsa)	(0,0,1,2,2,2,2)√2 + (1,1,1,1,1,1,1)						53760	13440
59	t0,1,6{4,3,3,3,3,3}	Hexitruncated 7-cube (Putsa)	(0,1,1,1,1,1,2)√2 + (1,1,1,1,1,1,1)						29568	5376
60	t0,1,5{4,3,3,3,3,3}	Pentitruncated 7-cube (Tetsa)	(0,1,1,1,1,2,2)√2 + (1,1,1,1,1,1,1)						87360	13440
61	t0,1,4{4,3,3,3,3,3}	Steritruncated 7-cube (Catsa)	(0,1,1,1,2,2,2)√2 + (1,1,1,1,1,1,1)						116480	17920
62	t0,1,3{4,3,3,3,3,3}	Runcitruncated 7-cube (Petsa)	(0,1,1,2,2,2,2)√2 + (1,1,1,1,1,1,1)						73920	13440
63	t0,1,2{4,3,3,3,3,3}	Cantitruncated 7-cube (Gersa)	(0,1,2,2,2,2,2)√2 + (1,1,1,1,1,1,1)						18816	5376
64	t0,1,2,3{3,3,3,3,3,4}	Runcicantitruncated 7-orthoplex (Gopaz)	(0,1,2,3,4,4,4)√2						60480	13440
65	t0,1,2,4{3,3,3,3,3,4}	Stericantitruncated 7-orthoplex (Cogarz)	(0,0,1,1,2,3,4)√2						241920	40320
66	t0,1,3,4{3,3,3,3,3,4}	Steriruncitruncated 7-orthoplex (Captaz)	(0,0,1,2,2,3,4)√2						181440	40320
67	t0,2,3,4{3,3,3,3,3,4}	Steriruncicantellated 7-orthoplex (Caparz)	(0,0,1,2,3,3,4)√2						181440	40320
68	t1,2,3,4{3,3,3,3,3,4}	Biruncicantitruncated 7-orthoplex (Gibpaz)	(0,0,1,2,3,4,4)√2						161280	40320
69	t0,1,2,5{3,3,3,3,3,4}	Penticantitruncated 7-orthoplex (Tograz)	(0,1,1,1,2,3,4)√2						295680	53760
70	t0,1,3,5{3,3,3,3,3,4}	Pentiruncitruncated 7-orthoplex (Toptaz)	(0,1,1,2,2,3,4)√2						443520	80640
71	t0,2,3,5{3,3,3,3,3,4}	Pentiruncicantellated 7-orthoplex (Toparz)	(0,1,1,2,3,3,4)√2						403200	80640
72	t1,2,3,5{3,3,3,3,3,4}	Bistericantitruncated 7-orthoplex (Becogarz)	(0,1,1,2,3,4,4)√2						362880	80640
73	t0,1,4,5{3,3,3,3,3,4}	Pentisteritruncated 7-orthoplex (Tacotaz)	(0,1,2,2,2,3,4)√2						241920	53760
74	t0,2,4,5{3,3,3,3,3,4}	Pentistericantellated 7-orthoplex (Tocarz)	(0,1,2,2,3,3,4)√2						403200	80640
75	t1,2,4,5{4,3,3,3,3,3}	Bisteriruncitruncated 7-cube (Bocaptosaz)	(0,1,2,2,3,4,4)√2						322560	80640
76	t0,3,4,5{3,3,3,3,3,4}	Pentisteriruncinated 7-orthoplex (Tecpaz)	(0,1,2,3,3,3,4)√2						241920	53760
77	t1,2,3,5{4,3,3,3,3,3}	Bistericantitruncated 7-cube (Becgresa)	(0,1,2,3,3,4,4)√2						362880	80640
78	t1,2,3,4{4,3,3,3,3,3}	Biruncicantitruncated 7-cube (Gibposa)	(0,1,2,3,4,4,4)√2						188160	53760
79	t0,1,2,6{3,3,3,3,3,4}	Hexicantitruncated 7-orthoplex (Pugarez)	(0,0,0,0,1,2,3)√2 + (1,1,1,1,1,1,1)						134400	26880
80	t0,1,3,6{3,3,3,3,3,4}	Hexiruncitruncated 7-orthoplex (Papataz)	(0,0,0,1,1,2,3)√2 + (1,1,1,1,1,1,1)						322560	53760
81	t0,2,3,6{3,3,3,3,3,4}	Hexiruncicantellated 7-orthoplex (Puparez)	(0,0,0,1,2,2,3)√2 + (1,1,1,1,1,1,1)						268800	53760
82	t0,3,4,5{4,3,3,3,3,3}	Pentisteriruncinated 7-cube (Tecpasa)	(0,0,0,1,2,3,3)√2 + (1,1,1,1,1,1,1)						241920	53760
83	t0,1,4,6{3,3,3,3,3,4}	Hexisteritruncated 7-orthoplex (Pucotaz)	(0,0,1,1,1,2,3)√2 + (1,1,1,1,1,1,1)						322560	53760
84	t0,2,4,6{4,3,3,3,3,3}	Hexistericantellated 7-cube (Pucrosaz)	(0,0,1,1,2,2,3)√2 + (1,1,1,1,1,1,1)						483840	80640
85	t0,2,4,5{4,3,3,3,3,3}	Pentistericantellated 7-cube (Tecresa)	(0,0,1,1,2,3,3)√2 + (1,1,1,1,1,1,1)						403200	80640
86	t0,2,3,6{4,3,3,3,3,3}	Hexiruncicantellated 7-cube (Pupresa)	(0,0,1,2,2,2,3)√2 + (1,1,1,1,1,1,1)						268800	53760
87	t0,2,3,5{4,3,3,3,3,3}	Pentiruncicantellated 7-cube (Topresa)	(0,0,1,2,2,3,3)√2 + (1,1,1,1,1,1,1)						403200	80640
88	t0,2,3,4{4,3,3,3,3,3}	Steriruncicantellated 7-cube (Copresa)	(0,0,1,2,3,3,3)√2 + (1,1,1,1,1,1,1)						215040	53760
89	t0,1,5,6{4,3,3,3,3,3}	Hexipentitruncated 7-cube (Putatosez)	(0,1,1,1,1,2,3)√2 + (1,1,1,1,1,1,1)						134400	26880
90	t0,1,4,6{4,3,3,3,3,3}	Hexisteritruncated 7-cube (Pacutsa)	(0,1,1,1,2,2,3)√2 + (1,1,1,1,1,1,1)						322560	53760
91	t0,1,4,5{4,3,3,3,3,3}	Pentisteritruncated 7-cube (Tecatsa)	(0,1,1,1,2,3,3)√2 + (1,1,1,1,1,1,1)						241920	53760
92	t0,1,3,6{4,3,3,3,3,3}	Hexiruncitruncated 7-cube (Pupetsa)	(0,1,1,2,2,2,3)√2 + (1,1,1,1,1,1,1)						322560	53760
93	t0,1,3,5{4,3,3,3,3,3}	Pentiruncitruncated 7-cube (Toptosa)	(0,1,1,2,2,3,3)√2 + (1,1,1,1,1,1,1)						443520	80640
94	t0,1,3,4{4,3,3,3,3,3}	Steriruncitruncated 7-cube (Captesa)	(0,1,1,2,3,3,3)√2 + (1,1,1,1,1,1,1)						215040	53760
95	t0,1,2,6{4,3,3,3,3,3}	Hexicantitruncated 7-cube (Pugrosa)	(0,1,2,2,2,2,3)√2 + (1,1,1,1,1,1,1)						134400	26880
96	t0,1,2,5{4,3,3,3,3,3}	Penticantitruncated 7-cube (Togresa)	(0,1,2,2,2,3,3)√2 + (1,1,1,1,1,1,1)						295680	53760
97	t0,1,2,4{4,3,3,3,3,3}	Stericantitruncated 7-cube (Cogarsa)	(0,1,2,2,3,3,3)√2 + (1,1,1,1,1,1,1)						268800	53760
98	t0,1,2,3{4,3,3,3,3,3}	Runcicantitruncated 7-cube (Gapsa)	(0,1,2,3,3,3,3)√2 + (1,1,1,1,1,1,1)						94080	26880
99	t0,1,2,3,4{3,3,3,3,3,4}	Steriruncicantitruncated 7-orthoplex (Gocaz)	(0,0,1,2,3,4,5)√2						322560	80640
100	t0,1,2,3,5{3,3,3,3,3,4}	Pentiruncicantitruncated 7-orthoplex (Tegopaz)	(0,1,1,2,3,4,5)√2						725760	161280
101	t0,1,2,4,5{3,3,3,3,3,4}	Pentistericantitruncated 7-orthoplex (Tecagraz)	(0,1,2,2,3,4,5)√2						645120	161280
102	t0,1,3,4,5{3,3,3,3,3,4}	Pentisteriruncitruncated 7-orthoplex (Tecpotaz)	(0,1,2,3,3,4,5)√2						645120	161280
103	t0,2,3,4,5{3,3,3,3,3,4}	Pentisteriruncicantellated 7-orthoplex (Tacparez)	(0,1,2,3,4,4,5)√2						645120	161280
104	t1,2,3,4,5{4,3,3,3,3,3}	Bisteriruncicantitruncated 7-cube (Gabcosaz)	(0,1,2,3,4,5,5)√2						564480	161280
105	t0,1,2,3,6{3,3,3,3,3,4}	Hexiruncicantitruncated 7-orthoplex (Pugopaz)	(0,0,0,1,2,3,4)√2 + (1,1,1,1,1,1,1)						483840	107520
106	t0,1,2,4,6{3,3,3,3,3,4}	Hexistericantitruncated 7-orthoplex (Pucagraz)	(0,0,1,1,2,3,4)√2 + (1,1,1,1,1,1,1)						806400	161280
107	t0,1,3,4,6{3,3,3,3,3,4}	Hexisteriruncitruncated 7-orthoplex (Pucpotaz)	(0,0,1,2,2,3,4)√2 + (1,1,1,1,1,1,1)						725760	161280
108	t0,2,3,4,6{4,3,3,3,3,3}	Hexisteriruncicantellated 7-cube (Pucprosaz)	(0,0,1,2,3,3,4)√2 + (1,1,1,1,1,1,1)						725760	161280
109	t0,2,3,4,5{4,3,3,3,3,3}	Pentisteriruncicantellated 7-cube (Tocpresa)	(0,0,1,2,3,4,4)√2 + (1,1,1,1,1,1,1)						645120	161280
110	t0,1,2,5,6{3,3,3,3,3,4}	Hexipenticantitruncated 7-orthoplex (Putegraz)	(0,1,1,1,2,3,4)√2 + (1,1,1,1,1,1,1)						483840	107520
111	t0,1,3,5,6{4,3,3,3,3,3}	Hexipentiruncitruncated 7-cube (Putpetsaz)	(0,1,1,2,2,3,4)√2 + (1,1,1,1,1,1,1)						806400	161280
112	t0,1,3,4,6{4,3,3,3,3,3}	Hexisteriruncitruncated 7-cube (Pucpetsa)	(0,1,1,2,3,3,4)√2 + (1,1,1,1,1,1,1)						725760	161280
113	t0,1,3,4,5{4,3,3,3,3,3}	Pentisteriruncitruncated 7-cube (Tecpetsa)	(0,1,1,2,3,4,4)√2 + (1,1,1,1,1,1,1)						645120	161280
114	t0,1,2,5,6{4,3,3,3,3,3}	Hexipenticantitruncated 7-cube (Putgresa)	(0,1,2,2,2,3,4)√2 + (1,1,1,1,1,1,1)						483840	107520
115	t0,1,2,4,6{4,3,3,3,3,3}	Hexistericantitruncated 7-cube (Pucagrosa)	(0,1,2,2,3,3,4)√2 + (1,1,1,1,1,1,1)						806400	161280
116	t0,1,2,4,5{4,3,3,3,3,3}	Pentistericantitruncated 7-cube (Tecgresa)	(0,1,2,2,3,4,4)√2 + (1,1,1,1,1,1,1)						645120	161280
117	t0,1,2,3,6{4,3,3,3,3,3}	Hexiruncicantitruncated 7-cube (Pugopsa)	(0,1,2,3,3,3,4)√2 + (1,1,1,1,1,1,1)						483840	107520
118	t0,1,2,3,5{4,3,3,3,3,3}	Pentiruncicantitruncated 7-cube (Togapsa)	(0,1,2,3,3,4,4)√2 + (1,1,1,1,1,1,1)						725760	161280
119	t0,1,2,3,4{4,3,3,3,3,3}	Steriruncicantitruncated 7-cube (Gacosa)	(0,1,2,3,4,4,4)√2 + (1,1,1,1,1,1,1)						376320	107520
120	t0,1,2,3,4,5{3,3,3,3,3,4}	Pentisteriruncicantitruncated 7-orthoplex (Gotaz)	(0,1,2,3,4,5,6)√2						1128960	322560
121	t0,1,2,3,4,6{3,3,3,3,3,4}	Hexisteriruncicantitruncated 7-orthoplex (Pugacaz)	(0,0,1,2,3,4,5)√2 + (1,1,1,1,1,1,1)						1290240	322560
122	t0,1,2,3,5,6{3,3,3,3,3,4}	Hexipentiruncicantitruncated 7-orthoplex (Putgapaz)	(0,1,1,2,3,4,5)√2 + (1,1,1,1,1,1,1)						1290240	322560
123	t0,1,2,4,5,6{4,3,3,3,3,3}	Hexipentistericantitruncated 7-cube (Putcagrasaz)	(0,1,2,2,3,4,5)√2 + (1,1,1,1,1,1,1)						1290240	322560
124	t0,1,2,3,5,6{4,3,3,3,3,3}	Hexipentiruncicantitruncated 7-cube (Putgapsa)	(0,1,2,3,3,4,5)√2 + (1,1,1,1,1,1,1)						1290240	322560
125	t0,1,2,3,4,6{4,3,3,3,3,3}	Hexisteriruncicantitruncated 7-cube (Pugacasa)	(0,1,2,3,4,4,5)√2 + (1,1,1,1,1,1,1)						1290240	322560
126	t0,1,2,3,4,5{4,3,3,3,3,3}	Pentisteriruncicantitruncated 7-cube (Gotesa)	(0,1,2,3,4,5,5)√2 + (1,1,1,1,1,1,1)						1128960	322560
127	t0,1,2,3,4,5,6{4,3,3,3,3,3}	Omnitruncated 7-cube (Guposaz)	(0,1,2,3,4,5,6)√2 + (1,1,1,1,1,1,1)						2257920	645120

The D₇ family

The D₇ family has symmetry of order 322560 (7 factorial x 2⁶).

This family has 3×32−1=95 Wythoffian uniform polytopes, generated by marking one or more nodes of the D₇ Coxeter-Dynkin diagram. Of these, 63 (2×32−1) are repeated from the B₇ family and 32 are unique to this family, listed below. Bowers names and acronym are given for cross-referencing.

See also list of D7 polytopes for Coxeter plane graphs of these polytopes.

D7 uniform polytopes
#	Coxeter diagram	Names	Base point (Alternately signed)	Element counts
				6	5	4	3	2	1	0
1	=	7-cube demihepteract (hesa)	(1,1,1,1,1,1,1)	78	532	1624	2800	2240	672	64
2	=	cantic 7-cube truncated demihepteract (thesa)	(1,1,3,3,3,3,3)	142	1428	5656	11760	13440	7392	1344
3	=	runcic 7-cube small rhombated demihepteract (sirhesa)	(1,1,1,3,3,3,3)						16800	2240
4	=	steric 7-cube small prismated demihepteract (sphosa)	(1,1,1,1,3,3,3)						20160	2240
5	=	pentic 7-cube small cellated demihepteract (sochesa)	(1,1,1,1,1,3,3)						13440	1344
6	=	hexic 7-cube small terated demihepteract (suthesa)	(1,1,1,1,1,1,3)						4704	448
7	=	runcicantic 7-cube great rhombated demihepteract (Girhesa)	(1,1,3,5,5,5,5)						23520	6720
8	=	stericantic 7-cube prismatotruncated demihepteract (pothesa)	(1,1,3,3,5,5,5)						73920	13440
9	=	steriruncic 7-cube prismatorhomated demihepteract (prohesa)	(1,1,1,3,5,5,5)						40320	8960
10	=	penticantic 7-cube cellitruncated demihepteract (cothesa)	(1,1,3,3,3,5,5)						87360	13440
11	=	pentiruncic 7-cube cellirhombated demihepteract (crohesa)	(1,1,1,3,3,5,5)						87360	13440
12	=	pentisteric 7-cube celliprismated demihepteract (caphesa)	(1,1,1,1,3,5,5)						40320	6720
13	=	hexicantic 7-cube tericantic demihepteract (tuthesa)	(1,1,3,3,3,3,5)						43680	6720
14	=	hexiruncic 7-cube terirhombated demihepteract (turhesa)	(1,1,1,3,3,3,5)						67200	8960
15	=	hexisteric 7-cube teriprismated demihepteract (tuphesa)	(1,1,1,1,3,3,5)						53760	6720
16	=	hexipentic 7-cube tericellated demihepteract (tuchesa)	(1,1,1,1,1,3,5)						21504	2688
17	=	steriruncicantic 7-cube great prismated demihepteract (Gephosa)	(1,1,3,5,7,7,7)						94080	26880
18	=	pentiruncicantic 7-cube celligreatorhombated demihepteract (cagrohesa)	(1,1,3,5,5,7,7)						181440	40320
19	=	pentistericantic 7-cube celliprismatotruncated demihepteract (capthesa)	(1,1,3,3,5,7,7)						181440	40320
20	=	pentisteriruncic 7-cube celliprismatorhombated demihepteract (coprahesa)	(1,1,1,3,5,7,7)						120960	26880
21	=	hexiruncicantic 7-cube terigreatorhombated demihepteract (tugrohesa)	(1,1,3,5,5,5,7)						120960	26880
22	=	hexistericantic 7-cube teriprismatotruncated demihepteract (tupthesa)	(1,1,3,3,5,5,7)						221760	40320
23	=	hexisteriruncic 7-cube teriprismatorhombated demihepteract (tuprohesa)	(1,1,1,3,5,5,7)						134400	26880
24	=	hexipenticantic 7-cube teriCellitruncated demihepteract (tucothesa)	(1,1,3,3,3,5,7)						147840	26880
25	=	hexipentiruncic 7-cube tericellirhombated demihepteract (tucrohesa)	(1,1,1,3,3,5,7)						161280	26880
26	=	hexipentisteric 7-cube tericelliprismated demihepteract (tucophesa)	(1,1,1,1,3,5,7)						80640	13440
27	=	pentisteriruncicantic 7-cube great cellated demihepteract (gochesa)	(1,1,3,5,7,9,9)						282240	80640
28	=	hexisteriruncicantic 7-cube terigreatoprimated demihepteract (tugphesa)	(1,1,3,5,7,7,9)						322560	80640
29	=	hexipentiruncicantic 7-cube tericelligreatorhombated demihepteract (tucagrohesa)	(1,1,3,5,5,7,9)						322560	80640
30	=	hexipentistericantic 7-cube tericelliprismatotruncated demihepteract (tucpathesa)	(1,1,3,3,5,7,9)						362880	80640
31	=	hexipentisteriruncic 7-cube tericellprismatorhombated demihepteract (tucprohesa)	(1,1,1,3,5,7,9)						241920	53760
32	=	hexipentisteriruncicantic 7-cube great terated demihepteract (guthesa)	(1,1,3,5,7,9,11)						564480	161280

The E₇ family

The E₇ Coxeter group has order 2,903,040.

There are 127 forms based on all permutations of the Coxeter-Dynkin diagrams with one or more rings.

See also a list of E7 polytopes for symmetric Coxeter plane graphs of these polytopes.

E7 uniform polytopes
#	Coxeter-Dynkin diagram Schläfli symbol	Names	Element counts
			6	5	4	3	2	1	0
1		231 (laq)	632	4788	16128	20160	10080	2016	126
2		Rectified 231 (rolaq)	758	10332	47880	100800	90720	30240	2016
3		Rectified 132 (rolin)	758	12348	72072	191520	241920	120960	10080
4		132 (lin)	182	4284	23688	50400	40320	10080	576
5		Birectified 321 (branq)	758	12348	68040	161280	161280	60480	4032
6		Rectified 321 (ranq)	758	44352	70560	48384	11592	12096	756
7		321 (naq)	702	6048	12096	10080	4032	756	56
8		Truncated 231 (talq)	758	10332	47880	100800	90720	32256	4032
9		Cantellated 231 (sirlaq)						131040	20160
10		Bitruncated 231 (botlaq)							30240
11		small demified 231 (shilq)	2774	22428	78120	151200	131040	42336	4032
12		demirectified 231 (hirlaq)							12096
13		truncated 132 (tolin)							20160
14		small demiprismated 231 (shiplaq)							20160
15		birectified 132 (berlin)	758	22428	142632	403200	544320	302400	40320
16		tritruncated 321 (totanq)							40320
17		demibirectified 321 (hobranq)							20160
18		small cellated 231 (scalq)							7560
19		small biprismated 231 (sobpalq)							30240
20		small birhombated 321 (sabranq)							60480
21		demirectified 321 (harnaq)							12096
22		bitruncated 321 (botnaq)							12096
23		small terated 321 (stanq)							1512
24		small demicellated 321 (shocanq)							12096
25		small prismated 321 (spanq)							40320
26		small demified 321 (shanq)							4032
27		small rhombated 321 (sranq)							12096
28		Truncated 321 (tanq)	758	11592	48384	70560	44352	12852	1512
29		great rhombated 231 (girlaq)							60480
30		demitruncated 231 (hotlaq)							24192
31		small demirhombated 231 (sherlaq)							60480
32		demibitruncated 231 (hobtalq)							60480
33		demiprismated 231 (hiptalq)							80640
34		demiprismatorhombated 231 (hiprolaq)							120960
35		bitruncated 132 (batlin)							120960
36		small prismated 231 (spalq)							80640
37		small rhombated 132 (sirlin)							120960
38		tritruncated 231 (tatilq)							80640
39		cellitruncated 231 (catalaq)							60480
40		cellirhombated 231 (crilq)							362880
41		biprismatotruncated 231 (biptalq)							181440
42		small prismated 132 (seplin)							60480
43		small biprismated 321 (sabipnaq)							120960
44		small demibirhombated 321 (shobranq)							120960
45		cellidemiprismated 231 (chaplaq)							60480
46		demibiprismatotruncated 321 (hobpotanq)							120960
47		great birhombated 321 (gobranq)							120960
48		demibitruncated 321 (hobtanq)							60480
49		teritruncated 231 (totalq)							24192
50		terirhombated 231 (trilq)							120960
51		demicelliprismated 321 (hicpanq)							120960
52		small teridemified 231 (sethalq)							24192
53		small cellated 321 (scanq)							60480
54		demiprismated 321 (hipnaq)							80640
55		terirhombated 321 (tranq)							60480
56		demicellirhombated 321 (hocranq)							120960
57		prismatorhombated 321 (pranq)							120960
58		small demirhombated 321 (sharnaq)							60480
59		teritruncated 321 (tetanq)							15120
60		demicellitruncated 321 (hictanq)							60480
61		prismatotruncated 321 (potanq)							120960
62		demitruncated 321 (hotnaq)							24192
63		great rhombated 321 (granq)							24192
64		great demified 231 (gahlaq)							120960
65		great demiprismated 231 (gahplaq)							241920
66		prismatotruncated 231 (potlaq)							241920
67		prismatorhombated 231 (prolaq)							241920
68		great rhombated 132 (girlin)							241920
69		celligreatorhombated 231 (cagrilq)							362880
70		cellidemitruncated 231 (chotalq)							241920
71		prismatotruncated 132 (patlin)							362880
72		biprismatorhombated 321 (bipirnaq)							362880
73		tritruncated 132 (tatlin)							241920
74		cellidemiprismatorhombated 231 (chopralq)							362880
75		great demibiprismated 321 (ghobipnaq)							362880
76		celliprismated 231 (caplaq)							241920
77		biprismatotruncated 321 (boptanq)							362880
78		great trirhombated 231 (gatralaq)							241920
79		terigreatorhombated 231 (togrilq)							241920
80		teridemitruncated 231 (thotalq)							120960
81		teridemirhombated 231 (thorlaq)							241920
82		celliprismated 321 (capnaq)							241920
83		teridemiprismatotruncated 231 (thoptalq)							241920
84		teriprismatorhombated 321 (tapronaq)							362880
85		demicelliprismatorhombated 321 (hacpranq)							362880
86		teriprismated 231 (toplaq)							241920
87		cellirhombated 321 (cranq)							362880
88		demiprismatorhombated 321 (hapranq)							241920
89		tericellitruncated 231 (tectalq)							120960
90		teriprismatotruncated 321 (toptanq)							362880
91		demicelliprismatotruncated 321 (hecpotanq)							362880
92		teridemitruncated 321 (thotanq)							120960
93		cellitruncated 321 (catnaq)							241920
94		demiprismatotruncated 321 (hiptanq)							241920
95		terigreatorhombated 321 (tagranq)							120960
96		demicelligreatorhombated 321 (hicgarnq)							241920
97		great prismated 321 (gopanq)							241920
98		great demirhombated 321 (gahranq)							120960
99		great prismated 231 (gopalq)							483840
100		great cellidemified 231 (gechalq)							725760
101		great birhombated 132 (gebrolin)							725760
102		prismatorhombated 132 (prolin)							725760
103		celliprismatorhombated 231 (caprolaq)							725760
104		great biprismated 231 (gobpalq)							725760
105		tericelliprismated 321 (ticpanq)							483840
106		teridemigreatoprismated 231 (thegpalq)							725760
107		teriprismatotruncated 231 (teptalq)							725760
108		teriprismatorhombated 231 (topralq)							725760
109		cellipriemsatorhombated 321 (copranq)							725760
110		tericelligreatorhombated 231 (tecgrolaq)							725760
111		tericellitruncated 321 (tectanq)							483840
112		teridemiprismatotruncated 321 (thoptanq)							725760
113		celliprismatotruncated 321 (coptanq)							725760
114		teridemicelligreatorhombated 321 (thocgranq)							483840
115		terigreatoprismated 321 (tagpanq)							725760
116		great demicellated 321 (gahcnaq)							725760
117		tericelliprismated laq (tecpalq)							483840
118		celligreatorhombated 321 (cogranq)							725760
119		great demified 321 (gahnq)							483840
120		great cellated 231 (gocalq)							1451520
121		terigreatoprismated 231 (tegpalq)							1451520
122		tericelliprismatotruncated 321 (tecpotniq)							1451520
123		tericellidemigreatoprismated 231 (techogaplaq)							1451520
124		tericelligreatorhombated 321 (tacgarnq)							1451520
125		tericelliprismatorhombated 231 (tecprolaq)							1451520
126		great cellated 321 (gocanq)							1451520
127		great terated 321 (gotanq)							2903040

Regular and uniform honeycombs

Coxeter-Dynkin diagram correspondences between families and higher symmetry within diagrams. Nodes of the same color in each row represent identical mirrors. Black nodes are not active in the correspondence.

There are five fundamental affine Coxeter groups and sixteen prismatic groups that generate regular and uniform tessellations in 6-space:

#	Coxeter group		Coxeter diagram	Forms
1	${\displaystyle {\tilde {A}}_{6}}$	[3[7]]		17
2	${\displaystyle {\tilde {C}}_{6}}$	[4,34,4]		71
3	${\displaystyle {\tilde {B}}_{6}}$	h[4,34,4] [4,33,31,1]		95 (32 new)
4	${\displaystyle {\tilde {D}}_{6}}$	q[4,34,4] [31,1,32,31,1]		41 (6 new)
5	${\displaystyle {\tilde {E}}_{6}}$	[32,2,2]		39

Regular and uniform tessellations include:

• ${\displaystyle {\tilde {A}}_{6}}$, 17 forms
  • Uniform 6-simplex honeycomb: {3^[7]}
  • Uniform Cyclotruncated 6-simplex honeycomb: t_0,1{3^[7]}
  • Uniform Omnitruncated 6-simplex honeycomb: t_{0,1,2,3,4,5,6,7}{3^[7]}
• ${\displaystyle {\tilde {C}}_{6}}$, [4,3⁴,4], 71 forms
  • Regular 6-cube honeycomb, represented by symbols {4,3⁴,4},
• ${\displaystyle {\tilde {B}}_{6}}$, [3^1,1,3³,4], 95 forms, 64 shared with ${\displaystyle {\tilde {C}}_{6}}$, 32 new
  • Uniform 6-demicube honeycomb, represented by symbols h{4,3⁴,4} = {3^1,1,3³,4}, =
• ${\displaystyle {\tilde {D}}_{6}}$, [3^1,1,3²,3^1,1], 41 unique ringed permutations, most shared with ${\displaystyle {\tilde {B}}_{6}}$ and ${\displaystyle {\tilde {C}}_{6}}$, and 6 are new. Coxeter calls the first one a quarter 6-cubic honeycomb.
  • =
  • =
  • =
  • =
  • =
  • =
• ${\displaystyle {\tilde {E}}_{6}}$: [3^2,2,2], 39 forms
  • Uniform 2₂₂ honeycomb: represented by symbols {3,3,3^2,2},
  • Uniform t₄(2₂₂) honeycomb: 4r{3,3,3^2,2},
  • Uniform 0₂₂₂ honeycomb: {3^2,2,2},
  • Uniform t₂(0₂₂₂) honeycomb: 2r{3^2,2,2},

Prismatic groups

#	Coxeter group		Coxeter-Dynkin diagram
1	${\displaystyle {\tilde {A}}_{5}}$x${\displaystyle {\tilde {I}}_{1}}$	[3[6],2,∞]
2	${\displaystyle {\tilde {B}}_{5}}$x${\displaystyle {\tilde {I}}_{1}}$	[4,3,31,1,2,∞]
3	${\displaystyle {\tilde {C}}_{5}}$x${\displaystyle {\tilde {I}}_{1}}$	[4,33,4,2,∞]
4	${\displaystyle {\tilde {D}}_{5}}$x${\displaystyle {\tilde {I}}_{1}}$	[31,1,3,31,1,2,∞]
5	${\displaystyle {\tilde {A}}_{4}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[3[5],2,∞,2,∞,2,∞]
6	${\displaystyle {\tilde {B}}_{4}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[4,3,31,1,2,∞,2,∞]
7	${\displaystyle {\tilde {C}}_{4}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[4,3,3,4,2,∞,2,∞]
8	${\displaystyle {\tilde {D}}_{4}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[31,1,1,1,2,∞,2,∞]
9	${\displaystyle {\tilde {F}}_{4}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[3,4,3,3,2,∞,2,∞]
10	${\displaystyle {\tilde {C}}_{3}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[4,3,4,2,∞,2,∞,2,∞]
11	${\displaystyle {\tilde {B}}_{3}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[4,31,1,2,∞,2,∞,2,∞]
12	${\displaystyle {\tilde {A}}_{3}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[3[4],2,∞,2,∞,2,∞]
13	${\displaystyle {\tilde {C}}_{2}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[4,4,2,∞,2,∞,2,∞,2,∞]
14	${\displaystyle {\tilde {H}}_{2}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[6,3,2,∞,2,∞,2,∞,2,∞]
15	${\displaystyle {\tilde {A}}_{2}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[3[3],2,∞,2,∞,2,∞,2,∞]
16	${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$x${\displaystyle {\tilde {I}}_{1}}$	[∞,2,∞,2,∞,2,∞,2,∞]

Regular and uniform hyperbolic honeycombs

There are no compact hyperbolic Coxeter groups of rank 7, groups that can generate honeycombs with all finite facets, and a finite vertex figure. However, there are 3 paracompact hyperbolic Coxeter groups of rank 7, each generating uniform honeycombs in 6-space as permutations of rings of the Coxeter diagrams.

${\displaystyle {\bar {P}}_{6}}$ = [3,3[6]]:

${\displaystyle {\bar {Q}}_{6}}$ = [31,1,3,32,1]:

${\displaystyle {\bar {S}}_{6}}$ = [4,3,3,32,1]:

Notes on the Wythoff construction for the uniform 7-polytopes

The reflective 7-dimensional uniform polytopes are constructed through a Wythoff construction process, and represented by a Coxeter-Dynkin diagram, where each node represents a mirror. An active mirror is represented by a ringed node. Each combination of active mirrors generates a unique uniform polytope. Uniform polytopes are named in relation to the regular polytopes in each family. Some families have two regular constructors and thus may be named in two equally valid ways.

Here are the primary operators available for constructing and naming the uniform 7-polytopes.

The prismatic forms and bifurcating graphs can use the same truncation indexing notation, but require an explicit numbering system on the nodes for clarity.

Operation	Extended Schläfli symbol	Coxeter- Dynkin diagram	Description
Parent	t0{p,q,r,s,t,u}		Any regular 7-polytope
Rectified	t1{p,q,r,s,t,u}		The edges are fully truncated into single points. The 7-polytope now has the combined faces of the parent and dual.
Birectified	t2{p,q,r,s,t,u}		Birectification reduces cells to their duals.
Truncated	t0,1{p,q,r,s,t,u}		Each original vertex is cut off, with a new face filling the gap. Truncation has a degree of freedom, which has one solution that creates a uniform truncated 7-polytope. The 7-polytope has its original faces doubled in sides, and contains the faces of the dual.
Bitruncated	t1,2{p,q,r,s,t,u}		Bitrunction transforms cells to their dual truncation.
Tritruncated	t2,3{p,q,r,s,t,u}		Tritruncation transforms 4-faces to their dual truncation.
Cantellated	t0,2{p,q,r,s,t,u}		In addition to vertex truncation, each original edge is beveled with new rectangular faces appearing in their place. A uniform cantellation is halfway between both the parent and dual forms.
Bicantellated	t1,3{p,q,r,s,t,u}		In addition to vertex truncation, each original edge is beveled with new rectangular faces appearing in their place. A uniform cantellation is halfway between both the parent and dual forms.
Runcinated	t0,3{p,q,r,s,t,u}		Runcination reduces cells and creates new cells at the vertices and edges.
Biruncinated	t1,4{p,q,r,s,t,u}		Runcination reduces cells and creates new cells at the vertices and edges.
Stericated	t0,4{p,q,r,s,t,u}		Sterication reduces 4-faces and creates new 4-faces at the vertices, edges, and faces in the gaps.
Pentellated	t0,5{p,q,r,s,t,u}		Pentellation reduces 5-faces and creates new 5-faces at the vertices, edges, faces, and cells in the gaps.
Hexicated	t0,6{p,q,r,s,t,u}		Hexication reduces 6-faces and creates new 6-faces at the vertices, edges, faces, cells, and 4-faces in the gaps. (expansion operation for 7-polytopes)
Omnitruncated	t0,1,2,3,4,5,6{p,q,r,s,t,u}		All six operators, truncation, cantellation, runcination, sterication, pentellation, and hexication are applied.

References

1. Richeson, D.; Euler's Gem: The Polyhedron Formula and the Birth of Topoplogy, Princeton, 2008.

• T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900
• A. Boole Stott: Geometrical deduction of semiregular from regular polytopes and space fillings, Verhandelingen of the Koninklijke academy van Wetenschappen width unit Amsterdam, Eerste Sectie 11,1, Amsterdam, 1910
• H.S.M. Coxeter:
  • H.S.M. Coxeter, M.S. Longuet-Higgins und J.C.P. Miller: Uniform Polyhedra, Philosophical Transactions of the Royal Society of London, Londne, 1954
  • H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
• Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN   978-0-471-01003-6 http://www.wiley.com/WileyCDA/WileyTitle/productCd-0471010030.html
  • (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
  • (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
  • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
• N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. Dissertation, University of Toronto, 1966
• Klitzing, Richard. "7D uniform polytopes (polyexa)".

External links

• Polytope names
• Polytopes of Various Dimensions
• Multi-dimensional Glossary

v t e Fundamental convex regular and uniform polytopes in dimensions 2–10
Family	An	Bn	I2(p) / Dn	E6 / E7 / E8 / F4 / G2	Hn
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform polychoron	Pentachoron	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	122 • 221
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	132 • 231 • 321
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	142 • 241 • 421
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
Uniform n-polytope	n-simplex	n-orthoplex • n-cube	n-demicube	1k2 • 2k1 • k21	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds