Cantic 7-cube

Last updated
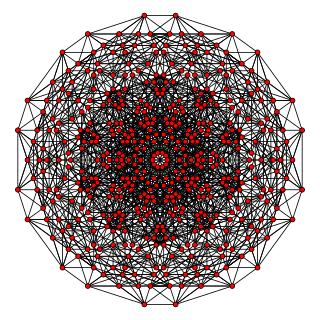
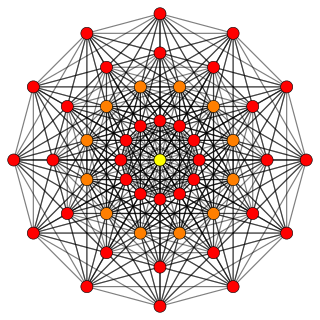
Truncated 7-demicube
Cantic 7-cube
Truncated 7-demicube D7.svg
D7 Coxeter plane projection
Type uniform 7-polytope
Schläfli symbol t{3,34,1}
h2{4,3,3,3,3,3}
Coxeter diagram CDel nodes 10ru.pngCDel split2.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png
CDel node h.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png
6-faces142
5-faces1428
4-faces5656
Cells11760
Faces13440
Edges7392
Vertices1344
Vertex figure ( )v{ }x{3,3,3}
Coxeter groups D7, [34,1,1]
Properties convex

In seven-dimensional geometry, a cantic 7-cube or truncated 7-demicube as a uniform 7-polytope, being a truncation of the 7-demicube.

Geometry branch of mathematics that measures the shape, size and position of objects

Geometry is a branch of mathematics concerned with questions of shape, size, relative position of figures, and the properties of space. A mathematician who works in the field of geometry is called a geometer.

Uniform 7-polytope vertex-transitive 7-polytope bounded by uniform facets

In seven-dimensional geometry, a 7-polytope is a polytope contained by 6-polytope facets. Each 5-polytope ridge being shared by exactly two 6-polytope facets.

Truncation (geometry) operation that cuts polytope vertices, creating a new facet in place of each vertex

In geometry, a truncation is an operation in any dimension that cuts polytope vertices, creating a new facet in place of each vertex. The term originates from Kepler's names for the Archimedean solids.

Contents

A uniform 7-polytope is vertex-transitive and constructed from uniform 6-polytope facets, and can be represented a coxeter diagram with ringed nodes representing active mirrors. A demihypercube is an alternation of a hypercube.

6-polytope 6-dimensional polytope

In six-dimensional geometry, a six-dimensional polytope or 6-polytope is a polytope, bounded by 5-polytope facets.

Demihypercube polytope constructed from alternation of an hypercube

In geometry, demihypercubes are a class of n-polytopes constructed from alternation of an n-hypercube, labeled as hγn for being half of the hypercube family, γn. Half of the vertices are deleted and new facets are formed. The 2n facets become 2n(n-1)-demicubes, and 2n(n-1)-simplex facets are formed in place of the deleted vertices.

Alternation (geometry) operation on a polyhedron or tiling that removes alternate vertices

In geometry, an alternation or partial truncation, is an operation on a polygon, polyhedron, tiling, or higher dimensional polytope that removes alternate vertices.

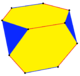
Its 3-dimensional analogue would be a truncated tetrahedron (truncated 3-demicube), and Coxeter diagram CDel nodes 10ru.pngCDel split2.pngCDel node 1.png or CDel node h.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.png as a cantic cube.

Truncated tetrahedron Archimedean solid

In geometry, the truncated tetrahedron is an Archimedean solid. It has 4 regular hexagonal faces, 4 equilateral triangle faces, 12 vertices and 18 edges. It can be constructed by truncating all 4 vertices of a regular tetrahedron at one third of the original edge length.

Alternate names

Cartesian coordinates

The Cartesian coordinates for the 1344 vertices of a truncated 7-demicube centered at the origin and edge length 6√2 are coordinate permutations:

(±1,±1,±3,±3,±3,±3,±3)

with an odd number of plus signs.

Images

It can be visualized as a 2-dimensional orthogonal projections, for example the a D7 Coxeter plane, containing 12-gonal symmetry. Most visualizations in symmetric projections will contain overlapping vertices, so the colors of the vertices are changed based on how many vertices are at each projective position, here shown with red color for no overlaps.

orthographic projections
Coxeter
plane 		B7D7D6
Graph 7-demicube t01 B7.svg 7-demicube t01 D7.svg 7-demicube t01 D6.svg
Dihedral
symmetry 		[14/2][12][10]
Coxeter planeD5D4D3
Graph 7-demicube t01 D5.svg 7-demicube t01 D4.svg 7-demicube t01 D3.svg
Dihedral
symmetry		[8][6][4]
Coxeter
plane		A5A3
Graph 7-demicube t01 A5.svg 7-demicube t01 A3.svg
Dihedral
symmetry		[6][4]
Dimensional family of cantic n-cubes
n345678
Symmetry
[1+,4,3n-2]		[1+,4,3]
= [3,3]		[1+,4,32]
= [3,31,1]		[1+,4,33]
= [3,32,1]		[1+,4,34]
= [3,33,1]		[1+,4,35]
= [3,34,1]		[1+,4,36]
= [3,35,1]
Cantic
figure		 Cantic cube.png Schlegel half-solid truncated 16-cell.png Truncated 5-demicube D5.svg Truncated 6-demicube D6.svg Truncated 7-demicube D7.svg Truncated 8-demicube D8.svg
Coxeter CDel node h1.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.png
= CDel nodes 10ru.pngCDel split2.pngCDel node 1.png		CDel node h1.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node.png
= CDel nodes 10ru.pngCDel split2.pngCDel node 1.pngCDel 3.pngCDel node.png		CDel node h1.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png
= CDel nodes 10ru.pngCDel split2.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png		CDel node h1.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png
= CDel nodes 10ru.pngCDel split2.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png		CDel node h1.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png
= CDel nodes 10ru.pngCDel split2.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png		CDel node h1.pngCDel 4.pngCDel node.pngCDel 3.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png
= CDel nodes 10ru.pngCDel split2.pngCDel node 1.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.pngCDel 3.pngCDel node.png
Schläfli h2{4,3} h2{4,32} h2{4,33} h2{4,34} h2{4,35} h2{4,36}

There are 95 uniform polytopes with D6 symmetry, 63 are shared by the B6 symmetry, and 32 are unique:

Notes

  1. ↑ Klitzing, (x3x3o *b3o3o3o3o - thesa)

Related Research Articles

5-demicube semiregular 5-polytope

In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a 5-hypercube (penteract) with alternated vertices removed.

6-demicube uniform 6-polytope

In geometry, a 6-demicube or demihexteract is a uniform 6-polytope, constructed from a 6-cube (hexeract) with alternated vertices removed. It is part of a dimensionally infinite family of uniform polytopes called demihypercubes.

7-demicube uniform 7-polytope

In geometry, a demihepteract or 7-demicube is a uniform 7-polytope, constructed from the 7-hypercube (hepteract) with alternated vertices removed. It is part of a dimensionally infinite family of uniform polytopes called demihypercubes.

Rectified 6-cubes

In six-dimensional geometry, a rectified 6-cube is a convex uniform 6-polytope, being a rectification of the regular 6-cube.

Cantic 6-cube

In six-dimensional geometry, a cantic 6-cube is a uniform 6-polytope.

Cantic 5-cube

In geometry of five dimensions or higher, a cantic 5-cube, cantihalf 5-cube, truncated 5-demicube is a uniform 5-polytope, being a truncation of the 5-demicube. It has half the vertices of a cantellated 5-cube.

In six-dimensional geometry, a truncated 6-cube is a convex uniform 6-polytope, being a truncation of the regular 6-cube.

B<sub>7</sub> polytope Wikimedia list article

In 7-dimensional geometry, there are 128 uniform polytopes with B7 symmetry. There are two regular forms, the 7-orthoplex, and 8-cube with 14 and 128 vertices respectively. The 7-demicube is added with half of the symmetry.

D<sub>6</sub> polytope Wikimedia list article

In 6-dimensional geometry, there are 47 uniform polytopes with D6 symmetry, 16 are unique, and 31 are shared with the B6 symmetry. There are two regular forms, the 6-orthoplex, and 6-demicube with 12 and 32 vertices respectively.

D<sub>7</sub> polytope Wikimedia list article

In 7-dimensional geometry, there are 95 uniform polytopes with D7 symmetry; 32 are unique, and 63 are shared with the B7 symmetry. There are two regular forms, the 7-orthoplex, and 7-demicube with 14 and 64 vertices respectively.

Runcinated 5-orthoplexes

In five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation (runcination) of the regular 5-orthoplex.

Runcic 5-cubes

In six-dimensional geometry, a runcic 5-cube or is a convex uniform 5-polytope. There are 2 runcic forms for the 5-cube. Runcic 5-cubes have half the vertices of runcinated 5-cubes.

Cantic 8-cube

In eight-dimensional geometry, a cantic 8-cube or truncated 8-demicube is a uniform 8-polytope, being a truncation of the 8-demicube.

Runcic 6-cubes

In six-dimensional geometry, a runcic 6-cube is a convex uniform 6-polytope. There are 2 unique runcic for the 6-cube.

Steric 6-cubes

In six-dimensional geometry, a steric 6-cube is a convex uniform 6-polytope. There are unique 4 steric forms of the 6-cube.

Runcic 7-cubes

In seven-dimensional geometry, a runcic 7-cube is a convex uniform 7-polytope, related to the uniform 7-demicube. There are 2 unique forms.

Pentic 7-cubes

In seven-dimensional geometry, a pentic 7-cube is a convex uniform 7-polytope, related to the uniform 7-demicube. There are 8 unique forms.

Hexic 7-cubes

In seven-dimensional geometry, a hexic 7-cube is a convex uniform 7-polytope, constructed from the uniform 7-demicube. There are 16 unique forms.

Steric 5-cubes

In five-dimensional geometry, a steric 5-cube or is a convex uniform 5-polytope. There are unique 4 steric forms of the 5-cube. Steric 5-cubes have half the vertices of stericated 5-cubes.

Steric 7-cubes

In seven-dimensional geometry, a stericated 7-cube is a convex uniform 7-polytope, being a runcination of the uniform 7-demicube. There are 4 unique runcinations for the 7-demicube including truncation and cantellation.

References

Fundamental convex regular and uniform polytopes in dimensions 2–10
Family An Bn I2(p) / Dn E6 / E7 / E8 / F4 / G2 Hn
Regular polygon Triangle Square p-gon Hexagon Pentagon
Uniform polyhedron Tetrahedron Octahedron • Cube Demicube Dodecahedron • Icosahedron
Uniform 4-polytope 5-cell 16-cell • Tesseract Demitesseract 24-cell 120-cell • 600-cell
Uniform 5-polytope 5-simplex 5-orthoplex • 5-cube 5-demicube
Uniform 6-polytope 6-simplex 6-orthoplex • 6-cube 6-demicube 122 • 221
Uniform 7-polytope 7-simplex 7-orthoplex • 7-cube 7-demicube 132 • 231 • 321
Uniform 8-polytope 8-simplex 8-orthoplex • 8-cube 8-demicube 142 • 241 • 421
Uniform 9-polytope 9-simplex 9-orthoplex • 9-cube 9-demicube
Uniform 10-polytope 10-simplex 10-orthoplex • 10-cube 10-demicube
Uniform n-polytope n-simplex n-orthoplex • n-cube n-demicube 1k2 • 2k1 • k21 n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.