Cantic 5-cube

Last updated March 18, 2019

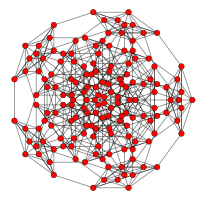
Truncated 5-demicube Cantic 5-cube
D5 Coxeter plane projection
Type	uniform 5-polytope
Schläfli symbol	h₂{4,3,3,3} t{3,3^2,1}
Coxeter-Dynkin diagram	=
4-faces	42 total: 16 r{3,3,3} 16 t{3,3,3} 10 t{3,3,4}
Cells	280 total: 80 {3,3} 120 t{3,3} 80 {3,4}
Faces	640 total: 480 {3} 160 {6}
Edges	560
Vertices	160
Vertex figure	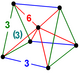( )v{ }×{3}
Coxeter groups	D₅, [3^2,1,1]
Properties	convex

In geometry of five dimensions or higher, a cantic 5-cube, cantihalf 5-cube, truncated 5-demicube is a uniform 5-polytope, being a truncation of the 5-demicube. It has half the vertices of a cantellated 5-cube.

Geometry is a branch of mathematics concerned with questions of shape, size, relative position of figures, and the properties of space. A mathematician who works in the field of geometry is called a geometer.

A five-dimensional space is a space with five dimensions. If interpreted physically, that is one more than the usual three spatial dimensions and the fourth dimension of time used in relativitistic physics. It is an abstraction which occurs frequently in mathematics, where it is a legitimate construct. In physics and mathematics, a sequence of N numbers can be understood to represent a location in an N-dimensional space. Whether or not the universe is five-dimensional is a topic of debate.

In geometry, a uniform 5-polytope is a five-dimensional uniform polytope. By definition, a uniform 5-polytope is vertex-transitive and constructed from uniform 4-polytope facets.

Cartesian coordinates

The Cartesian coordinates for the 160 vertices of a cantic 5-cube centered at the origin and edge length 6√2 are coordinate permutations:

(±1,±1,±3,±3,±3)

with an odd number of plus signs.

Alternate names

Cantic penteract, truncated demipenteract
Truncated hemipenteract (thin) (Jonathan Bowers)^[1]

Images

orthographic projections
Coxeter plane	B₅
Graph
Dihedral symmetry	[10/2]
Coxeter plane	D₅	D₄
Graph
Dihedral symmetry	[8]	[6]
Coxeter plane	D₃	A₃
Graph
Dihedral symmetry	[4]	[4]

Related polytopes

It has half the vertices of the cantellated 5-cube, as compared here in the B5 Coxeter plane projections:

Cantic 5-cube	Cantellated 5-cube

This polytope is based on the 5-demicube, a part of a dimensional family of uniform polytopes called demihypercubes for being alternation of the hypercube family.

In five-dimensional geometry, a demipenteract or 5-demicube is a semiregular 5-polytope, constructed from a 5-hypercube (penteract) with alternated vertices removed.

A uniform polytope of dimension three or higher is a vertex-transitive polytope bounded by uniform facets. The uniform polytopes in two dimensions are the regular polygons.

Demihypercube polytope constructed from alternation of an hypercube

In geometry, demihypercubes are a class of n-polytopes constructed from alternation of an n-hypercube, labeled as hγ_n for being half of the hypercube family, γ_n. Half of the vertices are deleted and new facets are formed. The 2n facets become 2n(n-1)-demicubes, and 2ⁿ(n-1)-simplex facets are formed in place of the deleted vertices.

Dimensional family of cantic n-cubes
n	3	4	5	6	7	8
Symmetry [1⁺,4,3^n-2]	[1⁺,4,3] = [3,3]	[1⁺,4,3²] = [3,3^1,1]	[1⁺,4,3³] = [3,3^2,1]	[1⁺,4,3⁴] = [3,3^3,1]	[1⁺,4,3⁵] = [3,3^4,1]	[1⁺,4,3⁶] = [3,3^5,1]
Cantic figure
Coxeter	=	=	=	=	=	=
Schläfli	h₂{4,3}	h₂{4,3²}	h₂{4,3³}	h₂{4,3⁴}	h₂{4,3⁵}	h₂{4,3⁶}

There are 23 uniform 5-polytope that can be constructed from the D₅ symmetry of the 5-demicube, of which are unique to this family, and 15 are shared within the 5-cube family.

In five-dimensional geometry, a 5-cube is a name for a five-dimensional hypercube with 32 vertices, 80 edges, 80 square faces, 40 cubic cells, and 10 tesseract 4-faces.

D5 polytopes
h{4,3,3,3}	h₂{4,3,3,3}	h₃{4,3,3,3}	h₄{4,3,3,3}	h_2,3{4,3,3,3}	h_2,4{4,3,3,3}	h_3,4{4,3,3,3}	h_2,3,4{4,3,3,3}

Notes

↑ Klitzing, (x3x3o *b3o3o - thin)

Related Research Articles

In four-dimensional geometry, a cantellated tesseract is a convex uniform 4-polytope, being a cantellation of the regular tesseract.

In geometry, a truncated tesseract is a uniform 4-polytope formed as the truncation of the regular tesseract.

In geometry, a 9-cube is a nine-dimensional hypercube with 512 vertices, 2304 edges, 4608 square faces, 5376 cubic cells, 4032 tesseract 4-faces, 2016 5-cube 5-faces, 672 6-cube 6-faces, 144 7-cube 7-faces, and 18 8-cube 8-faces.

In geometry, a 10-cube is a ten-dimensional hypercube. It has 1024 vertices, 5120 edges, 11520 square faces, 15360 cubic cells, 13440 tesseract 4-faces, 8064 5-cube 5-faces, 3360 6-cube 6-faces, 960 7-cube 7-faces, 180 8-cube 8-faces, and 20 9-cube 9-faces.

In six-dimensional geometry, a rectified 6-cube is a convex uniform 6-polytope, being a rectification of the regular 6-cube.

In six-dimensional geometry, a cantic 6-cube is a uniform 6-polytope.

In six-dimensional geometry, a truncated 6-cube is a convex uniform 6-polytope, being a truncation of the regular 6-cube.

In five-dimensional geometry, a runcinated 5-orthoplex is a convex uniform 5-polytope with 3rd order truncation (runcination) of the regular 5-orthoplex.

In five-dimensional geometry, a cantellated 5-orthoplex is a convex uniform 5-polytope, being a cantellation of the regular 5-orthoplex.

In six-dimensional geometry, a runcic 5-cube or is a convex uniform 5-polytope. There are 2 runcic forms for the 5-cube. Runcic 5-cubes have half the vertices of runcinated 5-cubes.

In six-dimensional geometry, a cantellated 5-cube is a convex uniform 5-polytope, being a cantellation of the regular 5-cube.

In seven-dimensional geometry, a cantic 7-cube or truncated 7-demicube as a uniform 7-polytope, being a truncation of the 7-demicube.

In six-dimensional geometry, a cantellated 6-cube is a convex uniform 6-polytope, being a cantellation of the regular 6-cube.

In eight-dimensional geometry, a cantic 8-cube or truncated 8-demicube is a uniform 8-polytope, being a truncation of the 8-demicube.

In six-dimensional geometry, a runcic 6-cube is a convex uniform 6-polytope. There are 2 unique runcic for the 6-cube.

In six-dimensional geometry, a steric 6-cube is a convex uniform 6-polytope. There are unique 4 steric forms of the 6-cube.

In seven-dimensional geometry, a runcic 7-cube is a convex uniform 7-polytope, related to the uniform 7-demicube. There are 2 unique forms.

In five-dimensional geometry, a steric 5-cube or is a convex uniform 5-polytope. There are unique 4 steric forms of the 5-cube. Steric 5-cubes have half the vertices of stericated 5-cubes.

In seven-dimensional geometry, a stericated 7-cube is a convex uniform 7-polytope, being a runcination of the uniform 7-demicube. There are 4 unique runcinations for the 7-demicube including truncation and cantellation.

References

H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
  - (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
  - (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
  - (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D.
Klitzing, Richard. "5D uniform polytopes (polytera) x3x3o *b3o3o - thin".

External links

v t e Fundamental convex regular and uniform polytopes in dimensions 2–10
Family	A_n	B_n	I₂(p) / D_n	E₆ / E₇ / E₈ / F₄ / G₂	H_n
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform 4-polytope	5-cell	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	1₂₂ • 2₂₁
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	1₃₂ • 2₃₁ • 3₂₁
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	1₄₂ • 2₄₁ • 4₂₁
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
Uniform n-polytope	n-simplex	n-orthoplex • n-cube	n-demicube	1_k2 • 2_k1 • k₂₁	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Klitzing, (x3x3o *b3o3o - thin)