Rectified 5-cell

Last updated November 20, 2024

Rectified 5-cell
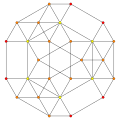
Schlegel diagram with the 5 tetrahedral cells shown.
Type	Uniform 4-polytope
Schläfli symbol	t₁{3,3,3} or r{3,3,3} {3^2,1} = $\left\{{\begin{array}{l}3\\3,3\end{array}}\right\}$
Coxeter-Dynkin diagram
Cells	10	5 {3,3} 5 3.3.3.3
Faces	30 {3}
Edges	30
Vertices	10
Vertex figure	Triangular prism
Symmetry group	A₄, [3,3,3], order 120
Petrie polygon	Pentagon
Properties	convex, isogonal, isotoxal
Uniform index	1 2 3

In four-dimensional geometry, the rectified 5-cell is a uniform 4-polytope composed of 5 regular tetrahedral and 5 regular octahedral cells. Each edge has one tetrahedron and two octahedra. Each vertex has two tetrahedra and three octahedra. In total it has 30 triangle faces, 30 edges, and 10 vertices. Each vertex is surrounded by 3 octahedra and 2 tetrahedra; the vertex figure is a triangular prism.

Topologically, under its highest symmetry, [3,3,3], there is only one geometrical form, containing 5 regular tetrahedra and 5 rectified tetrahedra (which is geometrically the same as a regular octahedron). It is also topologically identical to a tetrahedron-octahedron segmentochoron.^{[ clarification needed ]}

The vertex figure of the rectified 5-cell is a uniform triangular prism, formed by three octahedra around the sides, and two tetrahedra on the opposite ends.^[1]

Despite having the same number of vertices as cells (10) and the same number of edges as faces (30), the rectified 5-cell is not self-dual because the vertex figure (a uniform triangular prism) is not a dual of the polychoron's cells.

Wythoff construction

Seen in a configuration matrix, all incidence counts between elements are shown. The diagonal f-vector numbers are derived through the Wythoff construction, dividing the full group order of a subgroup order by removing one mirror at a time.^[2]

A₄	k-face	f_k	f₀	f₁	f₂		f₃		k-figure	Notes
A₂A₁	( )	f₀	10	6	3	6	3	2	{3}x{ }	A₄/A₂A₁ = 5!/3!/2 = 10
A₁A₁	{ }	f₁	2	30	1	2	2	1	{ }v( )	A₄/A₁A₁ = 5!/2/2 = 30
A₂A₁	{3}	f₂	3	3	10	*	2	0	{ }	A₄/A₂A₁ = 5!/3!/2 = 10
A₂	{3}	f₂	3	3	*	20	1	1	{ }	A₄/A₂ = 5!/3! = 20
A₃	r{3,3}	f₃	6	12	4	4	5	*	( )	A₄/A₃ = 5!/4! = 5
A₃	{3,3}	f₃	4	6	0	4	*	5	( )	A₄/A₃ = 5!/4! = 5

Structure

Together with the simplex and 24-cell, this shape and its dual (a polytope with ten vertices and ten triangular bipyramid facets) was one of the first 2-simple 2-simplicial 4-polytopes known. This means that all of its two-dimensional faces, and all of the two-dimensional faces of its dual, are triangles. In 1997, Tom Braden found another dual pair of examples, by gluing two rectified 5-cells together; since then, infinitely many 2-simple 2-simplicial polytopes have been constructed.^[3]^[4]

Semiregular polytope

It is one of three semiregular 4-polytopes made of two or more cells which are Platonic solids, discovered by Thorold Gosset in his 1900 paper. He called it a tetroctahedric for being made of tetrahedron and octahedron cells.^[5]

E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as tC₅.

Alternate names

Tetroctahedric (Thorold Gosset)
Dispentachoron
Rectified 5-cell (Norman W. Johnson)
Rectified 4-simplex
Fully truncated 4-simplex
Rectified pentachoron (Acronym: rap) (Jonathan Bowers)
Ambopentachoron (Neil Sloane & John Horton Conway)
(5,2)-hypersimplex (the convex hull of five-dimensional (0,1)-vectors with exactly two ones)

Images

orthographic projections
A_k Coxeter plane	A₄	A₃	A₂
Graph
Dihedral symmetry	[5]	[4]	[3]

stereographic projection (centered on octahedron)	Net (polytope)
	Tetrahedron-centered perspective projection into 3D space, with nearest tetrahedron to the 4D viewpoint rendered in red, and the 4 surrounding octahedra in green. Cells lying on the far side of the polytope have been culled for clarity (although they can be discerned from the edge outlines). The rotation is only of the 3D projection image, in order to show its structure, not a rotation in 4D space.

Coordinates

The Cartesian coordinates of the vertices of an origin-centered rectified 5-cell having edge length 2 are:

Coordinates
$\left({\sqrt {\frac {2}{5}}},\ {\frac {2}{\sqrt {6}}},\ {\frac {2}{\sqrt {3}}},\ 0\right)$ $\left({\sqrt {\frac {2}{5}}},\ {\frac {2}{\sqrt {6}}},\ {\frac {-1}{\sqrt {3}}},\ \pm 1\right)$ $\left({\sqrt {\frac {2}{5}}},\ {\frac {-2}{\sqrt {6}}},\ {\frac {1}{\sqrt {3}}},\ \pm 1\right)$ $\left({\sqrt {\frac {2}{5}}},\ {\frac {-2}{\sqrt {6}}},\ {\frac {-2}{\sqrt {3}}},\ 0\right)$	$\left({\frac {-3}{\sqrt {10}}},\ {\frac {1}{\sqrt {6}}},\ {\frac {1}{\sqrt {3}}},\ \pm 1\right)$ $\left({\frac {-3}{\sqrt {10}}},\ {\frac {1}{\sqrt {6}}},\ {\frac {-2}{\sqrt {3}}},\ 0\right)$ $\left({\frac {-3}{\sqrt {10}}},\ -{\sqrt {\frac {3}{2}}},\ 0,\ 0\right)$

More simply, the vertices of the rectified 5-cell can be positioned on a hyperplane in 5-space as permutations of (0,0,0,1,1) or (0,0,1,1,1). These construction can be seen as positive orthant facets of the rectified pentacross or birectified penteract respectively.

Related 4-polytopes

The rectified 5-cell is the vertex figure of the 5-demicube, and the edge figure of the uniform 2₂₁ polytope.

Compound of the rectified 5-cell and its dual

The convex hull the rectified 5-cell and its dual (of the same long radius) is a nonuniform polychoron composed of 30 cells: 10 tetrahedra, 20 octahedra (as triangular antiprisms), and 20 vertices. Its vertex figure is a triangular bifrustum.

Pentachoron polytopes

The rectified 5-cell is one of 9 Uniform 4-polytopes constructed from the [3,3,3] Coxeter group.

Name	5-cell	truncated 5-cell	rectified 5-cell	cantellated 5-cell	bitruncated 5-cell	cantitruncated 5-cell	runcinated 5-cell	runcitruncated 5-cell	omnitruncated 5-cell
Schläfli symbol	{3,3,3} 3r{3,3,3}	t{3,3,3} 2t{3,3,3}	r{3,3,3} 2r{3,3,3}	rr{3,3,3} r2r{3,3,3}	2t{3,3,3}	tr{3,3,3} t2r{3,3,3}	t_0,3{3,3,3}	t_0,1,3{3,3,3} t_0,2,3{3,3,3}	t_0,1,2,3{3,3,3}
Coxeter diagram
Schlegel diagram
A₄ Coxeter plane Graph
A₃ Coxeter plane Graph
A₂ Coxeter plane Graph

Semiregular polytopes

The rectified 5-cell is second in a dimensional series of semiregular polytopes. Each progressive uniform polytope is constructed as the vertex figure of the previous polytope. Thorold Gosset identified this series in 1900 as containing all regular polytope facets, containing all simplexes and orthoplexes (tetrahedrons and octahedrons in the case of the rectified 5-cell). The Coxeter symbol for the rectified 5-cell is 0₂₁.

k₂₁ figures in n dimensions
Space	Finite						Euclidean	Hyperbolic
E_n	3	4	5	6	7	8	9	10
Coxeter group	E₃=A₂A₁	E₄=A₄	E₅=D₅	E₆	E₇	E₈	E₉ = ${\tilde {E}}_{8}$ = E₈⁺	E₁₀ = ${\bar {T}}_{8}$ = E₈⁺⁺
Coxeter diagram
Symmetry	[3^−1,2,1]	[3^0,2,1]	[3^1,2,1]	[3^2,2,1]	[3^3,2,1]	[3^4,2,1]	[3^5,2,1]	[3^6,2,1]
Order	12	120	1,920	51,840	2,903,040	696,729,600	∞
Graph							-	-
Name	−1₂₁	0₂₁	1₂₁	2₂₁	3₂₁	4₂₁	5₂₁	6₂₁

Isotopic polytopes

Isotopic uniform truncated simplices
Dim.	2	3	4	5	6	7	8
Name Coxeter	Hexagon = t{3} = {6}	Octahedron = r{3,3} = {3^1,1} = {3,4} $\left\{{\begin{array}{l}3\\3\end{array}}\right\}$	Decachoron 2t{3³}	Dodecateron 2r{3⁴} = {3^2,2} $\left\{{\begin{array}{l}3,3\\3,3\end{array}}\right\}$	Tetradecapeton 3t{3⁵}	Hexadecaexon 3r{3⁶} = {3^3,3} $\left\{{\begin{array}{l}3,3,3\\3,3,3\end{array}}\right\}$	Octadecazetton 4t{3⁷}
Images
Vertex figure	( )∨( )	{ }×{ }	{ }∨{ }	{3}×{3}	{3}∨{3}	{3,3}×{3,3}	{3,3}∨{3,3}
Facets		{3}	t{3,3}	r{3,3,3}	2t{3,3,3,3}	2r{3,3,3,3,3}	3t{3,3,3,3,3,3}
As intersecting dual simplexes	∩	∩	∩	∩	∩	∩	∩

Notes

↑ Conway, 2008
↑ Klitzing, Richard. "o3x4o3o - rap".
↑ Eppstein, David; Kuperberg, Greg; Ziegler, Günter M. (2003), "Fat 4-polytopes and fatter 3-spheres", in Bezdek, Andras (ed.), Discrete Geometry: In honor of W. Kuperberg's 60th birthday, Pure and Applied Mathematics, vol. 253, pp. 239–265, arXiv: math.CO/0204007 .
↑ Paffenholz, Andreas; Ziegler, Günter M. (2004), "The E_t-construction for lattices, spheres and polytopes", Discrete & Computational Geometry, 32 (4): 601–621, arXiv: math.MG/0304492 , doi:10.1007/s00454-004-1140-4, MR 2096750, S2CID 7603863 .
↑ Gosset, 1900

Related Research Articles

In geometry, a convex uniform honeycomb is a uniform tessellation which fills three-dimensional Euclidean space with non-overlapping convex uniform polyhedral cells.

In geometry, a uniform 4-polytope is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedra, and faces are regular polygons.

In four-dimensional geometry, a runcinated 5-cell is a convex uniform 4-polytope, being a runcination of the regular 5-cell.

In geometry, the rectified 600-cell or rectified hexacosichoron is a convex uniform 4-polytope composed of 600 regular octahedra and 120 icosahedra cells. Each edge has two octahedra and one icosahedron. Each vertex has five octahedra and two icosahedra. In total it has 3600 triangle faces, 3600 edges, and 720 vertices.

In geometry, the snub 24-cell or snub disicositetrachoron is a convex uniform 4-polytope composed of 120 regular tetrahedral and 24 icosahedral cells. Five tetrahedra and three icosahedra meet at each vertex. In total it has 480 triangular faces, 432 edges, and 96 vertices. One can build it from the 600-cell by diminishing a select subset of icosahedral pyramids and leaving only their icosahedral bases, thereby removing 480 tetrahedra and replacing them with 24 icosahedra.

In four-dimensional geometry, a cantellated tesseract is a convex uniform 4-polytope, being a cantellation of the regular tesseract.

The cubic honeycomb or cubic cellulation is the only proper regular space-filling tessellation in Euclidean 3-space made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a regular octahedron. It is a self-dual tessellation with Schläfli symbol {4,3,4}. John Horton Conway called this honeycomb a cubille.

The tetrahedral-octahedral honeycomb, alternated cubic honeycomb is a quasiregular space-filling tessellation in Euclidean 3-space. It is composed of alternating regular octahedra and tetrahedra in a ratio of 1:2.

In geometry, a truncated 24-cell is a uniform 4-polytope formed as the truncation of the regular 24-cell.

In geometry, a truncated tesseract is a uniform 4-polytope formed as the truncation of the regular tesseract.

In geometry, a truncated 5-cell is a uniform 4-polytope formed as the truncation of the regular 5-cell.

In four-dimensional geometry, a cantellated 5-cell is a convex uniform 4-polytope, being a cantellation of the regular 5-cell.

In four-dimensional geometry, a runcinated 24-cell is a convex uniform 4-polytope, being a runcination of the regular 24-cell.

In four-dimensional geometry, a runcinated 120-cell is a convex uniform 4-polytope, being a runcination of the regular 120-cell.

<span class="mw-page-title-main">Demihypercube</span> Polytope constructed from alternation of an hypercube

In geometry, demihypercubes (also called n-demicubes, n-hemicubes, and half measure polytopes) are a class of n-polytopes constructed from alternation of an n-hypercube, labeled as hγ_n for being half of the hypercube family, γ_n. Half of the vertices are deleted and new facets are formed. The 2n facets become 2n(n−1)-demicubes, and 2ⁿ(n−1)-simplex facets are formed in place of the deleted vertices.

In geometry, a uniform polytope of dimension three or higher is a vertex-transitive polytope bounded by uniform facets. Here, "vertex-transitive" means that it has symmetries taking every vertex to every other vertex; the same must also be true within each lower-dimensional face of the polytope. In two dimensions a stronger definition is used: only the regular polygons are considered as uniform, disallowing polygons that alternate between two different lengths of edges.

In geometry, a uniform k₂₁ polytope is a polytope in k + 4 dimensions constructed from the E_n Coxeter group, and having only regular polytope facets. The family was named by their Coxeter symbol k₂₁ by its bifurcating Coxeter–Dynkin diagram, with a single ring on the end of the k-node sequence.

In five-dimensional geometry, a rectified 5-simplex is a convex uniform 5-polytope, being a rectification of the regular 5-simplex.

In 7-dimensional geometry, the 3₂₁ polytope is a uniform 7-polytope, constructed within the symmetry of the E₇ group. It was discovered by Thorold Gosset, published in his 1900 paper. He called it an 7-ic semi-regular figure.

In four-dimensional Euclidean geometry, the snub 24-cell honeycomb, or snub icositetrachoric honeycomb is a uniform space-filling tessellation by snub 24-cells, 16-cells, and 5-cells. It was discovered by Thorold Gosset with his 1900 paper of semiregular polytopes. It is not semiregular by Gosset's definition of regular facets, but all of its cells (ridges) are regular, either tetrahedra or icosahedra.

References

T. Gosset: On the Regular and Semi-Regular Figures in Space of n Dimensions, Messenger of Mathematics, Macmillan, 1900
J.H. Conway and M.J.T. Guy: Four-Dimensional Archimedean Polytopes, Proceedings of the Colloquium on Convexity at Copenhagen, page 38 and 39, 1965
H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
  - (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380–407, MR 2,10]
  - (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
  - (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. (1966)
John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26)

External links

Rectified 5-cell - data and images
- 1. Convex uniform polychora based on the pentachoron - Model 2 , George Olshevsky.
Klitzing, Richard. "4D uniform polytopes (polychora) x3o3o3o - rap".

v t e Fundamental convex regular and uniform polytopes in dimensions 2–10
Family	A_n	B_n	I₂(p) / D_n	E₆ / E₇ / E₈ / F₄ / G₂	H_n
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform polychoron	Pentachoron	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Uniform 5-polytope	5-simplex	5-orthoplex • 5-cube	5-demicube
Uniform 6-polytope	6-simplex	6-orthoplex • 6-cube	6-demicube	1₂₂ • 2₂₁
Uniform 7-polytope	7-simplex	7-orthoplex • 7-cube	7-demicube	1₃₂ • 2₃₁ • 3₂₁
Uniform 8-polytope	8-simplex	8-orthoplex • 8-cube	8-demicube	1₄₂ • 2₄₁ • 4₂₁
Uniform 9-polytope	9-simplex	9-orthoplex • 9-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	10-demicube
Uniform n-polytope	n-simplex	n-orthoplex • n-cube	n-demicube	1_k2 • 2_k1 • k₂₁	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Conway, 2008

[2] Klitzing, Richard. "o3x4o3o - rap".

[3] Eppstein, David; Kuperberg, Greg; Ziegler, Günter M. (2003), "Fat 4-polytopes and fatter 3-spheres", in Bezdek, Andras (ed.), Discrete Geometry: In honor of W. Kuperberg's 60th birthday, Pure and Applied Mathematics, vol. 253, pp. 239–265, arXiv: math.CO/0204007 .

[4] Paffenholz, Andreas; Ziegler, Günter M. (2004), "The E_t-construction for lattices, spheres and polytopes", Discrete & Computational Geometry, 32 (4): 601–621, arXiv: math.MG/0304492 , doi:10.1007/s00454-004-1140-4, MR 2096750, S2CID 7603863 .

[5] Gosset, 1900

[1]

[2]

[3]

[4]

[5]