The duodecimal system, also known as base twelve or dozenal, is a positional numeral system using twelve as its base. In duodecimal, the number twelve is denoted "10", meaning 1 twelve and 0 units; in the decimal system, this number is instead written as "12" meaning 1 ten and 2 units, and the string "10" means ten. In duodecimal, "100" means twelve squared, "1000" means twelve cubed, and "0.1" means a twelfth.
The system of Hebrew numerals is a quasi-decimal alphabetic numeral system using the letters of the Hebrew alphabet. The system was adapted from that of the Greek numerals sometime between 200 and 78 BCE, the latter being the date of the earliest archeological evidence.

A numeral system is a writing system for expressing numbers; that is, a mathematical notation for representing numbers of a given set, using digits or other symbols in a consistent manner.
In linguistics, a numeral in the broadest sense is a word or phrase that describes a numerical quantity. Some theories of grammar use the word "numeral" to refer to cardinal numbers that act as a determiner that specify the quantity of a noun, for example the "two" in "two hats". Some theories of grammar do not include determiners as a part of speech and consider "two" in this example to be an adjective. Some theories consider "numeral" to be a synonym for "number" and assign all numbers to a part of speech called "numerals". Numerals in the broad sense can also be analyzed as a noun, as a pronoun, or for a small number of words as an adverb.

Georgian is the most widely spoken Kartvelian language; it serves as the literary language or lingua franca for speakers of related languages. It is the official language of Georgia and the native or primary language of 88% of its population. Its speakers today amount to approximately 3.8 million. Georgian is written with its own unique Georgian scripts, alphabetical systems of unclear origin.

A vigesimal or base-20 (base-score) numeral system is based on twenty. Vigesimal is derived from the Latin adjective vicesimus, meaning 'twentieth'.
A numerical digit or numeral is a single symbol used alone, or in combinations, to represent numbers in positional notation, such as the common base 10. The name "digit" originates from the Latin digiti meaning fingers.

Positional notation, also known as place-value notation, positional numeral system, or simply place value, usually denotes the extension to any base of the Hindu–Arabic numeral system. More generally, a positional system is a numeral system in which the contribution of a digit to the value of a number is the value of the digit multiplied by a factor determined by the position of the digit. In early numeral systems, such as Roman numerals, a digit has only one value: I means one, X means ten and C a hundred. In modern positional systems, such as the decimal system, the position of the digit means that its value must be multiplied by some value: in 555, the three identical symbols represent five hundreds, five tens, and five units, respectively, due to their different positions in the digit string.

Khmer numerals ០ ១ ២ ៣ ៤ ៥ ៦ ៧ ៨ ៩ are the numerals used in the Khmer language. They have been in use since at least the early 7th century.
Numeral or number prefixes are prefixes derived from numerals or occasionally other numbers. In English and many other languages, they are used to coin numerous series of words. For example:
The traditional counting system used in the Welsh language is vigesimal, i.e. based on twenties where numbers from 11 to 14 are "x on ten", 16–19 are "x on fifteen" ; numbers from 21 to 39 are "1–19 on twenty", 40 is "two twenty", 60 is "three twenty", etc.
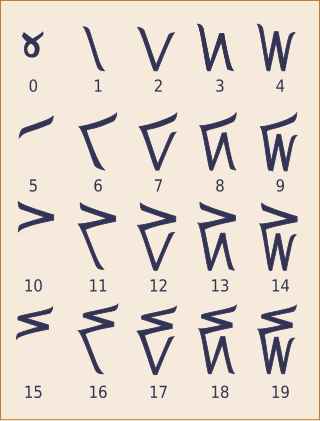
The Kaktovik numerals or Kaktovik Iñupiaq numerals are a base-20 system of numerical digits created by Alaskan Iñupiat. They are visually iconic, with shapes that indicate the number being represented.
Laz is a Kartvelian language. It is sometimes considered as a southern dialect of Zan languages, the northern dialect being the Mingrelian language.
Burmese numerals are a set of numerals traditionally used in the Burmese language, although Arabic numerals are also used. Burmese numerals follow the Hindu–Arabic numeral system commonly used in the rest of the world.
According to Lounge, the Yoruba language has a rather elaborate vigesimal (base-20) numeral system that involves both addition and subtraction and multiplication.

Muisca numerals were the numeric notation system used by the Muisca, one of the civilizations of the Americas before the Spanish conquest of the Muisca. Just like the Mayas, the Muisca had a vigesimal numerical system, based on multiples of twenty. The Muisca numerals were based on counting with fingers and toes. They had specific numbers from one to ten, yet for the numbers between eleven and nineteen they used "foot one" (11) to "foot nine" (19). The number 20 was the 'perfect' number for the Muisca which is visible in their calendar. To calculate higher numbers than 20 they used multiples of their 'perfect' number; gue-muyhica would be "20 times 4", so 80. To describe "50" they used "20 times 2 plus 10"; gue-bosa asaqui ubchihica, transcribed from guêboʒhas aſaqɣ hubchìhicâ. In their calendar, which was lunisolar, they only counted from one to ten and twenty. Each number had a special meaning, related to their deities and certain animals, especially the abundant toads.
An alphabetic numeral system is a type of numeral system. Developed in classical antiquity, it flourished during the early Middle Ages. In alphabetic numeral systems, numbers are written using the characters of an alphabet, syllabary, or another writing system. Unlike acrophonic numeral systems, where a numeral is represented by the first letter of the lexical name of the numeral, alphabetic numeral systems can arbitrarily assign letters to numerical values. Some systems, including the Arabic, Georgian and Hebrew systems, use an already established alphabetical order. Alphabetic numeral systems originated with Greek numerals around 600 BC and became largely extinct by the 16th century. After the development of positional numeral systems like Hindu–Arabic numerals, the use of alphabetic numeral systems dwindled to predominantly ordered lists, pagination, religious functions, and divinatory magic.
Undecimal is a positional numeral system that uses eleven as its base. While no known society counts by elevens, two are purported to have done so: the Māori and the Pañgwa. The idea of counting by elevens remains of interest for its relation to a traditional method of tally-counting practiced in Polynesia. During the French Revolution, undecimal was briefly considered as a possible basis for the reformed system of measurement. Undecimal numerals have applications in computer science, technology, and the International Standard Book Number system. They also occasionally feature in works of popular fiction. In undecimal, a capital letter or the digit ↊ is typically used as a transdecimal symbol to represent the number 10.








