In geometry, the gyrate bidiminished rhombicosidodecahedron is one of the Johnson solids (J82). It can be produced by removing two pentagonal cupolae and rotating a third pentagonal cupola through 36 degrees.

Geometry is a branch of mathematics concerned with questions of shape, size, relative position of figures, and the properties of space. A mathematician who works in the field of geometry is called a geometer.

In geometry, a Johnson solid is a strictly convex polyhedron, which is not uniform, and each face of which is a regular polygon. There is no requirement that each face must be the same polygon, or that the same polygons join around each vertex. An example of a Johnson solid is the square-based pyramid with equilateral sides (J1); it has 1 square face and 4 triangular faces.

In geometry, the pentagonal cupola is one of the Johnson solids (J5). It can be obtained as a slice of the rhombicosidodecahedron. The pentagonal cupola consists of 5 equilateral triangles, 5 squares, 1 pentagon, and 1 decagon.
A Johnson solid is one of 92 strictly convex polyhedra that have regular faces but are not uniform (that is, they are not Platonic solids, Archimedean solids, prisms, or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966. [1]

In geometry, a polyhedron is a solid in three dimensions with flat polygonal faces, straight edges and sharp corners or vertices. The word polyhedron comes from the Classical Greek πολύεδρον, as poly- + -hedron.
A regular polyhedron is a polyhedron whose symmetry group acts transitively on its flags. A regular polyhedron is highly symmetrical, being all of edge-transitive, vertex-transitive and face-transitive. In classical contexts, many different equivalent definitions are used; a common one is that faces are congruent regular polygons which are assembled in the same way around each vertex.
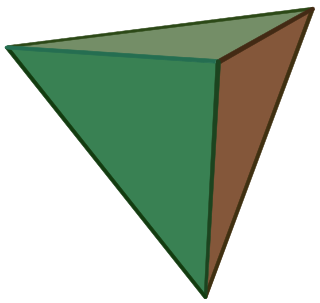
A uniform polyhedron is a polyhedron which has regular polygons as faces and is vertex-transitive. It follows that all vertices are congruent.
This page is based on this
Wikipedia article Text is available under the
CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.
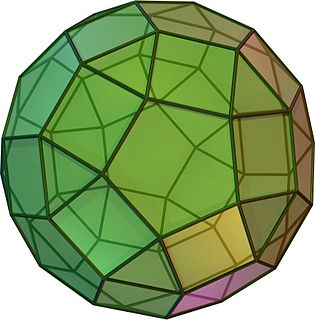
In geometry, the rhombicosidodecahedron, is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed of two or more types of regular polygon faces.

In geometry, the elongated square gyrobicupola or pseudorhombicuboctahedron is one of the Johnson solids (J37). It is not usually considered to be an Archimedean solid, even though its faces consist of regular polygons that meet in the same pattern at each of its vertices, because unlike the 13 Archimedean solids, it lacks a set of global symmetries that take every vertex to every other vertex. It strongly resembles, but should not be mistaken for, the small rhombicuboctahedron, which is an Archimedean solid.

In geometry, the diminished rhombicosidodecahedron is one of the
Johnson solids (J76).
It can be constructed as a rhombicosidodecahedron with one pentagonal cupola removed.

In geometry, the gyrate rhombicosidodecahedron is one of the
Johnson solids (J72).

In geometry, the parabidiminished rhombicosidodecahedron is one of the
Johnson solids (J80).

In geometry, the metabidiminished rhombicosidodecahedron is one of the
Johnson solids (J81).

In geometry, the tridiminished rhombicosidodecahedron is one of the Johnson solids (J83). It can be constructed as a rhombicosidodecahedron with three pentagonal cupolae removed.

In geometry, the trigyrate rhombicosidodecahedron is one of the Johnson solids (J75). It contains 20 triangles, 30 squares and 12 pentagons.
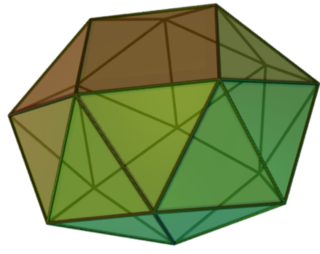
In geometry, the snub square antiprism is one of the Johnson solids (J85).
A Johnson solid is one of 92 strictly convex polyhedra that have regular faces but are not uniform. They were named by Norman Johnson, who first listed these polyhedra in 1966.
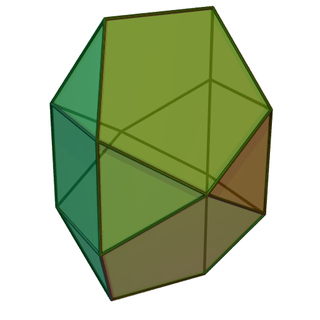
In geometry, the bilunabirotunda is one of the Johnson solids (J91).

In geometry, the pentagonal gyrobicupola is one of the Johnson solids (J31). Like the pentagonal orthobicupola (J30), it can be obtained by joining two pentagonal cupolae (J5) along their bases. The difference is that in this solid, the two halves are rotated 36 degrees with respect to one another.

In geometry, the biaugmented pentagonal prism is one of the Johnson solids (J53). As the name suggests, it can be constructed by doubly augmenting a pentagonal prism by attaching square pyramids (J1) to two of its nonadjacent equatorial faces.

In geometry, the parabigyrate rhombicosidodecahedron is one of the Johnson solids (J73). It can be constructed as a rhombicosidodecahedron with two opposing pentagonal cupolae rotated through 36 degrees.

In geometry, the paragyrate diminished rhombicosidodecahedron is one of the Johnson solids (J77). It can be constructed as a rhombicosidodecahedron with one pentagonal cupola rotated through 36 degrees, and the opposing pentagonal cupola removed.
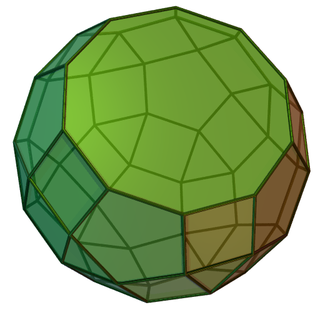
In geometry, the metagyrate diminished rhombicosidodecahedron is one of the Johnson solids (J78). It can be constructed as a rhombicosidodecahedron with one pentagonal cupola rotated through 36 degrees, and a non-opposing pentagonal cupola removed.

In geometry, the bigyrate diminished rhombicosidodecahedron is one of the Johnson solids (J79). It can be constructed as a rhombicosidodecahedron with two pentagonal cupolae rotated through 36 degrees, and a third pentagonal cupola removed.
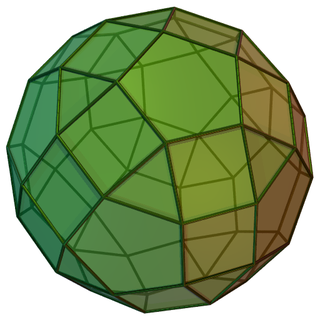
In geometry, the metabigyrate rhombicosidodecahedron is one of the Johnson solids (J74). It can be constructed as a rhombicosidodecahedron with two non-opposing pentagonal cupolae rotated through 36 degrees.
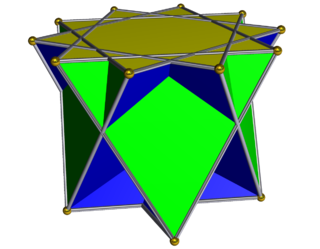
In geometry, the crossed pentagrammic cupola is one of the nonconvex Johnson solid isomorphs, being topologically identical to the convex pentagonal cupola. It can be obtained as a slice of the great rhombicosidodecahedron or quasirhombicosidodecahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; in this case the base polygon is a decagram.























