| Sphenocorona | |
|---|---|
 | |
| Type | Johnson J85 – J86 – J87 |
| Faces | 12 triangles 2 squares |
| Edges | 22 |
| Vertices | 10 |
| Vertex configuration | 4(33.4) 2(32.42) 2x2(35) |
| Symmetry group | C2v |
| Dual polyhedron | - |
| Properties | convex |
| Net | |
 | |
In geometry, the sphenocorona is a Johnson solid with 12 equilateral triangles and 2 squares as its faces.
The sphenocorona was named by Johnson (1966) in which he used the prefix spheno- referring to a wedge-like complex formed by two adjacent lunes —a square with equilateral triangles attached on its opposite sides. The suffix -corona refers to a crownlike complex of 8 equilateral triangles. [1] By joining both complexes together, the resulting polyhedron has 12 equilateral triangles and 2 squares, making 14 faces. [2] A convex polyhedron in which all faces are regular polygons is called a Johnson solid, and the sphenocorona is among them, enumerated as the 86th Johnson solid . [3] It is elementary, meaning it does not arise from "cut-and-paste" manipulations of both Platonic and Archimedean solids. [4]
The surface area of a sphenocorona with edge length can be calculated as: [2]
Let be the smallest positive root of the quartic polynomial . Then, Cartesian coordinates of a sphenocorona with edge length 2 are given by the union of the orbits of the points
under the action of the group generated by reflections about the xz-plane and the yz-plane. [5]
The sphenocorona is also the vertex figure of the isogonal n-gonal double antiprismoid where n is an odd number greater than one, including the grand antiprism with pairs of trapezoid rather than square faces.
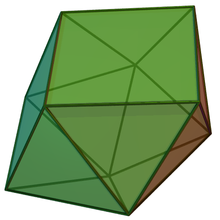
In geometry, an octahedron is a polyhedron with eight faces. The term is most commonly used to refer to the regular octahedron, a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.
In geometry, the snub cube, or snub cuboctahedron, is an Archimedean solid with 38 faces: 6 squares and 32 equilateral triangles. It has 60 edges and 24 vertices.
In geometry, the triakis icosahedron is an Archimedean dual solid, or a Catalan solid, with 60 isosceles triangle faces. Its dual is the truncated dodecahedron. It has also been called the kisicosahedron. It was first depicted, in a non-convex form with equilateral triangle faces, by Leonardo da Vinci in Luca Pacioli's Divina proportione, where it was named the icosahedron elevatum. The capsid of the Hepatitis A virus has the shape of a triakis icosahedron.
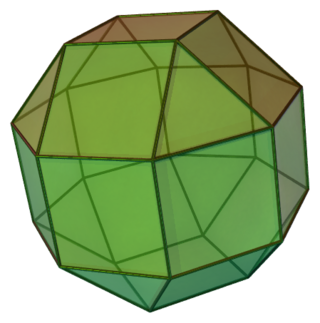
In geometry, the elongated square gyrobicupola is a polyhedron constructed by two square cupolas attaching onto the bases of octagonal prism, with one of them rotated. It is also known as pseudo-rhombicuboctahedron because many mathematicians mistakenly constructed a rhombicuboctahedron. It is not considered to be an Archimedean solid because it lacks a set of global symmetries that map every vertex to every other vertex, unlike the 13 Archimedean solids. It is also a canonical polyhedron.
In geometry, the pentagonal cupola is one of the Johnson solids. It can be obtained as a slice of the rhombicosidodecahedron. The pentagonal cupola consists of 5 equilateral triangles, 5 squares, 1 pentagon, and 1 decagon.
In geometry, the gyrate rhombicosidodecahedron is one of the Johnson solids. It is also a canonical polyhedron.
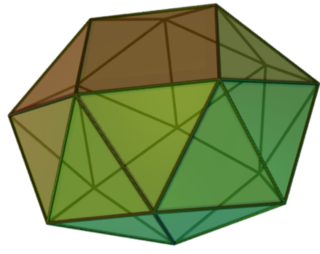
In geometry, the snub square antiprism is the Johnson solid that can be constructed by snubbing the square antiprism. It is one of the elementary Johnson solids that do not arise from "cut and paste" manipulations of the Platonic and Archimedean solids, although it is a relative of the icosahedron that has fourfold symmetry instead of threefold.
In geometry, the hebesphenomegacorona is a Johnson solid with 18 equilateral triangles and 3 squares as its faces.
In geometry, the sphenomegacorona is a Johnson solid with 16 equilateral triangles and 2 squares as its faces.
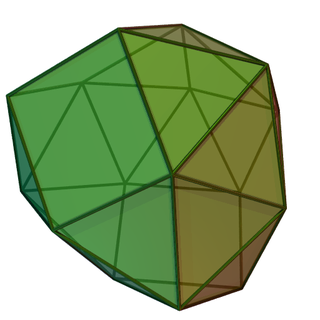
In geometry, the disphenocingulum is a Johnson solid with 20 equilateral triangles and 4 squares as its faces.
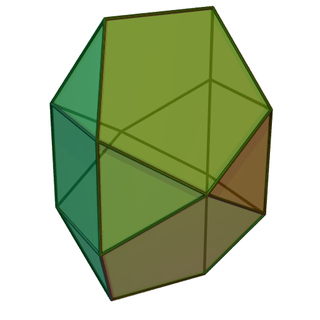
In geometry, the bilunabirotunda is a Johnson solid with faces of 8 equilateral triangles, 2 squares, and 4 regular pentagons.
In geometry, the triangular hebesphenorotunda is a Johnson solid with 13 equilateral triangles, 3 squares, 3 regular pentagons, and 1 regular hexagon, making the total of its faces is 20.
In geometry, the augmented sphenocorona is the Johnson solid that can be constructed by attaching an equilateral square pyramid to one of the square faces of the sphenocorona. It is the only Johnson solid arising from "cut and paste" manipulations where the components are not all prisms, antiprisms or sections of Platonic or Archimedean solids.
In geometry, the elongated square pyramid is a convex polyhedron constructed from a cube by attaching an equilateral square pyramid onto one of its faces. It is an example of Johnson solid. It is topologically self-dual.
In geometry, the elongated triangular bipyramid or triakis triangular prism is one of the Johnson solids, convex polyhedra whose faces are regular polygons. As the name suggests, it can be constructed by elongating a triangular bipyramid by inserting a triangular prism between its congruent halves.
In geometry, the gyrobifastigium is the 26th Johnson solid. It can be constructed by joining two face-regular triangular prisms along corresponding square faces, giving a quarter-turn to one prism. It is the only Johnson solid that can tile three-dimensional space.
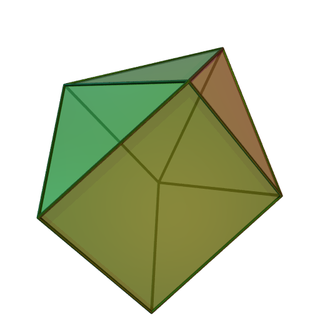
In geometry, the augmented triangular prism is a polyhedron constructed by attaching an equilateral square pyramid onto the square face of a triangular prism. As a result, it is an example of Johnson solid. It can be visualized as the chemical compound, known as capped trigonal prismatic molecular geometry.
In geometry, the biaugmented triangular prism is a polyhedron constructed from a triangular prism by attaching two equilateral square pyramids onto two of its square faces. It is an example of Johnson solid.
In geometry, the augmented pentagonal prism is a polyhedron that can be constructed by attaching an equilateral square pyramid onto the square face of pentagonal prism. It is an example of Johnson solid.
In geometry, chamfering or edge-truncation is a topological operator that modifies one polyhedron into another. It is similar to expansion: it moves the faces apart (outward), and adds a new face between each two adjacent faces; but contrary to expansion, it maintains the original vertices. For a polyhedron, this operation adds a new hexagonal face in place of each original edge.
